Bombieri–Vinogradov theorem
Encyclopedia
In mathematics
, the Bombieri–Vinogradov theorem (sometimes simply called Bombieri's theorem) is a major result of analytic number theory
, obtained in the mid-1960s. The first result of this kind was obtained by Barban in 1961 and the Bombieri–Vinogradov theorem is a refinement of Barban's result. The Bombieri-Vinogradov theorem is named after Enrico Bombieri
and A. I. Vinogradov, who published on a related topic, the density hypothesis, in 1965. Perhaps somewhat unjustly, Barban's name is often forgotten.
This result is a major application of the large sieve method, which developed rapidly in the early 1960s, from its beginnings in work of Yuri Linnik
two decades earlier. Besides Bombieri, Klaus Roth
was working in this area.
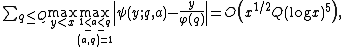
if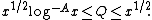
Here φ(q) is the Euler totient function, which is the number of summands for the modulus q, and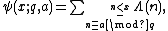
where
denotes the von Mangoldt function
.
A verbal description of this result is that it addresses the error term in the prime number theorem for arithmetic progressions
, averaged over the moduli q up to Q. For a certain range of Q, which are around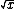
if we neglect logarithmic factors, the error averaged is nearly as small as 
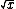. This is quite unobvious, and without the averaging is about of the strength of the Generalized Riemann Hypothesis
(GRH).
Mathematics
Mathematics is the study of quantity, space, structure, and change. Mathematicians seek out patterns and formulate new conjectures. Mathematicians resolve the truth or falsity of conjectures by mathematical proofs, which are arguments sufficient to convince other mathematicians of their validity...
, the Bombieri–Vinogradov theorem (sometimes simply called Bombieri's theorem) is a major result of analytic number theory
Analytic number theory
In mathematics, analytic number theory is a branch of number theory that uses methods from mathematical analysis to solve problems about the integers. It is often said to have begun with Dirichlet's introduction of Dirichlet L-functions to give the first proof of Dirichlet's theorem on arithmetic...
, obtained in the mid-1960s. The first result of this kind was obtained by Barban in 1961 and the Bombieri–Vinogradov theorem is a refinement of Barban's result. The Bombieri-Vinogradov theorem is named after Enrico Bombieri
Enrico Bombieri
Enrico Bombieri is a mathematician who has been working at the Institute for Advanced Study in Princeton, New Jersey. Bombieri's research in number theory, algebraic geometry, and mathematical analysis have earned him many international prizes --- a Fields Medal in 1974 and the Balzan Prize in 1980...
and A. I. Vinogradov, who published on a related topic, the density hypothesis, in 1965. Perhaps somewhat unjustly, Barban's name is often forgotten.
This result is a major application of the large sieve method, which developed rapidly in the early 1960s, from its beginnings in work of Yuri Linnik
Yuri Linnik
Yuri Vladimirovich Linnik was a Soviet mathematician active in number theory, probability theory and mathematical statistics.Linnik was born in Bila Tserkva, in present-day Ukraine. He went to St Petersburg University where his supervisor was Vladimir Tartakovski, and later worked at that...
two decades earlier. Besides Bombieri, Klaus Roth
Klaus Roth
Klaus Friedrich Roth is a British mathematician known for work on diophantine approximation, the large sieve, and irregularities of distribution. He was born in Breslau, Prussia, but raised and educated in the UK. He graduated from Peterhouse, Cambridge in 1945...
was working in this area.
Statement of the Bombieri–Vinogradov theorem
Let A be any positive real number. Then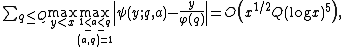if
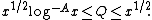
Here φ(q) is the Euler totient function, which is the number of summands for the modulus q, and
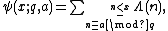
where
denotes the von Mangoldt functionVon Mangoldt function
In mathematics, the von Mangoldt function is an arithmetic function named after German mathematician Hans von Mangoldt.-Definition:The von Mangoldt function, conventionally written as Λ, is defined as...
.
A verbal description of this result is that it addresses the error term in the prime number theorem for arithmetic progressions
Prime number theorem
In number theory, the prime number theorem describes the asymptotic distribution of the prime numbers. The prime number theorem gives a general description of how the primes are distributed amongst the positive integers....
, averaged over the moduli q up to Q. For a certain range of Q, which are around
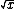if we neglect logarithmic factors, the error averaged is nearly as small as 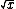. This is quite unobvious, and without the averaging is about of the strength of the Generalized Riemann HypothesisGeneralized Riemann hypothesis
The Riemann hypothesis is one of the most important conjectures in mathematics. It is a statement about the zeros of the Riemann zeta function. Various geometrical and arithmetical objects can be described by so-called global L-functions, which are formally similar to the Riemann zeta-function...
(GRH).