Boundary (topology) - AbsoluteAstronomy.com

Topology

Topology is a major area of mathematics concerned with properties that are preserved under continuous deformations of objects, such as deformations that involve stretching, but no tearing or gluing...

and mathematics

Mathematics

Mathematics is the study of quantity, space, structure, and change. Mathematicians seek out patterns and formulate new conjectures. Mathematicians resolve the truth or falsity of conjectures by mathematical proofs, which are arguments sufficient to convince other mathematicians of their validity...

in general, the boundary of a subset S of a topological space

Topological space

Topological spaces are mathematical structures that allow the formal definition of concepts such as convergence, connectedness, and continuity. They appear in virtually every branch of modern mathematics and are a central unifying notion...

X is the set of points which can be approached both from S and from the outside of S. More precisely, it is the set of points in the closure

Closure (topology)

In mathematics, the closure of a subset S in a topological space consists of all points in S plus the limit points of S. Intuitively, these are all the points that are "near" S. A point which is in the closure of S is a point of closure of S...

of S, not belonging to the interior

Interior (topology)

In mathematics, specifically in topology, the interior of a set S of points of a topological space consists of all points of S that do not belong to the boundary of S. A point that is in the interior of S is an interior point of S....

of S. An element of the boundary of S is called a boundary point of S. Notations used for boundary of a set S include bd(S), fr(S), and ∂S. Some authors (for example Willard, in General Topology) use the term frontier, instead of boundary in an attempt to avoid confusion with the concept of boundary used in algebraic topology

Algebraic topology

Algebraic topology is a branch of mathematics which uses tools from abstract algebra to study topological spaces. The basic goal is to find algebraic invariants that classify topological spaces up to homeomorphism, though usually most classify up to homotopy equivalence.Although algebraic topology...

and manifold theory.

A connected component of the boundary of S is called a boundary component of S.

Common definitions

There are several common (and equivalent) definitions to the boundary of a subset S of a topological space X:

the closure of S without the interior of S: ∂S = S \ S^o.
the intersection of the closure of S with the closure of its complement
Complement (set theory)
In set theory, a complement of a set A refers to things not in , A. The relative complement of A with respect to a set B, is the set of elements in B but not in A...

: ∂S = S ∩ (X \ S).
the set of points p of X such that every neighborhood of p contains at least one point of S and at least one point not of S.

Examples

Consider the real line R with the usual topology (i.e. the topology whose basis sets are open intervals). One has

∂(0,5) = ∂[0,5) = ∂(0,5] = ∂[0,5] = {0,5}
∂∅ = ∅
∂Q = R
∂(Q ∩ [0,1]) = [0,1]

These last two examples illustrate the fact that the boundary of a dense set

Dense set

In topology and related areas of mathematics, a subset A of a topological space X is called dense if any point x in X belongs to A or is a limit point of A...

with empty interior is its closure.

In the space of rational numbers with the usual topology (the subspace topology

Subspace topology

In topology and related areas of mathematics, a subspace of a topological space X is a subset S of X which is equipped with a natural topology induced from that of X called the subspace topology .- Definition :Given a topological space and a subset S of X, the...

of R), the boundary of

, where a is irrational, is empty.

The boundary of a set is a topological

Topology

notion and may change if one changes the topology. For example, given the usual topology on R², the boundary of a closed disk Ω = {(x,y) | x² + y² ≤ 1} is the disk's surrounding circle: ∂Ω = {(x,y) | x² + y² = 1}. If the disk is viewed as a set in R³ with its own usual topology, i.e. Ω = {(x,y,0) | x² + y² ≤ 1}, then the boundary of the disk is the disk itself: ∂Ω = Ω. If the disk is viewed as its own topological space (with the subspace topology of R²), then the boundary of the disk is empty.

Properties

The boundary of a set is closed
Closed set
In geometry, topology, and related branches of mathematics, a closed set is a set whose complement is an open set. In a topological space, a closed set can be defined as a set which contains all its limit points...

.
The boundary of a set is the boundary of the complement of the set: ∂S = ∂(S^C).

Hence:

p is a boundary point of a set if and only if every neighborhood of p contains at least one point in the set and at least one point not in the set.
A set is closed if and only if it contains its boundary, and open
Open set
The concept of an open set is fundamental to many areas of mathematics, especially point-set topology and metric topology. Intuitively speaking, a set U is open if any point x in U can be "moved" a small amount in any direction and still be in the set U...

if and only if it is disjoint from its boundary.
The closure of a set equals the union of the set with its boundary. S = S ∪ ∂S.
The boundary of a set is empty if and only if the set is both closed and open (that is, a clopen set
Clopen set
In topology, a clopen set in a topological space is a set which is both open and closed. That this is possible for a set is not as counter-intuitive as it might seem if the terms open and closed were thought of as antonyms; in fact they are not...

).
In Rⁿ, every closed set is the boundary of some set.

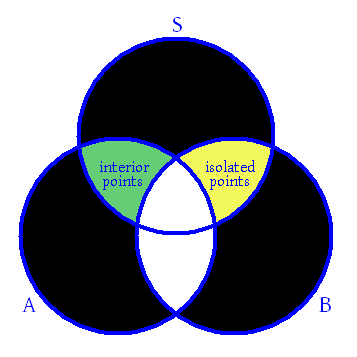
Concept

The word concept is used in ordinary language as well as in almost all academic disciplines. Particularly in philosophy, psychology and cognitive sciences the term is much used and much discussed. WordNet defines concept: "conception, construct ". However, the meaning of the term concept is much...

ual Venn diagram

Venn diagram

Venn diagrams or set diagrams are diagrams that show all possible logical relations between a finite collection of sets . Venn diagrams were conceived around 1880 by John Venn...

showing the relationships among different points of a subset S of Rⁿ. A = set of limit point

Limit point

In mathematics, a limit point of a set S in a topological space X is a point x in X that can be "approximated" by points of S in the sense that every neighbourhood of x with respect to the topology on X also contains a point of S other than x itself. Note that x does not have to be an element of S...

s of S, B = set of boundary points of S, area shaded green = set of interior points of S, area shaded yellow = set of isolated point

Isolated point

In topology, a branch of mathematics, a point x of a set S is called an isolated point of S, if there exists a neighborhood of x not containing other points of S.In particular, in a Euclidean space ,...

s of S, areas shaded black = empty sets. Every point of S is either an interior point or a boundary point. Also, every point of S is either an accumulation point or an isolated point. Likewise, every boundary point of S is either an accumulation point or an isolated point. Isolated points are always boundary points.

Boundary of a boundary

For any set S, ∂S ⊇ ∂∂S, with equality holding if and only if the boundary of S has no interior points. This is always true if S is either closed or open. Since the boundary of any set is closed, ∂∂S = ∂∂∂S for any set S. The boundary operator thus satisfies a weakened kind of idempotence

Idempotence

Idempotence is the property of certain operations in mathematics and computer science, that they can be applied multiple times without changing the result beyond the initial application...

.

In discussing boundaries of manifold

Manifold

In mathematics , a manifold is a topological space that on a small enough scale resembles the Euclidean space of a specific dimension, called the dimension of the manifold....

s or simplex

Simplex

In geometry, a simplex is a generalization of the notion of a triangle or tetrahedron to arbitrary dimension. Specifically, an n-simplex is an n-dimensional polytope which is the convex hull of its n + 1 vertices. For example, a 2-simplex is a triangle, a 3-simplex is a tetrahedron,...

es and their simplicial complex

Simplicial complex

In mathematics, a simplicial complex is a topological space of a certain kind, constructed by "gluing together" points, line segments, triangles, and their n-dimensional counterparts...

es, one often meets the assertion that the boundary of the boundary is always empty. Indeed, the construction of the singular homology

Singular homology

In algebraic topology, a branch of mathematics, singular homology refers to the study of a certain set of algebraic invariants of a topological space X, the so-called homology groups H_n....

rests critically on this fact. The explanation for the apparent incongruity is that the topological boundary (the subject of this article) is a slightly different concept than the boundary of a manifold or of a simplicial complex. For example, the topological boundary of a closed disk viewed as a topological space is empty, while its boundary in the sense of manifolds is the circle surrounding the disk.

Common definitions

Examples

Properties

Boundary of a boundary

See also