
Bragg plane
Encyclopedia
In physics
, the Bragg plane is the plane
in reciprocal space which bisects the reciprocal lattice vector . It is relevant to define this plane as part of the definition of the Von Laue condition for diffraction peaks in x-ray diffraction crystallography
. It is relevant to define this plane as part of the definition of the Von Laue condition for diffraction peaks in x-ray diffraction crystallography
.
Considering the diagram at right, the arriving x-ray
plane wave
is defined by:

Where is the incident wave vector given by:
is the incident wave vector given by:
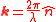
...where is the wavelength
is the wavelength
of the incident photon
. While the Bragg formulation
assumes a unique choice of direct lattice planes and specular reflection
of the incident X-rays, the Von Laue formula only assumes monochromatic light and that each scattering center acts as a source of secondary wavelets as described by the Huygens principle. Each scattered wave contributes to a new plane wave given by:
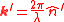
The condition for constructive interference in the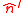 direction is that the path difference between the photons is an integer multiple (m) of their wavelength. We know then that for constructive interference we have:
direction is that the path difference between the photons is an integer multiple (m) of their wavelength. We know then that for constructive interference we have:
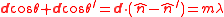
..where . Multiplying the above by
. Multiplying the above by 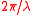 we formulate the condition in terms of the wave vectors
we formulate the condition in terms of the wave vectors  and
and 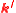 :
:
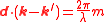
Now consider that a crystal is an array of scatterering centres, each at a point in the Bravais lattice. We can set one of the scattering centres as the origin of an array. Since the lattice points are displaced by the Bravais lattive vectors , scattered waves interfere constructively when the above condition holds simultaneously for all values of
, scattered waves interfere constructively when the above condition holds simultaneously for all values of  which are Bravais lattice vectors, the condition then becomes:
which are Bravais lattice vectors, the condition then becomes:
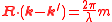
An equivalent statement (see mathematical description of the reciprocal lattice) is to say that:

By comparing this equation with the definition of a reciprocal lattice vector, we see that constructive interference occurs if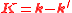 is a vector of the reciprocal lattice. We notice that
is a vector of the reciprocal lattice. We notice that  and
and 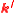 have the same magnitude, we can restate the Von Laue formulation as requiring that the tip of incident wave vector
have the same magnitude, we can restate the Von Laue formulation as requiring that the tip of incident wave vector  must lie in the plane that is a perpendicular bisector of the reciprocal lattice vector
must lie in the plane that is a perpendicular bisector of the reciprocal lattice vector  . This reciprocal space plane is the Bragg plane.
. This reciprocal space plane is the Bragg plane.
Physics
Physics is a natural science that involves the study of matter and its motion through spacetime, along with related concepts such as energy and force. More broadly, it is the general analysis of nature, conducted in order to understand how the universe behaves.Physics is one of the oldest academic...
, the Bragg plane is the plane
Plane
-Physical objects:* Aeroplane or airplane, a fixed-wing aircraft* Plane , a woodworking tool to smooth surfaces* Platanus, a genus of trees with the common name "plane"* Acer pseudoplatanus, a tree species sometimes called "plane"...
in reciprocal space which bisects the reciprocal lattice vector
 . It is relevant to define this plane as part of the definition of the Von Laue condition for diffraction peaks in x-ray diffraction crystallography
. It is relevant to define this plane as part of the definition of the Von Laue condition for diffraction peaks in x-ray diffraction crystallographyX-ray crystallography
X-ray crystallography is a method of determining the arrangement of atoms within a crystal, in which a beam of X-rays strikes a crystal and causes the beam of light to spread into many specific directions. From the angles and intensities of these diffracted beams, a crystallographer can produce a...
.
Considering the diagram at right, the arriving x-ray
X-ray
X-radiation is a form of electromagnetic radiation. X-rays have a wavelength in the range of 0.01 to 10 nanometers, corresponding to frequencies in the range 30 petahertz to 30 exahertz and energies in the range 120 eV to 120 keV. They are shorter in wavelength than UV rays and longer than gamma...
plane wave
Plane wave
In the physics of wave propagation, a plane wave is a constant-frequency wave whose wavefronts are infinite parallel planes of constant peak-to-peak amplitude normal to the phase velocity vector....
is defined by:

Where
 is the incident wave vector given by:
is the incident wave vector given by: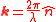
...where
 is the wavelength
is the wavelengthWavelength
In physics, the wavelength of a sinusoidal wave is the spatial period of the wave—the distance over which the wave's shape repeats.It is usually determined by considering the distance between consecutive corresponding points of the same phase, such as crests, troughs, or zero crossings, and is a...
of the incident photon
Photon
In physics, a photon is an elementary particle, the quantum of the electromagnetic interaction and the basic unit of light and all other forms of electromagnetic radiation. It is also the force carrier for the electromagnetic force...
. While the Bragg formulation
Bragg's law
In physics, Bragg's law gives the angles for coherent and incoherent scattering from a crystal lattice. When X-rays are incident on an atom, they make the electronic cloud move as does any electromagnetic wave...
assumes a unique choice of direct lattice planes and specular reflection
Specular reflection
Specular reflection is the mirror-like reflection of light from a surface, in which light from a single incoming direction is reflected into a single outgoing direction...
of the incident X-rays, the Von Laue formula only assumes monochromatic light and that each scattering center acts as a source of secondary wavelets as described by the Huygens principle. Each scattered wave contributes to a new plane wave given by:
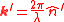
The condition for constructive interference in the
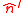 direction is that the path difference between the photons is an integer multiple (m) of their wavelength. We know then that for constructive interference we have:
direction is that the path difference between the photons is an integer multiple (m) of their wavelength. We know then that for constructive interference we have: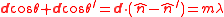
..where
 . Multiplying the above by
. Multiplying the above by 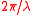 we formulate the condition in terms of the wave vectors
we formulate the condition in terms of the wave vectors  and
and 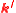 :
: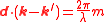
Now consider that a crystal is an array of scatterering centres, each at a point in the Bravais lattice. We can set one of the scattering centres as the origin of an array. Since the lattice points are displaced by the Bravais lattive vectors
 , scattered waves interfere constructively when the above condition holds simultaneously for all values of
, scattered waves interfere constructively when the above condition holds simultaneously for all values of  which are Bravais lattice vectors, the condition then becomes:
which are Bravais lattice vectors, the condition then becomes: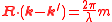
An equivalent statement (see mathematical description of the reciprocal lattice) is to say that:

By comparing this equation with the definition of a reciprocal lattice vector, we see that constructive interference occurs if
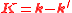 is a vector of the reciprocal lattice. We notice that
is a vector of the reciprocal lattice. We notice that  and
and 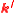 have the same magnitude, we can restate the Von Laue formulation as requiring that the tip of incident wave vector
have the same magnitude, we can restate the Von Laue formulation as requiring that the tip of incident wave vector  must lie in the plane that is a perpendicular bisector of the reciprocal lattice vector
must lie in the plane that is a perpendicular bisector of the reciprocal lattice vector  . This reciprocal space plane is the Bragg plane.
. This reciprocal space plane is the Bragg plane.See also
- X-ray crystallographyX-ray crystallographyX-ray crystallography is a method of determining the arrangement of atoms within a crystal, in which a beam of X-rays strikes a crystal and causes the beam of light to spread into many specific directions. From the angles and intensities of these diffracted beams, a crystallographer can produce a...
- Reciprocal latticeReciprocal latticeIn physics, the reciprocal lattice of a lattice is the lattice in which the Fourier transform of the spatial function of the original lattice is represented. This space is also known as momentum space or less commonly k-space, due to the relationship between the Pontryagin duals momentum and...
- Bravais lattice
- Powder diffractionPowder diffractionPowder diffraction is a scientific technique using X-ray, neutron, or electron diffraction on powder or microcrystalline samples for structural characterization of materials.-Explanation:...
- Kikuchi lineKikuchi lineKikuchi lines pair up to form bands in electron diffraction from single crystal specimens, there to serve as "roads in orientation-space" for microscopists not sure what they are looking at. In transmission electron microscopes, they are easily seen in diffraction from regions of the specimen thick...
- Brillouin zoneBrillouin zoneIn mathematics and solid state physics, the first Brillouin zone is a uniquely defined primitive cell in reciprocal space. The boundaries of this cell are given by planes related to points on the reciprocal lattice. It is found by the same method as for the Wigner–Seitz cell in the Bravais lattice...

