A central angle is an angle

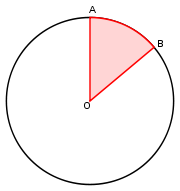
Angle

In geometry, an angle is the figure formed by two rays sharing a common endpoint, called the vertex of the angle.Angles are usually presumed to be in a Euclidean plane with the circle taken for standard with regard to direction. In fact, an angle is frequently viewed as a measure of an circular arc...

 which vertex is the center of a circle

Circle

A circle is a simple shape of Euclidean geometry consisting of those points in a plane that are a given distance from a given point, the centre. The distance between any of the points and the centre is called the radius....

, and whose sides pass through a pair of points on the circle, thereby subtending an arc

Arc (geometry)

In geometry, an arc is a closed segment of a differentiable curve in the two-dimensional plane; for example, a circular arc is a segment of the circumference of a circle...

 between those two points whose angle is (by definition) equal to the central angle itself. It is also known as the arc segment

Line segment

In geometry, a line segment is a part of a line that is bounded by two end points, and contains every point on the line between its end points. Examples of line segments include the sides of a triangle or square. More generally, when the end points are both vertices of a polygon, the line segment...

's angular distance

Angular distance

In mathematics and all natural sciences , the angular distance between two point objects, as observed from a location different from either of these objects, is the size of the angle between the two directions originating from...

.

Coordinates

On a sphere

Sphere

A sphere is a perfectly round geometrical object in three-dimensional space, such as the shape of a round ball. Like a circle in two dimensions, a perfect sphere is completely symmetrical around its center, with all points on the surface lying the same distance r from the center point...

 or ellipsoid, the central angle is delineated along a great circle

Great circle

A great circle, also known as a Riemannian circle, of a sphere is the intersection of the sphere and a plane which passes through the center point of the sphere, as opposed to a general circle of a sphere where the plane is not required to pass through the center...

.
The usually provided coordinates of a point on a sphere/ellipsoid is its conjugate latitude

Latitude

In geography, the latitude of a location on the Earth is the angular distance of that location south or north of the Equator. The latitude is an angle, and is usually measured in degrees . The equator has a latitude of 0°, the North pole has a latitude of 90° north , and the South pole has a...

 ("Lat"), , and longitude

Longitude

Longitude is a geographic coordinate that specifies the east-west position of a point on the Earth's surface. It is an angular measurement, usually expressed in degrees, minutes and seconds, and denoted by the Greek letter lambda ....

 ("Long"), . The "point", , is actually—relative to the great circle it is being measured on—the transverse colatitude ("TvL"), and the central angle/angular distance is the difference between two TvLs, .

Calculation of TvL

The calculation of and can be found using a common subroutine:

 

  _____________________________________________________________________
  ¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯

Each point has at least two values, both a forward and reverse value.

Occupying great circle

The arc path, , tracing the great circle that a central angle occupies, is measured as that great circle's azimuth at the equator, introducing an important property of spherical geometry, Clairaut's constant

Clairaut's theorem

Clairaut's theorem, published in 1743 by Alexis Claude Clairaut in his Théorie de la figure de la terre, tirée des principes de l'hydrostatique, synthesized physical and geodetic evidence that the Earth is an oblate rotational ellipsoid. It is a general mathematical law applying to spheroids of...

:

From this and relationships to ,

Angular distance formulary

The angular distance can be calculated either directly as the TvL difference, or via the common coordinates (here, either SA_w, SB_w value set can be used):


and, using half-angles,

   

It can, as well, be found by means of finding the chord length via Cartesian subtraction

Cartesian coordinate system

A Cartesian coordinate system specifies each point uniquely in a plane by a pair of numerical coordinates, which are the signed distances from the point to two fixed perpendicular directed lines, measured in the same unit of length...

:


Also, by using Cartesian products rather than differences, the origin of the spherical cosine for sides becomes apparent:



There is also a logarithmical form:

External links

• Central Angle of an Arc definition With interactive animation
• Central Angle Theorem described With interactive animation
• Inscribed and Central Angles in a Circle

The source of this article is wikipedia, the free encyclopedia.  The text of this article is licensed under the GFDL.