
Compacton
Encyclopedia
In the theory of integrable systems, a compacton, introduced in , is a soliton
with compact support.
An example of an equation with compacton solutions is the generalization
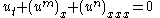
of the Korteweg–de Vries equation
with m, n > 1. (The case when m = 2, n = 1 is essentially the KdV equation.)
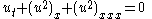
has a travelling wave solution given by
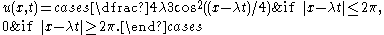
This has compact support in x, so is a compacton.
Soliton
In mathematics and physics, a soliton is a self-reinforcing solitary wave that maintains its shape while it travels at constant speed. Solitons are caused by a cancellation of nonlinear and dispersive effects in the medium...
with compact support.
An example of an equation with compacton solutions is the generalization
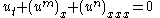
of the Korteweg–de Vries equation
Korteweg–de Vries equation
In mathematics, the Korteweg–de Vries equation is a mathematical model of waves on shallow water surfaces. It is particularly notable as the prototypical example of an exactly solvable model, that is, a non-linear partial differential equation whose solutions can be exactly and precisely specified...
with m, n > 1. (The case when m = 2, n = 1 is essentially the KdV equation.)
Example
The equation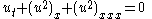
has a travelling wave solution given by
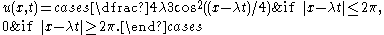
This has compact support in x, so is a compacton.

