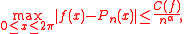Constructive function theory
Encyclopedia
In mathematical analysis
, constructive function theory is a field which studies the connection between the smoothness of a function
and its degree of approximation
. It is closely related to approximation theory
. The term was coined by Sergei Bernstein.
for some 0 < α < 1 if and only if for every natural n there exists a trigonometric polynomial
Pn of degree n such that
where C(f) is a positive number depending on f. The "only if" is due to Dunham Jackson
, see Jackson's inequality
; the "if" part is due to Sergei Bernstein, see Bernstein's theorem (approximation theory)
.
Mathematical analysis
Mathematical analysis, which mathematicians refer to simply as analysis, has its beginnings in the rigorous formulation of infinitesimal calculus. It is a branch of pure mathematics that includes the theories of differentiation, integration and measure, limits, infinite series, and analytic functions...
, constructive function theory is a field which studies the connection between the smoothness of a function
Function (mathematics)
In mathematics, a function associates one quantity, the argument of the function, also known as the input, with another quantity, the value of the function, also known as the output. A function assigns exactly one output to each input. The argument and the value may be real numbers, but they can...
and its degree of approximation
Approximation theory
In mathematics, approximation theory is concerned with how functions can best be approximated with simpler functions, and with quantitatively characterizing the errors introduced thereby...
. It is closely related to approximation theory
Approximation theory
In mathematics, approximation theory is concerned with how functions can best be approximated with simpler functions, and with quantitatively characterizing the errors introduced thereby...
. The term was coined by Sergei Bernstein.
Example
Let f be a 2π-periodic function. Then f is α-HölderHölder condition
In mathematics, a real or complex-valued function ƒ on d-dimensional Euclidean space satisfies a Hölder condition, or is Hölder continuous, when there are nonnegative real constants C, \alpha , such that...
for some 0 < α < 1 if and only if for every natural n there exists a trigonometric polynomial
Trigonometric polynomial
In the mathematical subfields of numerical analysis and mathematical analysis, a trigonometric polynomial is a finite linear combination of functions sin and cos with n a natural number. The coefficients may be taken as real numbers, for real-valued functions...
Pn of degree n such that
where C(f) is a positive number depending on f. The "only if" is due to Dunham Jackson
Dunham Jackson
Dunham Jackson was a mathematician who worked within approximation theory, notably with trigonometrical and orthogonal polynomials. He is known for Jackson's inequality. He was awarded the Chauvenet Prize in 1935...
, see Jackson's inequality
Jackson's inequality
In approximation theory, Jackson's inequality is an inequality bounding the value of function's best approximation by algebraic or trigonometric polynomials in terms of the modulus of continuity of its derivatives...
; the "if" part is due to Sergei Bernstein, see Bernstein's theorem (approximation theory)
Bernstein's theorem (approximation theory)
In approximation theory, Bernstein's theorem is a converse to Jackson's theorem. The first results of this type were proved by Sergei Bernstein in 1912.For approximation by trigonometric polynomials, the result is as follows:...
.