
Cotorsion group
Encyclopedia
In mathematics
, in the realm of abelian
group theory
, an abelian group
is said to be cotorsion if every extension of it by a torsion-free group splits. If the group is , this is equivalent to asserting that
, this is equivalent to asserting that 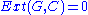 for all torsion-free groups
for all torsion-free groups  . It suffices to check the condition for
. It suffices to check the condition for  being the group of rational number
being the group of rational number
s.
Some properties of cotorsion groups:
Mathematics
Mathematics is the study of quantity, space, structure, and change. Mathematicians seek out patterns and formulate new conjectures. Mathematicians resolve the truth or falsity of conjectures by mathematical proofs, which are arguments sufficient to convince other mathematicians of their validity...
, in the realm of abelian
Abelian group
In abstract algebra, an abelian group, also called a commutative group, is a group in which the result of applying the group operation to two group elements does not depend on their order . Abelian groups generalize the arithmetic of addition of integers...
group theory
Group theory
In mathematics and abstract algebra, group theory studies the algebraic structures known as groups.The concept of a group is central to abstract algebra: other well-known algebraic structures, such as rings, fields, and vector spaces can all be seen as groups endowed with additional operations and...
, an abelian group
Group (mathematics)
In mathematics, a group is an algebraic structure consisting of a set together with an operation that combines any two of its elements to form a third element. To qualify as a group, the set and the operation must satisfy a few conditions called group axioms, namely closure, associativity, identity...
is said to be cotorsion if every extension of it by a torsion-free group splits. If the group is
 , this is equivalent to asserting that
, this is equivalent to asserting that 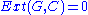 for all torsion-free groups
for all torsion-free groups  . It suffices to check the condition for
. It suffices to check the condition for  being the group of rational number
being the group of rational numberRational number
In mathematics, a rational number is any number that can be expressed as the quotient or fraction a/b of two integers, with the denominator b not equal to zero. Since b may be equal to 1, every integer is a rational number...
s.
Some properties of cotorsion groups:
- Any quotientQuotientIn mathematics, a quotient is the result of division. For example, when dividing 6 by 3, the quotient is 2, while 6 is called the dividend, and 3 the divisor. The quotient further is expressed as the number of times the divisor divides into the dividend e.g. The quotient of 6 and 2 is also 3.A...
of a cotorsion group is cotorsion. - A direct product of groupsDirect product of groupsIn the mathematical field of group theory, the direct product is an operation that takes two groups and and constructs a new group, usually denoted...
is cotorsion if and only ifIf and only ifIn logic and related fields such as mathematics and philosophy, if and only if is a biconditional logical connective between statements....
each factor is. - Every divisible groupDivisible groupIn mathematics, especially in the field of group theory, a divisible group is an abelian group in which every element can, in some sense, be divided by positive integers, or more accurately, every element is an nth multiple for each positive integer n...
or injective group is cotorsion. - The Baer Fomin Theorem states that a torsion group is cotorsion if and only if it is a direct sum of a divisible group and a bounded group, that is, a group of bounded exponent.
- A torsion-free abelian group is cotorsion if and only if it is algebraically compactAlgebraically compact groupIn mathematics, in the realm of abelian group theory, a group is said to be algebraically compact if it is a direct summand of every abelian group containing it as a pure subgroup.Equivalent characterizations of algebraic compactness:...
. - Ulm subgroups of cotorsion groups are cotorsion and Ulm factors of cotorsion groups are algebraically compact.

