
Craps principle
Encyclopedia
In probability theory
, the craps principle is a theorem about event
probabilities under repeated iid trials. Let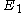 and
and  denote two mutually exclusive
denote two mutually exclusive
events which might occur on a given trial. Then for each trial, the conditional probability
that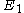 occurs given that
occurs given that 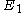 or
or 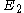 occur is
occur is
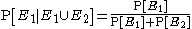
The events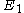 and
and 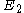 need not be collectively exhaustive
need not be collectively exhaustive
.
 and
and 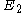 are mutually exclusive,
are mutually exclusive,

Also due to mutual exclusion,
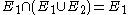
By conditional probability
,

Combining these three yields the desired result.
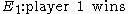
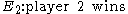
then the craps principle gives the respective conditional probabilities of each player winning a certain repetition, given that someone wins (i.e., given that a draw
does not occur). In fact, the result is only affected by the relative marginal probabilities of winning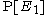 and
and 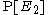 ; in particular, the probability of a draw is irrelevant.
; in particular, the probability of a draw is irrelevant.
, then this principle can greatly simplify the computation of the probability of winning in a certain scenario. Specifically, if the first roll is a 4, 5, 6, 8, 9, or 10, then the dice are repeatedly re-rolled until one of two events occurs:
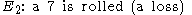
Since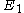 and
and 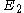 are mutually exclusive, the craps principle applies. For example, if the original roll was a 4, then the probability of winning is
are mutually exclusive, the craps principle applies. For example, if the original roll was a 4, then the probability of winning is
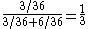
This avoids having to sum the infinite series corresponding to all the possible outcomes:
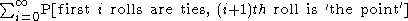
Mathematically, we can express the probability of rolling ties followed by rolling the point:
ties followed by rolling the point:
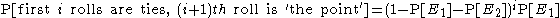
The summation becomes an infinite geometric series:

Probability theory
Probability theory is the branch of mathematics concerned with analysis of random phenomena. The central objects of probability theory are random variables, stochastic processes, and events: mathematical abstractions of non-deterministic events or measured quantities that may either be single...
, the craps principle is a theorem about event
Event (probability theory)
In probability theory, an event is a set of outcomes to which a probability is assigned. Typically, when the sample space is finite, any subset of the sample space is an event...
probabilities under repeated iid trials. Let
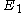 and
and  denote two mutually exclusive
denote two mutually exclusiveMutually exclusive
In layman's terms, two events are mutually exclusive if they cannot occur at the same time. An example is tossing a coin once, which can result in either heads or tails, but not both....
events which might occur on a given trial. Then for each trial, the conditional probability
Conditional probability
In probability theory, the "conditional probability of A given B" is the probability of A if B is known to occur. It is commonly notated P, and sometimes P_B. P can be visualised as the probability of event A when the sample space is restricted to event B...
that
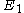 occurs given that
occurs given that 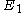 or
or 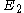 occur is
occur is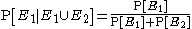
The events
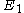 and
and 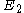 need not be collectively exhaustive
need not be collectively exhaustiveCollectively exhaustive
In probability theory, a set of events is jointly or collectively exhaustive if at least one of the events must occur. For example, when rolling a six-sided die, the outcomes 1, 2, 3, 4, 5, and 6 are collectively exhaustive, because they encompass the entire range of possible outcomes.Another way...
.
Proof
Since and
and 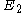 are mutually exclusive,
are mutually exclusive,
Also due to mutual exclusion,
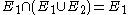
By conditional probability
Conditional probability
In probability theory, the "conditional probability of A given B" is the probability of A if B is known to occur. It is commonly notated P, and sometimes P_B. P can be visualised as the probability of event A when the sample space is restricted to event B...
,

Combining these three yields the desired result.
Application
If the trials are repetitions of a game between two players, and the events are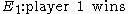
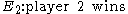
then the craps principle gives the respective conditional probabilities of each player winning a certain repetition, given that someone wins (i.e., given that a draw
Draw
Draw, draws or drawn may refer to:* The act of drawing, or making an image with a writing utensil* A part of many card games* A part of a lottery* Wire drawing...
does not occur). In fact, the result is only affected by the relative marginal probabilities of winning
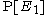 and
and 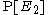 ; in particular, the probability of a draw is irrelevant.
; in particular, the probability of a draw is irrelevant.Stopping
If the game is played repeatedly until someone wins, then the conditional probability above turns out to be the probability that the player wins the game.Etymology
If the game being played is crapsCraps
Craps is a dice game in which players place wagers on the outcome of the roll, or a series of rolls, of a pair of dice. Players may wager money against each other or a bank...
, then this principle can greatly simplify the computation of the probability of winning in a certain scenario. Specifically, if the first roll is a 4, 5, 6, 8, 9, or 10, then the dice are repeatedly re-rolled until one of two events occurs:

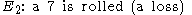
Since
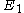 and
and 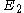 are mutually exclusive, the craps principle applies. For example, if the original roll was a 4, then the probability of winning is
are mutually exclusive, the craps principle applies. For example, if the original roll was a 4, then the probability of winning is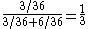
This avoids having to sum the infinite series corresponding to all the possible outcomes:
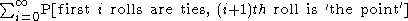
Mathematically, we can express the probability of rolling
 ties followed by rolling the point:
ties followed by rolling the point: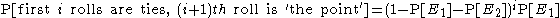
The summation becomes an infinite geometric series:

-
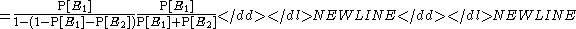
which agrees with the earlier result.

