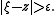Curvature of a measure
Encyclopedia
In mathematics
, the curvature of a measure defined on the Euclidean plane R2 is a quantification of how much the measure's "distribution of mass" is "curved". It is related to notions of curvature
in geometry
. In the form presented below, the concept was introduced in 1995 by the mathematician
Mark S. Melnikov; accordingly, it may be referred to as the Melnikov curvature or Menger-Melnikov curvature. Melnikov and Verdera (1995) established a powerful connection between the curvature of measures and the Cauchy kernel.
of the Euclidean circle
that joins all three of them, or +∞ if they are collinear
. The Menger curvature
c(x, y, z) is defined to be
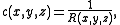
with the natural convention that c(x, y, z) = 0 if x, y and z are collinear. It is also conventional to extend this definition by setting c(x, y, z) = 0 if any of the points x, y and z coincide. The Menger-Melnikov curvature c2(μ) of μ is defined to be
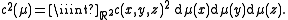
More generally, for α ≥ 0, define c2α(μ) by
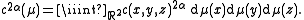
One may also refer to the curvature of μ at a given point x:
in which case
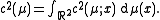
C. Melnikov and Verdera (1995) showed the precise relation of the boundedness
of the Cauchy kernel to the curvature of measures. They proved that if there is some constant C0 such that
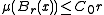
for all x in C and all r > 0, then there is another constant C, depending only on C0, such that
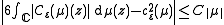
for all ε > 0. Here cε denotes a truncated version of the Menger-Melnikov curvature in which the integral is taken only over those points x, y and z such that
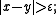
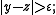
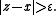
Similarly,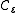
denotes a truncated Cauchy integral operator: for a measure μ on C and a point z in C, define
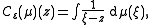
where the integral is taken over those points ξ in C with
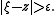
Mathematics
Mathematics is the study of quantity, space, structure, and change. Mathematicians seek out patterns and formulate new conjectures. Mathematicians resolve the truth or falsity of conjectures by mathematical proofs, which are arguments sufficient to convince other mathematicians of their validity...
, the curvature of a measure defined on the Euclidean plane R2 is a quantification of how much the measure's "distribution of mass" is "curved". It is related to notions of curvature
Curvature
In mathematics, curvature refers to any of a number of loosely related concepts in different areas of geometry. Intuitively, curvature is the amount by which a geometric object deviates from being flat, or straight in the case of a line, but this is defined in different ways depending on the context...
in geometry
Geometry
Geometry arose as the field of knowledge dealing with spatial relationships. Geometry was one of the two fields of pre-modern mathematics, the other being the study of numbers ....
. In the form presented below, the concept was introduced in 1995 by the mathematician
Mathematician
A mathematician is a person whose primary area of study is the field of mathematics. Mathematicians are concerned with quantity, structure, space, and change....
Mark S. Melnikov; accordingly, it may be referred to as the Melnikov curvature or Menger-Melnikov curvature. Melnikov and Verdera (1995) established a powerful connection between the curvature of measures and the Cauchy kernel.
Definition
Let μ be a Borel measure on the Euclidean plane R2. Given three (distinct) points x, y and z in R2, let R(x, y, z) be the radiusRadius
In classical geometry, a radius of a circle or sphere is any line segment from its center to its perimeter. By extension, the radius of a circle or sphere is the length of any such segment, which is half the diameter. If the object does not have an obvious center, the term may refer to its...
of the Euclidean circle
Circle
A circle is a simple shape of Euclidean geometry consisting of those points in a plane that are a given distance from a given point, the centre. The distance between any of the points and the centre is called the radius....
that joins all three of them, or +∞ if they are collinear
Line (geometry)
The notion of line or straight line was introduced by the ancient mathematicians to represent straight objects with negligible width and depth. Lines are an idealization of such objects...
. The Menger curvature
Menger curvature
In mathematics, the Menger curvature of a triple of points in n-dimensional Euclidean space Rn is the reciprocal of the radius of the circle that passes through the three points...
c(x, y, z) is defined to be
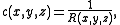
with the natural convention that c(x, y, z) = 0 if x, y and z are collinear. It is also conventional to extend this definition by setting c(x, y, z) = 0 if any of the points x, y and z coincide. The Menger-Melnikov curvature c2(μ) of μ is defined to be
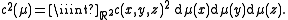
More generally, for α ≥ 0, define c2α(μ) by
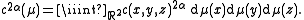
One may also refer to the curvature of μ at a given point x:
in which case
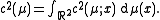
Examples
- The trivial measureTrivial measureIn mathematics, specifically in measure theory, the trivial measure on any measurable space is the measure μ which assigns zero measure to every measurable set: μ = 0 for all A in Σ.-Properties of the trivial measure:...
has zero curvature. - A Dirac measure δa supported at any point a has zero curvature.
- If μ is any measure whose supportSupport (measure theory)In mathematics, the support of a measure μ on a measurable topological space is a precise notion of where in the space X the measure "lives"...
is contained within a Euclidean line L, then μ has zero curvature. For example, one-dimensional Lebesgue measureLebesgue measureIn measure theory, the Lebesgue measure, named after French mathematician Henri Lebesgue, is the standard way of assigning a measure to subsets of n-dimensional Euclidean space. For n = 1, 2, or 3, it coincides with the standard measure of length, area, or volume. In general, it is also called...
on any line (or line segment) has zero curvature. - The Lebesgue measure defined on all of R2 has infinite curvature.
- If μ is the uniform one-dimensional Hausdorff measureHausdorff measureIn mathematics a Hausdorff measure is a type of outer measure, named for Felix Hausdorff, that assigns a number in [0,∞] to each set in Rn or, more generally, in any metric space. The zero dimensional Hausdorff measure is the number of points in the set or ∞ if the set is infinite...
on a circle Cr or radius r, then μ has curvature 1/r.
Relationship to the Cauchy kernel
In this section, R2 is thought of as the complex planeComplex plane
In mathematics, the complex plane or z-plane is a geometric representation of the complex numbers established by the real axis and the orthogonal imaginary axis...
C. Melnikov and Verdera (1995) showed the precise relation of the boundedness
Bounded operator
In functional analysis, a branch of mathematics, a bounded linear operator is a linear transformation L between normed vector spaces X and Y for which the ratio of the norm of L to that of v is bounded by the same number, over all non-zero vectors v in X...
of the Cauchy kernel to the curvature of measures. They proved that if there is some constant C0 such that
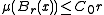
for all x in C and all r > 0, then there is another constant C, depending only on C0, such that
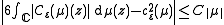
for all ε > 0. Here cε denotes a truncated version of the Menger-Melnikov curvature in which the integral is taken only over those points x, y and z such that
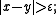
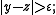
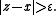
Similarly,
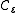denotes a truncated Cauchy integral operator: for a measure μ on C and a point z in C, define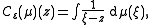where the integral is taken over those points ξ in C with