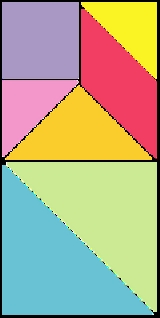
Dissection puzzle
Encyclopedia
A dissection puzzle, also called a transformation puzzle or Richter Puzzle, is a tiling puzzle
where a solver is given a set of pieces that can be assembled in different ways to produce two or more distinct geometric shape
s. The creation of new dissection puzzles is also considered to be a type of dissection puzzle. Puzzles may include various restraints, such as hinged pieces, pieces that can fold, or pieces that can twist. Creators of new dissection puzzles emphasize using a minimum number of pieces, or creating novel situations, such as ensuring that every piece connects to another with a hinge.
Dissection puzzles are an early form of geometric puzzle. The earliest known descriptions of dissection puzzles are from the time of Plato
(427–347 BCE) in Ancient Greece
, and involve the challenge of turning two equal squares into one larger square using four pieces. Other ancient dissection puzzles were used as graphic depictions of the Pythagorean theorem
(see square trisection
). A famous ancient Greek dissection puzzle is the Ostomachion
, a mathematical treatise attributed to Archimedes
.
In the 10th century, Arabic mathematicians used geometric dissections in their commentaries on Euclid's Elements
. In the 18th century, Chinese
scholar Tai Chen described an elegant dissection for approximating the value of π
.
The puzzles saw a major increase in general popularity in the late 19th century when newspapers and magazines began running dissection puzzles. Puzzle creators Sam Loyd
in the United States and Henry Dudeney
in the United Kingdom were among the most published. Since then, dissection puzzles have been used for entertainment and maths education, and creation of complex dissection puzzles is considered an entertaining use of geometric principles by mathematicians and math students.
Some types of dissection puzzle are intended to create a large number of different geometric shapes. Tangram
is a popular dissection puzzle of this type. The seven pieces can be configured into one of a few home shapes, such as the large square and rectangle that the pieces are often stored in, to any number of smaller squares, triangles, parallelogram
s, or esoteric shapes and figures. Some geometric forms are easy to create, while others present an extreme challenge. This variability has ensured the puzzle's popularity.
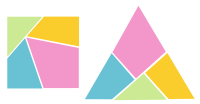Other dissections are intended to move between a pair of geometric shapes, such as a triangle to a square, or a square to a five-pointed star. A dissection puzzle of this description is the haberdasher's problem, proposed in 1907 by Henry Dudeney
. The puzzle is a dissection of a triangle to a square, in only four pieces. It is one of the simplest regular polygon to square dissections known, and is now a classic example.
Tiling puzzle
Tiling puzzles are puzzles involving two-dimensional packing problems in which a number of flat shapes have to be assembled into a larger given shape without overlaps . Some tiling puzzles ask you to dissect a given shape first and then rearrange the pieces into another shape...
where a solver is given a set of pieces that can be assembled in different ways to produce two or more distinct geometric shape
Shape
The shape of an object located in some space is a geometrical description of the part of that space occupied by the object, as determined by its external boundary – abstracting from location and orientation in space, size, and other properties such as colour, content, and material...
s. The creation of new dissection puzzles is also considered to be a type of dissection puzzle. Puzzles may include various restraints, such as hinged pieces, pieces that can fold, or pieces that can twist. Creators of new dissection puzzles emphasize using a minimum number of pieces, or creating novel situations, such as ensuring that every piece connects to another with a hinge.
History
Plato
Plato , was a Classical Greek philosopher, mathematician, student of Socrates, writer of philosophical dialogues, and founder of the Academy in Athens, the first institution of higher learning in the Western world. Along with his mentor, Socrates, and his student, Aristotle, Plato helped to lay the...
(427–347 BCE) in Ancient Greece
Ancient Greece
Ancient Greece is a civilization belonging to a period of Greek history that lasted from the Archaic period of the 8th to 6th centuries BC to the end of antiquity. Immediately following this period was the beginning of the Early Middle Ages and the Byzantine era. Included in Ancient Greece is the...
, and involve the challenge of turning two equal squares into one larger square using four pieces. Other ancient dissection puzzles were used as graphic depictions of the Pythagorean theorem
Pythagorean theorem
In mathematics, the Pythagorean theorem or Pythagoras' theorem is a relation in Euclidean geometry among the three sides of a right triangle...
(see square trisection
Square trisection
The square trisection consist in cutting a square into pieces that can be rearrange to form three identical squares.- Problem History :The dissection of a square in three congruent partitions is a geometrical problem that dates back to the Islamic Golden Age...
). A famous ancient Greek dissection puzzle is the Ostomachion
Ostomachion
Ostomachion, also known as loculus Archimedius and also as syntomachion, is a mathematical treatise attributed to Archimedes. This work has survived fragmentarily in an Arabic version and in a copy of the original ancient Greek text made in Byzantine times...
, a mathematical treatise attributed to Archimedes
Archimedes
Archimedes of Syracuse was a Greek mathematician, physicist, engineer, inventor, and astronomer. Although few details of his life are known, he is regarded as one of the leading scientists in classical antiquity. Among his advances in physics are the foundations of hydrostatics, statics and an...
.
In the 10th century, Arabic mathematicians used geometric dissections in their commentaries on Euclid's Elements
Euclid's Elements
Euclid's Elements is a mathematical and geometric treatise consisting of 13 books written by the Greek mathematician Euclid in Alexandria c. 300 BC. It is a collection of definitions, postulates , propositions , and mathematical proofs of the propositions...
. In the 18th century, Chinese
Han Chinese
Han Chinese are an ethnic group native to China and are the largest single ethnic group in the world.Han Chinese constitute about 92% of the population of the People's Republic of China , 98% of the population of the Republic of China , 78% of the population of Singapore, and about 20% of the...
scholar Tai Chen described an elegant dissection for approximating the value of π
Pi
' is a mathematical constant that is the ratio of any circle's circumference to its diameter. is approximately equal to 3.14. Many formulae in mathematics, science, and engineering involve , which makes it one of the most important mathematical constants...
.
The puzzles saw a major increase in general popularity in the late 19th century when newspapers and magazines began running dissection puzzles. Puzzle creators Sam Loyd
Sam Loyd
Samuel Loyd , born in Philadelphia and raised in New York, was an American chess player, chess composer, puzzle author, and recreational mathematician....
in the United States and Henry Dudeney
Henry Dudeney
Henry Ernest Dudeney was an English author and mathematician who specialised in logic puzzles and mathematical games. He is known as one of the country's foremost creators of puzzles...
in the United Kingdom were among the most published. Since then, dissection puzzles have been used for entertainment and maths education, and creation of complex dissection puzzles is considered an entertaining use of geometric principles by mathematicians and math students.
Types of dissection puzzle
Tangram
The tangram is a dissection puzzle consisting of seven flat shapes, called tans, which are put together to form shapes. The objective of the puzzle is to form a specific shape using all seven pieces, which may not overlap...
is a popular dissection puzzle of this type. The seven pieces can be configured into one of a few home shapes, such as the large square and rectangle that the pieces are often stored in, to any number of smaller squares, triangles, parallelogram
Parallelogram
In Euclidean geometry, a parallelogram is a convex quadrilateral with two pairs of parallel sides. The opposite or facing sides of a parallelogram are of equal length and the opposite angles of a parallelogram are of equal measure...
s, or esoteric shapes and figures. Some geometric forms are easy to create, while others present an extreme challenge. This variability has ensured the puzzle's popularity.
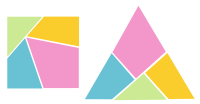
Henry Dudeney
Henry Ernest Dudeney was an English author and mathematician who specialised in logic puzzles and mathematical games. He is known as one of the country's foremost creators of puzzles...
. The puzzle is a dissection of a triangle to a square, in only four pieces. It is one of the simplest regular polygon to square dissections known, and is now a classic example.
See also
- OstomachionOstomachionOstomachion, also known as loculus Archimedius and also as syntomachion, is a mathematical treatise attributed to Archimedes. This work has survived fragmentarily in an Arabic version and in a copy of the original ancient Greek text made in Byzantine times...
- Pizza theoremPizza theoremIn elementary geometry, the pizza theorem states the equality of two areas that arise when one partitions a disk in a certain way.Let p be an interior point of the disk, and let n be a number that is evenly divisible by four and greater than or equal to eight...
- PuzzlePuzzleA puzzle is a problem or enigma that tests the ingenuity of the solver. In a basic puzzle, one is intended to put together pieces in a logical way in order to come up with the desired solution...
- Tiling puzzleTiling puzzleTiling puzzles are puzzles involving two-dimensional packing problems in which a number of flat shapes have to be assembled into a larger given shape without overlaps . Some tiling puzzles ask you to dissect a given shape first and then rearrange the pieces into another shape...
s