
Free loop
Encyclopedia
In the mathematical
field of topology
, a free loop is a variant of the mathematical notion of a loop
. Whereas a loop has a distinguished point on it, called a basepoint, a free loop lacks such a distinguished point. Formally, let X be a topological space
. Then a free loop in X is an equivalence class of continuous function
s from the circle
S1 to X. Two loops are equivalent if they differ by a reparameterization of the circle. That is, ƒ ≈ g if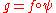
for a homeomorphism
ψ : S1 → S1.
Thus, a free loop, as opposed to a based loop used in the definition of the fundamental group
, is a map from the circle to the space without the basepoint-preserving restriction. Free homotopy classes of free loops correspond to conjugacy class
es in the fundamental group.
In the last years, interest in the space of all free loops LX has grown with the advent of string topology
, i.e. the study of new algebraic structures on the homology
of the free loop space.
Mathematics
Mathematics is the study of quantity, space, structure, and change. Mathematicians seek out patterns and formulate new conjectures. Mathematicians resolve the truth or falsity of conjectures by mathematical proofs, which are arguments sufficient to convince other mathematicians of their validity...
field of topology
Topology
Topology is a major area of mathematics concerned with properties that are preserved under continuous deformations of objects, such as deformations that involve stretching, but no tearing or gluing...
, a free loop is a variant of the mathematical notion of a loop
Loop (topology)
In mathematics, a loop in a topological space X is a path f from the unit interval I = [0,1] to X such that f = f...
. Whereas a loop has a distinguished point on it, called a basepoint, a free loop lacks such a distinguished point. Formally, let X be a topological space
Topological space
Topological spaces are mathematical structures that allow the formal definition of concepts such as convergence, connectedness, and continuity. They appear in virtually every branch of modern mathematics and are a central unifying notion...
. Then a free loop in X is an equivalence class of continuous function
Continuous function
In mathematics, a continuous function is a function for which, intuitively, "small" changes in the input result in "small" changes in the output. Otherwise, a function is said to be "discontinuous". A continuous function with a continuous inverse function is called "bicontinuous".Continuity of...
s from the circle
Circle
A circle is a simple shape of Euclidean geometry consisting of those points in a plane that are a given distance from a given point, the centre. The distance between any of the points and the centre is called the radius....
S1 to X. Two loops are equivalent if they differ by a reparameterization of the circle. That is, ƒ ≈ g if
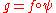
for a homeomorphism
Homeomorphism
In the mathematical field of topology, a homeomorphism or topological isomorphism or bicontinuous function is a continuous function between topological spaces that has a continuous inverse function. Homeomorphisms are the isomorphisms in the category of topological spaces—that is, they are...
ψ : S1 → S1.
Thus, a free loop, as opposed to a based loop used in the definition of the fundamental group
Fundamental group
In mathematics, more specifically algebraic topology, the fundamental group is a group associated to any given pointed topological space that provides a way of determining when two paths, starting and ending at a fixed base point, can be continuously deformed into each other...
, is a map from the circle to the space without the basepoint-preserving restriction. Free homotopy classes of free loops correspond to conjugacy class
Conjugacy class
In mathematics, especially group theory, the elements of any group may be partitioned into conjugacy classes; members of the same conjugacy class share many properties, and study of conjugacy classes of non-abelian groups reveals many important features of their structure...
es in the fundamental group.
In the last years, interest in the space of all free loops LX has grown with the advent of string topology
String topology
String topology, a branch of mathematics, is the study of algebraic structures on the homology of free loop spaces. The field was started by Chas and Sullivan in 1999 .-Motivation:...
, i.e. the study of new algebraic structures on the homology
Singular homology
In algebraic topology, a branch of mathematics, singular homology refers to the study of a certain set of algebraic invariants of a topological space X, the so-called homology groups H_n....
of the free loop space.

