
Graduate Texts in Mathematics
Encyclopedia
Graduate Texts in Mathematics (GTM) is a series of graduate-level
textbooks in mathematics
published by Springer-Verlag. The books in this series, like the other Springer-Verlag mathematics series, are yellow books of a standard size (with variable numbers of pages). The GTM series is easily identified by a white band at the top of the book.
Graduate school
A graduate school is a school that awards advanced academic degrees with the general requirement that students must have earned a previous undergraduate degree...
textbooks in mathematics
Mathematics
Mathematics is the study of quantity, space, structure, and change. Mathematicians seek out patterns and formulate new conjectures. Mathematicians resolve the truth or falsity of conjectures by mathematical proofs, which are arguments sufficient to convince other mathematicians of their validity...
published by Springer-Verlag. The books in this series, like the other Springer-Verlag mathematics series, are yellow books of a standard size (with variable numbers of pages). The GTM series is easily identified by a white band at the top of the book.
List of books
- 1 Introduction to Axiomatic Set Theory, Gaisi TakeutiGaisi Takeutiis a Japanese mathematician, known for his work in proof theory.After graduating from Tokyo University, he went to Princeton to study under Kurt Gödel.He later became a professor at the University of Illinois at Urbana-Champaign...
, Wilson. M. Zaring (ISBN 978-0-387-90024-7) - 2 Measure and Category, John C. Oxtoby (1997, ISBN 978-0-387-90508-2)
- 3 Topological Vector SpacesTopological vector spaceIn mathematics, a topological vector space is one of the basic structures investigated in functional analysis...
, H. H. Schaefer, M. P. Wolff (1999, ISBN 978-0-387-98726-2) - 4 A Course in Homological AlgebraHomological algebraHomological algebra is the branch of mathematics which studies homology in a general algebraic setting. It is a relatively young discipline, whose origins can be traced to investigations in combinatorial topology and abstract algebra at the end of the 19th century, chiefly by Henri Poincaré and...
, Peter HiltonPeter HiltonPeter John Hilton was a British mathematician, noted for his contributions to homotopy theory and for code-breaking during the Second World War.-Life:Hilton was born in London, and educated at St Paul's School...
, Urs Stammbach (1997, ISBN 978-0-387-94823-2) - 5 Categories for the Working MathematicianCategories for the Working MathematicianCategories for the Working Mathematician is a textbook in category theory written by American mathematician Saunders Mac Lane, who cofounded the subject together with Samuel Eilenberg. It was first published in 1971, and is based on his lectures on the subject given at the University of Chicago,...
, Saunders Mac LaneSaunders Mac LaneSaunders Mac Lane was an American mathematician who cofounded category theory with Samuel Eilenberg.-Career:...
(1998, ISBN 978-0-387-98403-2) - 6 Projective PlanesProjective planeIn mathematics, a projective plane is a geometric structure that extends the concept of a plane. In the ordinary Euclidean plane, two lines typically intersect in a single point, but there are some pairs of lines that do not intersect...
, Daniel R. Hughes, Fred C. Piper, (1982, ISBN 978-3-540-90043-6) - 7 A Course in Arithmetic, Jean-Pierre SerreJean-Pierre SerreJean-Pierre Serre is a French mathematician. He has made contributions in the fields of algebraic geometry, number theory, and topology.-Early years:...
(1996, ISBN 978-0-387-90040-7) - 8 Axiomatic Set Theory, Gaisi TakeutiGaisi Takeutiis a Japanese mathematician, known for his work in proof theory.After graduating from Tokyo University, he went to Princeton to study under Kurt Gödel.He later became a professor at the University of Illinois at Urbana-Champaign...
, Wilson. M. Zaring, (1973, ISBN 9783540900504) - 9 Introduction to Lie AlgebraLie algebraIn mathematics, a Lie algebra is an algebraic structure whose main use is in studying geometric objects such as Lie groups and differentiable manifolds. Lie algebras were introduced to study the concept of infinitesimal transformations. The term "Lie algebra" was introduced by Hermann Weyl in the...
s and Representation TheoryRepresentation theoryRepresentation theory is a branch of mathematics that studies abstract algebraic structures by representing their elements as linear transformations of vector spaces, and studiesmodules over these abstract algebraic structures...
, James E. Humphreys (1997, ISBN 978-0-387-90053-7) - 10 A Course in Simple-HomotopyHomotopyIn topology, two continuous functions from one topological space to another are called homotopic if one can be "continuously deformed" into the other, such a deformation being called a homotopy between the two functions...
Theory, Marshall. M. Cohen, (1973, ISBN 0-38790056-X) - 11 Functions of One Complex Variable I, John B. ConwayJohn B. ConwayJohn Bligh Conway is an American mathematician. He is currently the department chairman at the George Washington University. His specialty is functional analysis, particularly bounded operators on a Hilbert space....
(1995, ISBN 978-0-387-90328-6) - 12 Advanced Mathematical Analysis, R. Beals (1973, ISBN 978-0-387-90065-0)
- 13 Rings and Categories of Modules, Frank W. Anderson, Kent R. Fuller (1992, ISBN 978-0-387-97845-1)
- 14 Stable Mappings and Their Singularities, Martin Golubitsky, Victor GuilleminVictor GuilleminVictor William Guillemin is a mathematician, a leader in the field of symplectic geometry, who has also made fundamental contributions to the fields of microlocal analysis, spectral theory, and mathematical physics...
, (1974, ISBN 0387900721) - 15 Lectures in Functional AnalysisFunctional analysisFunctional analysis is a branch of mathematical analysis, the core of which is formed by the study of vector spaces endowed with some kind of limit-related structure and the linear operators acting upon these spaces and respecting these structures in a suitable sense...
and Operator TheoryOperator theoryIn mathematics, operator theory is the branch of functional analysis that focuses on bounded linear operators, but which includes closed operators and nonlinear operators.Operator theory also includes the study of algebras of operators....
, Sterling. K. Berberian, (1974, ISBN 0-387-90080-2) - 16 The Structure of FieldsField (mathematics)In abstract algebra, a field is a commutative ring whose nonzero elements form a group under multiplication. As such it is an algebraic structure with notions of addition, subtraction, multiplication, and division, satisfying certain axioms...
, David J. Winter, (1974, ISBN 0-387-90074-8) - 17 Random Processes, Murray RosenblattMurray RosenblattMurray Rosenblatt is a statistician specializing in time series analysis who is a Professor ofmathematics at University of California, San Diego. He received his Ph.D. at Cornell University....
, (1974, ISBN 0-387-90085-3) - 18 Measure Theory, Paul R. Halmos (1974, ISBN 978-0-387-90088-9)
- 19 A Hilbert SpaceHilbert spaceThe mathematical concept of a Hilbert space, named after David Hilbert, generalizes the notion of Euclidean space. It extends the methods of vector algebra and calculus from the two-dimensional Euclidean plane and three-dimensional space to spaces with any finite or infinite number of dimensions...
Problem Book, Paul R. Halmos (1982, ISBN 978-0-387-90685-0) - 20 Fibre BundlesFiber bundleIn mathematics, and particularly topology, a fiber bundle is intuitively a space which locally "looks" like a certain product space, but globally may have a different topological structure...
, Dale Husemoller (1994, ISBN 978-0-387-94087-8) - 21 Linear Algebraic GroupsLinear algebraic groupIn mathematics, a linear algebraic group is a subgroup of the group of invertible n×n matrices that is defined by polynomial equations...
, James E. Humphreys (1998, ISBN 978-0-387-90108-4) - 22 An Algebraic Introduction to Mathematical LogicMathematical logicMathematical logic is a subfield of mathematics with close connections to foundations of mathematics, theoretical computer science and philosophical logic. The field includes both the mathematical study of logic and the applications of formal logic to other areas of mathematics...
, Donald W. Barnes, John M. Mack (1975, ISBN 978-0387901091) - 23 Linear AlgebraLinear algebraLinear algebra is a branch of mathematics that studies vector spaces, also called linear spaces, along with linear functions that input one vector and output another. Such functions are called linear maps and can be represented by matrices if a basis is given. Thus matrix theory is often...
, Werner H. Greub (1981, ISBN 978-0-387-90110-7) - 24 Geometric Functional Analysis and Its Applications, Richard B. Holmes, (1975, ISBN 9780387901367)
- 25 Real and Abstract Analysis, Edwin Hewitt, Karl Stromberg (1975, ISBN 978-0-387-90138-1)
- 26 Algebraic Theories, Ernest G. Manes, (1976, ISBN 9783540901402)
- 27 General Topology, John L. KelleyJohn L. KelleyJohn Leroy Kelley was an American mathematician at University of California, Berkeley who worked in general topology and functional analysis....
(1975, ISBN 978-0-387-90125-1) - 28 Commutative Algebra I, Oscar ZariskiOscar ZariskiOscar Zariski was a Russian mathematician and one of the most influential algebraic geometers of the 20th century.-Education:...
, Pierre SamuelPierre SamuelPierre Samuel was a French mathematician, known for his work in commutative algebra and its applications to algebraic geometry. The two-volume work Commutative Algebra that he wrote with Oscar Zariski is a classic. Other books of his covered projective geometry and algebraic number theory...
, Cohen (1975, ISBN 978-0-387-90089-6) - 29 Commutative Algebra II, Oscar ZariskiOscar ZariskiOscar Zariski was a Russian mathematician and one of the most influential algebraic geometers of the 20th century.-Education:...
, Pierre SamuelPierre SamuelPierre Samuel was a French mathematician, known for his work in commutative algebra and its applications to algebraic geometry. The two-volume work Commutative Algebra that he wrote with Oscar Zariski is a classic. Other books of his covered projective geometry and algebraic number theory... - 30 Lectures in Abstract Algebra I, Nathan JacobsonNathan JacobsonNathan Jacobson was an American mathematician....
- 31 Lectures in Abstract Algebra II, Nathan JacobsonNathan JacobsonNathan Jacobson was an American mathematician....
- 32 Lectures in Abstract Algebra III, Nathan JacobsonNathan JacobsonNathan Jacobson was an American mathematician....
- 33 Differential Topology, Morris W. Hirsch
- 34 Principles of Random Walk, Frank Spitzer
- 35 Several Complex Variables and Banach Algebras, Herbert Alexander, John Wermer
- 36 Linear Topological Spaces, John L. KelleyJohn L. KelleyJohn Leroy Kelley was an American mathematician at University of California, Berkeley who worked in general topology and functional analysis....
, Isaac Namioka - 37 Mathematical Logic, J. Donald Monk
- 38 Several Complex Variables, Grauert, Fritzsche
- 39 An Invitation to
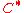 -Algebras, William Arveson
-Algebras, William Arveson - 40 Denumerable Markov Chains, John George KemenyJohn George KemenyJohn George Kemeny was a Hungarian American mathematician, computer scientist, and educator best known for co-developing the BASIC programming language in 1964 with Thomas E. Kurtz. Kemeny served as the 13th President of Dartmouth College from 1970 to 1981 and pioneered the use of computers in...
, Snell, Knapp et al. - 41 Modular Functions and Dirichlet Series in Number Theory, Tom M. ApostolTom M. ApostolTom Mike Apostol is a Greek-American analytic number theorist and professor at the California Institute of Technology.He was born in Helper, Utah in 1923. His parents, Emmanouil Apostolopoulos and Efrosini Papathanasopoulos, originated from Greece. Mr...
(ISBN 978-0-387-97127-8) - 42 Linear Representations of Finite Groups, Jean-Pierre SerreJean-Pierre SerreJean-Pierre Serre is a French mathematician. He has made contributions in the fields of algebraic geometry, number theory, and topology.-Early years:...
, Scott - 43 Rings of Continuous Functions, Gillman, Jerison
- 44 Elementary Algebraic GeometryAlgebraic geometryAlgebraic geometry is a branch of mathematics which combines techniques of abstract algebra, especially commutative algebra, with the language and the problems of geometry. It occupies a central place in modern mathematics and has multiple conceptual connections with such diverse fields as complex...
, K. Kendig - 45 Probability Theory I, M. Loève
- 46 Probability Theory II, M. Loève
- 47 Geometric Topology in Dimensions 2 and 3, Edwin E. MoiseEdwin E. MoiseEdwin Evariste Moise was an American mathematician and mathematics education reformer. After his retirement from mathematics he became a literary critic of 19th century English poetry and had several notes published in that field.-Early life and education:...
- 48 General Relativity for Mathematicians, R. K. Sachs, H. Wu
- 49 Linear Geometry, K. W. Gruenberg, A. J. Weir (ISBN 978-0-387-90227-2)
- 50 Fermat's Last Theorem, Harold M. Edwards (ISBN 978-0-387-90230-2)
- 51 A Course in Differential Geometry, Klingenberg
- 52 Algebraic GeometryHartshorne's Algebraic GeometryAlgebraic Geometry is an influential algebraic geometry textbook written by Robin Hartshorne and published by Springer-Verlag in 1977. It was the first extended treatment of scheme theory written as a text intended to be accessible to graduate students....
, Robin HartshorneRobin HartshorneRobin Cope Hartshorne is an American mathematician. Hartshorne is an algebraic geometer who studied with Zariski, Mumford, J.-P. Serre and Grothendieck.... - 53 A Course in Mathematical Logic for Mathematicians, Yu. I. Manin, Boris Zilber, (2009, ISBN 978-1-4419-0614-4)
- 54 Combinatorics with Emphasis on the Theory of Graphs, Graver, Watkins
- 55 Introduction to Operator Theory I, Brown, Pearcy
- 56 Algebraic Topology: An Introduction, William S. MasseyWilliam S. MasseyWilliam Schumacher Massey is an American mathematician, known for his work in algebraic topology. The Massey product is named for him. He worked also on the formulation of spectral sequences by means of exact couples, and wrote several textbooks, including Algebraic Topology .William Massey was...
- 57 Introduction to Knot Theory, Richard H. Crowell, Ralph H. Fox (1977, ISBN 978-0-387-90272-2)
- 58 P-adic Numbers, p-adic Analysis, and Zeta-Functions, Neal KoblitzNeal KoblitzNeal I. Koblitz is a Professor of Mathematics at the University of Washington in the Department of Mathematics. He is also an adjunct professor with the Centre for Applied Cryptographic Research at the University of Waterloo. He is the creator of hyperelliptic curve cryptography and the...
- 59 Cyclotomic FieldsCyclotomic fieldIn number theory, a cyclotomic field is a number field obtained by adjoining a complex primitive root of unity to Q, the field of rational numbers...
, Serge LangSerge LangSerge Lang was a French-born American mathematician. He was known for his work in number theory and for his mathematics textbooks, including the influential Algebra... - 60 Mathematical Methods of Classical Mechanics, V. I. Arnold
- 61 Elements of Homotopy Theory, George W. WhiteheadGeorge W. WhiteheadGeorge William Whitehead, Jr. was a professor of mathematics at the Massachusetts Institute of Technology, a member of the United States National Academy of Sciences, and a Fellow of the American Academy of Arts and Sciences. He is known for his work on algebraic topology...
- 62 Fundamentals of the Theory of Groups, Kargapolov, Merzljakov, Burns
- 63 Graph Theory, Béla BollobásBéla BollobásBéla Bollobás FRS is a Hungarian-born British mathematician who has worked in various areas of mathematics, including functional analysis, combinatorics, graph theory and percolation. As a student, he took part in the first three International Mathematical Olympiads, winning two gold medals...
- 64 Fourier Series I, Edwards
- 65 Differential Analysis on Complex Manifolds, R.O. Wells Jr.
- 66 Introduction to Affine Group Schemes, W. C. Waterhouse
- 67 Local Fields, Jean-Pierre SerreJean-Pierre SerreJean-Pierre Serre is a French mathematician. He has made contributions in the fields of algebraic geometry, number theory, and topology.-Early years:...
, Greenberg (1980) - 68 Linear Operators on Hilbert Spaces, Weidmann, Szuecs
- 69 Cyclotomic FieldsCyclotomic fieldIn number theory, a cyclotomic field is a number field obtained by adjoining a complex primitive root of unity to Q, the field of rational numbers...
II, Serge LangSerge LangSerge Lang was a French-born American mathematician. He was known for his work in number theory and for his mathematics textbooks, including the influential Algebra... - 70 Singular Homology Theory, William S. MasseyWilliam S. MasseyWilliam Schumacher Massey is an American mathematician, known for his work in algebraic topology. The Massey product is named for him. He worked also on the formulation of spectral sequences by means of exact couples, and wrote several textbooks, including Algebraic Topology .William Massey was...
- 71 Riemann Surfaces, Herschel Farkas, Irwin Kra
- 72 Classical Topology and Combinatorial Group Theory, John Stillwell
- 73 Algebra, Thomas W. HungerfordThomas W. HungerfordThomas William Hungerford is an American mathematician who works in algebra and mathematics education. He is the author or coauthor of several widely used and widely cited textbooks covering high-school to graduate-level mathematics. From 1963 until 1980 he taught at the University of Washington...
- 74 Multiplicative Number Theory, Harold DavenportHarold DavenportHarold Davenport FRS was an English mathematician, known for his extensive work in number theory.-Early life:...
, Hugh Montgomery - 75 Basic Theory of Algebraic Groups and Lie Algebras, G. P. Hochschild
- 76 Algebraic GeometryAlgebraic geometryAlgebraic geometry is a branch of mathematics which combines techniques of abstract algebra, especially commutative algebra, with the language and the problems of geometry. It occupies a central place in modern mathematics and has multiple conceptual connections with such diverse fields as complex...
, Iitaka - 77 Lectures on the Theory of Algebraic Numbers, HeckeErich HeckeErich Hecke was a German mathematician. He obtained his doctorate in Göttingen under the supervision of David Hilbert. Kurt Reidemeister and Heinrich Behnke were among his students....
, Brauer, Goldman et al. - 78 A Course in Universal AlgebraUniversal algebraUniversal algebra is the field of mathematics that studies algebraic structures themselves, not examples of algebraic structures....
, Burris, Sankappanavar (Online) - 79 An Introduction to Ergodic Theory, Peter Walters
- 80 A Course in the Theory of Groups, Derek J.S. Robinson
- 81 Lectures on Riemann Surfaces, Forster, Gilligan
- 82 Differential Forms in Algebraic Topology, Raoul BottRaoul BottRaoul Bott, FRS was a Hungarian mathematician known for numerous basic contributions to geometry in its broad sense...
, Loring Tu - 83 Introduction to Cyclotomic Fields, Lawrence C. Washington
- 84 A Classical Introduction to Modern Number Theory, Ireland, Rosen (1995, ISBN 0-387-97329-X)
- 85 Fourier Series A Modern Introduction, R. E. Edwards
- 86 Introduction to Coding Theory, J. H. van LintJack van LintJacobus Hendricus van Lint was a Dutch mathematician, professor at the Eindhoven University of Technology, of which he was rector magnificus from 1991 till 1996....
(3rd ed 1998, ISBN 3-540-64133-5) - 87 Cohomology of Groups, Kenneth S. Brown
- 88 Associative Algebras, R. S. Pierce
- 89 Introduction to Algebraic and Abelian Functions, Serge LangSerge LangSerge Lang was a French-born American mathematician. He was known for his work in number theory and for his mathematics textbooks, including the influential Algebra...
- 90 An Introduction to Convex Polytopes, Arne Brondsted
- 91 The Geometry of Discrete Groups, Alan F. Beardon
- 92 Sequences and Series in Banach Spaces, J. Diestel
- 93 Modern Geometry - Methods and Applications I, Dubrovin, Fomenko, Novikov et al.
- 94 Foundations of Differentiable Manifolds and Lie Groups, Frank W. Warner
- 95 Probability, Shiryaev, Boas
- 96 A Course in Functional Analysis, John B. ConwayJohn B. ConwayJohn Bligh Conway is an American mathematician. He is currently the department chairman at the George Washington University. His specialty is functional analysis, particularly bounded operators on a Hilbert space....
- 97 Introduction to Elliptic Curves and Modular Forms, Neal KoblitzNeal KoblitzNeal I. Koblitz is a Professor of Mathematics at the University of Washington in the Department of Mathematics. He is also an adjunct professor with the Centre for Applied Cryptographic Research at the University of Waterloo. He is the creator of hyperelliptic curve cryptography and the...
- 98 Representations of Compact Lie Groups, Broecker, Dieck
- 99 Finite Reflection Groups, Grove, Benson
- 100 Harmonic Analysis on Semigroups, Berg, Christensen, Ressel
- 101 Galois Theory, Harold M. Edwards
- 102 Lie Groups, Lie Algebras, and Their Representations, V. S. Varadarajan
- 103 Complex Analysis, Serge LangSerge LangSerge Lang was a French-born American mathematician. He was known for his work in number theory and for his mathematics textbooks, including the influential Algebra...
- 104 Modern Geometry - Methods and Applications II, Dubrovin, Fomenko, Novikov et al.
- 105 SL2(R), Serge LangSerge LangSerge Lang was a French-born American mathematician. He was known for his work in number theory and for his mathematics textbooks, including the influential Algebra...
- 106 The Arithmetic of Elliptic Curves, Joseph H. SilvermanJoseph H. SilvermanJoseph Hillel Silverman is currently a professor of mathematics at Brown University. Joseph Silverman received an Sc.B. from Brown University in 1977 and a Ph.D. from Harvard University in 1982 under the direction of John Tate. He taught at M.I.T...
- 107 Applications of Lie Groups to Differential Equations, Peter J. Olver
- 108 Holomorphic Functions and Integral Representations in Several Complex Variables, R. Michael Range
- 109 Univalent Functions and Teichmüller Spaces, O. Lehto
- 110 Algebraic Number Theory, Serge LangSerge LangSerge Lang was a French-born American mathematician. He was known for his work in number theory and for his mathematics textbooks, including the influential Algebra...
- 111 Elliptic Curves, Dale Husemöller
- 112 Elliptic Functions, Serge LangSerge LangSerge Lang was a French-born American mathematician. He was known for his work in number theory and for his mathematics textbooks, including the influential Algebra...
- 113 Brownian Motion and Stochastic Calculus, Ioannis Karatzas, Steven Shreve
- 114 A Course in Number Theory and Cryptography, Neal KoblitzNeal KoblitzNeal I. Koblitz is a Professor of Mathematics at the University of Washington in the Department of Mathematics. He is also an adjunct professor with the Centre for Applied Cryptographic Research at the University of Waterloo. He is the creator of hyperelliptic curve cryptography and the...
- 115 Differential Geometry, Berger, Gostiaux, Levy
- 116 Measure and Integral, John L. KelleyJohn L. KelleyJohn Leroy Kelley was an American mathematician at University of California, Berkeley who worked in general topology and functional analysis....
, Srinivasan - 117 Algebraic Groups and Class Fields, Jean-Pierre SerreJean-Pierre SerreJean-Pierre Serre is a French mathematician. He has made contributions in the fields of algebraic geometry, number theory, and topology.-Early years:...
- 118 Analysis Now, Gert K. Pedersen
- 119 An Introduction to Algebraic Topology, Joseph J. Rotman
- 120 Weakly Differentiable Functions, William P. Ziemer
- 121 Cyclotomic FieldsCyclotomic fieldIn number theory, a cyclotomic field is a number field obtained by adjoining a complex primitive root of unity to Q, the field of rational numbers...
I-II, Serge LangSerge LangSerge Lang was a French-born American mathematician. He was known for his work in number theory and for his mathematics textbooks, including the influential Algebra...
, Karl RubinKarl RubinKarl Rubin is an American mathematician at University of California, Irvine as Thorp Professor of Mathematics. His research interest is in elliptic curves. He was the first mathematician to show that some elliptic curves over the rationals have finite Tate-Shafarevich groups... - 122 Theory of Complex Functions, Remmert, Burckel
- 123 Numbers, Lamotke, Ewing, Ebbinghaus et al.
- 124 Modern Geometry - Methods and Applications III, B. A. Dubrovin, Anatoly Timofeevich Fomenko, Sergei Novikov et al. (1990, ISBN 978-0-387-97271-8)
- 125 Complex Variables, Berenstein, Gay
- 126 Linear Algebraic Groups, Armand BorelArmand BorelArmand Borel was a Swiss mathematician, born in La Chaux-de-Fonds, and was a permanent professor at the Institute for Advanced Study in Princeton, New Jersey, United States from 1957 to 1993...
- 127 A Basic Course in Algebraic Topology, William S. MasseyWilliam S. MasseyWilliam Schumacher Massey is an American mathematician, known for his work in algebraic topology. The Massey product is named for him. He worked also on the formulation of spectral sequences by means of exact couples, and wrote several textbooks, including Algebraic Topology .William Massey was...
- 128 Partial Differential Equations, Jeffrey Rauch
- 129 Representation Theory, William Fulton, Joe Harris
- 130 Tensor Geometry, C. T. J. Dodson, T. Poston
- 131 A First Course in Noncommutative Rings, T. Y. Lam
- 132 Iteration of Rational Functions, Alan F. Beardon
- 133 Algebraic GeometryAlgebraic geometryAlgebraic geometry is a branch of mathematics which combines techniques of abstract algebra, especially commutative algebra, with the language and the problems of geometry. It occupies a central place in modern mathematics and has multiple conceptual connections with such diverse fields as complex...
, Joe Harris - 134 Coding and Information Theory, Steven RomanSteven RomanSteven Roman is a mathematician, currently Emeritus Professor of Mathematics at California State University and Lecturer in Mathematics at University of California. He is one of the main developers of umbral calculus. He has written around 40 books on mathematics and computer programming.-External...
- 135 Advanced Linear Algebra, Steven RomanSteven RomanSteven Roman is a mathematician, currently Emeritus Professor of Mathematics at California State University and Lecturer in Mathematics at University of California. He is one of the main developers of umbral calculus. He has written around 40 books on mathematics and computer programming.-External...
- 136 Algebra, Adkins, Weintraub
- 137 Harmonic Function Theory, Axler, Bourdon, Ramey
- 138 A Course in Computational Algebraic Number Theory, Henri Cohen (1996, ISBN 0-387-55640-0)
- 139 Topology and Geometry, Glen E. Bredon
- 140 Optima and Equilibria, Jean-Pierre Aubin
- 141 Gröbner Bases, Becker, Weispfenning, Kredel
- 142 Real and Functional Analysis, Serge LangSerge LangSerge Lang was a French-born American mathematician. He was known for his work in number theory and for his mathematics textbooks, including the influential Algebra...
, (1993, ISBN 978-0387940014) - 143 Measure Theory, J. L. DoobJoseph Leo DoobJoseph Leo Doob was an American mathematician, specializing in analysis and probability theory.The theory of martingales was developed by Doob.-Early life and education:...
- 144 Noncommutative Algebra, Farb, Dennis
- 145 Homology Theory, James W. Vick
- 146 Computability, Douglas S. Bridges
- 147 Algebraic K-Theory and Its Applications, Jonathan Rosenberg
- 148 An Introduction to the Theory of Groups, Joseph J. Rotman
- 149 Foundations of Hyperbolic Manifolds, John G. Ratcliffe
- 150 Commutative Algebra with a View Toward Algebraic GeometryAlgebraic geometryAlgebraic geometry is a branch of mathematics which combines techniques of abstract algebra, especially commutative algebra, with the language and the problems of geometry. It occupies a central place in modern mathematics and has multiple conceptual connections with such diverse fields as complex...
, David EisenbudDavid EisenbudDavid Eisenbud is an American mathematician. He is a professor of mathematics at the University of California, Berkeley and was Director of the Mathematical Sciences Research Institute from 1997 to 2007.... - 151 Advanced Topics in the Arithmetic of Elliptic Curves, Joseph H. SilvermanJoseph H. SilvermanJoseph Hillel Silverman is currently a professor of mathematics at Brown University. Joseph Silverman received an Sc.B. from Brown University in 1977 and a Ph.D. from Harvard University in 1982 under the direction of John Tate. He taught at M.I.T...
- 152 Lectures on Polytopes, Günter M. ZieglerGünter M. ZieglerGünter M. Ziegler is a German mathematician. Ziegler is known for his research in discrete mathematics and geometry, and particularly on the combinatorics of polytopes.- Biography :...
- 153 Algebraic Topology, William Fulton
- 154 An Introduction to Analysis, Brown, Pearcy
- 155 Quantum Groups, Christian Kassel
- 156 Classical Descriptive Set Theory, Alexander S. KechrisAlexander S. KechrisAlexander Sotirios Kechris is a descriptive set theorist at Caltech. He has made major contributions to the theory of Borel equivalence relations....
- 157 Integration and Probability, Malliavin, Airault, Kay et al.
- 158 Field Theory, Steven RomanSteven RomanSteven Roman is a mathematician, currently Emeritus Professor of Mathematics at California State University and Lecturer in Mathematics at University of California. He is one of the main developers of umbral calculus. He has written around 40 books on mathematics and computer programming.-External...
- 159 Functions of One Complex Variable II, John B. ConwayJohn B. ConwayJohn Bligh Conway is an American mathematician. He is currently the department chairman at the George Washington University. His specialty is functional analysis, particularly bounded operators on a Hilbert space....
- 160 Differential and Riemannian Manifolds, Serge LangSerge LangSerge Lang was a French-born American mathematician. He was known for his work in number theory and for his mathematics textbooks, including the influential Algebra...
- 161 Polynomials and Polynomial Inequalities, Borwein, Erdelyi
- 162 Groups and Representations, J. L. Alperin, Bell
- 163 Permutation Groups, Dixon, Mortimer
- 164 Additive Number Theory I, Melvyn B. Nathanson (ISBN 0-387-94656-X)
- 165 Additive Number Theory II, Melvyn B. Nathanson (ISBN 0-387-94655-1)
- 166 Differential Geometry, R. W. Sharpe, Shiing-Shen ChernShiing-Shen ChernShiing-Shen Chern was a Chinese American mathematician, one of the leaders in differential geometry of the twentieth century.-Early years in China:...
- 167 Field and Galois Theory, Patrick Morandi
- 168 Combinatorial Convexity and Algebraic GeometryAlgebraic geometryAlgebraic geometry is a branch of mathematics which combines techniques of abstract algebra, especially commutative algebra, with the language and the problems of geometry. It occupies a central place in modern mathematics and has multiple conceptual connections with such diverse fields as complex...
, Guenter Ewald - 169 Matrix Analysis, Rajendra Bhatia
- 170 Sheaf Theory, Glen E. Bredon
- 171 Riemannian Geometry, Peter Petersen
- 172 Classical Topics in Complex Function Theory, Remmert, Kay
- 173 Graph Theory, Reinhard Diestel
- 174 Foundations of Real and Abstract Analysis, Douglas S. Bridges
- 175 An Introduction to Knot Theory, W. B. Raymond Lickorish
- 176 Riemannian Manifolds, John M. Lee
- 177 Analytic Number Theory , Donald J. Newman
- 178 Nonsmooth Analysis and Control Theory, Clarke, Ledyaev, Stern et al.
- 179 Banach Algebra Techniques in Operator Theory, Ronald G. Douglas
- 180 A Course on Borel Sets, S. M. Srivastava
- 181 Numerical Analysis, Rainer Kress
- 182 Ordinary Differential Equations, Walter, Thompson
- 183 An Introduction to Banach Space Theory, Robert E. Megginson
- 184 Modern Graph Theory, Béla BollobásBéla BollobásBéla Bollobás FRS is a Hungarian-born British mathematician who has worked in various areas of mathematics, including functional analysis, combinatorics, graph theory and percolation. As a student, he took part in the first three International Mathematical Olympiads, winning two gold medals...
- 185 Using Algebraic GeometryAlgebraic geometryAlgebraic geometry is a branch of mathematics which combines techniques of abstract algebra, especially commutative algebra, with the language and the problems of geometry. It occupies a central place in modern mathematics and has multiple conceptual connections with such diverse fields as complex...
, Cox, Little, O Shea - 186 Fourier Analysis on Number Fields, Ramakrishnan, Valenza
- 187 Moduli of Curves, Joe Harris, Morrison
- 188 Lectures on the Hyperreals, Robert Goldblatt
- 189 Lectures on Modules and Rings, Tsit-Yuen Lam
- 190 Problems in Algebraic Number Theory, Esmonde, Murty
- 191 Fundamentals of Differential Geometry, Serge LangSerge LangSerge Lang was a French-born American mathematician. He was known for his work in number theory and for his mathematics textbooks, including the influential Algebra...
- 192 Elements of Functional Analysis, Hirsch, Lacombe, Levy
- 193 Advanced Topics in Computational Number Theory, Henri Cohen (2000, ISBN 0-387-98727-4)
- 194 One-Parameter Semigroups for Linear Evolution Equations, Engel, Nagel
- 195 Elementary Methods in Number Theory, Melvyn B. Nathanson
- 196 Basic Homological Algebra, M. Scott Osborne
- 197 The Geometry of Schemes, EisenbudDavid EisenbudDavid Eisenbud is an American mathematician. He is a professor of mathematics at the University of California, Berkeley and was Director of the Mathematical Sciences Research Institute from 1997 to 2007....
, Joe Harris - 198 A Course in p-adic Analysis, Alain Robert
- 199 Theory of Bergman Spaces, Hedenmalm, Korenblum, Zhu
- 200 An Introduction to Riemann-Finsler Geometry, David Bao, Shiing-Shen ChernShiing-Shen ChernShiing-Shen Chern was a Chinese American mathematician, one of the leaders in differential geometry of the twentieth century.-Early years in China:...
, Zhongmin Shen - 201 Diophantine Geometry, Hindry, Joseph H. SilvermanJoseph H. SilvermanJoseph Hillel Silverman is currently a professor of mathematics at Brown University. Joseph Silverman received an Sc.B. from Brown University in 1977 and a Ph.D. from Harvard University in 1982 under the direction of John Tate. He taught at M.I.T...
(2000, ISBN 978-0-387-98975-4) - 202 Introduction to Topological Manifolds, John M. Lee
- 203 The Symmetric Group, Bruce E. Sagan
- 204 Galois Theory, Jean-Pierre Escofier
- 205 Rational Homotopy Theory, Yves Félix, Stephen Halperin, Jean-Claude Thomas (2000, ISBN 978-0-387-95068-0)
- 206 Problems in Analytic Number Theory, M. Ram MurtyM. Ram MurtyMaruti Ram Pedaprolu Murty, FRSC is an Indo-Canadian mathematician, currently head of the Department of Mathematics and Statistics at Queen's University, where he holds a Queen's Research Chair in mathematics.-Career:...
(2001, ISBN 978-0-387-95143-0) - 207 Algebraic Graph Theory, GodsilChris GodsilChristopher David Godsil is a professor at the Department of Combinatorics and Optimization in the faculty of mathematics at the University of Waterloo. He wrote the popular textbook on algebraic graph theory, entitled Algebraic graph theory, with Gordon Royle, His earlier textbook on...
, Royle (2001, ISBN 978-0-387-95241-3) - 208 Analysis for Applied Mathematics, Ward Cheney (2001, ISBN 978-0-387-95279-6)
- 209 A Short Course on Spectral Theory, William Arveson (2002, ISBN 978-0-387-95300-7)
- 210 Number Theory in Function Fields, Michael Rosen (2002, ISBN 978-0-387-95335-9)
- 211 Algebra, Serge LangSerge LangSerge Lang was a French-born American mathematician. He was known for his work in number theory and for his mathematics textbooks, including the influential Algebra...
- 212 Lectures on Discrete Geometry, Jiří MatoušekJirí Matoušek (mathematician)Jiří Matoušek is a Czech mathematician working in computational geometry. He is a professor at Charles University in Prague and is the author of several textbooks and research monographs....
- 213 From Holomorphic Functions to Complex Manifolds, Fritzsche, Grauert
- 214 Partial Differential Equations, Juergen Jost
- 215 Algebraic Functions and Projective Curves, David Goldschmidt
- 216 Matrices, Denis Serre
- 217 Model Theory: An Introduction, David Marker
- 218 Introduction to Smooth Manifolds, John M. Lee (2003, ISBN 978-0-387-95448-6)
- 219 The Arithmetic of Hyperbolic 3-Manifolds, Maclachlan, Reid
- 220 Smooth Manifolds and Observables, Jet Nestruev
- 221 Convex Polytopes, Branko GrünbaumBranko GrünbaumBranko Grünbaum is a Croatian-born mathematician and a professor emeritus at the University of Washington in Seattle. He received his Ph.D. in 1957 from Hebrew University of Jerusalem in Israel....
(2003, ISBN 0-387-00424-6) - 222 Lie Groups, Lie Algebras, and Representations, Brian C. Hall
- 223 Fourier Analysis and its Applications, Anders Vretblad
- 224 Metric Structures in Differential Geometry, Walschap, G., (2004, ISBN 978-0-387-20430-7)
- 225 Lie Groups, Daniel Bump, (2004, ISBN 978-0-387-21154-1)
- 226 Spaces of Holomorphic Functions in the Unit Ball, Zhu, K., (2005, ISBN 978-0-387-22036-9)
- 227 Combinatorial Commutative Algebra, Ezra Miller, Bernd SturmfelsBernd SturmfelsBernd Sturmfels is a Professor of Mathematics and Computer Science at the University of California, Berkeley.He received his PhD in 1987 from the University of Washington and the Technische Universität Darmstadt...
, (2005, ISBN 978-0-387-22356-8) - 228 A First Course in Modular Forms, Fred DiamondFred DiamondFred Diamond is an American mathematician, known for his role in proving the modularity theorem for elliptic curves. His research interest is in modular forms and Galois representations....
, J. Shurman, (2006, ISBN 978-0-387-23229-4) - 229 The Geometry of Syzygies, David EisenbudDavid EisenbudDavid Eisenbud is an American mathematician. He is a professor of mathematics at the University of California, Berkeley and was Director of the Mathematical Sciences Research Institute from 1997 to 2007....
(2005, ISBN 978-0-387-22215-8) - 230 An Introduction to Markov Processes, Daniel W. StroockDaniel W. StroockDaniel Wyler Stroock is an American mathematician, a probabilist.- Biography :He received his undergraduate degree from Harvard University in 1962 and his doctorate from Rockefeller University in 1966...
, (2005, ISBN 978-3-540-23499-9) - 231 Combinatorics of Coxeter Groups, Anders BjörnerAnders BjörnerAnders Björner is a Swedish professor of mathematics, Department of Mathematics, Royal Institute of Technology, Stockholm, Sweden...
, Francisco Brenti, (2005, ISBN 978-3-540-44238-7) - 232 An Introduction to Number Theory, Everest, G., Ward, T., (2005, ISBN 978-1-85233-917-3)
- 233 Topics in Banach Space Theory, Albiac, F., Kalton, N. J., (2006, ISBN 978-0-387-28141-4)
- 234 Analysis and Probability - Wavelets, Signals, Fractals, Jorgensen, P. E. T., (2006, ISBN 978-0-387-29519-0)
- 235 Compact Lie Groups, M. R. Sepanski, (2007, ISBN 978-0-387-30263-8)
- 236 Bounded Analytic Functions, Garnett, J., (2007, ISBN 978-0-387-33621-3)
- 237 An Introduction to Operators on the Hardy-Hilbert Space, Martinez-Avendano, R.A., Rosenthal, P., (2007, ISBN 978-0-387-35418-7)
- 238 A Course in Enumeration, Aigner, M., (2007, ISBN 978-3-540-39032-9)
- 239 Number Theory - Volume I: Tools and Diophantine Equations, Cohen, H., (2007, ISBN 978-0-387-49922-2)
- 240 Number Theory - Volume II: Analytic and Modern Tools, Cohen, H., (2007, ISBN 978-0-387-49893-5)
- 241 The Arithmetic of Dynamical Systems, Joseph H. SilvermanJoseph H. SilvermanJoseph Hillel Silverman is currently a professor of mathematics at Brown University. Joseph Silverman received an Sc.B. from Brown University in 1977 and a Ph.D. from Harvard University in 1982 under the direction of John Tate. He taught at M.I.T...
, (2007, ISBN 978-0-387-69903-5) - 242 Abstract Algebra, Grillet, Pierre Antoine, (2007, ISBN 978-0-387-71567-4)
- 243 Topological Methods in Group Theory, Geoghegan, Ross, (2007, ISBN 978-0-387-74611-1)
- 244 Graph Theory, Bondy, J. A., Murty, U. S. R., (2007, ISBN 978-1-84628-969-9)
- 245 Complex Analysis: Introduced in the Spirit of Lipman Bers,Gilman, Jane P., Kra, Irwin, Rodriguez, Rubi E. (2007, ISBN 978-0-387-74714-9)
- 246 A Course in Commutative Banach Algebras, Kaniuth, Eberhard, (2008, ISBN 978-0-387-72475-1)
- 247 Braid Groups, Kassel, Christian, Turaev, Vladimir, (2008, ISBN 978-0-387-33841-5)
- 248 Buildings Theory and Applications, Abramenko, Peter, Brown, Ken (2008, ISBN 978-0-387-78834-0)
- 249 Classical Fourier Analysis, Grafakos, Loukas, (2008, ISBN 978-0-387-09431-1)
- 250 Modern Fourier Analysis, Grafakos, Loukas, (2008, ISBN 978-0-387-09433-5)
- 251 The Finite Simple Groups, WilsonRobert Arnott WilsonRobert Arnott Wilson is a mathematician in London, England, who is best known for his work on classifying the maximal subgroups of finite simple groups and for the work in the Monster group.-Books:...
, Christopher W. Parker (2009, ISBN 978-1-84800-987-5) - 252 Distributions and Operators, Grubb, Gerd, (2009, ISBN 978-0-387-84894-5)
- 253 Elementary Functional Analysis, MacCluer, Barbara D., (2009, ISBN 978-0-387-85528-8)
- 254 Algebraic Function Fields and Codes, Stichtenoth, Henning, (2009, ISBN 978-3-540-76877-7)
- 255 Symmetry, Representations, and Invariants, Goodman, Roe, Wallach, Nolan R., (2009, ISBN 978-0-387-79851-6)
- 256 A Course in Commutative Algebra, Kemper, Gregor, (2010, ISBN 978-3-642-03544-9)
- 257 Deformation Theory, Robin HartshorneRobin HartshorneRobin Cope Hartshorne is an American mathematician. Hartshorne is an algebraic geometer who studied with Zariski, Mumford, J.-P. Serre and Grothendieck....
, (2010, ISBN 978-1-4419-1595-5) - 258 Foundations of Optimization in Finite Dimensions, Osman Guler, (2010, ISBN 978-0-387-34431-7)
- 259 Ergodic TheoryErgodic theoryErgodic theory is a branch of mathematics that studies dynamical systems with an invariant measure and related problems. Its initial development was motivated by problems of statistical physics....
, Thomas WardThomas WardThomas or Tommy Ward may refer to:*Thomas Ward , English author who converted to Catholicism*Thomas Ward , British Minister to Russia 1730-1731*Thomas Ward , U.S. Congressman from New Jersey...
, Manfred Einsiedler, (2010, ISBN 978-0-85729-020-5) - 260 Monomial Ideals, Jürgen Herzog, (2010, ISBN 978-0-85729-105-9)
- 261 ProbabilityProbabilityProbability is ordinarily used to describe an attitude of mind towards some proposition of whose truth we arenot certain. The proposition of interest is usually of the form "Will a specific event occur?" The attitude of mind is of the form "How certain are we that the event will occur?" The...
and Stochastics, Erhan ÇinlarErhan CinlarErhan Çinlar is a probabilist and the Norman J. Sollenberger Professor in Engineering at Princeton University. He is member of the Operations Research and Financial Engineering department at Princeton University...
, (2011, ISBN 978-0-387-87858-4) - 262 Essentials of Integration TheoryIntegralIntegration is an important concept in mathematics and, together with its inverse, differentiation, is one of the two main operations in calculus...
for AnalysisMathematical analysisMathematical analysis, which mathematicians refer to simply as analysis, has its beginnings in the rigorous formulation of infinitesimal calculus. It is a branch of pure mathematics that includes the theories of differentiation, integration and measure, limits, infinite series, and analytic functions...
, Daniel W. StroockDaniel W. StroockDaniel Wyler Stroock is an American mathematician, a probabilist.- Biography :He received his undergraduate degree from Harvard University in 1962 and his doctorate from Rockefeller University in 1966...
, (2012, ISBN 978-1-4614-1134-5)

