
Grandi's series
Encyclopedia
In mathematics
, the infinite series 1 − 1 + 1 − 1 + …, also written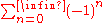
is sometimes called Grandi's series, after Italian mathematician, philosopher, and priest Guido Grandi
, who gave a memorable treatment of the series in 1703. It is a divergent series
, meaning that it lacks a sum in the usual sense. On the other hand, its Cesàro sum
is 1/2.
is to treat it like a telescoping series and perform the subtractions in place:
+ (1 − 1) + (1 − 1) + … = 0 + 0 + 0 + … = 0.
On the other hand, a similar bracketing procedure leads to the apparently contradictory result
Thus, by applying parentheses to Grandi's series in different ways, one can obtain either 0 or 1 as a "value". (Variations of this idea, called the Eilenberg–Mazur swindle
, are sometimes used in knot theory and algebra.)
Treating Grandi's series as a divergent geometric series we may use the same algebraic methods that evaluate convergent geometric series to obtain a third value:
resulting in S = 1/2.
The same conclusion results from calculating −S, subtracting the result from S, and solving 2S = 1.
The above manipulations do not consider what the sum of a series actually means. Still, to the extent that it is important to be able to bracket series at will, and that it is more important to be able to perform arithmetic with them, one can arrive at two conclusions:
In fact, both of these statements can be made precise and formally proven, but only using well-defined mathematical concepts that arose in the 19th century. After the late 17th-century introduction of calculus in Europe
, but before the advent of modern rigor, the tension between these answers fueled what has been characterized as an "endless" and "violent" dispute between mathematician
s.
.
It can be shown that it is not valid to perform many seemingly innocuous operations on a series, such as reordering individual terms, unless the series is absolutely convergent
. Otherwise these operations can alter the result of summation. It's easy to see how terms of Grandi's series can be rearranged to arrive at any integer number, not only 0 or 1.
Mathematics
Mathematics is the study of quantity, space, structure, and change. Mathematicians seek out patterns and formulate new conjectures. Mathematicians resolve the truth or falsity of conjectures by mathematical proofs, which are arguments sufficient to convince other mathematicians of their validity...
, the infinite series 1 − 1 + 1 − 1 + …, also written
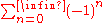
is sometimes called Grandi's series, after Italian mathematician, philosopher, and priest Guido Grandi
Guido Grandi
thumb|Guido GrandiDom Guido Grandi, O.S.B. Cam., was an Italian monk, priest, philosopher, mathematician, and engineer.-Life:...
, who gave a memorable treatment of the series in 1703. It is a divergent series
Divergent series
In mathematics, a divergent series is an infinite series that is not convergent, meaning that the infinite sequence of the partial sums of the series does not have a limit....
, meaning that it lacks a sum in the usual sense. On the other hand, its Cesàro sum
Cesàro summation
In mathematical analysis, Cesàro summation is an alternative means of assigning a sum to an infinite series. If the series converges in the usual sense to a sum A, then the series is also Cesàro summable and has Cesàro sum A...
is 1/2.
Heuristics
One obvious method to attack the series- 1 − 1 + 1 − 1 + 1 − 1 + 1 − 1 + …
is to treat it like a telescoping series and perform the subtractions in place:
+ (1 − 1) + (1 − 1) + … = 0 + 0 + 0 + … = 0.
On the other hand, a similar bracketing procedure leads to the apparently contradictory result
- 1 + (−1 + 1) + (−1 + 1) + (−1 + 1) + … = 1 + 0 + 0 + 0 + … = 1.
Thus, by applying parentheses to Grandi's series in different ways, one can obtain either 0 or 1 as a "value". (Variations of this idea, called the Eilenberg–Mazur swindle
Eilenberg–Mazur swindle
In mathematics, the Eilenberg–Mazur swindle, named after Samuel Eilenberg and Barry Mazur, is a method of proof that involves paradoxical properties of infinite sums. In geometric topology it was introduced by and is often called the Mazur swindle...
, are sometimes used in knot theory and algebra.)
Treating Grandi's series as a divergent geometric series we may use the same algebraic methods that evaluate convergent geometric series to obtain a third value:
- S = 1 − 1 + 1 − 1 + …, so
- 1 − S = 1 − (1 − 1 + 1 − 1 + …) = 1 − 1 + 1 − 1 + … = S,
resulting in S = 1/2.
The same conclusion results from calculating −S, subtracting the result from S, and solving 2S = 1.
The above manipulations do not consider what the sum of a series actually means. Still, to the extent that it is important to be able to bracket series at will, and that it is more important to be able to perform arithmetic with them, one can arrive at two conclusions:
- The series 1 − 1 + 1 − 1 + … has no sum.
- ...but its sum should be 1/2.
In fact, both of these statements can be made precise and formally proven, but only using well-defined mathematical concepts that arose in the 19th century. After the late 17th-century introduction of calculus in Europe
History of calculus
Calculus, historically known as infinitesimal calculus, is a mathematical discipline focused on limits, functions, derivatives, integrals, and infinite series. Ideas leading up to the notions of function, derivative, and integral were developed throughout the 17th century, but the decisive step was...
, but before the advent of modern rigor, the tension between these answers fueled what has been characterized as an "endless" and "violent" dispute between mathematician
Mathematician
A mathematician is a person whose primary area of study is the field of mathematics. Mathematicians are concerned with quantity, structure, space, and change....
s.
Divergence
In modern mathematics, the sum of an infinite series is defined to be the limit of the sequence of its partial sums, if it exists. The sequence of partial sums of Grandi's series is which clearly does not approach any number (although it does have two accumulation points at 0 and 1). Therefore, Grandi's series is divergentDivergent series
In mathematics, a divergent series is an infinite series that is not convergent, meaning that the infinite sequence of the partial sums of the series does not have a limit....
.
It can be shown that it is not valid to perform many seemingly innocuous operations on a series, such as reordering individual terms, unless the series is absolutely convergent
Absolute convergence
In mathematics, a series of numbers is said to converge absolutely if the sum of the absolute value of the summand or integrand is finite...
. Otherwise these operations can alter the result of summation. It's easy to see how terms of Grandi's series can be rearranged to arrive at any integer number, not only 0 or 1.
- E. W. Hobson, The theory of functions of a real variable and the theory of Fourier's series (Cambridge University Press, 1907), section 331. The University of Michigan Historical Mathematics Collection http://www.hti.umich.edu/u/umhistmath/
- E. T. Whittaker and G. N. Watson, A course of modern analysis, 4th edition, reprinted (Cambridge University Press, 1962), section 2.1.

