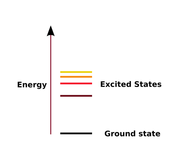
The ground state of a quantum mechanical

Quantum mechanics

Quantum mechanics, also known as quantum physics or quantum theory, is a branch of physics providing a mathematical description of much of the dual particle-like and wave-like behavior and interactions of energy and matter. It departs from classical mechanics primarily at the atomic and subatomic...

 system is its lowest-energy

Energy

In physics, energy is an indirectly observed quantity. It is often understood as the ability a physical system has to do work on other physical systems...

 state

Stationary state

In quantum mechanics, a stationary state is an eigenvector of the Hamiltonian, implying the probability density associated with the wavefunction is independent of time . This corresponds to a quantum state with a single definite energy...

; the energy of the ground state is known as the zero-point energy

Zero-point energy

Zero-point energy is the lowest possible energy that a quantum mechanical physical system may have; it is the energy of its ground state. All quantum mechanical systems undergo fluctuations even in their ground state and have an associated zero-point energy, a consequence of their wave-like nature...

 of the system. An excited state

Excited state

Excitation is an elevation in energy level above an arbitrary baseline energy state. In physics there is a specific technical definition for energy level which is often associated with an atom being excited to an excited state....

 is any state with energy greater than the ground state. The ground state of a quantum field theory

Quantum field theory

Quantum field theory provides a theoretical framework for constructing quantum mechanical models of systems classically parametrized by an infinite number of dynamical degrees of freedom, that is, fields and many-body systems. It is the natural and quantitative language of particle physics and...

 is usually called the vacuum state

Vacuum state

In quantum field theory, the vacuum state is the quantum state with the lowest possible energy. Generally, it contains no physical particles...

 or the vacuum.

If more than one ground state exists, they are said to be degenerate

Degenerate energy level

In physics, two or more different quantum states are said to be degenerate if they are all at the same energy level. Statistically this means that they are all equally probable of being filled, and in Quantum Mechanics it is represented mathematically by the Hamiltonian for the system having more...

. Many systems have degenerate ground states. It turns out that degeneracy occurs whenever a nontrivial

Nontrivial

Nontrivial is the opposite of trivial. In contexts where trivial has a formal meaning, nontrivial is its antonym.It is a term common among communities of engineers and mathematicians, to indicate a statement or theorem that is not obvious or easy to prove.-Examples:*In mathematics, it is often...

 unitary operator

Unitary operator

In functional analysis, a branch of mathematics, a unitary operator is a bounded linear operator U : H → H on a Hilbert space H satisfyingU^*U=UU^*=I...

 commutes

Commutator

In mathematics, the commutator gives an indication of the extent to which a certain binary operation fails to be commutative. There are different definitions used in group theory and ring theory.-Group theory:...

 with the Hamiltonian

Hamiltonian (quantum mechanics)

In quantum mechanics, the Hamiltonian H, also Ȟ or Ĥ, is the operator corresponding to the total energy of the system. Its spectrum is the set of possible outcomes when one measures the total energy of a system...

 of the system.

According to the third law of thermodynamics

Third law of thermodynamics

The third law of thermodynamics is a statistical law of nature regarding entropy:For other materials, the residual entropy is not necessarily zero, although it is always zero for a perfect crystal in which there is only one possible ground state.-History:...

, a system at absolute zero

Absolute zero

Absolute zero is the theoretical temperature at which entropy reaches its minimum value. The laws of thermodynamics state that absolute zero cannot be reached using only thermodynamic means....

 temperature

Temperature

Temperature is a physical property of matter that quantitatively expresses the common notions of hot and cold. Objects of low temperature are cold, while various degrees of higher temperatures are referred to as warm or hot...

 exists in its ground state; thus, its entropy

Entropy

Entropy is a thermodynamic property that can be used to determine the energy available for useful work in a thermodynamic process, such as in energy conversion devices, engines, or machines. Such devices can only be driven by convertible energy, and have a theoretical maximum efficiency when...

 is determined by the degeneracy of the ground state. Many systems, such as a perfect crystal lattice, have a unique ground state and therefore have zero entropy at absolute zero because the logarithm

Logarithm

The logarithm of a number is the exponent by which another fixed value, the base, has to be raised to produce that number. For example, the logarithm of 1000 to base 10 is 3, because 1000 is 10 to the power 3: More generally, if x = by, then y is the logarithm of x to base b, and is written...

 of 1 is 0. It is also possible for the highest excited state to have absolute zero

Absolute zero

Absolute zero is the theoretical temperature at which entropy reaches its minimum value. The laws of thermodynamics state that absolute zero cannot be reached using only thermodynamic means....

 temperature

Temperature

Temperature is a physical property of matter that quantitatively expresses the common notions of hot and cold. Objects of low temperature are cold, while various degrees of higher temperatures are referred to as warm or hot...

 for systems that exhibit negative temperature

Negative temperature

In physics, certain systems can achieve negative temperatures; that is, their thermodynamic temperature can be a negative quantity. Negative temperatures can be expressed as negative numbers on the kelvin scale....

.

Examples

• The wave function of the ground state of a particle in a one-dimensional well
  Particle in a box
  In quantum mechanics, the particle in a box model describes a particle free to move in a small space surrounded by impenetrable barriers. The model is mainly used as a hypothetical example to illustrate the differences between classical and quantum systems...
  
   is a half-period sine wave which goes to zero at the two edges of the well. The energy of the particle is given by , where h is the Planck constant
  Planck constant
  The Planck constant , also called Planck's constant, is a physical constant reflecting the sizes of energy quanta in quantum mechanics. It is named after Max Planck, one of the founders of quantum theory, who discovered it in 1899...
  
  , m is the mass of the particle, n is the energy state (n = 1 corresponds to the ground-state energy), and L is the width of the well.

• The wave function of the ground state of a hydrogen atom is a spherically-symmetric distribution centred on the nucleus
  Atomic nucleus
  The nucleus is the very dense region consisting of protons and neutrons at the center of an atom. It was discovered in 1911, as a result of Ernest Rutherford's interpretation of the famous 1909 Rutherford experiment performed by Hans Geiger and Ernest Marsden, under the direction of Rutherford. The...
  
  , which is largest at the center and reduces exponentially
  Exponential distribution
  In probability theory and statistics, the exponential distribution is a family of continuous probability distributions. It describes the time between events in a Poisson process, i.e...
  
   at larger distances. The electron
  Electron
  The electron is a subatomic particle with a negative elementary electric charge. It has no known components or substructure; in other words, it is generally thought to be an elementary particle. An electron has a mass that is approximately 1/1836 that of the proton...
  
   is most likely to be found at a distance from the nucleus equal to the Bohr radius
  Bohr radius
  The Bohr radius is a physical constant, approximately equal to the most probable distance between the proton and electron in a hydrogen atom in its ground state. It is named after Niels Bohr, due to its role in the Bohr model of an atom...
  
  . This function is known as the 1s atomic orbital
  Atomic orbital
  An atomic orbital is a mathematical function that describes the wave-like behavior of either one electron or a pair of electrons in an atom. This function can be used to calculate the probability of finding any electron of an atom in any specific region around the atom's nucleus...
  
  . For hydrogen (H), an electron in the ground state has energy , relative to 0.0 eV when the H atom is ionized
  Ionization energy
  The ionization energy of a chemical species, i.e. an atom or molecule, is the energy required to remove an electron from the species to a practically infinite distance. Large atoms or molecules have a low ionization energy, while small molecules tend to have higher ionization energies.The property...
  
  , e.g. the electron is completely removed.

• The exact definition of one second
  Second
  The second is a unit of measurement of time, and is the International System of Units base unit of time. It may be measured using a clock....
  
   of time
  Time
  Time is a part of the measuring system used to sequence events, to compare the durations of events and the intervals between them, and to quantify rates of change such as the motions of objects....
  
   since 1997 has been