
Homothetic vector field
Encyclopedia
A homothetic vector field (sometimes homothetic collineation or homothety) is a projective vector field
which satisfies the condition:
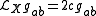
where c is a real constant. Homothetic vector fields find application in the study of singularities
in general relativity.
Projective vector field
A projective vector field is a smooth vector field on a semi Riemannian manifold M whose flow preserves the geodesic structure of M without necessarily preserving the affine parameter of any geodesic...
which satisfies the condition:
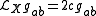
where c is a real constant. Homothetic vector fields find application in the study of singularities
Gravitational singularity
A gravitational singularity or spacetime singularity is a location where the quantities that are used to measure the gravitational field become infinite in a way that does not depend on the coordinate system...
in general relativity.
See also
- Affine vector fieldAffine vector fieldAn affine vector field is a projective vector field preserving geodesics and preserving the affine parameter...
- Curvature collineation
- Killing vector fieldKilling vector fieldIn mathematics, a Killing vector field , named after Wilhelm Killing, is a vector field on a Riemannian manifold that preserves the metric. Killing fields are the infinitesimal generators of isometries; that is, flows generated by Killing fields are continuous isometries of the manifold...
- Matter collineation
- Spacetime symmetriesSpacetime symmetriesSpacetime symmetries are features of spacetime that can be described as exhibiting some form of symmetry. The role of symmetry in physics is important in simplifying solutions to many problems, spacetime symmetries finding ample application in the study of exact solutions of Einstein's field...

