Homotopy fiber
Encyclopedia
In mathematics
, especially homotopy theory, the homotopy fiber is part of a construction that associates a fibration
to an arbitrary continuous function
of topological spaces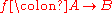
.
In particular, given such a map, define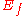
to be the set of pairs 
where 
and 
is a path such that 
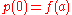. We give 
a topology by giving it the subspace topology as a subset of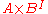 
(where 
is the space of paths in 
which as a function space
has the compact-open topology
). Then the map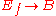
given by 
is a fibration. Furthermore, 
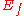is homotopy equivalent to 
as follows: Embed 
as a subspace of 
by 
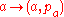where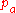 
is the constant path at 
. Then 
deformation retract
s to this subspace by contracting the paths.
The fiber of this fibration (which is only well-defined up to homotopy equivalence) is the homotopy fiber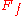
, which can be defined as the set of all 
with 
and 
a path such that 
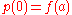and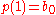 
, where 
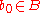is some fixed basepoint of 
.
Mathematics
Mathematics is the study of quantity, space, structure, and change. Mathematicians seek out patterns and formulate new conjectures. Mathematicians resolve the truth or falsity of conjectures by mathematical proofs, which are arguments sufficient to convince other mathematicians of their validity...
, especially homotopy theory, the homotopy fiber is part of a construction that associates a fibration
Fibration
In topology, a branch of mathematics, a fibration is a generalization of the notion of a fiber bundle. A fiber bundle makes precise the idea of one topological space being "parameterized" by another topological space . A fibration is like a fiber bundle, except that the fibers need not be the same...
to an arbitrary continuous function
Continuous function
In mathematics, a continuous function is a function for which, intuitively, "small" changes in the input result in "small" changes in the output. Otherwise, a function is said to be "discontinuous". A continuous function with a continuous inverse function is called "bicontinuous".Continuity of...
of topological spaces
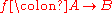.In particular, given such a map, define
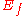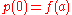to be the set of pairs where and is a path such that . We give a topology by giving it the subspace topology as a subset of 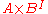(where is the space of paths in which as a function spaceFunction space
In mathematics, a function space is a set of functions of a given kind from a set X to a set Y. It is called a space because in many applications it is a topological space, a vector space, or both.-Examples:...
has the compact-open topology
Compact-open topology
In mathematics, the compact-open topology is a topology defined on the set of continuous maps between two topological spaces. The compact-open topology is one of the commonly-used topologies on function spaces, and is applied in homotopy theory and functional analysis...
). Then the map
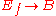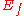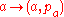given by is a fibration. Furthermore, is homotopy equivalent to as follows: Embed as a subspace of by where 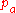is the constant path at . Then deformation retractDeformation retract
In topology, a branch of mathematics, a retraction , as the name suggests, "retracts" an entire space into a subspace. A deformation retraction is a map which captures the idea of continuously shrinking a space into a subspace.- Retract :...
s to this subspace by contracting the paths.
The fiber of this fibration (which is only well-defined up to homotopy equivalence) is the homotopy fiber
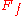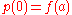, which can be defined as the set of all with and a path such that and 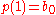, where 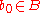is some fixed basepoint of .