In geometry

Geometry

Geometry arose as the field of knowledge dealing with spatial relationships. Geometry was one of the two fields of pre-modern mathematics, the other being the study of numbers ....

, an inscribed planar

Planar

In computer graphics, planar is the method of representing pixel colours with several bitplanes of RAM. Each bit in a bitplane is related to one pixel on the screen...

 shape

Shape

The shape of an object located in some space is a geometrical description of the part of that space occupied by the object, as determined by its external boundary – abstracting from location and orientation in space, size, and other properties such as colour, content, and material...

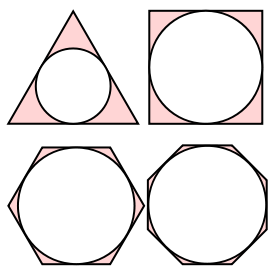
 or solid is one that is enclosed by and "fits snugly" inside another geometric shape or solid. To say that "Figure F is inscribed in figure G" means precisely the same thing as "figure G is circumscribed about figure F". A circle or ellipse inscribed in a convex polygon (or a sphere or ellipsoid inscribed in a convex polyhedron

Polyhedron

In elementary geometry a polyhedron is a geometric solid in three dimensions with flat faces and straight edges...

) is tangent to every side of the outer figure (but see Inscribed sphere

Inscribed sphere

In geometry, the inscribed sphere or insphere of a convex polyhedron is a sphere that is contained within the polyhedron and tangent to each of the polyhedron's faces...

 for semantic variants). A polygon inscribed in a circle, ellipse, or polygon (or a polyhedron inscribed in a sphere, ellipsoid, or polyhedron) has each vertex on the outer figure; if the outer figure is a polygon or polyhedron, there must be a vertex of the inscribed polygon or polyhedron on each side of the outer figure. Familiar examples include circle

Circle

A circle is a simple shape of Euclidean geometry consisting of those points in a plane that are a given distance from a given point, the centre. The distance between any of the points and the centre is called the radius....

s inscribed in triangle

Triangle

A triangle is one of the basic shapes of geometry: a polygon with three corners or vertices and three sides or edges which are line segments. A triangle with vertices A, B, and C is denoted ....

s or regular polygon

Regular polygon

A regular polygon is a polygon that is equiangular and equilateral . Regular polygons may be convex or star.-General properties:...

s, and triangles or regular polygons inscribed in circles.

An inscribed figure is not necessarily unique in orientation; this can easily be seen, for example, when the given outer figure is a circle, in which case a rotation of an inscribed figure gives another inscribed figure that is congruent to the original one.

The definition given above assumes that the objects concerned are embedded in two- or three-dimension

Dimension

In physics and mathematics, the dimension of a space or object is informally defined as the minimum number of coordinates needed to specify any point within it. Thus a line has a dimension of one because only one coordinate is needed to specify a point on it...

al Euclidean space

Euclidean space

In mathematics, Euclidean space is the Euclidean plane and three-dimensional space of Euclidean geometry, as well as the generalizations of these notions to higher dimensions...

, but can easily be generalized to higher dimensions and other metric space

Metric space

In mathematics, a metric space is a set where a notion of distance between elements of the set is defined.The metric space which most closely corresponds to our intuitive understanding of space is the 3-dimensional Euclidean space...

s.

The inradius or filling radius

Filling radius

In Riemannian geometry, the filling radius of a Riemannian manifold X is a metric invariant of X. It was originally introduced in 1983 by Mikhail Gromov, who used it to prove his systolic inequality for essential manifolds, vastly generalizing Loewner's torus inequality and Pu's inequality for the...

 of a given outer figure is the radius

Radius

In classical geometry, a radius of a circle or sphere is any line segment from its center to its perimeter. By extension, the radius of a circle or sphere is the length of any such segment, which is half the diameter. If the object does not have an obvious center, the term may refer to its...

 of the inscribed circle or sphere, if it exists.

Facts about inscribed figures

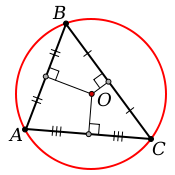
• Every circle has an inscribed triangle with any three given angle
  Angle
  In geometry, an angle is the figure formed by two rays sharing a common endpoint, called the vertex of the angle.Angles are usually presumed to be in a Euclidean plane with the circle taken for standard with regard to direction. In fact, an angle is frequently viewed as a measure of an circular arc...
  
   measures (summing of course to 180°), and every triangle can be inscribed in some circle (which is called its circumscribed circle
  Circumscribed circle
  In geometry, the circumscribed circle or circumcircle of a polygon is a circle which passes through all the vertices of the polygon. The center of this circle is called the circumcenter....
  
  ).

• Every triangle has an inscribed circle, called the incircle
  Incircle and excircles of a triangle
  In geometry, the incircle or inscribed circle of a triangle is the largest circle contained in the triangle; it touches the three sides...
  
  .

• Every circle has an inscribed regular polygon of n sides, for any n≥3, and every regular polygon can be inscribed in some circle.

• Every regular polygon has an inscribed circle, and every circle can be inscribed in some regular polygon of n sides, for any n≥3.

• Every triangle has an infinitude of inscribed ellipse
  Ellipse
  In geometry, an ellipse is a plane curve that results from the intersection of a cone by a plane in a way that produces a closed curve. Circles are special cases of ellipses, obtained when the cutting plane is orthogonal to the cone's axis...
  
  s. One of them is a circle, and one of them is the Steiner inellipse
  Steiner inellipse
  In geometry, the Steiner inellipse of a triangle is the unique ellipse inscribed in the triangle and tangent to the sides at their midpoints. It is an example of an inconic. By comparison the inscribed circle of a triangle is another inconic that is tangent to the sides, but not necessarily at the...
  
   which is tangent to the triangle at the midpoints of the sides.

• Every triangle has three inscribed squares, though two of them coincide with each other in the case of a right triangle.