In
geometryGeometry arose as the field of knowledge dealing with spatial relationships. Geometry was one of the two fields of pre-modern mathematics, the other being the study of numbers ....
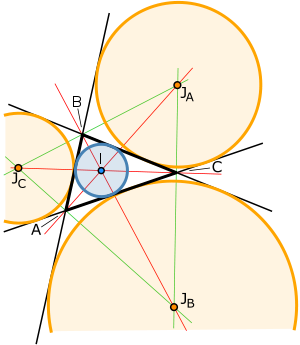
, the
incircle or
inscribed circle of a
triangleA triangle is one of the basic shapes of geometry: a polygon with three corners or vertices and three sides or edges which are line segments. A triangle with vertices A, B, and C is denoted ....
is the largest
circleA circle is a simple shape of Euclidean geometry consisting of those points in a plane that are a given distance from a given point, the centre. The distance between any of the points and the centre is called the radius....
contained in the triangle; it touches (is
tangentIn geometry, the tangent line to a plane curve at a given point is the straight line that "just touches" the curve at that point. More precisely, a straight line is said to be a tangent of a curve at a point on the curve if the line passes through the point on the curve and has slope where f...
to) the three sides. The center of the incircle is called the triangle's
incenter.
An
excircle or
escribed circle of the triangle is a circle lying outside the triangle, tangent to one of its sides and tangent to the extensions of the other two.
Every triangle has three distinct excircles, each tangent to one of the triangle's sides.
The center of the incircle can be found as the intersection of the three internal angle bisectors.
The center of an excircle is the intersection of the internal bisector of one angle and the external bisectors of the other two. Because the internal bisector of an angle is perpendicular to its external bisector, it follows that the center of the incircle together with the three excircle centers form an
orthocentric systemIn geometry, an orthocentric system is a set of four points in the plane one of which is the orthocenter of the triangle formed by the other three....
.
See also
Tangent lines to circlesIn Euclidean plane geometry, tangent lines to circles form the subject of several theorems, and play an important role in many geometrical constructions and proofs...
.
Relation to area of the triangle
The radii of the in- and excircles are closely related to the
areaArea is a quantity that expresses the extent of a two-dimensional surface or shape in the plane. Area can be understood as the amount of material with a given thickness that would be necessary to fashion a model of the shape, or the amount of paint necessary to cover the surface with a single coat...
of the triangle. Let
K be the triangle's area and let
a,
b and
c, be the lengths of its sides. By
Heron's formula, the area of the triangle is
where
is the semiperimeter and
P = 2
s is the perimeter.
The radius of the incircle (also known as the
inradius,
r ) is
-
Thus, the area
K of a triangle may be found by multiplying the inradius by the semiperimeter:
The radii in the excircles are called the
exradii. The excircle at side
a has radius
-
Similarly the radii of the excircles at sides
b and
c are respectively
-
and
-
From these formulas one can see that the excircles are always larger than the incircle and that the largest excircle is the one tangent to the longest side and the smallest excircle is tangent to the shortest side. Further, combining these formulas with Heron's area formula yields the result that
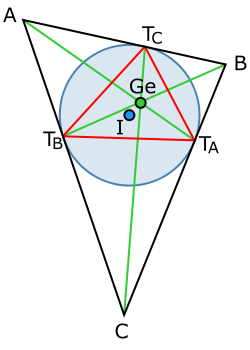
Nine-point circle and Feuerbach point
The circle tangent to all three of the excircles as well as the incircle is known as the
nine-point circleIn geometry, the nine-point circle is a circle that can be constructed for any given triangle. It is so named because it passes through nine significant points defined from the triangle...
. The point where the nine-point circle touches the incircle is known as the Feuerbach point.
Gergonne triangle and point
The
Gergonne triangle of
ABC is denoted by the vertices
TA,
TB and
TC that are the three points where the incircle touches the reference triangle
ABC and where
TA is opposite of
A, etc. This triangle
TATBTC is also known as the
contact triangle or
intouch triangle of
ABC. The incircle of
ABC is the circumcircle of
TATBTC. The three lines
ATA,
BTB and
CTC intersect in a single point, the triangle's
Gergonne point Ge -
X(7)In geometry a triangle center is a point in the plane that is in some sense a center of a triangle akin to the centers of squares and circles. For example the centroid, circumcenter, incenter and orthocenter were familiar to the ancient Greeks, and can be obtained by simple constructions. Each of...
. Interestingly, the Gergonne point of a triangle is the symmedian point of its Gergonne triangle. For a full set of properties of the Gergonne point see.
The touchpoints of the excircle with segments
BC,CA,AB are the vertices of the
extouch triangle. The points of intersection of the interior angle bisectors of
ABC with the segments
BC,CA,AB are the vertices of the
incentral triangle.
Nagel triangle and point
The
Nagel triangle of
ABC is denoted by the vertices
XA,
XB and
XC that are the three points where the excircles touches the reference triangle
ABC and where
XA is opposite of
A, etc. This triangle
XAXBXC is also known as the
extouch triangle of
ABC. The circumcircle of the extouch triangle
XAXBXC is called the
Mandart circle. The three lines
AXA,
BXB and
CXC intersect in a single point, the triangle's
Nagel pointIn geometry, the Nagel point is a point associated with any triangle. Given a triangle ABC, let TA, TB, and TC be the extouch points in which the A-excircle meets line BC, the B-excircle meets line CA, and C-excircle meets line AB, respectively...
Na -
X(8)In geometry a triangle center is a point in the plane that is in some sense a center of a triangle akin to the centers of squares and circles. For example the centroid, circumcenter, incenter and orthocenter were familiar to the ancient Greeks, and can be obtained by simple constructions. Each of...
.
Trilinear coordinatesIn geometry, the trilinear coordinates of a point relative to a given triangle describe the relative distances from the three sides of the triangle. Trilinear coordinates are an example of homogeneous coordinates...
for the vertices of the intouch triangle are given by
Trilinear coordinatesIn geometry, the trilinear coordinates of a point relative to a given triangle describe the relative distances from the three sides of the triangle. Trilinear coordinates are an example of homogeneous coordinates...
for the vertices of the extouch triangle are given by
Trilinear coordinatesIn geometry, the trilinear coordinates of a point relative to a given triangle describe the relative distances from the three sides of the triangle. Trilinear coordinates are an example of homogeneous coordinates...
for the vertices of the incentral triangle are given by
Trilinear coordinatesIn geometry, the trilinear coordinates of a point relative to a given triangle describe the relative distances from the three sides of the triangle. Trilinear coordinates are an example of homogeneous coordinates...
for the vertices of the excentral triangle are given by
Trilinear coordinatesIn geometry, the trilinear coordinates of a point relative to a given triangle describe the relative distances from the three sides of the triangle. Trilinear coordinates are an example of homogeneous coordinates...
for the Gergonne point are given by
-
,
or, equivalently, by the
Law of SinesIn trigonometry, the law of sines is an equation relating the lengths of the sides of an arbitrary triangle to the sines of its angles...
,
-
.
Trilinear coordinatesIn geometry, the trilinear coordinates of a point relative to a given triangle describe the relative distances from the three sides of the triangle. Trilinear coordinates are an example of homogeneous coordinates...
for the Nagel point are given by
-
,
or, equivalently, by the
Law of SinesIn trigonometry, the law of sines is an equation relating the lengths of the sides of an arbitrary triangle to the sines of its angles...
,
-
.
It is the isotomic conjugate of the Gergonne point.
Coordinates of the incenter
The Cartesian coordinates of the incenter are a weighted average of the coordinates of the three vertices using the side lengths of the triangle as weights. (The weights are positive so the incenter lies inside the triangle as stated above.) If the three vertices are located at
,
, and
, and the sides opposite these vertices have corresponding lengths
,
, and
, then the incenter is at
where
- Trilinear coordinates
In geometry, the trilinear coordinates of a point relative to a given triangle describe the relative distances from the three sides of the triangle. Trilinear coordinates are an example of homogeneous coordinates...
for the incenter are given by
-
- Barycentric coordinates
In geometry, the barycentric coordinate system is a coordinate system in which the location of a point is specified as the center of mass, or barycenter, of masses placed at the vertices of a simplex . Barycentric coordinates are a form of homogeneous coordinates...
for the incenter are given by
Equations for four circles
Let x : y : z be a variable point in
trilinear coordinatesIn geometry, the trilinear coordinates of a point relative to a given triangle describe the relative distances from the three sides of the triangle. Trilinear coordinates are an example of homogeneous coordinates...
, and let u = cos
2(A/2), v = cos
2(B/2), w = cos
2(C/2). The four circles described above are given by these equations:
-
Other incircle properties
Suppose the tangency points of the incircle divide the sides into lengths of
x and
y,
y and
z, and
z and
x. Then the incircle has the radius
If the altitudes from sides of lengths
a,
b, and
c are
ha,
hb, and
hc then the inradius
r is one-third of the harmonic mean of these altitudes, i.e.
The distance between the circumcenter and the incenter is
where
r is the incircle radius and
R is the circumcircle radius. Thus the incircle radius is no larger than half the circumcircle radius (Euler's triangle inequality).
The product of the incircle radius and the circumcircle radius of a triangle with sides
a,
b, and
c is
Any line through a triangle that splits both the triangle's area and its perimeter in half goes through the triangle's incenter (the center of its incircle). There are either one, two, or three of these for any given triangle.
See also
- Altitude (triangle)
In geometry, an altitude of a triangle is a straight line through a vertex and perpendicular to a line containing the base . This line containing the opposite side is called the extended base of the altitude. The intersection between the extended base and the altitude is called the foot of the...
- Circumscribed circle
In geometry, the circumscribed circle or circumcircle of a polygon is a circle which passes through all the vertices of the polygon. The center of this circle is called the circumcenter....
- Ex-tangential quadrilateral
In Euclidean geometry, an ex-tangential quadrilateral is a convex quadrilateral where the extensions of all four sides are tangent to a circle outside the quadrilateral. It has also been called an exscriptible quadrilateral. The circle is called its excircle or its escribed circle, its radius the...
- Inscribed sphere
In geometry, the inscribed sphere or insphere of a convex polyhedron is a sphere that is contained within the polyhedron and tangent to each of the polyhedron's faces...
- Power of a point
In elementary plane geometry, the power of a point is a real number h that reflects the relative distance of a given point from a given circle. Specifically, the power of a point P with respect to a circle C of radius r is defined...
- Steiner inellipse
In geometry, the Steiner inellipse of a triangle is the unique ellipse inscribed in the triangle and tangent to the sides at their midpoints. It is an example of an inconic. By comparison the inscribed circle of a triangle is another inconic that is tangent to the sides, but not necessarily at the...
- Tangential quadrilateral
In Euclidean geometry, a tangential quadrilateral or circumscribed quadrilateral is a convex quadrilateral whose sides all lie tangent to a single circle inscribed within the quadrilateral. This circle is called the incircle...
- Triangle center
In geometry a triangle center is a point in the plane that is in some sense a center of a triangle akin to the centers of squares and circles. For example the centroid, circumcenter, incenter and orthocenter were familiar to the ancient Greeks, and can be obtained by simple constructions. Each of...
External links
Interactive