
Invariant of a binary form
Encyclopedia
In mathematical invariant theory
, an invariant of a binary form is a polynomial in the coefficients of a binary form
in two variables x and y that remains invariant under unimodular transformations of the variables x and y.
In terms of representation theory, given any representation V of the group SL2(C) one can ask for the ring of invariant polynomials on V. Invariants of a binary form of degree n correspond to taking V to be the (n + 1)-dimensional irreducible representation, and covariants correspond to taking V to be the sum of the irreducible representations of dimensions 2 and n + 1.
The invariants of a binary form are a graded algebra, and proved that this algebra is finitely generated if the base field is the complex numbers.
Forms of degrees 2, 3, 4, 5, 6, 7, 8, 9, 10 are sometimes called quadrics, cubic, quartics, quintics, sextics, septics, octavics, nonics, and decimics. "Quantic" is an old name for a form of arbitrary degree. Forms in 1, 2, 3, ... variables are called unary, binary, ternary, ... forms.
The discriminant
of a form is an invariant.
The resultant of two forms is a simultaneous invariant of them.
The Hessian covariant of a form is the determinant of the Hessian matrix
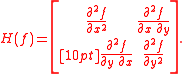
It is a covariant of order 2n− 4 and degree 2.
The catalecticant
is an invariant of a form of even degree.
The Jacobian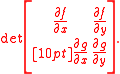
is a simultaneous invariant of two forms f, g.
The number of generators for invariants and covariants of binary forms can be found in and , respectively.
Invariant theory
Invariant theory is a branch of abstract algebra dealing with actions of groups on algebraic varieties from the point of view of their effect on functions...
, an invariant of a binary form is a polynomial in the coefficients of a binary form
Binary form
Binary form is a musical form in two related sections, both of which are usually repeated. Binary is also a structure used to choreograph dance....
in two variables x and y that remains invariant under unimodular transformations of the variables x and y.
Terminology
A binary form (of degree n) is a homogeneous polynomial Σ an−ixn−iyi = anxn + an−1xn−1y + ... + a0yn. The group SL2(C) acts on these forms by taking x to ax + by and y to cx + dy. This induces an action on the space spanned by a0, ..., an and on the polynomials in these variables. An invariant is a polynomial in these n + 1 variables a0, ..., an that is invariant under this action. More generally a covariant is a polynomial in a0, ..., an, x, y that is invariant, so an invariant is a special case of a covariant where the variables x and y do not occur. More generally still, a simultaneous invariant is a polynomial in the coefficients of several different forms in x and y.In terms of representation theory, given any representation V of the group SL2(C) one can ask for the ring of invariant polynomials on V. Invariants of a binary form of degree n correspond to taking V to be the (n + 1)-dimensional irreducible representation, and covariants correspond to taking V to be the sum of the irreducible representations of dimensions 2 and n + 1.
The invariants of a binary form are a graded algebra, and proved that this algebra is finitely generated if the base field is the complex numbers.
Forms of degrees 2, 3, 4, 5, 6, 7, 8, 9, 10 are sometimes called quadrics, cubic, quartics, quintics, sextics, septics, octavics, nonics, and decimics. "Quantic" is an old name for a form of arbitrary degree. Forms in 1, 2, 3, ... variables are called unary, binary, ternary, ... forms.
Examples
A form f is itself is a covariant of degree 1 and order n.The discriminant
Discriminant
In algebra, the discriminant of a polynomial is an expression which gives information about the nature of the polynomial's roots. For example, the discriminant of the quadratic polynomialax^2+bx+c\,is\Delta = \,b^2-4ac....
of a form is an invariant.
The resultant of two forms is a simultaneous invariant of them.
The Hessian covariant of a form is the determinant of the Hessian matrix
Hessian matrix
In mathematics, the Hessian matrix is the square matrix of second-order partial derivatives of a function; that is, it describes the local curvature of a function of many variables. The Hessian matrix was developed in the 19th century by the German mathematician Ludwig Otto Hesse and later named...
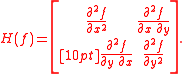
It is a covariant of order 2n− 4 and degree 2.
The catalecticant
Catalecticant
In mathematical invariant theory, the catalecticant of a binary form of degree 2n is a polynomial in its coefficients that vanishes when the binary form is a sum of at most n powers . It was introduced by ; see...
is an invariant of a form of even degree.
The Jacobian
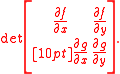
is a simultaneous invariant of two forms f, g.
The ring of invariants
The structure of the ring of invariants has been worked out for small degrees. gave tables of the numbers of generators of invariants and covariants for forms of degree up to 10.- For linear forms ax + by the only invariants are constants. The algebra of covariants is generated by the form itself of degree 1 and order 1.
- The algebra of invariants of the quadratic form ax2 + 2bxy + cy2 is a polynomial algebra in 1 variable generated by the discriminant b2 − ac of degree 2. The algebra of covariants is a polynomial algebra in 2 variables generated by the discriminant together with the form f itself (of degree 1 and order 2).
- The algebra of invariants of the cubic form ax3 + 3bx2y + 3cxy2 + dy3 is a polynomial algebra in 1 variable generated by the discriminant D = 3b2c2 + 6abcd − 4b3d − 4c3a − a2d2 of degree 4. The algebra of covariants is generated by the discriminant, the form itself (degree 1, order 3), the Hessian H (degree 2, order 2) and a covariant T of degree 3 and order 3. They are related by the syzygy 4h3=Df2-T2 of degree 6 and order 6.
- The algebra of invariants of a quartic form is generated by invariants i, j of degrees 2, 3. The algebra of covariants is generated by these two invariants together with the form f of degree 1 and order 4, the Hessian H of degree 2 and order 4, and a covariant T of degree 3 and order 6. They are related by a syzygy jf3−Hf2i+4H3+T2=0 of degree 6 and order 12.
- The algebra of invariants of a quintic form was found by Sylvester and is generated by invariants of degree 4, 8, 12, 18. The generators of degrees 4, 8, 12 generate a polynomial ring, which contains the square of the generator of degree 18. The invariants are rather complicated to write out explicitly: Sylvester showed that the generators of degrees 4, 8, 12, 18 have 12, 59, 228, and 848 terms often with very large coefficients.
- The algebra of invariants of a sextic form is generated by invariants of degree 2, 4, 6, 10, 15. The generators of degrees 2, 4, 6, 10 generate a polynomial ring, which contains the square of the generator of degree 15.
- and showed that the algebra of invariants of a degree 7 form is generated by a set with 1 invariant of degree 4, 3 of degree 8, 6 of degree 12, 4 of degree 14, 2 of degree 16, 9 of degree 18, and one of each of the degrees 20, 22, 26, 30
- and showed that the algebra of invariants of a degree 8 form is generated by 9 invariants of degrees 2, 3, 4, 5, 6, 7, 8, 9, 10, and the ideal of relations between them is generated by elements of degrees 16, 17, 18, 19, 20.
- showed that the algebra of invariants of a degree 9 form is generated by 92 invariants
- showed that the algebra of invariants of a degree 10 form is generated by 106 invariants
The number of generators for invariants and covariants of binary forms can be found in and , respectively.

