
Langlands decomposition
Encyclopedia
In mathematics, the Langlands decomposition writes a parabolic subgroup P of a semisimple Lie group as a product 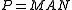 of a reductive subgroup M, an abelian
of a reductive subgroup M, an abelian
subgroup A, and a nilpotent
subgroup N.
, which leads to the Langlands program
: if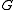 is a reductive algebraic group and
is a reductive algebraic group and 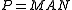 is the Langlands decomposition of a parabolic subgroup P, then parabolic induction
is the Langlands decomposition of a parabolic subgroup P, then parabolic induction
consists of taking a representation of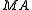 , extending it to
, extending it to 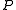 by letting
by letting 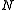 act trivially, and inducing
act trivially, and inducing
the result from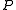 to
to  .
.
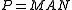 of a reductive subgroup M, an abelian
of a reductive subgroup M, an abelianAbelian group
In abstract algebra, an abelian group, also called a commutative group, is a group in which the result of applying the group operation to two group elements does not depend on their order . Abelian groups generalize the arithmetic of addition of integers...
subgroup A, and a nilpotent
Nilpotent
In mathematics, an element x of a ring R is called nilpotent if there exists some positive integer n such that xn = 0....
subgroup N.
Applications
A key application is in parabolic inductionParabolic induction
In mathematics, parabolic induction is a method of constructing representations of a reductive group from representations of its parabolic subgroups....
, which leads to the Langlands program
Langlands program
The Langlands program is a web of far-reaching and influential conjectures that relate Galois groups in algebraic number theory to automorphic forms and representation theory of algebraic groups over local fields and adeles. It was proposed by ....
: if
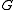 is a reductive algebraic group and
is a reductive algebraic group and 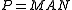 is the Langlands decomposition of a parabolic subgroup P, then parabolic induction
is the Langlands decomposition of a parabolic subgroup P, then parabolic inductionParabolic induction
In mathematics, parabolic induction is a method of constructing representations of a reductive group from representations of its parabolic subgroups....
consists of taking a representation of
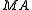 , extending it to
, extending it to 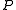 by letting
by letting 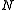 act trivially, and inducing
act trivially, and inducingInduced representation
In mathematics, and in particular group representation theory, the induced representation is one of the major general operations for passing from a representation of a subgroup H to a representation of the group G itself. It was initially defined as a construction by Frobenius, for linear...
the result from
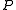 to
to  .
.

