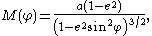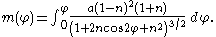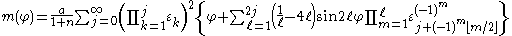Meridian arc
Encyclopedia
In geodesy
, a meridian arc measurement is a highly accurate determination of the distance between two points with the same longitude. Two or more such determinations at different locations then specify the shape of the reference ellipsoid
which best approximates the shape of the geoid
. This process is called the determination of the Figure of the Earth
. The earliest determinations of the size of a spherical Earth required a single arc. The latest determinations use astro-geodetic
measurements and the methods of satellite geodesy
to determine the reference ellipsoids.
s in the 9th century, but it was the Alexandrian scientist Eratosthenes
who first calculated the circumference a reasonably good approximate value for the radius. He knew that on the summer solstice
at local noon the sun
goes through the zenith
in the ancient Egyptian city of Syene (Assuan). He also knew from his own measurements that, at the same moment in his hometown of Alexandria
, the zenith distance was 1/50 of a full circle (7.2°).
Assuming that Alexandria was due north of Syene, Eratosthenes concluded that the distance between Alexandria and Syene must be 1/50 of Earth's circumference. Using data from caravan
travels, he estimated the distance to be 5000 stadia
(about 500 nautical miles)—which implies a circumference of 252,000 stadia. Assuming the Attic stadion (185 m) this corresponds to 46,620 km, or 16% too great. However, if Eratosthenes used the Egyptian stadion (157.5 m) his measurement turns out to be 39,690 km, an error of only 1%. Considering geometry and ancient conditions, a 16% error is more probable: Syene is not precisely on the Tropic of Cancer
and not directly south of Alexandria. The sun appears as a disk of 0.5°, and an estimate of the overland distance traveling along the Nile
or through the desert couldn't be more accurate than about 10%.
Eratosthenes' estimation of Earth’s size was accepted for nearly two thousand years. A similar method was used by Posidonius
about 150 years later, and slightly better results were calculated in AD 827 by the Gradmessung of the Caliph al-Ma'mun
.
The geographic latitudes of both end points, φs (standpoint) and φf (forepoint) and possibly at other points are determined by astrogeodesy, observing the zenith distances of sufficient numbers of star
s. If latitudes are measured at end points only, the radius of curvature at the mid-point of the meridian arc can be calculated from R = Δ'/(|φs-φf|). A second meridian arc will allow the derivation of two parameters required to specify a reference ellipsoid
. Longer arcs with intermediate latitude determinations can completely determine the ellipsoid. In practice multiple arc measurements are used to determine the ellipsoid parameters by the method of least squares. The parameters determined are usually the semi-major axis,
, and either the semi-minor axis, 
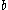, or the inverse flattening 
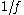, (where the flattening is 
).
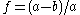over the period 1684–1718. The arc was measured with at least three latitude determinations, so they were able to deduce mean curvatures for the northern and southern halves of the arc, allowing a determination of the overall shape. The results indicated that the Earth was a prolate spheroid (with an equatorial radius less than the polar radius). (The history of the meridian arc from 1600 to 1880 is fully covered in the first chapter of Geodesy by Alexander Ross Clarke.).
To resolve the issue, the French Academy of Sciences
(1735) proposed expeditions to Peru (Bouguer
, Louis Godin
, de La Condamine
, Antonio de Ulloa
, Jorge Juan
) and Lappland (Maupertuis
, Clairaut, Camus
, Le Monnier
, Abbe Outhier, Celsius
). (The expedition to Peru is described on the page French Geodesic Mission
and that to Lappland is described on the page Torne Valley
.) The resulting measurements at equatorial and polar latitudes confirmed that the earth was best modelled by an oblate spheroid, supporting Newton.
By the end of the century the French arc had been remeasured and extended from Dunkirk to the Mediterranean. (By Delambre). It was divided into five parts by four intermediate determinations of latitude.
By combining the measurements together with those for the arc of Peru,
ellipsoid shape parameters were determined and the distance between the equator and pole along the Paris Meridian
was calculated as 5130762 toise
(as specified by the standard toise bar in Paris). Defining this distance as exactly 10,000,000 m led to the construction of a new standard metre bar as 0.5130762 toise.
(See Clarke, pp18–22).
, Everest 1830 and Clarke 1866. A comprehensive list of ellipsoids is given under Figure of the Earth.
Geodesy
no longer uses simple meridian arcs, but complex networks with hundreds of fixed points
linked by the methods of satellite geodesy
.
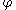on the ellipsoid is an important problem in the theory of map projections, particularly the Transverse Mercator projection
. Ellipsoids are normally specified in terms of the parameters defined above,
, 
, 
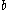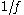, but in theoretical work it is useful to define extra parameters, particularly the eccentricity
,
, and the third flattening
. Only two of these parameters are independent and there are many relations between them:
Geodesy
Geodesy , also named geodetics, a branch of earth sciences, is the scientific discipline that deals with the measurement and representation of the Earth, including its gravitational field, in a three-dimensional time-varying space. Geodesists also study geodynamical phenomena such as crustal...
, a meridian arc measurement is a highly accurate determination of the distance between two points with the same longitude. Two or more such determinations at different locations then specify the shape of the reference ellipsoid
Reference ellipsoid
In geodesy, a reference ellipsoid is a mathematically-defined surface that approximates the geoid, the truer figure of the Earth, or other planetary body....
which best approximates the shape of the geoid
Geoid
The geoid is that equipotential surface which would coincide exactly with the mean ocean surface of the Earth, if the oceans were in equilibrium, at rest , and extended through the continents . According to C.F...
. This process is called the determination of the Figure of the Earth
Figure of the Earth
The expression figure of the Earth has various meanings in geodesy according to the way it is used and the precision with which the Earth's size and shape is to be defined. The actual topographic surface is most apparent with its variety of land forms and water areas. This is, in fact, the surface...
. The earliest determinations of the size of a spherical Earth required a single arc. The latest determinations use astro-geodetic
Astro-geodetic
Astro-geodetic methods are a group of important methods in geodesy, satellite techniques and astrometry.The classical astro-geodetic leveling is a reasonably accurate method to derive the terrestrial geoid, provided a starting point is given...
measurements and the methods of satellite geodesy
Satellite geodesy
Satellite geodesy is the measurement of the form and dimensions of the Earth, the location of objects on its surface and the figure of the Earth's gravity field by means of artificial satellite techniques—geodesy by means of artificial satellites...
to determine the reference ellipsoids.
The Earth as a sphere
Early estimations of Earth's radius are recorded from Egypt in 240 BC, and from Baghdad caliphCaliph
The Caliph is the head of state in a Caliphate, and the title for the ruler of the Islamic Ummah, an Islamic community ruled by the Shari'ah. It is a transcribed version of the Arabic word which means "successor" or "representative"...
s in the 9th century, but it was the Alexandrian scientist Eratosthenes
Eratosthenes
Eratosthenes of Cyrene was a Greek mathematician, poet, athlete, geographer, astronomer, and music theorist.He was the first person to use the word "geography" and invented the discipline of geography as we understand it...
who first calculated the circumference a reasonably good approximate value for the radius. He knew that on the summer solstice
Summer solstice
The summer solstice occurs exactly when the axial tilt of a planet's semi-axis in a given hemisphere is most inclined towards the star that it orbits. Earth's maximum axial tilt to our star, the Sun, during a solstice is 23° 26'. Though the summer solstice is an instant in time, the term is also...
at local noon the sun
Sun
The Sun is the star at the center of the Solar System. It is almost perfectly spherical and consists of hot plasma interwoven with magnetic fields...
goes through the zenith
Zenith
The zenith is an imaginary point directly "above" a particular location, on the imaginary celestial sphere. "Above" means in the vertical direction opposite to the apparent gravitational force at that location. The opposite direction, i.e...
in the ancient Egyptian city of Syene (Assuan). He also knew from his own measurements that, at the same moment in his hometown of Alexandria
Alexandria
Alexandria is the second-largest city of Egypt, with a population of 4.1 million, extending about along the coast of the Mediterranean Sea in the north central part of the country; it is also the largest city lying directly on the Mediterranean coast. It is Egypt's largest seaport, serving...
, the zenith distance was 1/50 of a full circle (7.2°).
Assuming that Alexandria was due north of Syene, Eratosthenes concluded that the distance between Alexandria and Syene must be 1/50 of Earth's circumference. Using data from caravan
Caravan (travellers)
A caravan is a group of people traveling together, often on a trade expedition. Caravans were used mainly in desert areas and throughout the Silk Road, where traveling in groups aided in defence against bandits as well as helped to improve economies of scale in trade.In historical times, caravans...
travels, he estimated the distance to be 5000 stadia
Stadion (unit of length)
The stadion, Latinized as stadium and anglicized as stade, is an ancient Greek unit of length. According to Herodotus, one stade is equal to 600 feet. However, there were several different lengths of “feet”, depending on the country of origin....
(about 500 nautical miles)—which implies a circumference of 252,000 stadia. Assuming the Attic stadion (185 m) this corresponds to 46,620 km, or 16% too great. However, if Eratosthenes used the Egyptian stadion (157.5 m) his measurement turns out to be 39,690 km, an error of only 1%. Considering geometry and ancient conditions, a 16% error is more probable: Syene is not precisely on the Tropic of Cancer
Tropic of Cancer
The Tropic of Cancer, also referred to as the Northern tropic, is the circle of latitude on the Earth that marks the most northerly position at which the Sun may appear directly overhead at its zenith...
and not directly south of Alexandria. The sun appears as a disk of 0.5°, and an estimate of the overland distance traveling along the Nile
Nile
The Nile is a major north-flowing river in North Africa, generally regarded as the longest river in the world. It is long. It runs through the ten countries of Sudan, South Sudan, Burundi, Rwanda, Democratic Republic of the Congo, Tanzania, Kenya, Ethiopia, Uganda and Egypt.The Nile has two major...
or through the desert couldn't be more accurate than about 10%.
Eratosthenes' estimation of Earth’s size was accepted for nearly two thousand years. A similar method was used by Posidonius
Posidonius
Posidonius "of Apameia" or "of Rhodes" , was a Greek Stoic philosopher, politician, astronomer, geographer, historian and teacher native to Apamea, Syria. He was acclaimed as the greatest polymath of his age...
about 150 years later, and slightly better results were calculated in AD 827 by the Gradmessung of the Caliph al-Ma'mun
Al-Ma'mun
Abū Jaʿfar Abdullāh al-Māʾmūn ibn Harūn was an Abbasid caliph who reigned from 813 until his death in 833...
.
The Earth as an ellipsoid
Comment: early literature uses the term oblate spheroid to describe a sphere "squashed at the poles". Modern literature uses the term "ellipsoid of revolution" although the qualifying words "of revolution" are usually dropped. An ellipsoid which is not an ellipsoid of revolution is called a tri-axial ellipsoid. Spheroid and ellipsoid are used interchangeably in this article.The method of determining the ellipsoid
High precision land surveys can be used determine the distance between two places at "almost" the same longitude by measuring a base line and a chain of triangles. (Suitable stations for the end points are rarely at the same longitude). The distance Δ along the meridian from one end point to a point at the same latitude as the second end point is then calculated by trigonometry. The surface distance Δ is reduced to Δ', the corresponding distance at mean sea level. The intermediate distances to points on the meridian at the same latitudes as other stations of the survey may also be calculated.The geographic latitudes of both end points, φs (standpoint) and φf (forepoint) and possibly at other points are determined by astrogeodesy, observing the zenith distances of sufficient numbers of star
Star
A star is a massive, luminous sphere of plasma held together by gravity. At the end of its lifetime, a star can also contain a proportion of degenerate matter. The nearest star to Earth is the Sun, which is the source of most of the energy on Earth...
s. If latitudes are measured at end points only, the radius of curvature at the mid-point of the meridian arc can be calculated from R = Δ'/(|φs-φf|). A second meridian arc will allow the derivation of two parameters required to specify a reference ellipsoid
Reference ellipsoid
In geodesy, a reference ellipsoid is a mathematically-defined surface that approximates the geoid, the truer figure of the Earth, or other planetary body....
. Longer arcs with intermediate latitude determinations can completely determine the ellipsoid. In practice multiple arc measurements are used to determine the ellipsoid parameters by the method of least squares. The parameters determined are usually the semi-major axis,
, and either the semi-minor axis, 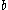, or the inverse flattening 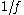, (where the flattening is 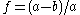).The eighteenth century
In 1687 Newton had published in the Principia a proof that the earth was an oblate spheroid (of inverse flattening equal to 230). This was contended by some, but not all, French scientists. A meridian arc of Picard was extended to a longer arc by Cassini (J.D.)Dominique, comte de Cassini
This article is about the French astronomer. For his Italian-born great-grandfather, see Giovanni Domenico Cassini.Jean-Dominique, comte de Cassini was a French astronomer, son of César-François Cassini de Thury....
over the period 1684–1718. The arc was measured with at least three latitude determinations, so they were able to deduce mean curvatures for the northern and southern halves of the arc, allowing a determination of the overall shape. The results indicated that the Earth was a prolate spheroid (with an equatorial radius less than the polar radius). (The history of the meridian arc from 1600 to 1880 is fully covered in the first chapter of Geodesy by Alexander Ross Clarke.).
To resolve the issue, the French Academy of Sciences
French Academy of Sciences
The French Academy of Sciences is a learned society, founded in 1666 by Louis XIV at the suggestion of Jean-Baptiste Colbert, to encourage and protect the spirit of French scientific research...
(1735) proposed expeditions to Peru (Bouguer
Pierre Bouguer
Pierre Bouguer was a French mathematician, geophysicist, geodesist, and astronomer. He is also known as "the father of naval architecture"....
, Louis Godin
Louis Godin
Louis Godin was a French astronomer and member of the French Academy of Sciences. He worked in Peru, Spain, Portugal and France.-Biography:...
, de La Condamine
Charles Marie de La Condamine
Charles Marie de La Condamine was a French explorer, geographer, and mathematician. He spent ten years in present-day Ecuador measuring the length of a degree latitude at the equator and preparing the first map of the Amazon region based on astronomical observations.-Biography:Charles Marie de La...
, Antonio de Ulloa
Antonio de Ulloa
Antonio de Ulloa y de la Torre-Girault was a Spanish general, explorer, author, astronomer, colonial administrator and the first Spanish governor of Louisiana.Rebellion of 1768]]....
, Jorge Juan
Jorge Juan y Santacilia
Jorge Juan y Santacilia was a Spanish mathematician, scientist, naval officer, and mariner.-Family and Education:...
) and Lappland (Maupertuis
Pierre Louis Maupertuis
Pierre-Louis Moreau de Maupertuis was a French mathematician, philosopher and man of letters. He became the Director of the Académie des Sciences, and the first President of the Berlin Academy of Science, at the invitation of Frederick the Great....
, Clairaut, Camus
Charles Étienne Louis Camus
Charles Étienne Louis Camus , was a French mathematician and mechanician who was born at Crecy-en-Brie, near Meaux....
, Le Monnier
Pierre Charles Le Monnier
Pierre Charles Le Monnier was a French astronomer. His name is sometimes given as Lemonnier.-Biography:...
, Abbe Outhier, Celsius
Anders Celsius
Anders Celsius was a Swedish astronomer. He was professor of astronomy at Uppsala University from 1730 to 1744, but traveled from 1732 to 1735 visiting notable observatories in Germany, Italy and France. He founded the Uppsala Astronomical Observatory in 1741, and in 1742 he proposed the Celsius...
). (The expedition to Peru is described on the page French Geodesic Mission
French Geodesic Mission
The French Geodesic Mission was an 18th-century expedition to what is now Ecuador carried out for the purpose of measuring the roundness of the Earth and measuring the length of a degree of longitude at the Equator...
and that to Lappland is described on the page Torne Valley
Torne Valley
The Torne Valley or Torne River Valley lies at the border of Sweden and Finland. In 2009 it became one of the Sub-regions of Finland. It is named after the Torne River flowing through the valley and into the Gulf of Bothnia...
.) The resulting measurements at equatorial and polar latitudes confirmed that the earth was best modelled by an oblate spheroid, supporting Newton.
By the end of the century the French arc had been remeasured and extended from Dunkirk to the Mediterranean. (By Delambre). It was divided into five parts by four intermediate determinations of latitude.
By combining the measurements together with those for the arc of Peru,
ellipsoid shape parameters were determined and the distance between the equator and pole along the Paris Meridian
Paris Meridian
The Paris Meridian is a meridian line running through the Paris Observatory in Paris, France—now longitude 2°20′14.025″ east. It was a long-standing rival to Greenwich as the prime meridian of the world, as was the Meridian of Antwerp in Antwerp, Belgium....
was calculated as 5130762 toise
Toise
A toise is a unit of measure for length, area and volume originating in pre-revolutionary France. In North America, it was used in colonial French establishments in early New France, French Louisiana , and Quebec...
(as specified by the standard toise bar in Paris). Defining this distance as exactly 10,000,000 m led to the construction of a new standard metre bar as 0.5130762 toise.
(See Clarke, pp18–22).
The nineteenth and twentieth centuries
In the 19th century, many astronomers and geodesists were engaged in detailed studies of the Earth's curvature along different meridian arcs. The analyses resulted in a great many model ellipsoids such as Plessis 1817, Airy 1830, Bessel 1830Bessel ellipsoid
The Bessel ellipsoid is an important reference ellipsoid of geodesy. It is currently used by several countries for their national geodetic surveys, in Europe and on other continents, but will be replaced in the next decades by modern ellipsoids of satellite geodesy.The Bessel ellipsoid was derived...
, Everest 1830 and Clarke 1866. A comprehensive list of ellipsoids is given under Figure of the Earth.
Geodesy
Geodesy
Geodesy , also named geodetics, a branch of earth sciences, is the scientific discipline that deals with the measurement and representation of the Earth, including its gravitational field, in a three-dimensional time-varying space. Geodesists also study geodynamical phenomena such as crustal...
no longer uses simple meridian arcs, but complex networks with hundreds of fixed points
Benchmark (surveying)
The term bench mark, or benchmark, originates from the chiseled horizontal marks that surveyors made in stone structures, into which an angle-iron could be placed to form a "bench" for a leveling rod, thus ensuring that a leveling rod could be accurately repositioned in the same place in the future...
linked by the methods of satellite geodesy
Satellite geodesy
Satellite geodesy is the measurement of the form and dimensions of the Earth, the location of objects on its surface and the figure of the Earth's gravity field by means of artificial satellite techniques—geodesy by means of artificial satellites...
.
Meridian distance on the ellipsoid
The determination of the meridian distance, that is the distance from the equator to a point at a latitude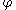 on the ellipsoid is an important problem in the theory of map projections, particularly the Transverse Mercator projectionTransverse Mercator projection
The transverse Mercator map projection is an adaptation of the standard Mercator projection. The transverse version is widely used in national and international mapping systems around the world, including the UTM...
. Ellipsoids are normally specified in terms of the parameters defined above,
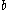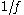, , , but in theoretical work it is useful to define extra parameters, particularly the eccentricityEccentricity (mathematics)
In mathematics, the eccentricity, denoted e or \varepsilon, is a parameter associated with every conic section. It can be thought of as a measure of how much the conic section deviates from being circular.In particular,...
,
, and the third flatteningFlattening
The flattening, ellipticity, or oblateness of an oblate spheroid is a measure of the "squashing" of the spheroid's pole, towards its equator...
. Only two of these parameters are independent and there are many relations between them:
-
The radius of curvature is defined as
so that the arc length of an infinitesimal element of the meridian is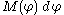
(with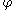 
in radians). Therefore the meridian distance from the equator to latitude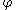
is
-
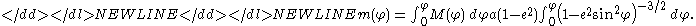
The distance from the equator to the pole, the polar distance, is-
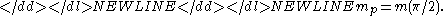
Relation to elliptic integrals
The above integral is related to a special case of an incomplete elliptic integral of the third kind. In the notation of the online NIST handbookhttp://dlmf.nist.gov (Chapter 19.2),-
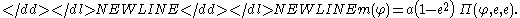
It may also be written in terms of incomplete elliptic integrals of the second kind by a change of integration variable from latitude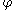
to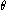 
, the complement of the reduced latitude:
-
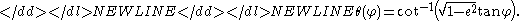
The result is-
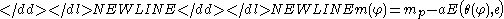
where,-
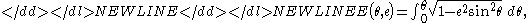
and the polar distance is given by a complete elliptic integral of the second kind.-
The calculation (to arbitrary precision) of the elliptic integrals and approximations are also discussed in the NIST handbook. These functions are also implemented in computer algebra programs such as Mathematica and Maxima.
Series in eccentricity
The above integral may be approximated by a truncated series in the square of the eccentricity (approximately 1/150) by expanding the integrand in a binomial seriesBinomial seriesIn mathematics, the binomial series is the Taylor series at x = 0 of the function f given by f = α, where is an arbitrary complex number...
. Setting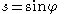
,
-
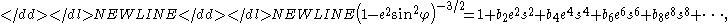
where-
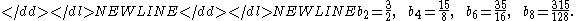
Using simple trigonometric identities the powers of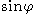
may be reduced to combinations of factors of 
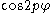. Collecting terms with the same cosine factors and integrating gives the following series, first given by Delambre in 1799.
-
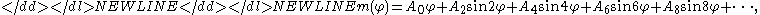
where-
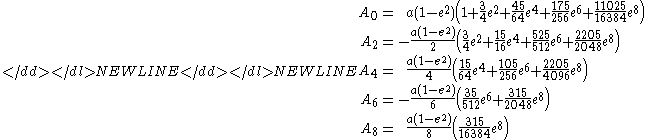
The numerical values for the semi-major axis and eccentricity of the WGS84 ellipsoid
give, in metres,-
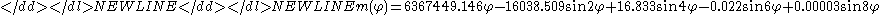
The first four terms have been rounded to the nearest millimetre whilst the eighth order term gives rise to sub-millimetre corrections. Tenth order series are employed in modern "wide zone" implementations of the transverse Mercator projection. (See Stuifbergen).
For the WGS84 ellipsoid the distance from equator to pole is given (in metres) by-
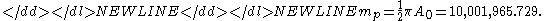
The perimeter of a meridian ellipse is
. Therefore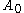 
is the radius of the circle whose circumference is the same as the perimeter of a meridian ellipse. This defines the mean Earth radiusEarth radiusBecause the Earth is not perfectly spherical, no single value serves as its natural radius. Distances from points on the surface to the center range from 6,353 km to 6,384 km...
as 6,367,449.146 m. Dividing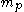
by 90 gives the mean value of the meridian distance per degree of latitude as 111,132 m.
On the ellipsoid the exact distance between parallels at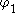
and 
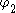is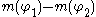 
. For WGS84 an approximate expression for the distance 
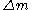between the two parallels at one half of a degree from the circle at latitude 
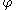is given (in metres) by
-
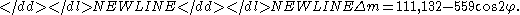
Series in third flattening (n)
The third flattening
is related to the eccentricity by
-
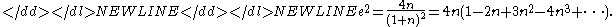
With this substition the integral for the meridian distance becomes
This integral has been expanded in several ways, all of which can be related to the Delambre series.
Bessel
In 1837 BesselFriedrich Bessel-References:* John Frederick William Herschel, A brief notice of the life, researches, and discoveries of Friedrich Wilhelm Bessel, London: Barclay, 1847 -External links:...
expanded this integral in a series of the form:
-
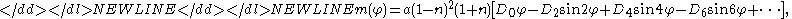
where
-
Since n is approximately one quarter of the value of the squared eccentricity, the above series for the coefficients converge 16 times as fast as the Delambre series.
Helmert
In 1880 HelmertFriedrich Robert HelmertFriedrich Robert Helmert was a German geodesist and an important writer on the theory of errors.Helmert was born in Freiberg, Kingdom of Saxony. After schooling in Freiberg and Dresden, he entered the Polytechnische Schule, now Technische Universität, in Dresden to study engineering science in 1859...
extended and simplified the above series by rewriting
-
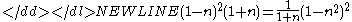
and expanding the numerator terms.
-
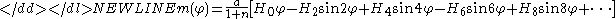
with
-
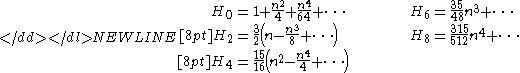
UTM
Despite the simplicity and fast convergence of Helmert's expansion
the U.S. Defense Mapping Agency adopted the fully expanded form of the Bessel series reported by HinksArthur Robert HinksArthur Robert Hinks, CBE, FRS was a British astronomer and geographer..As an astronomer, he is best known for his work in determining the distance from the Sun to the Earth from 1900–1909: for this achievement, he was awarded the Gold Medal of the Royal Astronomical Society and was elected a...
in 1927. This expansion is important, despite the poorer convergence of series in
, because it is used in the definition of UTMUniversal Transverse Mercator coordinate systemThe Universal Transverse Mercator geographic coordinate system uses a 2-dimensional Cartesian coordinate system to give locations on the surface of the Earth. It is a horizontal position representation, i.e...
.
where the coefficients are given to order n5 by
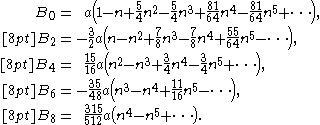
OSGB
A similar fully expanded series of slow convergence was adopted by the Ordnance Survey of Great Britain.
Generalized series
The above series, to eighth order in eccentricity or fourth order in third flattening, are adequate for most practical applications. Each can be written quite generally. For example, Kazushige Kawase (2009) derived following general formula.:
where
-
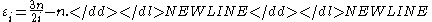
Truncating the summation at j = 2 gives Helmert's approximation.
Approximations
The polar distance may be approximated by the Thomas Muir'sThomas Muir (mathematician)Sir Thomas Muir FRS was a Scottish mathematician, remembered as an authority on determinants. He was born in Stonebyres in South Lanarkshire, and brought up in the small town of Biggar. At the University of Glasgow he changed his studies from classics to mathematics after advice from the future...
formula:
See also
- History of geodesyHistory of geodesyGeodesy ,[1] also named geodetics, is the scientific discipline that deals with the measurement and representation of the Earth.Humanity has always been interested in the Earth...
- Erdmessung, Reference ellipsoidReference ellipsoidIn geodesy, a reference ellipsoid is a mathematically-defined surface that approximates the geoid, the truer figure of the Earth, or other planetary body....
- French Geodesic MissionFrench Geodesic MissionThe French Geodesic Mission was an 18th-century expedition to what is now Ecuador carried out for the purpose of measuring the roundness of the Earth and measuring the length of a degree of longitude at the Equator...
- Struve Geodetic ArcStruve Geodetic ArcThe Struve Geodetic Arc is a chain of survey triangulations stretching from Hammerfest in Norway to the Black Sea, through ten countries and over 2,820 km, which yielded the first accurate measurement of a meridian....
- Torne ValleyTorne ValleyThe Torne Valley or Torne River Valley lies at the border of Sweden and Finland. In 2009 it became one of the Sub-regions of Finland. It is named after the Torne River flowing through the valley and into the Gulf of Bothnia...
- History of geodesy
-
-
-
-
-
-
-
-
-
-
-
-
-
-
-
-
-
-
-
-
-
