Moment distribution method
Encyclopedia
The moment distribution method (not to be confused with moment redistribution
) is a structural analysis
method for statically indeterminate
beam
s and frames developed by Hardy Cross
. It was published in 1930 in an ASCE
journal. The method only accounts for flexural effects and ignores axial and shear effects. From the 1930s until computers began to be widely used in the design and analysis of structures, the moment distribution method was the most widely practiced method.
of the structure to be analysed is fixed so as to develop the fixed-end moments. Then each fixed joint is sequentially released and the fixed-end moments (which by the time of release are not in equilibrium) are distributed to adjacent members until equilibrium
is achieved. The moment distribution method in mathematical terms can be demonstrated as the process of solving a set of simultaneous equations
by means of iteration
.
The moment distribution method falls into the category of displacement method of structural analysis.
(I) divided by the length (L) of the member. What is needed in the moment distribution method is not the exact value but the ratio
of flexural stiffness of all members.
framed at joint 
is given as: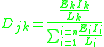
where n is the number of members framed at the joint.
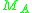while the other end (end B) remains fixed. This will cause end A to rotate through an angle 
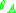. Once the magnitude of 
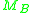developed at end B is found, the carryover factor of this member is given as the ratio of 
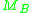over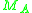 
:
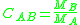
In case of a beam of length L with constant cross-section whose flexural rigidity is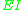
,
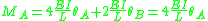
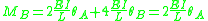
therefore the carryover factor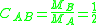
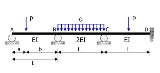
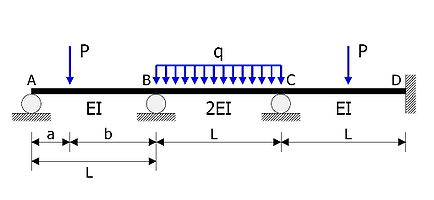The statically indeterminate beam shown in the figure is to be analysed.
In the following calcuations, counterclockwise moments are positive.
, 
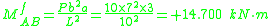
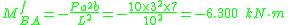
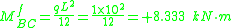
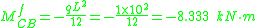
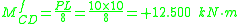
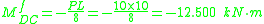
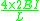and 
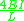, respectively. Therefore:
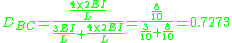
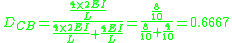
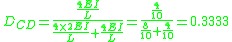
The distribution factors of joints A and D are
and 
.
, except for the carryover factor from D (fixed support) to C which is zero.
Numbers in grey are balanced moments; arrows ( → / ← ) represent the carry-over of moment from one end to the other end of a member.
For comparison purposes, the following are the results generated using a matrix method
. Note that in the analysis above, the iterative process was carried to >0.01 precision. The fact that the matrix analysis results and the moment distribution analysis results match to 0.001 precision is mere coincidence.
The complete shear and bending moment diagrams are as shown. Note that the moment distribution method only determines the moments at the joints. Developing complete bending moment diagrams require additional calculations using the determined joint moments and internal section equilibrium.
Moment redistribution
Moment redistribution refers to the behavior of statically indeterminate structures that are not completely elastic, but have some reserve plastic capacity. When one location first yields, further load to the structure causes bending moment to redistribute differently than would be expected in a...
) is a structural analysis
Structural analysis
Structural analysis is the determination of the effects of loads on physical structures and their components. Structures subject to this type of analysis include all that must withstand loads, such as buildings, bridges, vehicles, machinery, furniture, attire, soil strata, prostheses and...
method for statically indeterminate
Statically indeterminate
In statics, a structure is statically indeterminate when the static equilibrium equations are insufficient for determining the internal forces and reactions on that structure....
beam
Beam (structure)
A beam is a horizontal structural element that is capable of withstanding load primarily by resisting bending. The bending force induced into the material of the beam as a result of the external loads, own weight, span and external reactions to these loads is called a bending moment.- Overview...
s and frames developed by Hardy Cross
Hardy Cross
Hardy Cross, 1885–1959, born in Nansemond County, Virginia, was a U.S. structural engineer and the developer of the moment distribution method for structural calculation of large buildings. The method was in general use from c.1935 until c.1960 when it was gradually superseded by other methods...
. It was published in 1930 in an ASCE
American Society of Civil Engineers
The American Society of Civil Engineers is a professional body founded in 1852 to represent members of the civil engineering profession worldwide. It is the oldest national engineering society in the United States. ASCE's vision is to have engineers positioned as global leaders who strive toward...
journal. The method only accounts for flexural effects and ignores axial and shear effects. From the 1930s until computers began to be widely used in the design and analysis of structures, the moment distribution method was the most widely practiced method.
Introduction
In the moment distribution method, every jointJoint
A joint is the location at which two or more bones make contact. They are constructed to allow movement and provide mechanical support, and are classified structurally and functionally.-Classification:...
of the structure to be analysed is fixed so as to develop the fixed-end moments. Then each fixed joint is sequentially released and the fixed-end moments (which by the time of release are not in equilibrium) are distributed to adjacent members until equilibrium
Mechanical equilibrium
A standard definition of static equilibrium is:This is a strict definition, and often the term "static equilibrium" is used in a more relaxed manner interchangeably with "mechanical equilibrium", as defined next....
is achieved. The moment distribution method in mathematical terms can be demonstrated as the process of solving a set of simultaneous equations
Simultaneous equations
In mathematics, simultaneous equations are a set of equations containing multiple variables. This set is often referred to as a system of equations. A solution to a system of equations is a particular specification of the values of all variables that simultaneously satisfies all of the equations...
by means of iteration
Iteration
Iteration means the act of repeating a process usually with the aim of approaching a desired goal or target or result. Each repetition of the process is also called an "iteration," and the results of one iteration are used as the starting point for the next iteration.-Mathematics:Iteration in...
.
The moment distribution method falls into the category of displacement method of structural analysis.
Implementation
In order to apply the moment distribution method to analyse a structure, the following things must be considered.Fixed end moments
Fixed end moments are the moments produced at member ends by external loads when the joints are fixed.Flexural stiffness
The flexural stiffness (EI/L) of a member is represented as the product of the modulus of elasticity (E) and the second moment of areaSecond moment of area
The second moment of area, also known as the area moment of inertia, moment of inertia of plane area, or second moment of inertia is a property of a cross section that can be used to predict the resistance of beams to bending and deflection, around an axis that lies in the cross-sectional plane...
(I) divided by the length (L) of the member. What is needed in the moment distribution method is not the exact value but the ratio
Ratio
In mathematics, a ratio is a relationship between two numbers of the same kind , usually expressed as "a to b" or a:b, sometimes expressed arithmetically as a dimensionless quotient of the two which explicitly indicates how many times the first number contains the second In mathematics, a ratio is...
of flexural stiffness of all members.
Distribution factors
When a joint is released and begins to rotate under the unbalanced moment, resisting forces develop at each member framed together at the joint. Although the total resistance is equal to the unbalanced moment, the magnitudes of resisting forces developed at each member differ by the members' flexural stiffness. Distribution factors can be defined as the proportions of the unbalanced moments carried by each of the members. In mathematical terms, distribution factor of member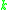 framed at joint 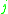is given as: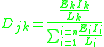where n is the number of members framed at the joint.
Carryover factors
When a joint is released, balancing moment occurs to counterbalance the unbalanced moment which is initially the same as the fixed-end moment. This balancing moment is then carried over to the member's other end. The ratio of the carried-over moment at the other end to the fixed-end moment of the initial end is the carryover factor.Determination of carryover factors
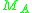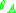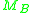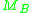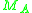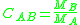
Let one end (end A) of a fixed beam be released and applied a moment while the other end (end B) remains fixed. This will cause end A to rotate through an angle . Once the magnitude of developed at end B is found, the carryover factor of this member is given as the ratio of over :In case of a beam of length L with constant cross-section whose flexural rigidity is
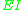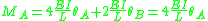
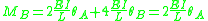
,therefore the carryover factor
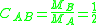
Sign convention
Once a sign convention has been chosen, it has to be maintained for the whole structure. The traditional engineer's sign convention is not used in the calculations of the moment distiribution method although the results can be expressed in the conventional way.Framed structures
Framed structures with or without sidesway can be analysed using the moment distribution method.Example
- Members AB, BC, CD have the same length
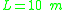. - Flexural rigidities are EI, 2EI, EI respectively.
- Concentrated load of magnitude
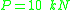acts at a distance 
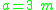from the support A. - Uniform load of intensity
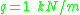acts on BC. - Member CD is loaded at its midspan with a concentrated load of magnitude
.
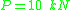In the following calcuations, counterclockwise moments are positive.
Fixed end moments
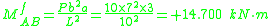
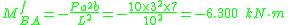
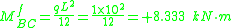
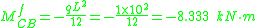
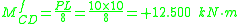
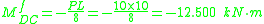
Flexural stiffness and distribution factors
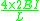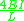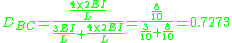
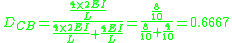
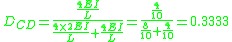
The flexural stiffness of members AB, BC and CD are, and , respectively. Therefore:The distribution factors of joints A and D are
and .Carryover factors
The carryover factors are, except for the carryover factor from D (fixed support) to C which is zero.Moment distribution
 |
|||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| Joint | A | Joint | B | Joint | C | Joint | D | ||||
| Distrib. factors | 0 | 1 | 0.2727 | 0.7273 | 0.6667 | 0.3333 | 0 | 0 | |||
| Fixed-end moments | 14.700 | -6.300 | 8.333 | -8.333 | 12.500 | -12.500 | |||||
| Step 1 | -14.700 | → | -7.350 | ||||||||
| Step 2 | 1.450 | 3.867 | → | 1.934 | |||||||
| Step 3 | -2.034 | ← | -4.067 | -2.034 | → | -1.017 | |||||
| Step 4 | 0.555 | 1.479 | → | 0.739 | |||||||
| Step 5 | -0.246 | ← | -0.493 | -0.246 | → | -0.123 | |||||
| Step 6 | 0.067 | 0.179 | → | 0.090 | |||||||
| Step 7 | -0.030 | ← | -0.060 | -0.030 | → | -0.015 | |||||
| Step 8 | 0.008 | 0.022 | → | 0.011 | |||||||
| Step 9 | -0.004 | ← | -0.007 | -0.004 | → | -0.002 | |||||
| Step 10 | 0.001 | 0.003 | |||||||||
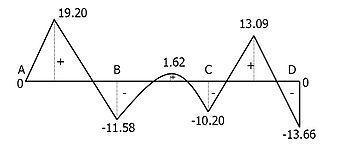
| Sum of moments | 0 | -11.569 | 11.569 | -10.186 | 10.186 | -13.657 | |||||
Numbers in grey are balanced moments; arrows ( → / ← ) represent the carry-over of moment from one end to the other end of a member.
- Step 1
- As joint A is released, balancing moment of magnitude equal to the fixed end moment
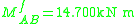develops and is carried-over from joint A to joint B.
- Step 2
- The unbalanced moment at joint B now is the summation of the fixed end moments
, 
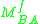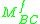and the carry-over moment from joint A. This unbalanced moment is distributed to members BA and BC in accordance with the distribution factors 
and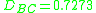 
. Step 2 ends with carry-over of balanced moment 
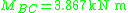to joint C. Joint A is a roller support which has no rotational restraint, so moment carryover from joint B to joint A is zero.
- Step 3
- The unbalanced moment at joint C now is the summation of the fixed end moments
, 
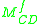and the carryover moment from joint B. As in the previous step, this unbalanced moment is distributed to each member and then carried over to joint C and back to joint B. Joint D is a fixed support and carried-over moments to this joint will not be distributed nor be carried over to joint C.
- Step 4
- Joint B still has unbalanced moment which was carried over from joint C in step 3. Joint B is released once again to induce moment distribution and to achieve equilibrium.
- Steps 5 - 10
- Joints are released and fixed again until every joint has unbalanced moments of size zero or neglectably small in required precision. Arithmetically summing all moments in each respective columns gives the final moment values.
Result
- Moments at joints determined by the moment distribution method
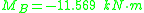
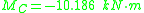
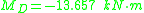
- The conventional engineer's sign convention is used here, i.e. positive moments cause elongation at the bottom part of a beam member.
For comparison purposes, the following are the results generated using a matrix method
Matrix method
The matrix method is a structural analysis method used as a fundamental principle in many applications in civil engineering.The method is carried out, using either a stiffness matrix or a flexibility matrix...
. Note that in the analysis above, the iterative process was carried to >0.01 precision. The fact that the matrix analysis results and the moment distribution analysis results match to 0.001 precision is mere coincidence.
- Moments at joints determined by the matrix method
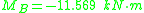
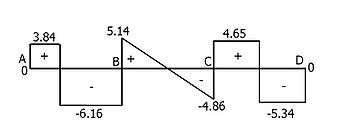
The complete shear and bending moment diagrams are as shown. Note that the moment distribution method only determines the moments at the joints. Developing complete bending moment diagrams require additional calculations using the determined joint moments and internal section equilibrium.
- SFD and BMD
See also
- Finite element methodFinite element methodThe finite element method is a numerical technique for finding approximate solutions of partial differential equations as well as integral equations...
- Slope deflection methodSlope deflection methodThe slope deflection method is a structural analysis method for beams and frames introduced in 1915 by George A. Maney. The slope deflection method was widely used for more than a decade until the moment distribution method was developed.- Introduction :...
- Free Moment Distribution Program in Visual Basic