Observable
Encyclopedia
In physics
, particularly in quantum physics, a system observable is a property of the system state that can be determined by some sequence of physical operations
. For example, these operations might involve submitting the system to various electromagnetic field
s and eventually reading a value off some gauge. In systems governed by classical mechanics
, any experiment
ally observable value can be shown to be given by a real
-valued function
on the set of all possible system states.
Physically meaningful observables must also satisfy transformation laws which relate observations performed by different observer
s in different frames of reference
. These transformation laws are automorphism
s of the state space, that is bijective transformation
s which preserve some mathematical property.
for its description. In the mathematical formulation of quantum mechanics
, states are given by non-zero vectors in a Hilbert space
V (where two vectors are considered to specify the same state if, and only if, they are scalar multiples of each other) and observables are given by self-adjoint operator
s on V. However, as indicated below, not every self-adjoint operator corresponds to a physically meaningful observable. For the case of a system of particle
s, the space V consists of functions called wave functions or state vector
s.
In the case of transformation laws in quantum mechanics, the requisite automorphisms are unitary
(or antiunitary) linear transformations of the Hilbert space
V. Under Galilean relativity or special relativity
, the mathematics of frames of reference is particularly simple, and in fact restricts considerably the set of physically meaningful observables.
In quantum mechanics, measurement of observables exhibits some seemingly unintuitive properties. Specifically, if a system is in a state described by a vector in a Hilbert space
, the measurement process affects the state in a non-deterministic, but statistically predictable way. In particular, after a measurement is applied, the state description by a single vector may be destroyed, being replaced by a statistical ensemble. The irreversible nature of measurement operations in quantum physics is sometimes referred to as the measurement problem
and is described mathematically by quantum operation
s. By the structure of quantum operations, this description is mathematically equivalent to that offered by relative state interpretation where the original system is regarded as a subsystem of a larger system and the state of the original system is given by the partial trace
of the state of the larger system.
In quantum mechanics each dynamical variable (e.g. position, translational momentum, orbital angular momentum, spin, total angular momentum, energy, etc.) is associated with a Hermitian operator that acts on the state of the quantum system and whose eigenvalues correspond to the possible values of the dynamical variable. For example, suppose
is an eigenket (eigenvector) of the observable 
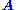, with eigenvalue 
, and exists in a d-dimensional Hilbert space
. Then
= 
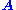
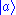This eigenket equation says that if a measurement
of the observable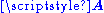
is made while the system of interest is in the state 
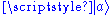, then the observed value of that particular measurement must return the eigenvalue 
with certainty. However, if the system of interest is in the general state 
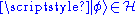, then the eigenvalue 
is returned with probability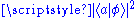 
(Born rule
). One must note that the above definition is somewhat dependent upon our convention of choosing real numbers to represent real physical quantities. Indeed, just because dynamical variables are "real" and not "unreal" in the metaphysical sense does not mean that they must correspond to real numbers in the mathematical sense.
To be more precise, the dynamical variable/observable is a (not necessarily bounded) Hermitian operator in a Hilbert Space and thus is represented by a Hermitian matrix if the space is finite-dimensional. In an infinite-dimensional Hilbert space, the observable is represented by a symmetric operator, which may not be defined everywhere (i.e. its domain
is not the whole space - there exist some states that are not in the domain of the operator). The reason for such a change is that in an infinite-dimensional Hilbert space, the operator becomes unbounded, which means that it no longer has a largest eigenvalue. This is not the case in a finite-dimensional Hilbert space, where every operator is bounded - it has a largest eigenvalue. For example, if we consider the position of a point particle moving along a line, this particle's position variable can take on any number on the real-line, which is uncountably infinite. Since the eigenvalue of an observable represents a real physical quantity for that particular dynamical variable, then we must conclude that there is no largest eigenvalue for the position observable in this uncountably infinite-dimensional Hilbert space, since the field
we're working over consists of the real-line. Nonetheless, whether we are working in an infinite-dimensional or finite-dimensional Hilbert space, the role of an observable in quantum mechanics is to assign real numbers to outcomes of particular measurements; this means that only certain measurements can determine the value of an observable for some state of a quantum system. In classical mechanics, any measurement can be made to determine the value of an observable.
of the corresponding operators, to the effect that
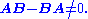
This inequality expresses a dependence of measurement results on the order in which measurements of observables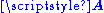
and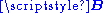 
are performed. Observables corresponding to non-commutative operators are called incompatible.
Physics
Physics is a natural science that involves the study of matter and its motion through spacetime, along with related concepts such as energy and force. More broadly, it is the general analysis of nature, conducted in order to understand how the universe behaves.Physics is one of the oldest academic...
, particularly in quantum physics, a system observable is a property of the system state that can be determined by some sequence of physical operations
Operational definition
An operational definition defines something in terms of the specific process or set of validation tests used to determine its presence and quantity. That is, one defines something in terms of the operations that count as measuring it. The term was coined by Percy Williams Bridgman and is a part of...
. For example, these operations might involve submitting the system to various electromagnetic field
Electromagnetic field
An electromagnetic field is a physical field produced by moving electrically charged objects. It affects the behavior of charged objects in the vicinity of the field. The electromagnetic field extends indefinitely throughout space and describes the electromagnetic interaction...
s and eventually reading a value off some gauge. In systems governed by classical mechanics
Classical mechanics
In physics, classical mechanics is one of the two major sub-fields of mechanics, which is concerned with the set of physical laws describing the motion of bodies under the action of a system of forces...
, any experiment
Experiment
An experiment is a methodical procedure carried out with the goal of verifying, falsifying, or establishing the validity of a hypothesis. Experiments vary greatly in their goal and scale, but always rely on repeatable procedure and logical analysis of the results...
ally observable value can be shown to be given by a real
Real number
In mathematics, a real number is a value that represents a quantity along a continuum, such as -5 , 4/3 , 8.6 , √2 and π...
-valued function
Function (mathematics)
In mathematics, a function associates one quantity, the argument of the function, also known as the input, with another quantity, the value of the function, also known as the output. A function assigns exactly one output to each input. The argument and the value may be real numbers, but they can...
on the set of all possible system states.
Physically meaningful observables must also satisfy transformation laws which relate observations performed by different observer
Observation
Observation is either an activity of a living being, such as a human, consisting of receiving knowledge of the outside world through the senses, or the recording of data using scientific instruments. The term may also refer to any data collected during this activity...
s in different frames of reference
Frames of Reference
Frames of Reference is a 1960 educational film by Physical Sciences Study Committee.The film was made to be shown in high school physics courses. In the film University of Toronto physics professors Patterson Hume and Donald Ivey explain the distinction between inertial and nonintertial frames of...
. These transformation laws are automorphism
Automorphism
In mathematics, an automorphism is an isomorphism from a mathematical object to itself. It is, in some sense, a symmetry of the object, and a way of mapping the object to itself while preserving all of its structure. The set of all automorphisms of an object forms a group, called the automorphism...
s of the state space, that is bijective transformation
Transformation (mathematics)
In mathematics, a transformation could be any function mapping a set X on to another set or on to itself. However, often the set X has some additional algebraic or geometric structure and the term "transformation" refers to a function from X to itself that preserves this structure.Examples include...
s which preserve some mathematical property.
Quantum mechanics
In quantum physics, the relation between system state and the value of an observable requires some basic linear algebraLinear algebra
Linear algebra is a branch of mathematics that studies vector spaces, also called linear spaces, along with linear functions that input one vector and output another. Such functions are called linear maps and can be represented by matrices if a basis is given. Thus matrix theory is often...
for its description. In the mathematical formulation of quantum mechanics
Mathematical formulation of quantum mechanics
The mathematical formulations of quantum mechanics are those mathematical formalisms that permit a rigorous description of quantum mechanics. Such are distinguished from mathematical formalisms for theories developed prior to the early 1900s by the use of abstract mathematical structures, such as...
, states are given by non-zero vectors in a Hilbert space
Hilbert space
The mathematical concept of a Hilbert space, named after David Hilbert, generalizes the notion of Euclidean space. It extends the methods of vector algebra and calculus from the two-dimensional Euclidean plane and three-dimensional space to spaces with any finite or infinite number of dimensions...
V (where two vectors are considered to specify the same state if, and only if, they are scalar multiples of each other) and observables are given by self-adjoint operator
Self-adjoint operator
In mathematics, on a finite-dimensional inner product space, a self-adjoint operator is an operator that is its own adjoint, or, equivalently, one whose matrix is Hermitian, where a Hermitian matrix is one which is equal to its own conjugate transpose...
s on V. However, as indicated below, not every self-adjoint operator corresponds to a physically meaningful observable. For the case of a system of particle
Elementary particle
In particle physics, an elementary particle or fundamental particle is a particle not known to have substructure; that is, it is not known to be made up of smaller particles. If an elementary particle truly has no substructure, then it is one of the basic building blocks of the universe from which...
s, the space V consists of functions called wave functions or state vector
State vector
*A state vector in general control systems describes the observed states of an object in state space, e.g. in variables of the degrees of freedom for motion *A state vector in general control systems describes the observed states of an object in state space, e.g. in variables of the degrees of...
s.
In the case of transformation laws in quantum mechanics, the requisite automorphisms are unitary
Unitary operator
In functional analysis, a branch of mathematics, a unitary operator is a bounded linear operator U : H → H on a Hilbert space H satisfyingU^*U=UU^*=I...
(or antiunitary) linear transformations of the Hilbert space
Hilbert space
The mathematical concept of a Hilbert space, named after David Hilbert, generalizes the notion of Euclidean space. It extends the methods of vector algebra and calculus from the two-dimensional Euclidean plane and three-dimensional space to spaces with any finite or infinite number of dimensions...
V. Under Galilean relativity or special relativity
Special relativity
Special relativity is the physical theory of measurement in an inertial frame of reference proposed in 1905 by Albert Einstein in the paper "On the Electrodynamics of Moving Bodies".It generalizes Galileo's...
, the mathematics of frames of reference is particularly simple, and in fact restricts considerably the set of physically meaningful observables.
In quantum mechanics, measurement of observables exhibits some seemingly unintuitive properties. Specifically, if a system is in a state described by a vector in a Hilbert space
Hilbert space
The mathematical concept of a Hilbert space, named after David Hilbert, generalizes the notion of Euclidean space. It extends the methods of vector algebra and calculus from the two-dimensional Euclidean plane and three-dimensional space to spaces with any finite or infinite number of dimensions...
, the measurement process affects the state in a non-deterministic, but statistically predictable way. In particular, after a measurement is applied, the state description by a single vector may be destroyed, being replaced by a statistical ensemble. The irreversible nature of measurement operations in quantum physics is sometimes referred to as the measurement problem
Measurement problem
The measurement problem in quantum mechanics is the unresolved problem of how wavefunction collapse occurs. The inability to observe this process directly has given rise to different interpretations of quantum mechanics, and poses a key set of questions that each interpretation must answer...
and is described mathematically by quantum operation
Quantum operation
In quantum mechanics, a quantum operation is a mathematical formalism used to describe a broad class of transformations that a quantum mechanical system can undergo. This was first discussed as a general stochastic transformation for a density matrix by George Sudarshan...
s. By the structure of quantum operations, this description is mathematically equivalent to that offered by relative state interpretation where the original system is regarded as a subsystem of a larger system and the state of the original system is given by the partial trace
Partial trace
In linear algebra and functional analysis, the partial trace is a generalization of the trace. Whereas the trace is a scalar valued function on operators, the partial trace is an operator-valued function...
of the state of the larger system.
In quantum mechanics each dynamical variable (e.g. position, translational momentum, orbital angular momentum, spin, total angular momentum, energy, etc.) is associated with a Hermitian operator that acts on the state of the quantum system and whose eigenvalues correspond to the possible values of the dynamical variable. For example, suppose
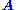is an eigenket (eigenvector) of the observable , with eigenvalue , and exists in a d-dimensional Hilbert spaceHilbert space
The mathematical concept of a Hilbert space, named after David Hilbert, generalizes the notion of Euclidean space. It extends the methods of vector algebra and calculus from the two-dimensional Euclidean plane and three-dimensional space to spaces with any finite or infinite number of dimensions...
. Then
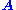
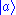= This eigenket equation says that if a measurement
Measurement
Measurement is the process or the result of determining the ratio of a physical quantity, such as a length, time, temperature etc., to a unit of measurement, such as the metre, second or degree Celsius...
of the observable
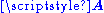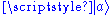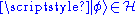is made while the system of interest is in the state , then the observed value of that particular measurement must return the eigenvalue with certainty. However, if the system of interest is in the general state , then the eigenvalue is returned with probability 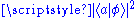(Born ruleBorn rule
The Born rule is a law of quantum mechanics which gives the probability that a measurement on a quantum system will yield a given result. It is named after its originator, the physicist Max Born. The Born rule is one of the key principles of quantum mechanics...
). One must note that the above definition is somewhat dependent upon our convention of choosing real numbers to represent real physical quantities. Indeed, just because dynamical variables are "real" and not "unreal" in the metaphysical sense does not mean that they must correspond to real numbers in the mathematical sense.
To be more precise, the dynamical variable/observable is a (not necessarily bounded) Hermitian operator in a Hilbert Space and thus is represented by a Hermitian matrix if the space is finite-dimensional. In an infinite-dimensional Hilbert space, the observable is represented by a symmetric operator, which may not be defined everywhere (i.e. its domain
Domain (mathematics)
In mathematics, the domain of definition or simply the domain of a function is the set of "input" or argument values for which the function is defined...
is not the whole space - there exist some states that are not in the domain of the operator). The reason for such a change is that in an infinite-dimensional Hilbert space, the operator becomes unbounded, which means that it no longer has a largest eigenvalue. This is not the case in a finite-dimensional Hilbert space, where every operator is bounded - it has a largest eigenvalue. For example, if we consider the position of a point particle moving along a line, this particle's position variable can take on any number on the real-line, which is uncountably infinite. Since the eigenvalue of an observable represents a real physical quantity for that particular dynamical variable, then we must conclude that there is no largest eigenvalue for the position observable in this uncountably infinite-dimensional Hilbert space, since the field
Field (mathematics)
In abstract algebra, a field is a commutative ring whose nonzero elements form a group under multiplication. As such it is an algebraic structure with notions of addition, subtraction, multiplication, and division, satisfying certain axioms...
we're working over consists of the real-line. Nonetheless, whether we are working in an infinite-dimensional or finite-dimensional Hilbert space, the role of an observable in quantum mechanics is to assign real numbers to outcomes of particular measurements; this means that only certain measurements can determine the value of an observable for some state of a quantum system. In classical mechanics, any measurement can be made to determine the value of an observable.
Incompatibility of observables in quantum mechanics
A crucial difference between classical quantities and quantum mechanical observables is that the latter may not be simultaneously measurable. This is mathematically expressed by non-commutativityCommutativity
In mathematics an operation is commutative if changing the order of the operands does not change the end result. It is a fundamental property of many binary operations, and many mathematical proofs depend on it...
of the corresponding operators, to the effect that
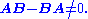
This inequality expresses a dependence of measurement results on the order in which measurements of observables
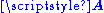and 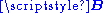are performed. Observables corresponding to non-commutative operators are called incompatible.Further reading
- S. Auyang, How is Quantum Field Theory Possible, Oxford University Press, 1995.
- G. Mackey, Mathematical Foundations of Quantum Mechanics, W. A. Benjamin, 1963.
- V. Varadarajan, The Geometry of Quantum Mechanics vols 1 and 2, Springer-Verlag 1985.
- Leslie E. Ballentine, "Quantum Mechanics: A Modern Development", World Scientific, 1998
- R. Blume-Kohout, "Lecture 14:
and Hilbert space. Wavefunctions, unbounded operators, and rigged Hilbert space.", www.am473.ca, 10/26/08