Born rule
Encyclopedia
The Born rule is a law
of quantum mechanics
which gives the probability
that a measurement
on a quantum system will yield a given result. It is named after its originator, the physicist Max Born
. The Born rule is one of the key principles of quantum mechanics. There have been many attempts to derive
the Born rule from the other assumptions of quantum mechanics
, with inconclusive results.
corresponding to a Hermitian operator
with discrete spectrum
is measured in a system with normalized wave function
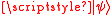
(see Bra-ket notation
), then
In the case where the spectrum of
is not wholly discrete, the spectral theorem
proves the existence of a certain projection-valued measure
, the spectral measure of 
. In this case,
If we are given a wave function
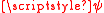
for a single structureless particle in position space,
this reduces to saying that the probability density function
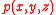
for a measurement of the position at time
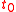
will be given by
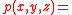
In this paper, Born solves the Schrödinger equation
for a scattering problem and, inspired by Einstein's work on the photoelectric effect, concluded, in a footnote, that the Born rule gives the only possible interpretation of the solution. In 1954, together with Walter Bothe, Born was awarded the Nobel Prize in Physics for this and other work. John von Neumann
discussed the application of spectral theory
to Born's rule in his 1932 book. A 2011 article by Armando V.D.B. Assis claims to show that Born's Rule can be derived within a game-theoretical framework.:
Physical law
A physical law or scientific law is "a theoretical principle deduced from particular facts, applicable to a defined group or class of phenomena, and expressible by the statement that a particular phenomenon always occurs if certain conditions be present." Physical laws are typically conclusions...
of quantum mechanics
Quantum mechanics
Quantum mechanics, also known as quantum physics or quantum theory, is a branch of physics providing a mathematical description of much of the dual particle-like and wave-like behavior and interactions of energy and matter. It departs from classical mechanics primarily at the atomic and subatomic...
which gives the probability
Probability
Probability is ordinarily used to describe an attitude of mind towards some proposition of whose truth we arenot certain. The proposition of interest is usually of the form "Will a specific event occur?" The attitude of mind is of the form "How certain are we that the event will occur?" The...
that a measurement
Measurement
Measurement is the process or the result of determining the ratio of a physical quantity, such as a length, time, temperature etc., to a unit of measurement, such as the metre, second or degree Celsius...
on a quantum system will yield a given result. It is named after its originator, the physicist Max Born
Max Born
Max Born was a German-born physicist and mathematician who was instrumental in the development of quantum mechanics. He also made contributions to solid-state physics and optics and supervised the work of a number of notable physicists in the 1920s and 30s...
. The Born rule is one of the key principles of quantum mechanics. There have been many attempts to derive
Formal proof
A formal proof or derivation is a finite sequence of sentences each of which is an axiom or follows from the preceding sentences in the sequence by a rule of inference. The last sentence in the sequence is a theorem of a formal system...
the Born rule from the other assumptions of quantum mechanics
Quantum mechanics
Quantum mechanics, also known as quantum physics or quantum theory, is a branch of physics providing a mathematical description of much of the dual particle-like and wave-like behavior and interactions of energy and matter. It departs from classical mechanics primarily at the atomic and subatomic...
, with inconclusive results.
The rule
The Born rule states that if an observableObservable
In physics, particularly in quantum physics, a system observable is a property of the system state that can be determined by some sequence of physical operations. For example, these operations might involve submitting the system to various electromagnetic fields and eventually reading a value off...
corresponding to a Hermitian operator
with discrete spectrum
Spectrum (functional analysis)
In functional analysis, the concept of the spectrum of a bounded operator is a generalisation of the concept of eigenvalues for matrices. Specifically, a complex number λ is said to be in the spectrum of a bounded linear operator T if λI − T is not invertible, where I is the...
is measured in a system with normalized wave function
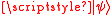
(see Bra-ket notation
Bra-ket notation
Bra-ket notation is a standard notation for describing quantum states in the theory of quantum mechanics composed of angle brackets and vertical bars. It can also be used to denote abstract vectors and linear functionals in mathematics...
), then
- the measured result will be one of the eigenvaluesEigenvalue, eigenvector and eigenspaceThe eigenvectors of a square matrix are the non-zero vectors that, after being multiplied by the matrix, remain parallel to the original vector. For each eigenvector, the corresponding eigenvalue is the factor by which the eigenvector is scaled when multiplied by the matrix...
of 
, and - the probability of measuring a given eigenvalue
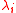will equal 
, where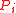 
is the projection onto the eigenspace of 
corresponding to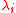 
.. Equivalently, the probability can be written as 
.)
In the case where the spectrum of
is not wholly discrete, the spectral theoremSpectral theorem
In mathematics, particularly linear algebra and functional analysis, the spectral theorem is any of a number of results about linear operators or about matrices. In broad terms the spectral theorem provides conditions under which an operator or a matrix can be diagonalized...
proves the existence of a certain projection-valued measure
Projection-valued measure
In mathematics, particularly functional analysis a projection-valued measure is a function defined on certain subsets of a fixed set and whose values are self-adjoint projections on a Hilbert space...
, the spectral measure of . In this case,
- the probability that the result of the measurement lies in a measurable set
will be given by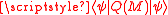 
.
If we are given a wave function
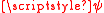
for a single structureless particle in position space,
this reduces to saying that the probability density function
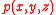
for a measurement of the position at time
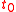
will be given by
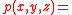
History
The Born rule was formulated by Born in a 1926 paper.In this paper, Born solves the Schrödinger equation
Schrödinger equation
The Schrödinger equation was formulated in 1926 by Austrian physicist Erwin Schrödinger. Used in physics , it is an equation that describes how the quantum state of a physical system changes in time....
for a scattering problem and, inspired by Einstein's work on the photoelectric effect, concluded, in a footnote, that the Born rule gives the only possible interpretation of the solution. In 1954, together with Walter Bothe, Born was awarded the Nobel Prize in Physics for this and other work. John von Neumann
John von Neumann
John von Neumann was a Hungarian-American mathematician and polymath who made major contributions to a vast number of fields, including set theory, functional analysis, quantum mechanics, ergodic theory, geometry, fluid dynamics, economics and game theory, computer science, numerical analysis,...
discussed the application of spectral theory
Spectral theory
In mathematics, spectral theory is an inclusive term for theories extending the eigenvector and eigenvalue theory of a single square matrix to a much broader theory of the structure of operators in a variety of mathematical spaces. It is a result of studies of linear algebra and the solutions of...
to Born's rule in his 1932 book. A 2011 article by Armando V.D.B. Assis claims to show that Born's Rule can be derived within a game-theoretical framework.:
External links
- Quantum Mechanics Not in Jeopardy: Physicists Confirm a Decades-Old Key Principle Experimentally ScienceDaily (July 23, 2010)