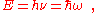Schrödinger equation
Encyclopedia
The Schrödinger equation was formulated in 1926 by Austria
n physicist
Erwin Schrödinger
. Used in physics
(specifically quantum mechanics
), it is an equation that describes how the quantum state of a physical system
changes in time.
In the standard interpretation of quantum mechanics
, the quantum state, also called a wavefunction
or state vector, is the most complete description that can be given to a physical system. Solutions to Schrödinger's equation describe not only molecular
, atom
ic, and subatomic
systems, but also macroscopic systems, possibly even the whole universe
.
The most general form is the time-dependent Schrödinger equation, which gives a description of a system evolving with time. For systems in a stationary state
(i.e., where the Hamiltonian
is not explicitly dependent on time), the time-independent Schrödinger equation is sufficient. Approximate solutions to the time-independent Schrödinger equation are commonly used to calculate the energy level
s and other properties of atoms and molecules.
Schrödinger's equation can be mathematically transformed into Werner Heisenberg
's matrix mechanics
, and into Richard Feynman
's path integral formulation
. The Schrödinger equation describes time in a way that is inconvenient for relativistic theories, a problem that is not as severe in matrix mechanics and completely absent in the path integral formulation.
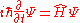
where
V in position space, the Schrödinger equation takes the form:
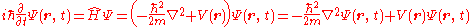
where
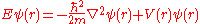
This equation describes the standing wave
solutions of the time-dependent equation, which are the states with definite energy (instead of a probability distribution of different energies). In physics, these standing waves are called "stationary state
s" or "energy eigenstates"; in chemistry they are called "atomic orbital
s" or "molecular orbital
s".
's quantization of light (see black body radiation), Albert Einstein
interpreted Planck's quanta
to be photon
s, particles of light, and proposed that the energy of a photon is proportional to its frequency, one of the first signs of wave–particle duality
. Since energy
and momentum
are related in the same way as frequency
and wavenumber
in special relativity
, it followed that the momentum p of a photon is proportional to its wavenumber k.

Louis de Broglie hypothesized that this is true for all particles, even particles such as electrons. He showed that, assuming that the matter waves propagate along with their particle counterparts, electrons form standing wave
s, meaning that only certain discrete rotational frequencies about the nucleus of an atom are allowed. These quantized orbits correspond to discrete energy level
s, which reproduced the old quantum condition
.
Following up on these ideas, Schrödinger decided to find a proper wave equation for the electron. He was guided by William R. Hamilton
's analogy between mechanics
and optics
, encoded in the observation that the zero-wavelength limit of optics resembles a mechanical system — the trajectories of light rays become sharp tracks that obey Fermat's principle
, an analog of the principle of least action
. A modern version of his reasoning is reproduced in the next section. The equation he found is:

Using this equation, Schrödinger computed the hydrogen spectral series
by treating a hydrogen atom
's electron
as a wave Ψ(x, t), moving in a potential well
V, created by the proton
. This computation accurately reproduced the energy levels of the Bohr model
.
However, by that time, Arnold Sommerfeld
had refined the Bohr model with relativistic corrections
. Schrödinger used the relativistic energy momentum relation to find what is now known as the Klein–Gordon equation in a Coulomb potential (in natural units
):

He found the standing waves of this relativistic equation, but the relativistic corrections disagreed with Sommerfeld's formula. Discouraged, he put away his calculations and secluded himself in an isolated mountain cabin with a lover.
While at the cabin, Schrödinger decided that his earlier non-relativistic calculations were novel enough to publish, and decided to leave off the problem of relativistic corrections for the future. He put together his wave equation and the spectral analysis of hydrogen in a paper in 1926. The paper was enthusiastically endorsed by Einstein, who saw the matter-waves as an intuitive depiction of nature, as opposed to Heisenberg's matrix mechanics
, which he considered overly formal.
The Schrödinger equation details the behaviour of ψ but says nothing of its nature. Schrödinger tried to interpret it as a charge density in his fourth paper, but he was unsuccessful. In 1926, just a few days after Schrödinger's fourth and final paper was published, Max Born
successfully interpreted ψ as a quantity related to the probability amplitude
, which is equal to the squared magnitude of ψ. Schrödinger, though, always opposed a statistical or probabilistic approach, with its associated discontinuities
—much like Einstein, who believed that quantum mechanics was a statistical approximation to an underlying deterministic theory
— and never reconciled with the Copenhagen interpretation
.
The three assumptions above allow one to derive the equation for plane wave
s only. To conclude that it is true in general requires the superposition principle
, and thus, one must separately postulate that the Schrödinger equation is linear
.
of a plane wave
as a complex
phase factor
:
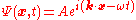
and to realize that since

then
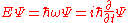
and similarly since
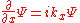
and
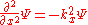
we find:
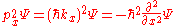
so that, again for a plane wave, he obtained:

And, by inserting these expressions for the energy and momentum into the classical formula we started with, we get Schrödinger's famed equation, for a single particle in the 3-dimensional case in the presence of a potential V:

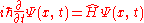
where is a linear operator acting on the wavefunction Ψ. For the specific case of a single particle in one dimension moving under the influence of a potential V.
is a linear operator acting on the wavefunction Ψ. For the specific case of a single particle in one dimension moving under the influence of a potential V.
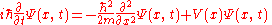
and the operator can be read off:
can be read off:
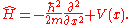
For a particle in three dimensions, the only difference is more derivatives:

and for N particles, the difference is that the wavefunction is in 3N-dimensional configuration space, the space of all possible particle positions.

This last equation is in a very high dimension, so that the solutions are not easy to visualize.
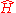 . The time-independent Schrödinger equation can be obtained from the time-dependent version by assuming a trivial time dependence of the wavefunction of the form
. The time-independent Schrödinger equation can be obtained from the time-dependent version by assuming a trivial time dependence of the wavefunction of the form 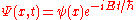 .
.
This is possible only if the Hamiltonian is not an explicit function of time, as otherwise the equation is not separable
into its spatial and temporal parts. The operator can then be replaced by
can then be replaced by  . In abstract form, for a general quantum system, it is written:.
. In abstract form, for a general quantum system, it is written:.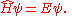
For a particle in one dimension,
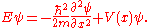
But there is a further restriction—the solution must not grow at infinity, so that it has either a finite L2-norm (if it is a bound state
) or a slowly diverging norm (if it is part of a continuum
):
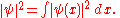
For example, when there is no potential, the equation reads:
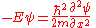
which has oscillatory solutions for E > 0 (the Cn are arbitrary constants):
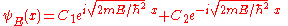
and exponential solutions for E < 0

The exponentially growing solutions have an infinite norm, and are not physical. They are not allowed in a finite volume with periodic or fixed boundary conditions.
For a constant potential V the solution is oscillatory for E > V and exponential for E < V, corresponding to energies that are allowed or disallowed in classical mechanics. Oscillatory solutions have a classically allowed energy and correspond to actual classical motions, while the exponential solutions have a disallowed energy and describe a small amount of quantum bleeding into the classically disallowed region, due to quantum tunneling. If the potential V grows at infinity, the motion is classically confined to a finite region, which means that in quantum mechanics every solution becomes an exponential far enough away. The condition that the exponential is decreasing restricts the energy levels to a discrete set, called the allowed energies.
(in dimensionless form)

for the complex
field ψ(x,t).
This equation arises from the Hamiltonian
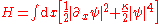
with the Poisson bracket
s
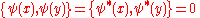

This is a classical field equation and, unlike its linear counterpart, it never describes the time evolution of a quantum state.
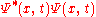 . The probability flux is defined as [in units of (probability)/(area × time)]:
. The probability flux is defined as [in units of (probability)/(area × time)]:

The probability flux satisfies the continuity equation
:
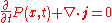
where is the probability density
is the probability density
[measured in units of (probability)/(volume)]. This equation is the mathematical equivalent of the probability
conservation law
.
For a plane wave
:

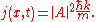
So that not only is the probability of finding the particle the same everywhere but the probability flux is as expected from an object moving at the classical velocity p/m. The reason that the Schrödinger equation admits a probability flux is because all the hopping is local and forward in time.
s and Lorentz transformation
s, the Schrödinger equation is not invariant. Changing inertial reference frames requires a transformation of the wavefunction analogous to requiring gauge invariance. This transformation introduces a phase factor that is normally ignored as non-physical, but has application in some problems. See A. B. van Oosten Apeiron, pg 449 Vol. 13, No. 2, April 2006. In order to extend Schrödinger's formalism to include relativity, the physical picture must be altered.
The Klein–Gordon equation and Dirac equation
use the relativistic mass–energy relation:

to produce the differential equations:
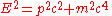
and
respectively, which are relativistically invariant.
In some special cases, special methods can be used:
Austria
Austria , officially the Republic of Austria , is a landlocked country of roughly 8.4 million people in Central Europe. It is bordered by the Czech Republic and Germany to the north, Slovakia and Hungary to the east, Slovenia and Italy to the south, and Switzerland and Liechtenstein to the...
n physicist
Physicist
A physicist is a scientist who studies or practices physics. Physicists study a wide range of physical phenomena in many branches of physics spanning all length scales: from sub-atomic particles of which all ordinary matter is made to the behavior of the material Universe as a whole...
Erwin Schrödinger
Erwin Schrödinger
Erwin Rudolf Josef Alexander Schrödinger was an Austrian physicist and theoretical biologist who was one of the fathers of quantum mechanics, and is famed for a number of important contributions to physics, especially the Schrödinger equation, for which he received the Nobel Prize in Physics in 1933...
. Used in physics
Physics
Physics is a natural science that involves the study of matter and its motion through spacetime, along with related concepts such as energy and force. More broadly, it is the general analysis of nature, conducted in order to understand how the universe behaves.Physics is one of the oldest academic...
(specifically quantum mechanics
Quantum mechanics
Quantum mechanics, also known as quantum physics or quantum theory, is a branch of physics providing a mathematical description of much of the dual particle-like and wave-like behavior and interactions of energy and matter. It departs from classical mechanics primarily at the atomic and subatomic...
), it is an equation that describes how the quantum state of a physical system
Physical system
In physics, the word system has a technical meaning, namely, it is the portion of the physical universe chosen for analysis. Everything outside the system is known as the environment, which in analysis is ignored except for its effects on the system. The cut between system and the world is a free...
changes in time.
| Time-dependent Schrödinger equation |
 |
| Time-independent Schrödinger equation |
 |
In the standard interpretation of quantum mechanics
Copenhagen interpretation
The Copenhagen interpretation is one of the earliest and most commonly taught interpretations of quantum mechanics. It holds that quantum mechanics does not yield a description of an objective reality but deals only with probabilities of observing, or measuring, various aspects of energy quanta,...
, the quantum state, also called a wavefunction
Wavefunction
Not to be confused with the related concept of the Wave equationA wave function or wavefunction is a probability amplitude in quantum mechanics describing the quantum state of a particle and how it behaves. Typically, its values are complex numbers and, for a single particle, it is a function of...
or state vector, is the most complete description that can be given to a physical system. Solutions to Schrödinger's equation describe not only molecular
Molecule
A molecule is an electrically neutral group of at least two atoms held together by covalent chemical bonds. Molecules are distinguished from ions by their electrical charge...
, atom
Atom
The atom is a basic unit of matter that consists of a dense central nucleus surrounded by a cloud of negatively charged electrons. The atomic nucleus contains a mix of positively charged protons and electrically neutral neutrons...
ic, and subatomic
Subatomic particle
In physics or chemistry, subatomic particles are the smaller particles composing nucleons and atoms. There are two types of subatomic particles: elementary particles, which are not made of other particles, and composite particles...
systems, but also macroscopic systems, possibly even the whole universe
Universe
The Universe is commonly defined as the totality of everything that exists, including all matter and energy, the planets, stars, galaxies, and the contents of intergalactic space. Definitions and usage vary and similar terms include the cosmos, the world and nature...
.
The most general form is the time-dependent Schrödinger equation, which gives a description of a system evolving with time. For systems in a stationary state
Stationary state
In quantum mechanics, a stationary state is an eigenvector of the Hamiltonian, implying the probability density associated with the wavefunction is independent of time . This corresponds to a quantum state with a single definite energy...
(i.e., where the Hamiltonian
Hamiltonian (quantum mechanics)
In quantum mechanics, the Hamiltonian H, also Ȟ or Ĥ, is the operator corresponding to the total energy of the system. Its spectrum is the set of possible outcomes when one measures the total energy of a system...
is not explicitly dependent on time), the time-independent Schrödinger equation is sufficient. Approximate solutions to the time-independent Schrödinger equation are commonly used to calculate the energy level
Energy level
A quantum mechanical system or particle that is bound -- that is, confined spatially—can only take on certain discrete values of energy. This contrasts with classical particles, which can have any energy. These discrete values are called energy levels...
s and other properties of atoms and molecules.
Schrödinger's equation can be mathematically transformed into Werner Heisenberg
Werner Heisenberg
Werner Karl Heisenberg was a German theoretical physicist who made foundational contributions to quantum mechanics and is best known for asserting the uncertainty principle of quantum theory...
's matrix mechanics
Matrix mechanics
Matrix mechanics is a formulation of quantum mechanics created by Werner Heisenberg, Max Born, and Pascual Jordan in 1925.Matrix mechanics was the first conceptually autonomous and logically consistent formulation of quantum mechanics. It extended the Bohr Model by describing how the quantum jumps...
, and into Richard Feynman
Richard Feynman
Richard Phillips Feynman was an American physicist known for his work in the path integral formulation of quantum mechanics, the theory of quantum electrodynamics and the physics of the superfluidity of supercooled liquid helium, as well as in particle physics...
's path integral formulation
Path integral formulation
The path integral formulation of quantum mechanics is a description of quantum theory which generalizes the action principle of classical mechanics...
. The Schrödinger equation describes time in a way that is inconvenient for relativistic theories, a problem that is not as severe in matrix mechanics and completely absent in the path integral formulation.
The Schrödinger equation
The Schrödinger equation takes several different forms, depending on the physical situation. This section presents the equation for the general case and for the simple case encountered in many textbooks.General quantum system
For a general quantum system: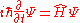
where
-
 is the wave function,
is the wave function, -
 is the energy operator (
is the energy operator (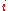 is the imaginary unitImaginary unitIn mathematics, the imaginary unit allows the real number system ℝ to be extended to the complex number system ℂ, which in turn provides at least one root for every polynomial . The imaginary unit is denoted by , , or the Greek...
is the imaginary unitImaginary unitIn mathematics, the imaginary unit allows the real number system ℝ to be extended to the complex number system ℂ, which in turn provides at least one root for every polynomial . The imaginary unit is denoted by , , or the Greek...
and is the reduced Planck constant),
is the reduced Planck constant), -
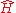 is the HamiltonianHamiltonian (quantum mechanics)In quantum mechanics, the Hamiltonian H, also Ȟ or Ĥ, is the operator corresponding to the total energy of the system. Its spectrum is the set of possible outcomes when one measures the total energy of a system...
is the HamiltonianHamiltonian (quantum mechanics)In quantum mechanics, the Hamiltonian H, also Ȟ or Ĥ, is the operator corresponding to the total energy of the system. Its spectrum is the set of possible outcomes when one measures the total energy of a system...
operatorOperator (physics)In physics, an operator is a function acting on the space of physical states. As a resultof its application on a physical state, another physical state is obtained, very often along withsome extra relevant information....
.
Single particle in a potential
For a single particle with potential energyPotential energy
In physics, potential energy is the energy stored in a body or in a system due to its position in a force field or due to its configuration. The SI unit of measure for energy and work is the Joule...
V in position space, the Schrödinger equation takes the form:
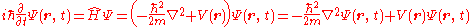
where
-
 is the kinetic energyKinetic energyThe kinetic energy of an object is the energy which it possesses due to its motion.It is defined as the work needed to accelerate a body of a given mass from rest to its stated velocity. Having gained this energy during its acceleration, the body maintains this kinetic energy unless its speed changes...
is the kinetic energyKinetic energyThe kinetic energy of an object is the energy which it possesses due to its motion.It is defined as the work needed to accelerate a body of a given mass from rest to its stated velocity. Having gained this energy during its acceleration, the body maintains this kinetic energy unless its speed changes...
operator, where m is the massMassMass can be defined as a quantitive measure of the resistance an object has to change in its velocity.In physics, mass commonly refers to any of the following three properties of matter, which have been shown experimentally to be equivalent:...
of the particle. -
 is the Laplace operatorLaplace operatorIn mathematics the Laplace operator or Laplacian is a differential operator given by the divergence of the gradient of a function on Euclidean space. It is usually denoted by the symbols ∇·∇, ∇2 or Δ...
is the Laplace operatorLaplace operatorIn mathematics the Laplace operator or Laplacian is a differential operator given by the divergence of the gradient of a function on Euclidean space. It is usually denoted by the symbols ∇·∇, ∇2 or Δ...
. In three dimensions, the Laplace operator is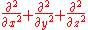 , where x, y, and z are the Cartesian coordinatesCartesian coordinate systemA Cartesian coordinate system specifies each point uniquely in a plane by a pair of numerical coordinates, which are the signed distances from the point to two fixed perpendicular directed lines, measured in the same unit of length...
, where x, y, and z are the Cartesian coordinatesCartesian coordinate systemA Cartesian coordinate system specifies each point uniquely in a plane by a pair of numerical coordinates, which are the signed distances from the point to two fixed perpendicular directed lines, measured in the same unit of length...
of space. -
 is the time-independent potential energyPotential energyIn physics, potential energy is the energy stored in a body or in a system due to its position in a force field or due to its configuration. The SI unit of measure for energy and work is the Joule...
is the time-independent potential energyPotential energyIn physics, potential energy is the energy stored in a body or in a system due to its position in a force field or due to its configuration. The SI unit of measure for energy and work is the Joule...
at the position r. -
 is the wave function for the particle at position r at time t.
is the wave function for the particle at position r at time t. -
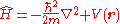 is the Hamiltonian operator for a single particle in a potential.
is the Hamiltonian operator for a single particle in a potential.
Time independent or stationary equation
The time independent equation, again for a single particle with potential energy V, takes the form: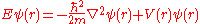
This equation describes the standing wave
Standing wave
In physics, a standing wave – also known as a stationary wave – is a wave that remains in a constant position.This phenomenon can occur because the medium is moving in the opposite direction to the wave, or it can arise in a stationary medium as a result of interference between two waves traveling...
solutions of the time-dependent equation, which are the states with definite energy (instead of a probability distribution of different energies). In physics, these standing waves are called "stationary state
Stationary state
In quantum mechanics, a stationary state is an eigenvector of the Hamiltonian, implying the probability density associated with the wavefunction is independent of time . This corresponds to a quantum state with a single definite energy...
s" or "energy eigenstates"; in chemistry they are called "atomic orbital
Atomic orbital
An atomic orbital is a mathematical function that describes the wave-like behavior of either one electron or a pair of electrons in an atom. This function can be used to calculate the probability of finding any electron of an atom in any specific region around the atom's nucleus...
s" or "molecular orbital
Molecular orbital
In chemistry, a molecular orbital is a mathematical function describing the wave-like behavior of an electron in a molecule. This function can be used to calculate chemical and physical properties such as the probability of finding an electron in any specific region. The term "orbital" was first...
s".
Historical background and development
Following Max PlanckMax Planck
Max Karl Ernst Ludwig Planck, ForMemRS, was a German physicist who actualized the quantum physics, initiating a revolution in natural science and philosophy. He is regarded as the founder of the quantum theory, for which he received the Nobel Prize in Physics in 1918.-Life and career:Planck came...
's quantization of light (see black body radiation), Albert Einstein
Albert Einstein
Albert Einstein was a German-born theoretical physicist who developed the theory of general relativity, effecting a revolution in physics. For this achievement, Einstein is often regarded as the father of modern physics and one of the most prolific intellects in human history...
interpreted Planck's quanta
Quantum
In physics, a quantum is the minimum amount of any physical entity involved in an interaction. Behind this, one finds the fundamental notion that a physical property may be "quantized," referred to as "the hypothesis of quantization". This means that the magnitude can take on only certain discrete...
to be photon
Photon
In physics, a photon is an elementary particle, the quantum of the electromagnetic interaction and the basic unit of light and all other forms of electromagnetic radiation. It is also the force carrier for the electromagnetic force...
s, particles of light, and proposed that the energy of a photon is proportional to its frequency, one of the first signs of wave–particle duality
Wave–particle duality
Wave–particle duality postulates that all particles exhibit both wave and particle properties. A central concept of quantum mechanics, this duality addresses the inability of classical concepts like "particle" and "wave" to fully describe the behavior of quantum-scale objects...
. Since energy
Energy
In physics, energy is an indirectly observed quantity. It is often understood as the ability a physical system has to do work on other physical systems...
and momentum
Momentum
In classical mechanics, linear momentum or translational momentum is the product of the mass and velocity of an object...
are related in the same way as frequency
Frequency
Frequency is the number of occurrences of a repeating event per unit time. It is also referred to as temporal frequency.The period is the duration of one cycle in a repeating event, so the period is the reciprocal of the frequency...
and wavenumber
Wavenumber
In the physical sciences, the wavenumber is a property of a wave, its spatial frequency, that is proportional to the reciprocal of the wavelength. It is also the magnitude of the wave vector...
in special relativity
Special relativity
Special relativity is the physical theory of measurement in an inertial frame of reference proposed in 1905 by Albert Einstein in the paper "On the Electrodynamics of Moving Bodies".It generalizes Galileo's...
, it followed that the momentum p of a photon is proportional to its wavenumber k.

Louis de Broglie hypothesized that this is true for all particles, even particles such as electrons. He showed that, assuming that the matter waves propagate along with their particle counterparts, electrons form standing wave
Standing wave
In physics, a standing wave – also known as a stationary wave – is a wave that remains in a constant position.This phenomenon can occur because the medium is moving in the opposite direction to the wave, or it can arise in a stationary medium as a result of interference between two waves traveling...
s, meaning that only certain discrete rotational frequencies about the nucleus of an atom are allowed. These quantized orbits correspond to discrete energy level
Energy level
A quantum mechanical system or particle that is bound -- that is, confined spatially—can only take on certain discrete values of energy. This contrasts with classical particles, which can have any energy. These discrete values are called energy levels...
s, which reproduced the old quantum condition
Old quantum theory
The old quantum theory was a collection of results from the years 1900–1925 which predate modern quantum mechanics. The theory was never complete or self-consistent, but was a collection of heuristic prescriptions which are now understood to be the first quantum corrections to classical mechanics...
.
Following up on these ideas, Schrödinger decided to find a proper wave equation for the electron. He was guided by William R. Hamilton
William Rowan Hamilton
Sir William Rowan Hamilton was an Irish physicist, astronomer, and mathematician, who made important contributions to classical mechanics, optics, and algebra. His studies of mechanical and optical systems led him to discover new mathematical concepts and techniques...
's analogy between mechanics
Mechanics
Mechanics is the branch of physics concerned with the behavior of physical bodies when subjected to forces or displacements, and the subsequent effects of the bodies on their environment....
and optics
Optics
Optics is the branch of physics which involves the behavior and properties of light, including its interactions with matter and the construction of instruments that use or detect it. Optics usually describes the behavior of visible, ultraviolet, and infrared light...
, encoded in the observation that the zero-wavelength limit of optics resembles a mechanical system — the trajectories of light rays become sharp tracks that obey Fermat's principle
Fermat's principle
In optics, Fermat's principle or the principle of least time is the principle that the path taken between two points by a ray of light is the path that can be traversed in the least time. This principle is sometimes taken as the definition of a ray of light...
, an analog of the principle of least action
Principle of least action
In physics, the principle of least action – or, more accurately, the principle of stationary action – is a variational principle that, when applied to the action of a mechanical system, can be used to obtain the equations of motion for that system...
. A modern version of his reasoning is reproduced in the next section. The equation he found is:

Using this equation, Schrödinger computed the hydrogen spectral series
Hydrogen spectral series
The emission spectrum of atomic hydrogen is divided into a number of spectral series, with wavelengths given by the Rydberg formula. These observed spectral lines are due to electrons moving between energy levels in the atom. The spectral series are important in astronomy for detecting the presence...
by treating a hydrogen atom
Hydrogen atom
A hydrogen atom is an atom of the chemical element hydrogen. The electrically neutral atom contains a single positively-charged proton and a single negatively-charged electron bound to the nucleus by the Coulomb force...
's electron
Electron
The electron is a subatomic particle with a negative elementary electric charge. It has no known components or substructure; in other words, it is generally thought to be an elementary particle. An electron has a mass that is approximately 1/1836 that of the proton...
as a wave Ψ(x, t), moving in a potential well
Potential well
A potential well is the region surrounding a local minimum of potential energy. Energy captured in a potential well is unable to convert to another type of energy because it is captured in the local minimum of a potential well...
V, created by the proton
Proton
The proton is a subatomic particle with the symbol or and a positive electric charge of 1 elementary charge. One or more protons are present in the nucleus of each atom, along with neutrons. The number of protons in each atom is its atomic number....
. This computation accurately reproduced the energy levels of the Bohr model
Bohr model
In atomic physics, the Bohr model, introduced by Niels Bohr in 1913, depicts the atom as a small, positively charged nucleus surrounded by electrons that travel in circular orbits around the nucleus—similar in structure to the solar system, but with electrostatic forces providing attraction,...
.
However, by that time, Arnold Sommerfeld
Arnold Sommerfeld
Arnold Johannes Wilhelm Sommerfeld was a German theoretical physicist who pioneered developments in atomic and quantum physics, and also educated and groomed a large number of students for the new era of theoretical physics...
had refined the Bohr model with relativistic corrections
Fine structure
In atomic physics, the fine structure describes the splitting of the spectral lines of atoms due to first order relativistic corrections.The gross structure of line spectra is the line spectra predicted by non-relativistic electrons with no spin. For a hydrogenic atom, the gross structure energy...
. Schrödinger used the relativistic energy momentum relation to find what is now known as the Klein–Gordon equation in a Coulomb potential (in natural units
Natural units
In physics, natural units are physical units of measurement based only on universal physical constants. For example the elementary charge e is a natural unit of electric charge, or the speed of light c is a natural unit of speed...
):

He found the standing waves of this relativistic equation, but the relativistic corrections disagreed with Sommerfeld's formula. Discouraged, he put away his calculations and secluded himself in an isolated mountain cabin with a lover.
While at the cabin, Schrödinger decided that his earlier non-relativistic calculations were novel enough to publish, and decided to leave off the problem of relativistic corrections for the future. He put together his wave equation and the spectral analysis of hydrogen in a paper in 1926. The paper was enthusiastically endorsed by Einstein, who saw the matter-waves as an intuitive depiction of nature, as opposed to Heisenberg's matrix mechanics
Matrix mechanics
Matrix mechanics is a formulation of quantum mechanics created by Werner Heisenberg, Max Born, and Pascual Jordan in 1925.Matrix mechanics was the first conceptually autonomous and logically consistent formulation of quantum mechanics. It extended the Bohr Model by describing how the quantum jumps...
, which he considered overly formal.
The Schrödinger equation details the behaviour of ψ but says nothing of its nature. Schrödinger tried to interpret it as a charge density in his fourth paper, but he was unsuccessful. In 1926, just a few days after Schrödinger's fourth and final paper was published, Max Born
Max Born
Max Born was a German-born physicist and mathematician who was instrumental in the development of quantum mechanics. He also made contributions to solid-state physics and optics and supervised the work of a number of notable physicists in the 1920s and 30s...
successfully interpreted ψ as a quantity related to the probability amplitude
Probability amplitude
In quantum mechanics, a probability amplitude is a complex number whose modulus squared represents a probability or probability density.For example, if the probability amplitude of a quantum state is \alpha, the probability of measuring that state is |\alpha|^2...
, which is equal to the squared magnitude of ψ. Schrödinger, though, always opposed a statistical or probabilistic approach, with its associated discontinuities
Wavefunction collapse
In quantum mechanics, wave function collapse is the phenomenon in which a wave function—initially in a superposition of several different possible eigenstates—appears to reduce to a single one of those states after interaction with an observer...
—much like Einstein, who believed that quantum mechanics was a statistical approximation to an underlying deterministic theory
Determinism
Determinism is the general philosophical thesis that states that for everything that happens there are conditions such that, given them, nothing else could happen. There are many versions of this thesis. Each of them rests upon various alleged connections, and interdependencies of things and...
— and never reconciled with the Copenhagen interpretation
Copenhagen interpretation
The Copenhagen interpretation is one of the earliest and most commonly taught interpretations of quantum mechanics. It holds that quantum mechanics does not yield a description of an objective reality but deals only with probabilities of observing, or measuring, various aspects of energy quanta,...
.
Short heuristic derivation
Schrödinger's equation can be derived in the following short heuristic way.Assumptions
- The total energyEnergyIn physics, energy is an indirectly observed quantity. It is often understood as the ability a physical system has to do work on other physical systems...
E of a particle is
- This is the classical expression for a particle with mass m where the total energy E is the sum of the kinetic energyKinetic energyThe kinetic energy of an object is the energy which it possesses due to its motion.It is defined as the work needed to accelerate a body of a given mass from rest to its stated velocity. Having gained this energy during its acceleration, the body maintains this kinetic energy unless its speed changes...
T, and the potential energyPotential energyIn physics, potential energy is the energy stored in a body or in a system due to its position in a force field or due to its configuration. The SI unit of measure for energy and work is the Joule...
V (which can vary with positionHarmonic functionIn mathematics, mathematical physics and the theory of stochastic processes, a harmonic function is a twice continuously differentiable function f : U → R which satisfies Laplace's equation, i.e....
, and timeTimeTime is a part of the measuring system used to sequence events, to compare the durations of events and the intervals between them, and to quantify rates of change such as the motions of objects....
). p and m are respectively the momentumMomentumIn classical mechanics, linear momentum or translational momentum is the product of the mass and velocity of an object...
and the massMassMass can be defined as a quantitive measure of the resistance an object has to change in its velocity.In physics, mass commonly refers to any of the following three properties of matter, which have been shown experimentally to be equivalent:...
of the particle.
- Einstein's light quanta hypothesisPhotoelectric effectIn the photoelectric effect, electrons are emitted from matter as a consequence of their absorption of energy from electromagnetic radiation of very short wavelength, such as visible or ultraviolet light. Electrons emitted in this manner may be referred to as photoelectrons...
of 1905, which asserts that the energy E of a photon is proportional to the frequencyFrequencyFrequency is the number of occurrences of a repeating event per unit time. It is also referred to as temporal frequency.The period is the duration of one cycle in a repeating event, so the period is the reciprocal of the frequency...
ν (or angular frequencyAngular frequencyIn physics, angular frequency ω is a scalar measure of rotation rate. Angular frequency is the magnitude of the vector quantity angular velocity...
, ω = 2πν) of the corresponding electromagnetic wave: - The de Broglie hypothesis of 1924, which states that any particle can be associated with a wave, and that the momentum p of the particle is related to the wavelengthWavelengthIn physics, the wavelength of a sinusoidal wave is the spatial period of the wave—the distance over which the wave's shape repeats.It is usually determined by considering the distance between consecutive corresponding points of the same phase, such as crests, troughs, or zero crossings, and is a...
λ (or wavenumberWavenumberIn the physical sciences, the wavenumber is a property of a wave, its spatial frequency, that is proportional to the reciprocal of the wavelength. It is also the magnitude of the wave vector...
k) of such a wave by: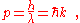
- Expressing p and k as vectors, we have

The three assumptions above allow one to derive the equation for plane wave
Plane wave
In the physics of wave propagation, a plane wave is a constant-frequency wave whose wavefronts are infinite parallel planes of constant peak-to-peak amplitude normal to the phase velocity vector....
s only. To conclude that it is true in general requires the superposition principle
Superposition principle
In physics and systems theory, the superposition principle , also known as superposition property, states that, for all linear systems, the net response at a given place and time caused by two or more stimuli is the sum of the responses which would have been caused by each stimulus individually...
, and thus, one must separately postulate that the Schrödinger equation is linear
Linear differential equation
Linear differential equations are of the formwhere the differential operator L is a linear operator, y is the unknown function , and the right hand side ƒ is a given function of the same nature as y...
.
Expressing the wave function as a complex plane wave
Schrödinger's idea was to express the phasePhase (waves)
Phase in waves is the fraction of a wave cycle which has elapsed relative to an arbitrary point.-Formula:The phase of an oscillation or wave refers to a sinusoidal function such as the following:...
of a plane wave
Plane wave
In the physics of wave propagation, a plane wave is a constant-frequency wave whose wavefronts are infinite parallel planes of constant peak-to-peak amplitude normal to the phase velocity vector....
as a complex
Complex number
A complex number is a number consisting of a real part and an imaginary part. Complex numbers extend the idea of the one-dimensional number line to the two-dimensional complex plane by using the number line for the real part and adding a vertical axis to plot the imaginary part...
phase factor
Phase factor
For any complex number written in polar form , the phase factor is the exponential part, i.e. eiθ. As such, the term "phase factor" is similar to the term phasor, although the former term is more common in quantum mechanics. This phase factor is itself a complex number of absolute value 1...
:
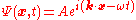
and to realize that since

then
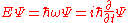
and similarly since
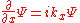
and
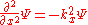
we find:
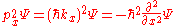
so that, again for a plane wave, he obtained:

And, by inserting these expressions for the energy and momentum into the classical formula we started with, we get Schrödinger's famed equation, for a single particle in the 3-dimensional case in the presence of a potential V:

Time dependent equation
This is the equation of motion for the quantum state. In the most general form, it is written: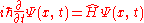
where
 is a linear operator acting on the wavefunction Ψ. For the specific case of a single particle in one dimension moving under the influence of a potential V.
is a linear operator acting on the wavefunction Ψ. For the specific case of a single particle in one dimension moving under the influence of a potential V.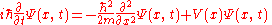
and the operator
 can be read off:
can be read off: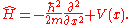
For a particle in three dimensions, the only difference is more derivatives:

and for N particles, the difference is that the wavefunction is in 3N-dimensional configuration space, the space of all possible particle positions.

This last equation is in a very high dimension, so that the solutions are not easy to visualize.
Time-independent equation
This is the equation for the standing waves, the eigenvalue equation for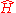 . The time-independent Schrödinger equation can be obtained from the time-dependent version by assuming a trivial time dependence of the wavefunction of the form
. The time-independent Schrödinger equation can be obtained from the time-dependent version by assuming a trivial time dependence of the wavefunction of the form 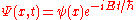 .
.This is possible only if the Hamiltonian is not an explicit function of time, as otherwise the equation is not separable
Separation of variables
In mathematics, separation of variables is any of several methods for solving ordinary and partial differential equations, in which algebra allows one to rewrite an equation so that each of two variables occurs on a different side of the equation....
into its spatial and temporal parts. The operator
 can then be replaced by
can then be replaced by  . In abstract form, for a general quantum system, it is written:.
. In abstract form, for a general quantum system, it is written:.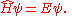
For a particle in one dimension,
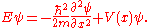
But there is a further restriction—the solution must not grow at infinity, so that it has either a finite L2-norm (if it is a bound state
Bound state
In physics, a bound state describes a system where a particle is subject to a potential such that the particle has a tendency to remain localised in one or more regions of space...
) or a slowly diverging norm (if it is part of a continuum
Continuum (theory)
Continuum theories or models explain variation as involving a gradual quantitative transition without abrupt changes or discontinuities. It can be contrasted with 'categorical' models which propose qualitatively different states.-In physics:...
):
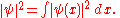
For example, when there is no potential, the equation reads:
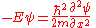
which has oscillatory solutions for E > 0 (the Cn are arbitrary constants):
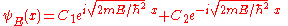
and exponential solutions for E < 0

The exponentially growing solutions have an infinite norm, and are not physical. They are not allowed in a finite volume with periodic or fixed boundary conditions.
For a constant potential V the solution is oscillatory for E > V and exponential for E < V, corresponding to energies that are allowed or disallowed in classical mechanics. Oscillatory solutions have a classically allowed energy and correspond to actual classical motions, while the exponential solutions have a disallowed energy and describe a small amount of quantum bleeding into the classically disallowed region, due to quantum tunneling. If the potential V grows at infinity, the motion is classically confined to a finite region, which means that in quantum mechanics every solution becomes an exponential far enough away. The condition that the exponential is decreasing restricts the energy levels to a discrete set, called the allowed energies.
Nonlinear equation
The nonlinear Schrödinger equation is the partial differential equationPartial differential equation
In mathematics, partial differential equations are a type of differential equation, i.e., a relation involving an unknown function of several independent variables and their partial derivatives with respect to those variables...
(in dimensionless form)

for the complex
Complex number
A complex number is a number consisting of a real part and an imaginary part. Complex numbers extend the idea of the one-dimensional number line to the two-dimensional complex plane by using the number line for the real part and adding a vertical axis to plot the imaginary part...
field ψ(x,t).
This equation arises from the Hamiltonian
Hamiltonian (quantum mechanics)
In quantum mechanics, the Hamiltonian H, also Ȟ or Ĥ, is the operator corresponding to the total energy of the system. Its spectrum is the set of possible outcomes when one measures the total energy of a system...
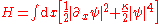
with the Poisson bracket
Poisson bracket
In mathematics and classical mechanics, the Poisson bracket is an important binary operation in Hamiltonian mechanics, playing a central role in Hamilton's equations of motion, which govern the time-evolution of a Hamiltonian dynamical system...
s
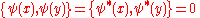

This is a classical field equation and, unlike its linear counterpart, it never describes the time evolution of a quantum state.
Local conservation of probability
The probability density of a particle is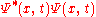 . The probability flux is defined as [in units of (probability)/(area × time)]:
. The probability flux is defined as [in units of (probability)/(area × time)]:
The probability flux satisfies the continuity equation
Continuity equation
A continuity equation in physics is a differential equation that describes the transport of a conserved quantity. Since mass, energy, momentum, electric charge and other natural quantities are conserved under their respective appropriate conditions, a variety of physical phenomena may be described...
:
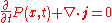
where
 is the probability density
is the probability densityProbability density function
In probability theory, a probability density function , or density of a continuous random variable is a function that describes the relative likelihood for this random variable to occur at a given point. The probability for the random variable to fall within a particular region is given by the...
[measured in units of (probability)/(volume)]. This equation is the mathematical equivalent of the probability
Probability
Probability is ordinarily used to describe an attitude of mind towards some proposition of whose truth we arenot certain. The proposition of interest is usually of the form "Will a specific event occur?" The attitude of mind is of the form "How certain are we that the event will occur?" The...
conservation law
Conservation law
In physics, a conservation law states that a particular measurable property of an isolated physical system does not change as the system evolves....
.
For a plane wave
Plane wave
In the physics of wave propagation, a plane wave is a constant-frequency wave whose wavefronts are infinite parallel planes of constant peak-to-peak amplitude normal to the phase velocity vector....
:

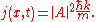
So that not only is the probability of finding the particle the same everywhere but the probability flux is as expected from an object moving at the classical velocity p/m. The reason that the Schrödinger equation admits a probability flux is because all the hopping is local and forward in time.
Relativity
Under both Galilean transformationGalilean transformation
The Galilean transformation is used to transform between the coordinates of two reference frames which differ only by constant relative motion within the constructs of Newtonian physics. This is the passive transformation point of view...
s and Lorentz transformation
Lorentz transformation
In physics, the Lorentz transformation or Lorentz-Fitzgerald transformation describes how, according to the theory of special relativity, two observers' varying measurements of space and time can be converted into each other's frames of reference. It is named after the Dutch physicist Hendrik...
s, the Schrödinger equation is not invariant. Changing inertial reference frames requires a transformation of the wavefunction analogous to requiring gauge invariance. This transformation introduces a phase factor that is normally ignored as non-physical, but has application in some problems. See A. B. van Oosten Apeiron, pg 449 Vol. 13, No. 2, April 2006. In order to extend Schrödinger's formalism to include relativity, the physical picture must be altered.
The Klein–Gordon equation and Dirac equation
Dirac equation
The Dirac equation is a relativistic quantum mechanical wave equation formulated by British physicist Paul Dirac in 1928. It provided a description of elementary spin-½ particles, such as electrons, consistent with both the principles of quantum mechanics and the theory of special relativity, and...
use the relativistic mass–energy relation:

to produce the differential equations:
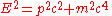
and

respectively, which are relativistically invariant.
Solutions
Some general techniques are:- Perturbation theoryPerturbation theory (quantum mechanics)In quantum mechanics, perturbation theory is a set of approximation schemes directly related to mathematical perturbation for describing a complicated quantum system in terms of a simpler one. The idea is to start with a simple system for which a mathematical solution is known, and add an...
- The variational method
- Quantum Monte CarloQuantum Monte CarloQuantum Monte Carlo is a large class of computer algorithms that simulate quantum systems with the idea of solving the quantum many-body problem. They use, in one way or another, the Monte Carlo method to handle the many-dimensional integrals that arise...
methods - Density functional theoryDensity functional theoryDensity functional theory is a quantum mechanical modelling method used in physics and chemistry to investigate the electronic structure of many-body systems, in particular atoms, molecules, and the condensed phases. With this theory, the properties of a many-electron system can be determined by...
- The WKB approximationWKB approximationIn mathematical physics, the WKB approximation or WKB method is a method for finding approximate solutions to linear partial differential equations with spatially varying coefficients...
and semi-classical expansion
In some special cases, special methods can be used:
- List of quantum-mechanical systems with analytical solutions
- Hartree-FockHartree-FockIn computational physics and chemistry, the Hartree–Fock method is an approximate method for the determination of the ground-state wave function and ground-state energy of a quantum many-body system....
method and post Hartree-Fock methods - Discrete delta-potential methodDiscrete delta-potential methodThe discrete delta potential method is a combination of both numerical and analytic method used to solve the Schrödinger equation the main feature of this method is to obtain first a discrete approximation of the potential in the form:...
See also
- Basic concepts of quantum mechanicsBasic concepts of quantum mechanicsQuantum mechanics explains the behaviour of matter and energy on the scale of atoms and subatomic particles.Classical physics explains matter and energy at the macroscopic level of the scale familiar to human experience, including the behavior of astronomical bodies...
- Dirac equationDirac equationThe Dirac equation is a relativistic quantum mechanical wave equation formulated by British physicist Paul Dirac in 1928. It provided a description of elementary spin-½ particles, such as electrons, consistent with both the principles of quantum mechanics and the theory of special relativity, and...
- P-adic quantum mechanicsP-adic quantum mechanicsP-adic quantum mechanics is a relatively recent approach to understanding the nature of fundamental physics. It is the application of p-adic analysis to quantum mechanics. The p-adic numbers are a counterintuitive arithmetic system that was discovered by the German mathematician Kurt Hensel in...
- Quantum chaosQuantum chaosQuantum chaos is a branch of physics which studies how chaotic classical dynamical systems can be described in terms of quantum theory. The primary question that quantum chaos seeks to answer is, "What is the relationship between quantum mechanics and classical chaos?" The correspondence principle...
- Quantum numberQuantum numberQuantum numbers describe values of conserved quantities in the dynamics of the quantum system. Perhaps the most peculiar aspect of quantum mechanics is the quantization of observable quantities. This is distinguished from classical mechanics where the values can range continuously...
- Relation between Schrödinger's equation and the path integral formulation of quantum mechanicsRelation between Schrödinger's equation and the path integral formulation of quantum mechanicsThis article relates the Schrödinger equation with the path integral formulation of quantum mechanics using a simple nonrelativistic one-dimensional single-particle Hamiltonian composed of kinetic and potential energy.-Schrödinger's equation:...
- Schrödinger's catSchrödinger's catSchrödinger's cat is a thought experiment, usually described as a paradox, devised by Austrian physicist Erwin Schrödinger in 1935. It illustrates what he saw as the problem of the Copenhagen interpretation of quantum mechanics applied to everyday objects. The scenario presents a cat that might be...
- Schrödinger fieldSchrödinger fieldIn quantum mechanics and quantum field theory, a Schrödinger field, named after Erwin Schrödinger, is a quantum field which obeys the Schrödinger equation...
- Schrödinger pictureSchrödinger pictureIn physics, the Schrödinger picture is a formulation of quantum mechanics in which the state vectors evolve in time, but the operators are constant. This differs from the Heisenberg picture which keeps the states constant while the observables evolve in time...
- Theoretical and experimental justification for the Schrödinger equationTheoretical and experimental justification for the Schrödinger equationThe theoretical and experimental justification for the Schrödinger equation motivates the discovery of the Schrödinger equation, the equation that describes the dynamics of nonrelativistic particles...
External links
- Quantum Physics - textbook with a treatment of the time-independent Schrödinger equation
- Linear Schrödinger Equation at EqWorld: The World of Mathematical Equations.
- Nonlinear Schrödinger Equation at EqWorld: The World of Mathematical Equations.
- The Schrödinger Equation in One Dimension as well as the directory of the book.
- All about 3D Schrödinger Equation
- Mathematical aspects of Schrödinger equations are discussed on the Dispersive PDE Wiki.
- Web-Schrödinger: Interactive solution of the 2D time dependent Schrödinger equation
- An alternate derivation of the Schrödinger Equation
- Online software-Periodic Potential Lab Solves the time independent Schrödinger equation for arbitrary periodic potentials.