Natural units
Encyclopedia
In physics
, natural units are physical units of measurement
based only on universal physical constants. For example the elementary charge
e is a natural unit of electric charge
, or the speed of light
c is a natural unit of speed
. A purely natural system of units is defined in such a way that some set of selected universal physical constants are normalized to unity; that is, their numerical values in terms of these units become exactly 1.
particular algebraic expression
s appearing in physical law or to normalize
some chosen physical quantities that are properties of universal elementary particle
s and that may be reasonably believed to be constant. However, what may be believed and forced to be constant in one system of natural units can very well be allowed or even assumed to vary in another natural unit system.
Natural units are natural because the origin of their definition comes only from properties of nature
and not from any human construct. Planck units
are often, without qualification, called "natural units", when in fact they constitute only one of several systems of natural units, albeit the best known such system. Planck units might be considered unique in that the set of units are not based on properties of any prototype
, object, or particle
but are solely derived from the properties of free space.
As with other systems of units, the base units of a set of natural units will include definitions and values for length
, mass
, time
, temperature
, and electric charge
(in lieu of electric current
). Some physicists do not recognize temperature as a fundamental physical quantity, since it simply expresses the energy per degree of freedom of a particle, which can be expressed in terms of energy (or mass, length, and time). Virtually every system of natural units normalizes Boltzmann's constant kB to 1, which can be thought of as simply a way of defining the unit temperature.
In the SI
unit system, electric charge is a separate fundamental dimension of physical quantity, but in natural unit systems charge is expressed in terms of the mechanical units of mass, length, and time, similarly to cgs. There are two common ways to relate charge to mass, length, and time: In "rationalized" (or "Heaviside–Lorentz") natural unit systems, Coulomb's law
is F=q1q2/(4πr2), and in "non-rationalized" (or "Gaussian
"), Coulomb's law is F=q1q2/r2. Both possibilities are incorporated into different natural unit systems.
. If a velocity v is half the speed of light, then from the equations v = c and c = 1, the consequence is v = . The equation v = means "the velocity v has the value one-half when measured in Planck units", or "the velocity v is one-half the Planck unit of velocity".
The equation c = 1 can be plugged in anywhere else. For example, Einstein's equation E = mc2 can be rewritten in Planck units as E = m. This equation means "The rest-energy of a particle, measured in Planck units of energy, equals the rest-mass of a particle, measured in Planck units of mass."
or other unit systems, natural units have both advantages and disadvantages:
Judiciously choosing units can only normalize physical constants that have dimension. Dimensionless physical constant
s cannot take on a different numerical value no matter what system of units is used. One such constant important to physics is the fine-structure constant
, α:
Since α is a fixed dimensionless number not equal to 1, it is not possible to define a system of natural units that will normalize all of the physical constants that comprise α. Any three of the four constants: c, ℏ, e, or 4πε0, can be normalized (leaving the remaining physical constant to take on a value that is a simple function of α, attesting to the fundamental nature of the fine-structure constant) but not all four.
Planck units are unique among systems of natural units, because they are not defined in terms of properties of any prototype, physical object, or even elementary particle
. They only refer to the basic structure of the laws of physics: c and G are part of the structure of spacetime
in general relativity
, and ℏ captures the relationship between energy and frequency which is at the foundation of quantum mechanics
. This makes Planck units particularly useful and common in theories of quantum gravity
, including string theory
.
Some may consider Planck units to be "more natural" even than other natural unit systems discussed below. For example, some other systems use the mass of an electron as a parameter to be normalized. But the electron is just one of 15 known massive elementary particle
s, all with different masses, and there is no compelling reason, within fundamental physics, to emphasize the electron mass over some other elementary particle's mass.
In particle physics
, the phrase "natural units" generally means:
This is yet not enough information to define a unit system. Next, this equation is almost always supplemented with a definition of charge, from one of two possibilities:
In Heaviside–Lorentz units, there are factors of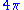
in Coulomb's law
and the Biot–Savart law but not in Maxwell's equations
; In Gaussian units, it is the reverse. Both systems are used, although Heaviside-Lorentz is more common. The value of the elementary charge
in the two systems is:
(Gaussian).
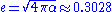(Lorentz–Heaviside).
Finally, one more unit is needed. Most commonly, electron-volt (eV) is used, despite the fact that this is not a "natural" unit in the sense discussed above. (The SI prefix
ed multiples of eV are used as well: keV, MeV, GeV, etc.)
With the addition of eV (or any other auxiliary unit), any quantity can be expressed. For example, a distance of 1 cm can be expressed in terms of eV, in natural units, as:
Stoney units are defined by:
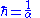
where α is the fine-structure constant
.
George Johnstone Stoney
was the first physicist to introduce the concept of natural units. He presented the idea in a lecture entitled "On the Physical Units of Nature" delivered to the British Association in 1874. Stoney units differ from Planck units by fixing the elementary charge
at 1, instead of Planck's constant (only discovered after Stoney's proposal).
Stoney units are rarely used in modern physics for calculations, but they are of historical interest.
There are two types of atomic units, closely related:
Hartree atomic units: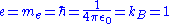
Rydberg atomic units: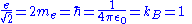
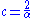
These units are designed to simplify atomic and molecular physics and chemistry, especially the hydrogen atom
, and are widely used in these fields. The Hartree units were first proposed by Douglas Hartree
, and are more common than the Rydberg units.
The units are designed especially to characterize the behavior of an electron in the ground state of a hydrogen atom. For example, using the Hartree convention, in the Bohr model
of the hydrogen atom, an electron in the ground state has orbital velocity = 1, orbital radius = 1, angular momentum = 1, ionization energy
= ½, etc.
The unit of energy
is called the Hartree energy
in the Hartree system and the Rydberg energy in the Rydberg system. They differ by a factor of 2. The speed of light
is relatively large in atomic units (137 in Hartree or 274 in Rydberg), which comes from the fact that an electron in hydrogen tends to move much slower than the speed of light. The gravitational constant
is extremely small in atomic units (around 10−45), which comes from the fact that the gravitational force between two electrons is far weaker than the Coulomb force. The unit length, mA, is the Bohr radius
, a0.
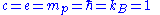
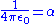
The electron mass is replaced with that of the proton
and the permittivity of free space is not fixed by definition. Strong units are convenient for work in QCD
and nuclear physics, where quantum mechanics and relativity are omnipresent and the proton is an object of central interest.
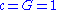
The geometrized unit system is not a completely defined or unique system. In this system, the base physical units are chosen so that the speed of light
and the gravitational constant
are set equal to unity, leaving latitude to also set some other constant such as the Boltzmann constant and Coulomb force constant
equal to unity:
If the reduced Planck constant is also set equal to unity,
then geometrized units are identical to Planck units
.
where:
Physics
Physics is a natural science that involves the study of matter and its motion through spacetime, along with related concepts such as energy and force. More broadly, it is the general analysis of nature, conducted in order to understand how the universe behaves.Physics is one of the oldest academic...
, natural units are physical units of measurement
Measurement
Measurement is the process or the result of determining the ratio of a physical quantity, such as a length, time, temperature etc., to a unit of measurement, such as the metre, second or degree Celsius...
based only on universal physical constants. For example the elementary charge
Elementary charge
The elementary charge, usually denoted as e, is the electric charge carried by a single proton, or equivalently, the absolute value of the electric charge carried by a single electron. This elementary charge is a fundamental physical constant. To avoid confusion over its sign, e is sometimes called...
e is a natural unit of electric charge
Electric charge
Electric charge is a physical property of matter that causes it to experience a force when near other electrically charged matter. Electric charge comes in two types, called positive and negative. Two positively charged substances, or objects, experience a mutual repulsive force, as do two...
, or the speed of light
Speed of light
The speed of light in vacuum, usually denoted by c, is a physical constant important in many areas of physics. Its value is 299,792,458 metres per second, a figure that is exact since the length of the metre is defined from this constant and the international standard for time...
c is a natural unit of speed
Speed
In kinematics, the speed of an object is the magnitude of its velocity ; it is thus a scalar quantity. The average speed of an object in an interval of time is the distance traveled by the object divided by the duration of the interval; the instantaneous speed is the limit of the average speed as...
. A purely natural system of units is defined in such a way that some set of selected universal physical constants are normalized to unity; that is, their numerical values in terms of these units become exactly 1.
Introduction
Natural units are intended to elegantly simplifyNondimensionalization
Nondimensionalization is the partial or full removal of units from an equation involving physical quantities by a suitable substitution of variables. This technique can simplify and parameterize problems where measured units are involved. It is closely related to dimensional analysis...
particular algebraic expression
Algebraic expression
In mathematics, an algebraic expression is an expression that contains only algebraic numbers, variables and algebraic operations. Algebraic operations are addition, subtraction, multiplication, division and exponentiation with integral or fractional exponents...
s appearing in physical law or to normalize
Normalizing constant
The concept of a normalizing constant arises in probability theory and a variety of other areas of mathematics.-Definition and examples:In probability theory, a normalizing constant is a constant by which an everywhere non-negative function must be multiplied so the area under its graph is 1, e.g.,...
some chosen physical quantities that are properties of universal elementary particle
Elementary particle
In particle physics, an elementary particle or fundamental particle is a particle not known to have substructure; that is, it is not known to be made up of smaller particles. If an elementary particle truly has no substructure, then it is one of the basic building blocks of the universe from which...
s and that may be reasonably believed to be constant. However, what may be believed and forced to be constant in one system of natural units can very well be allowed or even assumed to vary in another natural unit system.
Natural units are natural because the origin of their definition comes only from properties of nature
Nature
Nature, in the broadest sense, is equivalent to the natural world, physical world, or material world. "Nature" refers to the phenomena of the physical world, and also to life in general...
and not from any human construct. Planck units
Planck units
In physics, Planck units are physical units of measurement defined exclusively in terms of five universal physical constants listed below, in such a manner that these five physical constants take on the numerical value of 1 when expressed in terms of these units. Planck units elegantly simplify...
are often, without qualification, called "natural units", when in fact they constitute only one of several systems of natural units, albeit the best known such system. Planck units might be considered unique in that the set of units are not based on properties of any prototype
Prototype
A prototype is an early sample or model built to test a concept or process or to act as a thing to be replicated or learned from.The word prototype derives from the Greek πρωτότυπον , "primitive form", neutral of πρωτότυπος , "original, primitive", from πρῶτος , "first" and τύπος ,...
, object, or particle
Subatomic particle
In physics or chemistry, subatomic particles are the smaller particles composing nucleons and atoms. There are two types of subatomic particles: elementary particles, which are not made of other particles, and composite particles...
but are solely derived from the properties of free space.
As with other systems of units, the base units of a set of natural units will include definitions and values for length
Length
In geometric measurements, length most commonly refers to the longest dimension of an object.In certain contexts, the term "length" is reserved for a certain dimension of an object along which the length is measured. For example it is possible to cut a length of a wire which is shorter than wire...
, mass
Mass
Mass can be defined as a quantitive measure of the resistance an object has to change in its velocity.In physics, mass commonly refers to any of the following three properties of matter, which have been shown experimentally to be equivalent:...
, time
Time
Time is a part of the measuring system used to sequence events, to compare the durations of events and the intervals between them, and to quantify rates of change such as the motions of objects....
, temperature
Temperature
Temperature is a physical property of matter that quantitatively expresses the common notions of hot and cold. Objects of low temperature are cold, while various degrees of higher temperatures are referred to as warm or hot...
, and electric charge
Electric charge
Electric charge is a physical property of matter that causes it to experience a force when near other electrically charged matter. Electric charge comes in two types, called positive and negative. Two positively charged substances, or objects, experience a mutual repulsive force, as do two...
(in lieu of electric current
Electric current
Electric current is a flow of electric charge through a medium.This charge is typically carried by moving electrons in a conductor such as wire...
). Some physicists do not recognize temperature as a fundamental physical quantity, since it simply expresses the energy per degree of freedom of a particle, which can be expressed in terms of energy (or mass, length, and time). Virtually every system of natural units normalizes Boltzmann's constant kB to 1, which can be thought of as simply a way of defining the unit temperature.
In the SI
Si
Si, si, or SI may refer to :- Measurement, mathematics and science :* International System of Units , the modern international standard version of the metric system...
unit system, electric charge is a separate fundamental dimension of physical quantity, but in natural unit systems charge is expressed in terms of the mechanical units of mass, length, and time, similarly to cgs. There are two common ways to relate charge to mass, length, and time: In "rationalized" (or "Heaviside–Lorentz") natural unit systems, Coulomb's law
Coulomb's law
Coulomb's law or Coulomb's inverse-square law, is a law of physics describing the electrostatic interaction between electrically charged particles. It was first published in 1785 by French physicist Charles Augustin de Coulomb and was essential to the development of the theory of electromagnetism...
is F=q1q2/(4πr2), and in "non-rationalized" (or "Gaussian
Gaussian units
Gaussian units comprise a metric system of physical units. This system is the most common of the several electromagnetic unit systems based on cgs units. It is also called the Gaussian unit system, Gaussian-cgs units, or often just cgs units...
"), Coulomb's law is F=q1q2/r2. Both possibilities are incorporated into different natural unit systems.
Notation and use
Natural units are most commonly used by setting the units to one. For example, many natural unit systems include the equation c = 1 in the unit-system definition, where c is the speed of lightSpeed of light
The speed of light in vacuum, usually denoted by c, is a physical constant important in many areas of physics. Its value is 299,792,458 metres per second, a figure that is exact since the length of the metre is defined from this constant and the international standard for time...
. If a velocity v is half the speed of light, then from the equations v = c and c = 1, the consequence is v = . The equation v = means "the velocity v has the value one-half when measured in Planck units", or "the velocity v is one-half the Planck unit of velocity".
The equation c = 1 can be plugged in anywhere else. For example, Einstein's equation E = mc2 can be rewritten in Planck units as E = m. This equation means "The rest-energy of a particle, measured in Planck units of energy, equals the rest-mass of a particle, measured in Planck units of mass."
Advantages and disadvantages
Compared to SISi
Si, si, or SI may refer to :- Measurement, mathematics and science :* International System of Units , the modern international standard version of the metric system...
or other unit systems, natural units have both advantages and disadvantages:
- Simplified equations: By setting constants to 1, equations containing those constants appear more compact and in some cases may be simpler to understand. For example, the special relativityInvariant massThe invariant mass, rest mass, intrinsic mass, proper mass or just mass is a characteristic of the total energy and momentum of an object or a system of objects that is the same in all frames of reference related by Lorentz transformations...
equation E2 = p2c2 + m2c4 appears somewhat complicated, but the natural units version, E2 = p2 + m2, appears simpler.
- Physical interpretation: Natural unit systems automatically incorporate dimensional analysisDimensional analysisIn physics and all science, dimensional analysis is a tool to find or check relations among physical quantities by using their dimensions. The dimension of a physical quantity is the combination of the basic physical dimensions which describe it; for example, speed has the dimension length per...
. For example, in Planck unitsPlanck unitsIn physics, Planck units are physical units of measurement defined exclusively in terms of five universal physical constants listed below, in such a manner that these five physical constants take on the numerical value of 1 when expressed in terms of these units. Planck units elegantly simplify...
, the units are defined by properties of quantum mechanics and gravity. Not coincidentally, the Planck unit of length is approximately the length where quantum gravityQuantum gravityQuantum gravity is the field of theoretical physics which attempts to develop scientific models that unify quantum mechanics with general relativity...
effects become important. Likewise, atomic unitsAtomic unitsAtomic units form a system of natural units which is especially convenient for atomic physics calculations. There are two different kinds of atomic units, which one might name Hartree atomic units and Rydberg atomic units, which differ in the choice of the unit of mass and charge. This article...
are based on the mass and charge of an electron, and not coincidentally the atomic unit of length is the Bohr radiusBohr radiusThe Bohr radius is a physical constant, approximately equal to the most probable distance between the proton and electron in a hydrogen atom in its ground state. It is named after Niels Bohr, due to its role in the Bohr model of an atom...
describing the orbit of the electron in a hydrogen atomHydrogen atomA hydrogen atom is an atom of the chemical element hydrogen. The electrically neutral atom contains a single positively-charged proton and a single negatively-charged electron bound to the nucleus by the Coulomb force...
.
- No prototypes: A prototype is a physical object that defines a unit, such as the International Prototype KilogramKilogramThe kilogram or kilogramme , also known as the kilo, is the base unit of mass in the International System of Units and is defined as being equal to the mass of the International Prototype Kilogram , which is almost exactly equal to the mass of one liter of water...
, a certain cylinder whose mass is by definition exactly one kilogramKilogramThe kilogram or kilogramme , also known as the kilo, is the base unit of mass in the International System of Units and is defined as being equal to the mass of the International Prototype Kilogram , which is almost exactly equal to the mass of one liter of water...
. A prototype definition always has imperfect reproducibility between different places and between different times, and it is an advantage of natural unit systems that they use no prototypes. (They share this advantage with other non-natural unit systems, such as conventional electrical units.)
- Less precise measurements: SISiSi, si, or SI may refer to :- Measurement, mathematics and science :* International System of Units , the modern international standard version of the metric system...
units are designed to be used in precision measurements. For example, the secondSecondThe second is a unit of measurement of time, and is the International System of Units base unit of time. It may be measured using a clock....
is defined by an atomic transition frequency in cesium atoms, because this transition frequency can be precisely reproduced with atomic clockAtomic clockAn atomic clock is a clock that uses an electronic transition frequency in the microwave, optical, or ultraviolet region of the electromagnetic spectrum of atoms as a frequency standard for its timekeeping element...
technology. Natural unit systems are generally not based on quantities that can be precisely reproduced in a lab. Therefore, a quantity measured in natural units can have fewer digits of precision than the same quantity measured in SI. For example, Planck units use the gravitational constant GGravitational constantThe gravitational constant, denoted G, is an empirical physical constant involved in the calculation of the gravitational attraction between objects with mass. It appears in Newton's law of universal gravitation and in Einstein's theory of general relativity. It is also known as the universal...
, which is measurable in a laboratory only to four significant digits.
- Greater potential for ambiguity: Consider the equation a = 1010 in Planck units. If a represents a length, then the equation means a = . If a represents a mass, then the equation means a = 220 kg. Therefore, if the variable a was not clearly defined, then the equation a = 1010 might be misinterpreted. By contrast, in SISiSi, si, or SI may refer to :- Measurement, mathematics and science :* International System of Units , the modern international standard version of the metric system...
units, the equation would be a = 220 kg, and it would be automatically clear that a represents a mass, not a length or anything else. In fact, natural units are especially useful when this ambiguity is deliberate: For example, in special relativitySpecial relativitySpecial relativity is the physical theory of measurement in an inertial frame of reference proposed in 1905 by Albert Einstein in the paper "On the Electrodynamics of Moving Bodies".It generalizes Galileo's...
space and time are so closely related that it can be useful to not specify whether a variable represents a distance or a time.
Candidate physical constants used in natural unit systems
The candidate physical constants to be normalized are chosen from those in the following table. Note that only a smaller subset of the following can be normalized in any one system of units without contradiction in definition (e.g., me and mp cannot both be defined as the unit mass in a single system).| Constant | Symbol | Dimension | ||||
|---|---|---|---|---|---|---|
| Speed of light in vacuum |  |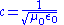
L Length In geometric measurements, length most commonly refers to the longest dimension of an object.In certain contexts, the term "length" is reserved for a certain dimension of an object along which the length is measured. For example it is possible to cut a length of a wire which is shorter than wire... |
T Time Time is a part of the measuring system used to sequence events, to compare the durations of events and the intervals between them, and to quantify rates of change such as the motions of objects.... −1 |
|||
| Magnetic constant |  |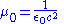
Q Electric charge Electric charge is a physical property of matter that causes it to experience a force when near other electrically charged matter. Electric charge comes in two types, called positive and negative. Two positively charged substances, or objects, experience a mutual repulsive force, as do two... −2 |
M Mass Mass can be defined as a quantitive measure of the resistance an object has to change in its velocity.In physics, mass commonly refers to any of the following three properties of matter, which have been shown experimentally to be equivalent:... |
L | ||
| Electric constant Electric constant The physical constant ε0, commonly called the vacuum permittivity, permittivity of free space or electric constant is an ideal, physical constant, which is the value of the absolute dielectric permittivity of classical vacuum... |
 |
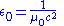Q2 | M−1 | L−3 | T2 | |
| Coulomb constant |  |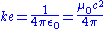
Q−2 | M | L3 | T−2 | |
| Characteristic impedance of free space |  |
Q−2 | M | L2 | T−1 | |
| Gravitational constant Gravitational constant The gravitational constant, denoted G, is an empirical physical constant involved in the calculation of the gravitational attraction between objects with mass. It appears in Newton's law of universal gravitation and in Einstein's theory of general relativity. It is also known as the universal... |
 |
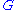M−1 | L3 | T−2 | ||
| Planck constant Planck constant The Planck constant , also called Planck's constant, is a physical constant reflecting the sizes of energy quanta in quantum mechanics. It is named after Max Planck, one of the founders of quantum theory, who discovered it in 1899... (reduced) |
 |
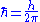M | L2 | T−1 | ||
| Boltzmann constant |  |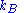
M | L2 | T−2 | Θ Temperature Temperature is a physical property of matter that quantitatively expresses the common notions of hot and cold. Objects of low temperature are cold, while various degrees of higher temperatures are referred to as warm or hot... −1 |
|
| Elementary charge Elementary charge The elementary charge, usually denoted as e, is the electric charge carried by a single proton, or equivalently, the absolute value of the electric charge carried by a single electron. This elementary charge is a fundamental physical constant. To avoid confusion over its sign, e is sometimes called... |
 |
Q | ||||
| Electron mass |  |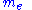
M | ||||
| Proton mass |  |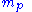
M |
Judiciously choosing units can only normalize physical constants that have dimension. Dimensionless physical constant
Dimensionless physical constant
In physics, a dimensionless physical constant is a universal physical constant that is dimensionless - having no unit attached, so its numerical value is the same under all possible systems of units...
s cannot take on a different numerical value no matter what system of units is used. One such constant important to physics is the fine-structure constant
Fine-structure constant
In physics, the fine-structure constant is a fundamental physical constant, namely the coupling constant characterizing the strength of the electromagnetic interaction. Being a dimensionless quantity, it has constant numerical value in all systems of units...
, α:
Since α is a fixed dimensionless number not equal to 1, it is not possible to define a system of natural units that will normalize all of the physical constants that comprise α. Any three of the four constants: c, ℏ, e, or 4πε0, can be normalized (leaving the remaining physical constant to take on a value that is a simple function of α, attesting to the fundamental nature of the fine-structure constant) but not all four.
Planck units
| Quantity | Expression | Metric value |
|---|---|---|
| Length Length In geometric measurements, length most commonly refers to the longest dimension of an object.In certain contexts, the term "length" is reserved for a certain dimension of an object along which the length is measured. For example it is possible to cut a length of a wire which is shorter than wire... (L) |
 |
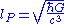1.616252×10−35 m |
| Mass Mass Mass can be defined as a quantitive measure of the resistance an object has to change in its velocity.In physics, mass commonly refers to any of the following three properties of matter, which have been shown experimentally to be equivalent:... (M) |
 |
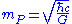2.17644(11)×10−8 kg |
| Time Time Time is a part of the measuring system used to sequence events, to compare the durations of events and the intervals between them, and to quantify rates of change such as the motions of objects.... (T) |
 |
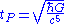5.39124 ×10−44 s |
| Electric charge Electric charge Electric charge is a physical property of matter that causes it to experience a force when near other electrically charged matter. Electric charge comes in two types, called positive and negative. Two positively charged substances, or objects, experience a mutual repulsive force, as do two... (Q) |
 |
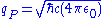1.87554573×10−18 C |
| Temperature Temperature Temperature is a physical property of matter that quantitatively expresses the common notions of hot and cold. Objects of low temperature are cold, while various degrees of higher temperatures are referred to as warm or hot... (Θ) |
 |
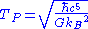1.416785×1032 K |
Planck units are unique among systems of natural units, because they are not defined in terms of properties of any prototype, physical object, or even elementary particle
Elementary particle
In particle physics, an elementary particle or fundamental particle is a particle not known to have substructure; that is, it is not known to be made up of smaller particles. If an elementary particle truly has no substructure, then it is one of the basic building blocks of the universe from which...
. They only refer to the basic structure of the laws of physics: c and G are part of the structure of spacetime
Spacetime
In physics, spacetime is any mathematical model that combines space and time into a single continuum. Spacetime is usually interpreted with space as being three-dimensional and time playing the role of a fourth dimension that is of a different sort from the spatial dimensions...
in general relativity
General relativity
General relativity or the general theory of relativity is the geometric theory of gravitation published by Albert Einstein in 1916. It is the current description of gravitation in modern physics...
, and ℏ captures the relationship between energy and frequency which is at the foundation of quantum mechanics
Quantum mechanics
Quantum mechanics, also known as quantum physics or quantum theory, is a branch of physics providing a mathematical description of much of the dual particle-like and wave-like behavior and interactions of energy and matter. It departs from classical mechanics primarily at the atomic and subatomic...
. This makes Planck units particularly useful and common in theories of quantum gravity
Quantum gravity
Quantum gravity is the field of theoretical physics which attempts to develop scientific models that unify quantum mechanics with general relativity...
, including string theory
String theory
String theory is an active research framework in particle physics that attempts to reconcile quantum mechanics and general relativity. It is a contender for a theory of everything , a manner of describing the known fundamental forces and matter in a mathematically complete system...
.
Some may consider Planck units to be "more natural" even than other natural unit systems discussed below. For example, some other systems use the mass of an electron as a parameter to be normalized. But the electron is just one of 15 known massive elementary particle
Elementary particle
In particle physics, an elementary particle or fundamental particle is a particle not known to have substructure; that is, it is not known to be made up of smaller particles. If an elementary particle truly has no substructure, then it is one of the basic building blocks of the universe from which...
s, all with different masses, and there is no compelling reason, within fundamental physics, to emphasize the electron mass over some other elementary particle's mass.
"Natural units" (particle physics)
| Unit | Metric value | Derivation |
|---|---|---|
| 1 eV−1 of length | 1.97×10−7 m |  |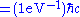
| 1 eV of mass | 1.78×10−36 kg |  |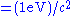
| 1 eV−1 of time | 6.58×10−16 s |  |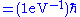
| 1 unit of electric charge (for rationalized) |
5.29×10−19 C |  |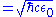
| 1 eV of temperature | 1.16×104 K |  |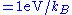
In particle physics
Particle physics
Particle physics is a branch of physics that studies the existence and interactions of particles that are the constituents of what is usually referred to as matter or radiation. In current understanding, particles are excitations of quantum fields and interact following their dynamics...
, the phrase "natural units" generally means:
This is yet not enough information to define a unit system. Next, this equation is almost always supplemented with a definition of charge, from one of two possibilities:
- Rationalized (Heaviside–Lorentz units):
-
- Not rationalized (Gaussian unitsGaussian unitsGaussian units comprise a metric system of physical units. This system is the most common of the several electromagnetic unit systems based on cgs units. It is also called the Gaussian unit system, Gaussian-cgs units, or often just cgs units...
):
- Not rationalized (Gaussian units
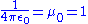
In Heaviside–Lorentz units, there are factors of
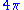in Coulomb's lawCoulomb's law
Coulomb's law or Coulomb's inverse-square law, is a law of physics describing the electrostatic interaction between electrically charged particles. It was first published in 1785 by French physicist Charles Augustin de Coulomb and was essential to the development of the theory of electromagnetism...
and the Biot–Savart law but not in Maxwell's equations
Maxwell's equations
Maxwell's equations are a set of partial differential equations that, together with the Lorentz force law, form the foundation of classical electrodynamics, classical optics, and electric circuits. These fields in turn underlie modern electrical and communications technologies.Maxwell's equations...
; In Gaussian units, it is the reverse. Both systems are used, although Heaviside-Lorentz is more common. The value of the elementary charge
Elementary charge
The elementary charge, usually denoted as e, is the electric charge carried by a single proton, or equivalently, the absolute value of the electric charge carried by a single electron. This elementary charge is a fundamental physical constant. To avoid confusion over its sign, e is sometimes called...
in the two systems is:
(Gaussian).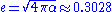 (Lorentz–Heaviside).Finally, one more unit is needed. Most commonly, electron-volt (eV) is used, despite the fact that this is not a "natural" unit in the sense discussed above. (The SI prefix
SI prefix
The International System of Units specifies a set of unit prefixes known as SI prefixes or metric prefixes. An SI prefix is a name that precedes a basic unit of measure to indicate a decadic multiple or fraction of the unit. Each prefix has a unique symbol that is prepended to the unit symbol...
ed multiples of eV are used as well: keV, MeV, GeV, etc.)
With the addition of eV (or any other auxiliary unit), any quantity can be expressed. For example, a distance of 1 cm can be expressed in terms of eV, in natural units, as:
Stoney units
| Quantity | Expression | Metric value |
|---|---|---|
| Length Length In geometric measurements, length most commonly refers to the longest dimension of an object.In certain contexts, the term "length" is reserved for a certain dimension of an object along which the length is measured. For example it is possible to cut a length of a wire which is shorter than wire... (L) |
 |
1.38068×10−36 m |
| Mass Mass Mass can be defined as a quantitive measure of the resistance an object has to change in its velocity.In physics, mass commonly refers to any of the following three properties of matter, which have been shown experimentally to be equivalent:... (M) |
 |
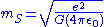1.85921×10−9 kg |
| Time Time Time is a part of the measuring system used to sequence events, to compare the durations of events and the intervals between them, and to quantify rates of change such as the motions of objects.... (T) |
 |
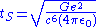4.60544×10−45 s |
| Electric charge Electric charge Electric charge is a physical property of matter that causes it to experience a force when near other electrically charged matter. Electric charge comes in two types, called positive and negative. Two positively charged substances, or objects, experience a mutual repulsive force, as do two... (Q) |
 |
1.60218×10−19 C |
| Temperature Temperature Temperature is a physical property of matter that quantitatively expresses the common notions of hot and cold. Objects of low temperature are cold, while various degrees of higher temperatures are referred to as warm or hot... (Θ) |
 |
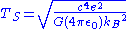1.21028×1031 K |
Stoney units are defined by:
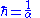
where α is the fine-structure constant
Fine-structure constant
In physics, the fine-structure constant is a fundamental physical constant, namely the coupling constant characterizing the strength of the electromagnetic interaction. Being a dimensionless quantity, it has constant numerical value in all systems of units...
.
George Johnstone Stoney
George Johnstone Stoney
George Johnstone Stoney was an Irish physicist most famous for introducing the term electron as the "fundamental unit quantity of electricity"....
was the first physicist to introduce the concept of natural units. He presented the idea in a lecture entitled "On the Physical Units of Nature" delivered to the British Association in 1874. Stoney units differ from Planck units by fixing the elementary charge
Elementary charge
The elementary charge, usually denoted as e, is the electric charge carried by a single proton, or equivalently, the absolute value of the electric charge carried by a single electron. This elementary charge is a fundamental physical constant. To avoid confusion over its sign, e is sometimes called...
at 1, instead of Planck's constant (only discovered after Stoney's proposal).
Stoney units are rarely used in modern physics for calculations, but they are of historical interest.
Atomic units
| Quantity | Expression (Hartree atomic units) |
Metric value (Hartree atomic units) |
|---|---|---|
| Length Length In geometric measurements, length most commonly refers to the longest dimension of an object.In certain contexts, the term "length" is reserved for a certain dimension of an object along which the length is measured. For example it is possible to cut a length of a wire which is shorter than wire... (L) |
 |
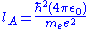5.29177×10−11 m |
| Mass Mass Mass can be defined as a quantitive measure of the resistance an object has to change in its velocity.In physics, mass commonly refers to any of the following three properties of matter, which have been shown experimentally to be equivalent:... (M) |
 |
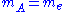9.10938×10−31 kg |
| Time Time Time is a part of the measuring system used to sequence events, to compare the durations of events and the intervals between them, and to quantify rates of change such as the motions of objects.... (T) |
 |
2.41889×10−17 s |
| Electric charge Electric charge Electric charge is a physical property of matter that causes it to experience a force when near other electrically charged matter. Electric charge comes in two types, called positive and negative. Two positively charged substances, or objects, experience a mutual repulsive force, as do two... (Q) |
 |
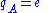1.60218×10−19 C |
| Temperature Temperature Temperature is a physical property of matter that quantitatively expresses the common notions of hot and cold. Objects of low temperature are cold, while various degrees of higher temperatures are referred to as warm or hot... (Θ) |
 |
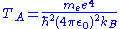3.15774×105 K |
There are two types of atomic units, closely related:
Hartree atomic units:
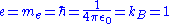
Rydberg atomic units:
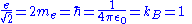
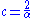
These units are designed to simplify atomic and molecular physics and chemistry, especially the hydrogen atom
Hydrogen atom
A hydrogen atom is an atom of the chemical element hydrogen. The electrically neutral atom contains a single positively-charged proton and a single negatively-charged electron bound to the nucleus by the Coulomb force...
, and are widely used in these fields. The Hartree units were first proposed by Douglas Hartree
Douglas Hartree
Douglas Rayner Hartree PhD, FRS was an English mathematician and physicist most famous for the development of numerical analysis and its application to the Hartree-Fock equations of atomic physics and the construction of the meccano differential analyser.-Early life:Douglas Hartree was born in...
, and are more common than the Rydberg units.
The units are designed especially to characterize the behavior of an electron in the ground state of a hydrogen atom. For example, using the Hartree convention, in the Bohr model
Bohr model
In atomic physics, the Bohr model, introduced by Niels Bohr in 1913, depicts the atom as a small, positively charged nucleus surrounded by electrons that travel in circular orbits around the nucleus—similar in structure to the solar system, but with electrostatic forces providing attraction,...
of the hydrogen atom, an electron in the ground state has orbital velocity = 1, orbital radius = 1, angular momentum = 1, ionization energy
Ionization energy
The ionization energy of a chemical species, i.e. an atom or molecule, is the energy required to remove an electron from the species to a practically infinite distance. Large atoms or molecules have a low ionization energy, while small molecules tend to have higher ionization energies.The property...
= ½, etc.
The unit of energy
Energy
In physics, energy is an indirectly observed quantity. It is often understood as the ability a physical system has to do work on other physical systems...
is called the Hartree energy
Hartree energy
The hartree , also known as the Hartree energy, is the atomic unit of energy, named after the British physicist Douglas Hartree. It is defined as...
in the Hartree system and the Rydberg energy in the Rydberg system. They differ by a factor of 2. The speed of light
Speed of light
The speed of light in vacuum, usually denoted by c, is a physical constant important in many areas of physics. Its value is 299,792,458 metres per second, a figure that is exact since the length of the metre is defined from this constant and the international standard for time...
is relatively large in atomic units (137 in Hartree or 274 in Rydberg), which comes from the fact that an electron in hydrogen tends to move much slower than the speed of light. The gravitational constant
Gravitational constant
The gravitational constant, denoted G, is an empirical physical constant involved in the calculation of the gravitational attraction between objects with mass. It appears in Newton's law of universal gravitation and in Einstein's theory of general relativity. It is also known as the universal...
is extremely small in atomic units (around 10−45), which comes from the fact that the gravitational force between two electrons is far weaker than the Coulomb force. The unit length, mA, is the Bohr radius
Bohr radius
The Bohr radius is a physical constant, approximately equal to the most probable distance between the proton and electron in a hydrogen atom in its ground state. It is named after Niels Bohr, due to its role in the Bohr model of an atom...
, a0.
Quantum chromodynamics (QCD) system of units
| Quantity | Expression | Metric value |
|---|---|---|
| Length Length In geometric measurements, length most commonly refers to the longest dimension of an object.In certain contexts, the term "length" is reserved for a certain dimension of an object along which the length is measured. For example it is possible to cut a length of a wire which is shorter than wire... (L) |
 |
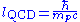2.10308885 × 10−16 m |
| Mass Mass Mass can be defined as a quantitive measure of the resistance an object has to change in its velocity.In physics, mass commonly refers to any of the following three properties of matter, which have been shown experimentally to be equivalent:... (M) |
 |
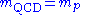1.67262158 × 10−27 kg |
| Time Time Time is a part of the measuring system used to sequence events, to compare the durations of events and the intervals between them, and to quantify rates of change such as the motions of objects.... (T) |
 |
7.0151493 × 10−25 s |
| Electric charge Electric charge Electric charge is a physical property of matter that causes it to experience a force when near other electrically charged matter. Electric charge comes in two types, called positive and negative. Two positively charged substances, or objects, experience a mutual repulsive force, as do two... (Q) |
 |
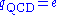1.60217646 × 10−19 C |
| Temperature Temperature Temperature is a physical property of matter that quantitatively expresses the common notions of hot and cold. Objects of low temperature are cold, while various degrees of higher temperatures are referred to as warm or hot... (Θ) |
 |
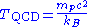1.0888183 × 1013 K |
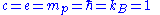
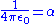
The electron mass is replaced with that of the proton
Proton
The proton is a subatomic particle with the symbol or and a positive electric charge of 1 elementary charge. One or more protons are present in the nucleus of each atom, along with neutrons. The number of protons in each atom is its atomic number....
and the permittivity of free space is not fixed by definition. Strong units are convenient for work in QCD
Quantum chromodynamics
In theoretical physics, quantum chromodynamics is a theory of the strong interaction , a fundamental force describing the interactions of the quarks and gluons making up hadrons . It is the study of the SU Yang–Mills theory of color-charged fermions...
and nuclear physics, where quantum mechanics and relativity are omnipresent and the proton is an object of central interest.
Geometrized units
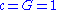
The geometrized unit system is not a completely defined or unique system. In this system, the base physical units are chosen so that the speed of light
Speed of light
The speed of light in vacuum, usually denoted by c, is a physical constant important in many areas of physics. Its value is 299,792,458 metres per second, a figure that is exact since the length of the metre is defined from this constant and the international standard for time...
and the gravitational constant
Gravitational constant
The gravitational constant, denoted G, is an empirical physical constant involved in the calculation of the gravitational attraction between objects with mass. It appears in Newton's law of universal gravitation and in Einstein's theory of general relativity. It is also known as the universal...
are set equal to unity, leaving latitude to also set some other constant such as the Boltzmann constant and Coulomb force constant
Coulomb's law
Coulomb's law or Coulomb's inverse-square law, is a law of physics describing the electrostatic interaction between electrically charged particles. It was first published in 1785 by French physicist Charles Augustin de Coulomb and was essential to the development of the theory of electromagnetism...
equal to unity:
If the reduced Planck constant is also set equal to unity,
then geometrized units are identical to Planck units
Planck units
In physics, Planck units are physical units of measurement defined exclusively in terms of five universal physical constants listed below, in such a manner that these five physical constants take on the numerical value of 1 when expressed in terms of these units. Planck units elegantly simplify...
.
Summary table
| Quantity / Symbol | Planck | Stoney | Hartree | Rydberg | Heaviside–Lorentz | Gauss | QCD |
|---|---|---|---|---|---|---|---|
Speed of light in vacuum  |
 |
 |
 |
 |
 |
 |
 |
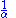| Electric constant Electric constant The physical constant ε0, commonly called the vacuum permittivity, permittivity of free space or electric constant is an ideal, physical constant, which is the value of the absolute dielectric permittivity of classical vacuum... (vacuum permittivity)  |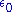
 |
 |
 |
 |
 |
 |
 |
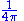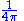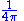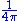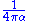Magnetic constant (vacuum permeability)  |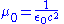
 |
 |
 |
 |
 |
 |
 |
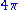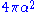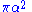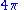Characteristic impedance of vacuum  |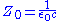
 |
 |
 |
 |
 |
 |
 |
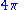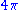Planck's constant (reduced)  |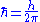
 |
 |
 |
 |
 |
 |
 |
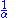| Elementary charge Elementary charge The elementary charge, usually denoted as e, is the electric charge carried by a single proton, or equivalently, the absolute value of the electric charge carried by a single electron. This elementary charge is a fundamental physical constant. To avoid confusion over its sign, e is sometimes called...  |
 |
 |
 |
 |
 |
 |
 |
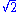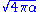Josephson constant  |
 |
 |
 |
 |
 |
 |
 |
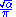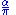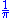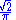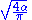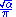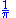von Klitzing constant  |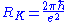
 |
 |
 |
 |
 |
 |
 |
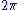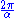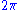| Gravitational constant Gravitational constant The gravitational constant, denoted G, is an empirical physical constant involved in the calculation of the gravitational attraction between objects with mass. It appears in Newton's law of universal gravitation and in Einstein's theory of general relativity. It is also known as the universal...  |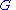
 |
 |
 |
 |
 |
 |
 |
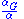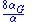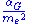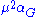Boltzmann constant  |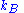
 |
 |
 |
 |
 |
 |
 |
Electron mass  |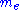
 |
 |
 |
 |
 |
 |
 |
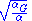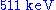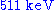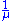where:
- α is the fine-structure constantFine-structure constantIn physics, the fine-structure constant is a fundamental physical constant, namely the coupling constant characterizing the strength of the electromagnetic interaction. Being a dimensionless quantity, it has constant numerical value in all systems of units...
, 7.2973525376 = (137.035999679)−1, - αG is the gravitational coupling constantGravitational coupling constantIn physics, the gravitational coupling constant, αG, is the coupling constant characterizing the gravitational attraction between two charged elementary particles having nonzero mass...
,
, - μ is the proton-to-electron mass ratioProton-to-electron mass ratioIn physics, the proton-to-electron mass ratio, μ or β, is simply the rest mass of the proton divided by that of the electron...
, about 1836.15267247.
See also
- Anthropic unitsAnthropic unitsAnthropic units are units of measurement which explicitly arose from human physiology or behavior. Some were derived directly from the dimensions of the human body, and as such, are commonly referred to as anthropomorphic...
- Dimensional analysisDimensional analysisIn physics and all science, dimensional analysis is a tool to find or check relations among physical quantities by using their dimensions. The dimension of a physical quantity is the combination of the basic physical dimensions which describe it; for example, speed has the dimension length per...
- Fundamental unitFundamental unitA set of fundamental units is a set of units for physical quantities from which every other unit can be generated.In the language of measurement, quantities are quantifiable aspects of the world, such as time, distance, velocity, mass, momentum, energy, and weight, and units are used to describe...
- N-body unitsN-body unitsN-body units are a completely self-contained system of units used for N-body simulations of self gravitating systems in astrophysics. In this system, the base physical units are chosen so that the total mass, M, the gravitational constant, G, and the virial radius, R, are normalised...
- Physical constantPhysical constantA physical constant is a physical quantity that is generally believed to be both universal in nature and constant in time. It can be contrasted with a mathematical constant, which is a fixed numerical value but does not directly involve any physical measurement.There are many physical constants in...
- Units of measurementUnits of measurementA unit of measurement is a definite magnitude of a physical quantity, defined and adopted by convention and/or by law, that is used as a standard for measurement of the same physical quantity. Any other value of the physical quantity can be expressed as a simple multiple of the unit of...
External links
- The NIST website (National Institute of Standards and Technology) is a convenient source of data on the commonly recognized constants.
- K.A. Tomilin: NATURAL SYSTEMS OF UNITS; To the Centenary Anniversary of the Planck System A comparative overview/tutorial of various systems of natural units having historical use.
- Pedagogic Aides to Quantum Field Theory Click on the link for Chap. 2 to find an extensive, simplified introduction to natural units.