Orbit portrait
Encyclopedia
In mathematics
, an orbit portrait is a combinatorial tool used in complex dynamics for understanding the behavior of one-complex dimensional quadratic maps
.
In simple words one can say that it is :
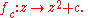
from the complex plane
to itself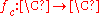
and a repelling or parabolic
periodic orbit
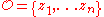of 
, so that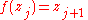 
(where subscripts are taken 1 + modulo 
), let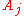 
be the set of angles
whose corresponding external ray
s land at
.
Then the set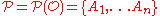
is called the orbit portrait of the periodic orbit 
.
All of the sets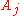
must have the same number of elements, which is called the valence of the portrait.
for complex quadratic polynomial
with c= -0.03111+0.79111*i portrait of parabolic period 3 orbit is :
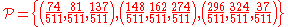
Valence = 3 rays per orbit point.
Rays for above angles land on points of that orbit . Parameter c is a center of period 9 hyperbolic component of Mandelbrot set.
has the following properties:
of subsets of the circle which satisfy these four properties above is called a formal orbit portrait. It is a theorem of John Milnor
that every formal orbit portrait is realized by the actual orbit portrait of a periodic orbit of some quadratic one-complex-dimensional map. Orbit portraits contain dynamical information about how external rays and their landing points map in the plane, but formal orbit portraits are no more than combinatorial objects. Milnor's theorem states that, in truth, there is no distinction between the two.
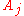have only a single element are called trivial, except for orbit portrait 
. An alternative definition is that an orbit portrait is nontrivial if it is maximal, which in this case means that there is no orbit portrait that strictly contains it (i.e. there does not exist an orbit portrait 
such that 
). It is easy to see that every trivial formal orbit portrait is realized as the orbit portrait of some orbit of the map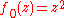 
, since every external ray of this map lands, and they all land at distinct points of the Julia Set
. Trivial orbit portraits are pathological in some respects, and in the sequel we will refer only to nontrivial orbit portraits.
, each 
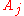is a finite subset of the circle 
, so each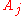 
divides the circle into a number of disjoint intervals, called complementary arcs based at the point 
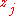. The length of each interval is referred to as its angular width.
Each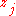
has a unique largest arc based at it, which is called its critical arc. The critical arc always has length greater than 
These arcs have the property that every arc based at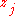
, except for the critical arc, maps diffeomorphically to an arc based 
, and the critical arc covers every arc based at 
once, except for a single arc, which it covers twice. The arc that it covers twice is called the critical value arc for 
. This is not necessarily distinct from the critical arc.
When
escapes to infinity under iteration of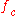 
, or when 
is in the Julia set, then 
has a well-defined external angle. Call this angle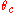 
. 
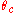is in every critical value arc. Also, the two inverse images of 
under the doubling map (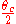
and 
) are both in every critical arc.
Among all of the critical value arcs for all of the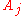
's, there is a unique smallest critical value arc 
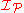, called the characteristic arc which is strictly contained within every other critical value arc. The characteristic arc is a complete invariant of an orbit portrait, in the sense that two orbit portraits are identical if and only if they have the same characteristic arc.
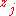of the orbit, the external ray
s landing at
divide the plane into 
open sets called sectors based at 
. Sectors are naturally identified the complementary arcs based at the same point. The angular width of a sector is defined as the length of its corresponding complementary arc. Sectors are called critical sectors or critical value sectors when the corresponding arcs are, respectively, critical arcs and critical value arcs.
Sectors also have the interesting property that
is in the critical sector of every point, and 
, the critical value of 
, is in the critical value sector.
with angles
and 
land at the same point of the Mandelbrot Set
in parameter space if and only if there exists an orbit portrait
with the interval 
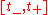as its characteristic arc. For any orbit portrait 
let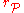 
be the common landing point of the two external angles in parameter space corresponding to the characteristic arc of 
. These two parameter rays, along with their common landing point, split the parameter space into two open components. Let the component that does not contain the point 
be called the 
-wake and denoted as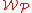 
. A quadratic polynomial
realizes the orbit portrait 
with a repelling orbit exactly when 
. 
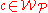is realized with a parabolic orbit only for the single value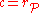 
for about
is the valence of an orbit portrait 
and 
is the recurrent ray period, then these two types may be characterized as follows:
Mathematics
Mathematics is the study of quantity, space, structure, and change. Mathematicians seek out patterns and formulate new conjectures. Mathematicians resolve the truth or falsity of conjectures by mathematical proofs, which are arguments sufficient to convince other mathematicians of their validity...
, an orbit portrait is a combinatorial tool used in complex dynamics for understanding the behavior of one-complex dimensional quadratic maps
Complex quadratic polynomial
A complex quadratic polynomial is a quadratic polynomial whose coefficients are complex numbers.-Forms:When the quadratic polynomial has only one variable , one can distinguish its 4 main forms:...
.
In simple words one can say that it is :
- a list of external angles for which rays land on points of that orbit
- graph showing above list
Definition
Given a quadratic mapComplex quadratic polynomial
A complex quadratic polynomial is a quadratic polynomial whose coefficients are complex numbers.-Forms:When the quadratic polynomial has only one variable , one can distinguish its 4 main forms:...
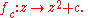
from the complex plane
Complex plane
In mathematics, the complex plane or z-plane is a geometric representation of the complex numbers established by the real axis and the orthogonal imaginary axis...
to itself
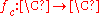
and a repelling or parabolic
Periodic points of complex quadratic mappings
This article describes periodic points of some complex quadratic maps. A map is a formula for computing a value of a variable based on its own previous value or values; a quadratic map is one that involves the previous value raised to the powers one and two; and a complex map is one in which the...
periodic orbit
Orbit (dynamics)
In mathematics, in the study of dynamical systems, an orbit is a collection of points related by the evolution function of the dynamical system. The orbit is a subset of the phase space and the set of all orbits is a partition of the phase space, that is different orbits do not intersect in the...
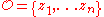of , so that 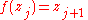(where subscripts are taken 1 + modulo ), let 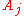be the set of anglesExternal ray
An external ray is a curve that runs from infinity toward a Julia or Mandelbrot set.This curve is only sometimes a half-line but is called ray because it is image of ray....
whose corresponding external ray
External ray
An external ray is a curve that runs from infinity toward a Julia or Mandelbrot set.This curve is only sometimes a half-line but is called ray because it is image of ray....
s land at
.Then the set
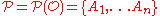is called the orbit portrait of the periodic orbit .All of the sets
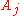must have the same number of elements, which is called the valence of the portrait.Examples
- Parabolic orbit portrait
for complex quadratic polynomial
Complex quadratic polynomial
A complex quadratic polynomial is a quadratic polynomial whose coefficients are complex numbers.-Forms:When the quadratic polynomial has only one variable , one can distinguish its 4 main forms:...
with c= -0.03111+0.79111*i portrait of parabolic period 3 orbit is :
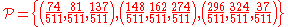
Valence = 3 rays per orbit point.
Rays for above angles land on points of that orbit . Parameter c is a center of period 9 hyperbolic component of Mandelbrot set.
Properties
Every orbit portrait has the following properties:- Each
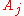is a finite subset of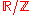 
- The doubling map on the circle gives a bijection from
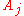to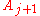 
and preserves cyclic order of the angles.
- All of the angles in all of the sets
are periodic under the doubling map of the circle, and all of the angles have the same exact period. This period must be a multiple of 
, so the period is of the form 
, where 
is called the recurrent ray period.
- The sets
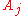are pairwise unlinked, which is to say that given any pair of them, there are two disjoint intervals of 
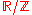where each interval contains one of the sets.
Formal orbit portraits
Any collection of subsets of the circle which satisfy these four properties above is called a formal orbit portrait. It is a theorem of John MilnorJohn Milnor
John Willard Milnor is an American mathematician known for his work in differential topology, K-theory and dynamical systems. He won the Fields Medal in 1962, the Wolf Prize in 1989, and the Abel Prize in 2011. Milnor is a distinguished professor at Stony Brook University...
that every formal orbit portrait is realized by the actual orbit portrait of a periodic orbit of some quadratic one-complex-dimensional map. Orbit portraits contain dynamical information about how external rays and their landing points map in the plane, but formal orbit portraits are no more than combinatorial objects. Milnor's theorem states that, in truth, there is no distinction between the two.
Trivial orbit portraits
Orbit portrait where all of the sets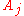 have only a single element are called trivial, except for orbit portrait . An alternative definition is that an orbit portrait is nontrivial if it is maximal, which in this case means that there is no orbit portrait that strictly contains it (i.e. there does not exist an orbit portrait such that ). It is easy to see that every trivial formal orbit portrait is realized as the orbit portrait of some orbit of the map 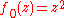, since every external ray of this map lands, and they all land at distinct points of the Julia SetJulia set
In the context of complex dynamics, a topic of mathematics, the Julia set and the Fatou set are two complementary sets defined from a function...
. Trivial orbit portraits are pathological in some respects, and in the sequel we will refer only to nontrivial orbit portraits.
Arcs
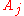In an orbit portrait, each is a finite subset of the circle , so each 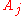divides the circle into a number of disjoint intervals, called complementary arcs based at the point 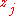. The length of each interval is referred to as its angular width.Each
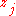has a unique largest arc based at it, which is called its critical arc. The critical arc always has length greater than These arcs have the property that every arc based at
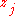, except for the critical arc, maps diffeomorphically to an arc based , and the critical arc covers every arc based at once, except for a single arc, which it covers twice. The arc that it covers twice is called the critical value arc for . This is not necessarily distinct from the critical arc.When
escapes to infinity under iteration of 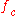, or when is in the Julia set, then has a well-defined external angle. Call this angle 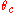. 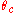is in every critical value arc. Also, the two inverse images of under the doubling map (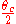 and ) are both in every critical arc.Among all of the critical value arcs for all of the
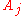's, there is a unique smallest critical value arc 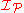, called the characteristic arc which is strictly contained within every other critical value arc. The characteristic arc is a complete invariant of an orbit portrait, in the sense that two orbit portraits are identical if and only if they have the same characteristic arc.Sectors
Much as the rays landing on the orbit divide up the circle, they divide up the complex plane. For every point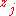 of the orbit, the external rayExternal ray
An external ray is a curve that runs from infinity toward a Julia or Mandelbrot set.This curve is only sometimes a half-line but is called ray because it is image of ray....
s landing at
divide the plane into open sets called sectors based at . Sectors are naturally identified the complementary arcs based at the same point. The angular width of a sector is defined as the length of its corresponding complementary arc. Sectors are called critical sectors or critical value sectors when the corresponding arcs are, respectively, critical arcs and critical value arcs.Sectors also have the interesting property that
is in the critical sector of every point, and , the critical value of , is in the critical value sector.Parameter wakes
Two parameter raysExternal ray
An external ray is a curve that runs from infinity toward a Julia or Mandelbrot set.This curve is only sometimes a half-line but is called ray because it is image of ray....
with angles
and land at the same point of the Mandelbrot SetMandelbrot set
The Mandelbrot set is a particular mathematical set of points, whose boundary generates a distinctive and easily recognisable two-dimensional fractal shape...
in parameter space if and only if there exists an orbit portrait
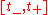with the interval as its characteristic arc. For any orbit portrait let 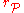be the common landing point of the two external angles in parameter space corresponding to the characteristic arc of . These two parameter rays, along with their common landing point, split the parameter space into two open components. Let the component that does not contain the point be called the -wake and denoted as 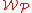. A quadratic polynomialComplex quadratic polynomial
A complex quadratic polynomial is a quadratic polynomial whose coefficients are complex numbers.-Forms:When the quadratic polynomial has only one variable , one can distinguish its 4 main forms:...
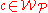realizes the orbit portrait with a repelling orbit exactly when . is realized with a parabolic orbit only for the single value 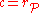for about
Primitive and satellite orbit portraits
Other than the zero portrait, there are two types of orbit portraits: primitive and satellite. If is the valence of an orbit portrait and is the recurrent ray period, then these two types may be characterized as follows:
- Primitive orbit portraits have
and 
. Every ray in the portrait is mapped to itself by 
. Each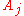 
is a pair of angles, each in a distinct orbit of the doubling map. In this case, 
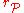is the base point of a baby Mandelbrot set in parameter space. - Satellite orbit portraits have
. In this case, all of the angles make up a single orbit under the doubling map. Additionally,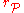 
is the base point of a parabolic bifurcation in parameter space.