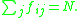Oscillator strength
Encyclopedia
An atom or a molecule can absorb light and undergo a transition from
one quantum state to another. The oscillator strength is a dimensionless
quantity to express the strength of the transition.
The oscillator strength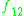 of a transition from a lower state
of a transition from a lower state
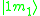 to an upper state
to an upper state 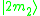 may be defined by
may be defined by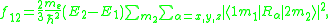
where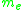 is the mass of an electron and
is the mass of an electron and 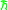 is
is
the reduced Planck constant. The quantum states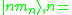 1,2,..., are assumed to have several
1,2,..., are assumed to have several
degenerate sub-states, which are labeled by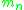 . "Degenerate" means
. "Degenerate" means
that they all have the same energy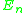 .
.
The operator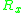 is the sum of the x-coordinates
is the sum of the x-coordinates 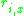
of all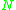 electrons in the system, etc.:
electrons in the system, etc.:
The oscillator strength is the same for each sub-state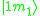 .
.
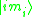 to all
to all
other states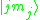 is equal to the number of electrons
is equal to the number of electrons 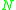 :
: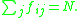
one quantum state to another. The oscillator strength is a dimensionless
quantity to express the strength of the transition.
The oscillator strength
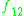 of a transition from a lower state
of a transition from a lower state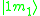 to an upper state
to an upper state 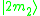 may be defined by
may be defined by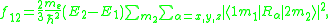
where
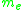 is the mass of an electron and
is the mass of an electron and 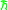 is
isthe reduced Planck constant. The quantum states
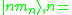 1,2,..., are assumed to have several
1,2,..., are assumed to have severaldegenerate sub-states, which are labeled by
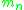 . "Degenerate" means
. "Degenerate" meansthat they all have the same energy
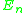 .
.The operator
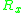 is the sum of the x-coordinates
is the sum of the x-coordinates 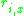
of all
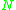 electrons in the system, etc.:
electrons in the system, etc.:
The oscillator strength is the same for each sub-state
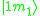 .
.Thomas-Reiche-Kuhn sum rule
The sum of the oscillator strength from one sub-state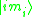 to all
to allother states
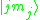 is equal to the number of electrons
is equal to the number of electrons 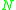 :
: