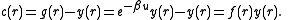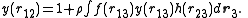Percus–Yevick approximation
Encyclopedia
In statistical mechanics
the Percus–Yevick approximation is a closure
relation to solve the Ornstein–Zernike equation
. It is also referred to as the Percus–Yevick equation. It is commonly used in fluid theory to obtain e.g. expressions for the radial distribution function
.
where is the radial distribution function
is the radial distribution function
, i.e.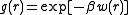 (with w(r) the potential of mean force
(with w(r) the potential of mean force
) and is the radial distribution function without the direct interaction between pairs
is the radial distribution function without the direct interaction between pairs 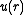 included; i.e. we write
included; i.e. we write 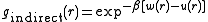 . Thus we approximate c(r) by
. Thus we approximate c(r) by
If we introduce the function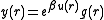 into the approximation for c(r) one obtains
into the approximation for c(r) one obtains
This is the essence of the Percus–Yevick approximation for if we substitute this result in the Ornstein–Zernike equation
, one obtains the Percus–Yevick equation:
The approximation was defined by Percus and Yevick in 1958.
Statistical mechanics
Statistical mechanics or statistical thermodynamicsThe terms statistical mechanics and statistical thermodynamics are used interchangeably...
the Percus–Yevick approximation is a closure
Closure (mathematics)
In mathematics, a set is said to be closed under some operation if performance of that operation on members of the set always produces a unique member of the same set. For example, the real numbers are closed under subtraction, but the natural numbers are not: 3 and 8 are both natural numbers, but...
relation to solve the Ornstein–Zernike equation
Ornstein–Zernike equation
In statistical mechanics the Ornstein–Zernike equation is an integral equation for defining the direct correlation function. It basically describes how the correlation between two molecules can be calculated...
. It is also referred to as the Percus–Yevick equation. It is commonly used in fluid theory to obtain e.g. expressions for the radial distribution function
Radial distribution function
In statistical mechanics, a radial distribution function , g, describes how the atomic density varies as a function of the distance from one particular atom....
.
Derivation
The direct correlation function represents the direct correlation between two particles in a system containing N − 2 other particles. It can be represented bywhere
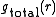 is the radial distribution function
is the radial distribution functionRadial distribution function
In statistical mechanics, a radial distribution function , g, describes how the atomic density varies as a function of the distance from one particular atom....
, i.e.
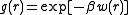 (with w(r) the potential of mean force
(with w(r) the potential of mean forcePotential of mean force
The Potential of Mean Force of a system with N molecules is strictly the potential that gives the average force over all the configurations of all the n+1...N molecules acting on a particle at any fixed configuration keeping fixed a set of molecules 1...n...
) and
 is the radial distribution function without the direct interaction between pairs
is the radial distribution function without the direct interaction between pairs 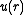 included; i.e. we write
included; i.e. we write 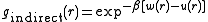 . Thus we approximate c(r) by
. Thus we approximate c(r) byIf we introduce the function
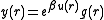 into the approximation for c(r) one obtains
into the approximation for c(r) one obtainsThis is the essence of the Percus–Yevick approximation for if we substitute this result in the Ornstein–Zernike equation
Ornstein–Zernike equation
In statistical mechanics the Ornstein–Zernike equation is an integral equation for defining the direct correlation function. It basically describes how the correlation between two molecules can be calculated...
, one obtains the Percus–Yevick equation:
The approximation was defined by Percus and Yevick in 1958.