
Quadrifolium
Encyclopedia
The quadrifolium
is a type of rose curve
with n=2. It has polar equation:

with corresponding algebraic equation
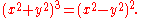
Rotated by 45°, this becomes

with corresponding algebraic equation
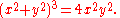
In either form, it is a plane algebraic curve
of genus
zero.
The dual curve
to the quadrifolium is
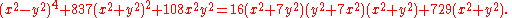
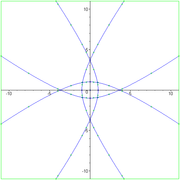 The area inside the curve is
The area inside the curve is  , which is exactly half of the area of the circumcircle of the quadrifolium. The length of the curve is ca. 9.6884.
, which is exactly half of the area of the circumcircle of the quadrifolium. The length of the curve is ca. 9.6884.
is a type of rose curve
Rose (mathematics)
In mathematics, a rose or rhodonea curve is a sinusoid plotted in polar coordinates. Up to similarity, thesecurves can all be expressed by a polar equation of the form\!\,r=\cos.If k is an integer, the curve will be rose shaped with...
with n=2. It has polar equation:

with corresponding algebraic equation
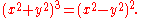
Rotated by 45°, this becomes

with corresponding algebraic equation
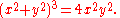
In either form, it is a plane algebraic curve
Algebraic curve
In algebraic geometry, an algebraic curve is an algebraic variety of dimension one. The theory of these curves in general was quite fully developed in the nineteenth century, after many particular examples had been considered, starting with circles and other conic sections.- Plane algebraic curves...
of genus
Geometric genus
In algebraic geometry, the geometric genus is a basic birational invariant pg of algebraic varieties and complex manifolds.-Definition:...
zero.
The dual curve
Dual curve
In projective geometry, a dual curve of a given plane curve C is a curve in the dual projective plane consisting of the set of lines tangent to C. There is a map from a curve to its dual, sending each point to the point dual to its tangent line. If C is algebraic then so is its dual and the degree...
to the quadrifolium is
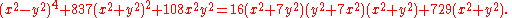

 , which is exactly half of the area of the circumcircle of the quadrifolium. The length of the curve is ca. 9.6884.
, which is exactly half of the area of the circumcircle of the quadrifolium. The length of the curve is ca. 9.6884.
