
Scanning tunneling spectroscopy
Encyclopedia
Scanning Tunneling Spectroscopy (STS) is an extension of Scanning Tunneling Microscopy (STM) which is used to provide information about the density of electrons in a sample as a function of their energy.
In scanning tunneling microscopy, a metal tip is moved over a conducting sample without making mechanical contact with the sample. A "bias voltage" between the sample and tip allows a current to flow between the tip and the sample even though they are not in contact. This can occur because of quantum mechanical tunneling, hence the name of the instrument.
The scanning tunneling microscope is used to obtain "topographs" - topographic maps - of surfaces. The tip is rastered across a surface and (in constant current mode), a constant current is maintained between the tip and the sample by adjusting the height of the tip. A plot of the tip height at all measurement positions on the raster provides the topograph. These topographic images can obtain information that is atomically resolved, and many beautiful images of metal and superconductor surfaces have been obtained with atomic precision.
However, the scanning tunneling microscope does NOT measure the height of surface features. This can be shown dramatically when a molecule is adsorbed on a surface - the STM image may appear to have either INCREASED or DECREASED height at that feature, although the geometry alone is certainly an INCREASED height. A detailed analysis of the way in which an image is formed shows that the transmission of the electric current between the tip and the sample depends on two factors: (1) the geometry of the sample and (2) the arrangement of the electrons in the sample. The arrangement of the electrons in the sample is described quantum mechanically by an "electron density". The electron density is a function of both position AND energy, and is formally described as the Local Density Of electron States, which is abbreviated to Local Density Of States (LDOS), which is a function of energy.
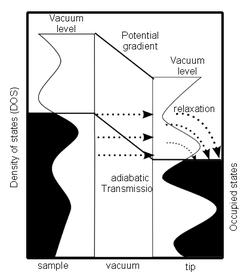
Spectroscopy, in its most general sense, refers to a measurement of the number of something as a function of energy. For scanning tunneling spectroscopy the scanning tunneling microscope is used to measure the number of electrons (the Local Density Of States) as a function of the electron energy. The electron energy is set by the electrical potential difference (voltage) between the sample and the tip. The location (Local) is set by the position of the tip.
At it's simplest, a "scanning tunneling spectrum" is obtained by placing a scanning tunneling microscope tip above a particular place on the sample. WITH THE HEIGHT OF THE TIP FIXED, the electron tunneling current is then measured as a function of electron energy by varying the voltage between the tip and the sample (the tip to sample voltage sets the electron energy). The change of the current with the energy of the electrons is the simplest spectrum that can be obtained, it us often referred to as an I-V curve. As is shown below, it is the SLOPE of the I-V curve at each voltage (often called the dI/dV-curve) which is more fundamental because dI/dV corresponds to the electron density of states at the local position of the tip, the Local Density of States - LDOS.
In what follows, this simple idea is given a more detailed physical analysis, and some more sophisticated measurement ideas are presented.
 Scanning tunneling spectroscopy (STS) is an experimental technique which uses a scanning tunneling microscope
Scanning tunneling spectroscopy (STS) is an experimental technique which uses a scanning tunneling microscope
(STM) to probe the local density of electronic states (LDOS) and the band gap
of surfaces and materials on surfaces at the atom
ic scale. Generally, STS involves observation of changes in constant-current topographs with tip-sample bias, local measurement of the tunneling current versus tip-sample bias (I-V) curve, measurement of the tunneling conductance, , or more than one of these. Since the tunneling current in a scanning tunneling microscope only flows in a region with diameter ~5 Å, STS is unusual in comparison with other surface spectroscopy
, or more than one of these. Since the tunneling current in a scanning tunneling microscope only flows in a region with diameter ~5 Å, STS is unusual in comparison with other surface spectroscopy
techniques, which average over a larger surface region. The origins of STS are found in some of the earliest STM work of Gerd Binnig
and Heinrich Rohrer
, in which they observed changes in the appearance of some atom
s in the (7 x 7) unit cell of the Si(111) – (7 x 7) surface with tip-sample bias
. STS provides the possibility for probing the local electronic structure of metals, semiconductor
s, and thin insulators on a scale unobtainable with other spectroscopic methods. Additionally, topographic and spectroscopic data can be recorded simultaneously.
phenomena and measurement of the tunneling current or its derivative
, understanding the expressions for the tunneling current is very important. Using the modified Bardeen transfer Hamiltonian method, which treats tunneling as a perturbation
, the tunneling current (I) is found to be
In scanning tunneling microscopy, a metal tip is moved over a conducting sample without making mechanical contact with the sample. A "bias voltage" between the sample and tip allows a current to flow between the tip and the sample even though they are not in contact. This can occur because of quantum mechanical tunneling, hence the name of the instrument.
The scanning tunneling microscope is used to obtain "topographs" - topographic maps - of surfaces. The tip is rastered across a surface and (in constant current mode), a constant current is maintained between the tip and the sample by adjusting the height of the tip. A plot of the tip height at all measurement positions on the raster provides the topograph. These topographic images can obtain information that is atomically resolved, and many beautiful images of metal and superconductor surfaces have been obtained with atomic precision.
However, the scanning tunneling microscope does NOT measure the height of surface features. This can be shown dramatically when a molecule is adsorbed on a surface - the STM image may appear to have either INCREASED or DECREASED height at that feature, although the geometry alone is certainly an INCREASED height. A detailed analysis of the way in which an image is formed shows that the transmission of the electric current between the tip and the sample depends on two factors: (1) the geometry of the sample and (2) the arrangement of the electrons in the sample. The arrangement of the electrons in the sample is described quantum mechanically by an "electron density". The electron density is a function of both position AND energy, and is formally described as the Local Density Of electron States, which is abbreviated to Local Density Of States (LDOS), which is a function of energy.
Spectroscopy, in its most general sense, refers to a measurement of the number of something as a function of energy. For scanning tunneling spectroscopy the scanning tunneling microscope is used to measure the number of electrons (the Local Density Of States) as a function of the electron energy. The electron energy is set by the electrical potential difference (voltage) between the sample and the tip. The location (Local) is set by the position of the tip.
At it's simplest, a "scanning tunneling spectrum" is obtained by placing a scanning tunneling microscope tip above a particular place on the sample. WITH THE HEIGHT OF THE TIP FIXED, the electron tunneling current is then measured as a function of electron energy by varying the voltage between the tip and the sample (the tip to sample voltage sets the electron energy). The change of the current with the energy of the electrons is the simplest spectrum that can be obtained, it us often referred to as an I-V curve. As is shown below, it is the SLOPE of the I-V curve at each voltage (often called the dI/dV-curve) which is more fundamental because dI/dV corresponds to the electron density of states at the local position of the tip, the Local Density of States - LDOS.
In what follows, this simple idea is given a more detailed physical analysis, and some more sophisticated measurement ideas are presented.
Introduction

Scanning tunneling microscope
A scanning tunneling microscope is an instrument for imaging surfaces at the atomic level. Its development in 1981 earned its inventors, Gerd Binnig and Heinrich Rohrer , the Nobel Prize in Physics in 1986. For an STM, good resolution is considered to be 0.1 nm lateral resolution and...
(STM) to probe the local density of electronic states (LDOS) and the band gap
Band gap
In solid state physics, a band gap, also called an energy gap or bandgap, is an energy range in a solid where no electron states can exist. In graphs of the electronic band structure of solids, the band gap generally refers to the energy difference between the top of the valence band and the...
of surfaces and materials on surfaces at the atom
Atom
The atom is a basic unit of matter that consists of a dense central nucleus surrounded by a cloud of negatively charged electrons. The atomic nucleus contains a mix of positively charged protons and electrically neutral neutrons...
ic scale. Generally, STS involves observation of changes in constant-current topographs with tip-sample bias, local measurement of the tunneling current versus tip-sample bias (I-V) curve, measurement of the tunneling conductance,
 , or more than one of these. Since the tunneling current in a scanning tunneling microscope only flows in a region with diameter ~5 Å, STS is unusual in comparison with other surface spectroscopy
, or more than one of these. Since the tunneling current in a scanning tunneling microscope only flows in a region with diameter ~5 Å, STS is unusual in comparison with other surface spectroscopySpectroscopy
Spectroscopy is the study of the interaction between matter and radiated energy. Historically, spectroscopy originated through the study of visible light dispersed according to its wavelength, e.g., by a prism. Later the concept was expanded greatly to comprise any interaction with radiative...
techniques, which average over a larger surface region. The origins of STS are found in some of the earliest STM work of Gerd Binnig
Gerd Binnig
Gerd Binnig is a German physicist, and a Nobel laureate.He was born in Frankfurt am Main and played in the ruins of the city during his childhood. His family lived partly in Frankfurt and partly in Offenbach am Main, and he attended school in both cities. At the age of 10, he decided to become a...
and Heinrich Rohrer
Heinrich Rohrer
Heinrich Rohrer is a Swiss physicist who shared half of the 1986 Nobel Prize in Physics with Gerd Binnig for the design of the scanning tunneling microscope .-Biography:...
, in which they observed changes in the appearance of some atom
Atom
The atom is a basic unit of matter that consists of a dense central nucleus surrounded by a cloud of negatively charged electrons. The atomic nucleus contains a mix of positively charged protons and electrically neutral neutrons...
s in the (7 x 7) unit cell of the Si(111) – (7 x 7) surface with tip-sample bias
Biasing (electronics)
Biasing in electronics is the method of establishing predetermined voltages and/or currents at various points of an electronic circuit to set an appropriate operating point...
. STS provides the possibility for probing the local electronic structure of metals, semiconductor
Semiconductor
A semiconductor is a material with electrical conductivity due to electron flow intermediate in magnitude between that of a conductor and an insulator. This means a conductivity roughly in the range of 103 to 10−8 siemens per centimeter...
s, and thin insulators on a scale unobtainable with other spectroscopic methods. Additionally, topographic and spectroscopic data can be recorded simultaneously.
Tunneling current
Since STS relies on the tunnelingQuantum tunnelling
Quantum tunnelling refers to the quantum mechanical phenomenon where a particle tunnels through a barrier that it classically could not surmount. This plays an essential role in several physical phenomena, such as the nuclear fusion that occurs in main sequence stars like the sun, and has important...
phenomena and measurement of the tunneling current or its derivative
Derivative
In calculus, a branch of mathematics, the derivative is a measure of how a function changes as its input changes. Loosely speaking, a derivative can be thought of as how much one quantity is changing in response to changes in some other quantity; for example, the derivative of the position of a...
, understanding the expressions for the tunneling current is very important. Using the modified Bardeen transfer Hamiltonian method, which treats tunneling as a perturbation
Perturbation theory (quantum mechanics)
In quantum mechanics, perturbation theory is a set of approximation schemes directly related to mathematical perturbation for describing a complicated quantum system in terms of a simpler one. The idea is to start with a simple system for which a mathematical solution is known, and add an...
, the tunneling current (I) is found to be
-
-

where is the Fermi distribution function,
is the Fermi distribution function,  and
and  are the density of statesDensity of statesIn solid-state and condensed matter physics, the density of states of a system describes the number of states per interval of energy at each energy level that are available to be occupied by electrons. Unlike isolated systems, like atoms or molecules in gas phase, the density distributions are not...
are the density of statesDensity of statesIn solid-state and condensed matter physics, the density of states of a system describes the number of states per interval of energy at each energy level that are available to be occupied by electrons. Unlike isolated systems, like atoms or molecules in gas phase, the density distributions are not...
(DOS) in the sample and tip, respectively, and is the tunneling matrix element between the modified wavefunctions of the tip and the sample surface. The tunneling matrix element,
is the tunneling matrix element between the modified wavefunctions of the tip and the sample surface. The tunneling matrix element,
describes the energy lowering due to the interaction between the two states. Here and
and  are the sample wavefunction modified by the tip potential and the tip wavefunction modified by sample potential, respectively.
are the sample wavefunction modified by the tip potential and the tip wavefunction modified by sample potential, respectively.
For low temperatures and a constant tunneling matrix element, the tunneling current reduces to
which is a convolution of the DOS of the tip and the sample. Generally, STS experiments attempt to probe the sample DOS, but equation (3) shows that the tip DOS must be known for the measurement to have meaning. Equation (3) implies that
under the gross assumption that the tip DOS is constant. For these ideal assumptions, the tunneling conductance is directly proportional to the sample DOS.
For higher bias voltages, the predictions of simple planar tunneling models using the Wentzel-Kramers Brillouin (WKB) approximation are useful. In the WKB theory, the tunneling current is predicted to be
where and
and  are the density of states (DOS) in the sample and tip, respectively. The energy- and bias-dependent electron tunneling transition probability, T, is given by
are the density of states (DOS) in the sample and tip, respectively. The energy- and bias-dependent electron tunneling transition probability, T, is given by
where and
and  are the respective work functions of the sample and tip and
are the respective work functions of the sample and tip and  is the distance from the sample to the tip.
is the distance from the sample to the tip.
Experimental methods
Acquiring standardStandard (metrology)In the science of measurement, a standard is an object, system, or experiment that bears a defined relationship to a unit of measurement of a physical quantity. Standards are the fundamental reference for a system of weights and measures, against which all other measuring devices are compared...
STM topographs at many different tip-sample biases and comparing to experimental topographic information is perhaps the most straightforward spectroscopic method. The tip-sample bias can also be changed on a line-by-line basis during a single scan. This method creates two interleaved images at different biases. Since only the states between the Fermi levelFermi levelThe Fermi level is a hypothetical level of potential energy for an electron inside a crystalline solid. Occupying such a level would give an electron a potential energy \epsilon equal to its chemical potential \mu as they both appear in the Fermi-Dirac distribution function,which...
s of the sample and the tip contribute to I, this method is a quick way to determine whether there are any interesting bias-dependent features on the surface. However, only limited information about the electronic structure can be extracted by this method, since the constant I topographs depend on the tip and sample DOS’s and the tunneling transmission probability, which depends on the tip-sample spacing, as described in equation (5).
By using modulation techniques, a constant current topograph and the spatially resolved can be acquired simultaneously. A small, high frequency sinusoidal modulation voltage is superimposed on the D.C.Direct currentDirect current is the unidirectional flow of electric charge. Direct current is produced by such sources as batteries, thermocouples, solar cells, and commutator-type electric machines of the dynamo type. Direct current may flow in a conductor such as a wire, but can also flow through...
can be acquired simultaneously. A small, high frequency sinusoidal modulation voltage is superimposed on the D.C.Direct currentDirect current is the unidirectional flow of electric charge. Direct current is produced by such sources as batteries, thermocouples, solar cells, and commutator-type electric machines of the dynamo type. Direct current may flow in a conductor such as a wire, but can also flow through...
tip-sample bias. The A.C.Alternating currentIn alternating current the movement of electric charge periodically reverses direction. In direct current , the flow of electric charge is only in one direction....
component of the tunneling current is recorded using a lock-in amplifier, and the component in-phase with the tip-sample bias modulation gives directly. In practice, the modulation frequency is chosen slightly higher than the bandwidth of the STM feedback system. This choice prevents the feedback control from compensating for the modulation by changing the tip-sample spacing and minimizes the displacement current 90° out-of-phase with the applied bias modulation. Such effects arise from the capacitance between the tip and the sample, which grows as the modulation frequency increases.
directly. In practice, the modulation frequency is chosen slightly higher than the bandwidth of the STM feedback system. This choice prevents the feedback control from compensating for the modulation by changing the tip-sample spacing and minimizes the displacement current 90° out-of-phase with the applied bias modulation. Such effects arise from the capacitance between the tip and the sample, which grows as the modulation frequency increases.
In order to obtain I-V curves simultaneously with a topograph, a sample-and-hold circuit is used in the feedback loop for the z piezo signal. The sample-and-hold circuit freezes the voltage applied to the z piezo, which freezes the tip-sample distance, at the desired location allowing I-V measurements without the feedback system responding. The tip-sample bias is swept between the specified values, and the tunneling current is recorded. After the spectra acquisition, the tip-sample bias is returned to the scanning value, and the scan resumes. Using this method, the local electronic structure of semiconductors in the band gap can be probed.
There are two ways to record I-V curves in the manner described above. In constant-spacing scanning tunneling spectroscopy (CS-STS), the tip stops scanning at the desired location to obtain an I-V curve. The tip-sample spacing is adjusted to reach the desired initial current, which may be different from the initial current setpoint, at a specified tip-sample bias. A sample-and-hold amplifier freezes the z piezo feedback signal, which holds the tip-sample spacing constant by preventing the feedback system from changing the bias applied to the z piezo. The tip-sample bias is swept through the specified values, and the tunneling current is recorded. Either numerical differentiation of I(V) or lock-in detection as described above for modulation techniques can be used to find . If lock-in detection is used, then an A.C. modulation voltage is applied to the D.C. tip-sample bias during the bias sweep and the A.C. component of the current in-phase with the modulation voltage is recorded.
. If lock-in detection is used, then an A.C. modulation voltage is applied to the D.C. tip-sample bias during the bias sweep and the A.C. component of the current in-phase with the modulation voltage is recorded.
In variable-spacing scanning tunneling spectroscopy (VS-STS), the same steps occur as in CS-STS through turning off the feedback. As the tip-sample bias is swept through the specified values, the tip-sample spacing is decreased continuously as the magnitude of the bias is reduced. Generally, a minimum tip-sample spacing is specified to prevent the tip from crashing into the sample surface at the 0 V tip-sample bias. Lock-in detection and modulation techniques are used to find the conductivity, because the tunneling current is a function also of the varying tip-sample spacing. Numerical differentiation of I(V) with respect to V would include the contributions from the varying tip-sample spacing. Introduced by Mårtensson and Feenstra to allow conductivity measurements over several orders of magnitude, VS-STS is useful for conductivity measurements on systems with large band gaps. Such measurements are necessary to properly define the band edges and examine the gap for states.
Current-imaging-tunneling spectroscopy (CITS) is an STS technique where an I-V curve is recorded at each pixel in the STM topograph. Either variable-spacing or constant-spacing spectroscopy may be used to record the I-V curves. The conductance, , can be obtained by numerical differentiation of I with respect to V or acquired using lock-in detection as described above. As a practical concern, the number of pixels in the scan or the scan area may be reduced to prevent piezo creep or thermal drift from moving the feature of study or the scan area during the duration of the scan. Since some CITS scans can last in excess of 12 hours, low drift and creep are absolutely necessary.
, can be obtained by numerical differentiation of I with respect to V or acquired using lock-in detection as described above. As a practical concern, the number of pixels in the scan or the scan area may be reduced to prevent piezo creep or thermal drift from moving the feature of study or the scan area during the duration of the scan. Since some CITS scans can last in excess of 12 hours, low drift and creep are absolutely necessary.
Data interpretation
From the obtained I-V curves, the band gap of the sample at the location of the I-V measurement can be determined. By plotting the magnitude of I on a log scale versus the tip-sample bias, the band gap can clearly be determined. Although determination of the band gap is possible from a linear plot of the I-V curve, the log scale increases the sensitivity. Alternatively, a plot of the conductance, , versus the tip-sample bias, V, allows one to locate the band edges that determine the band gap.
, versus the tip-sample bias, V, allows one to locate the band edges that determine the band gap.
The structure in the , as a function of the tip-sample bias, is associated with the density of statesDensity of statesIn solid-state and condensed matter physics, the density of states of a system describes the number of states per interval of energy at each energy level that are available to be occupied by electrons. Unlike isolated systems, like atoms or molecules in gas phase, the density distributions are not...
, as a function of the tip-sample bias, is associated with the density of statesDensity of statesIn solid-state and condensed matter physics, the density of states of a system describes the number of states per interval of energy at each energy level that are available to be occupied by electrons. Unlike isolated systems, like atoms or molecules in gas phase, the density distributions are not...
of the surface when the tip-sample bias is less than the work functions of the tip and the sample. Usually, the WKB approximationWKB approximationIn mathematical physics, the WKB approximation or WKB method is a method for finding approximate solutions to linear partial differential equations with spatially varying coefficients...
for the tunneling current is used to interpret these measurements at low tip-sample bias relative to the tip and sample work functions. The derivative of equation (5), I in the WKB approximation, is
where is the sample density of states,
is the sample density of states,  is the tip density of states, and T is the tunneling transmission probability. Although the tunneling transmission probability T is generally unknown, at a fixed location T increases smoothly and monotonically with the tip-sample bias in the WKB approximation. Hence, structure in the
is the tip density of states, and T is the tunneling transmission probability. Although the tunneling transmission probability T is generally unknown, at a fixed location T increases smoothly and monotonically with the tip-sample bias in the WKB approximation. Hence, structure in the  is usually assigned to features in the density of states in the first term of equation (7).
is usually assigned to features in the density of states in the first term of equation (7).
Interpretation of as a function of position is more complicated. Spatial variations in T show up in measurements of
as a function of position is more complicated. Spatial variations in T show up in measurements of  as an inverted topographic background. When obtained in constant current mode, images of the spatial variation of
as an inverted topographic background. When obtained in constant current mode, images of the spatial variation of  contain a convolution of topographic and electronic structure. An additional complication arises since
contain a convolution of topographic and electronic structure. An additional complication arises since  in the low-bias limit. Thus,
in the low-bias limit. Thus,  diverges as V approaches 0, preventing investigation of the local electronic structure near the Fermi level.
diverges as V approaches 0, preventing investigation of the local electronic structure near the Fermi level.
Since both the tunneling current, equation (5), and the conductance, equation (7), depend on the tip DOS and the tunneling transition probability, T, quantitative information about the sample DOS is very difficult to obtain. Additionally, the voltage dependence of T, which is usually unknown, can vary with position due to local fluctuations in the electronic structure of the surface. For some cases, normalizing by dividing by
by dividing by  can minimize the effect of the voltage dependence of T and the influence of the tip-sample spacing. Using the WKB approximation, equations (5) and (7), we obtain:
can minimize the effect of the voltage dependence of T and the influence of the tip-sample spacing. Using the WKB approximation, equations (5) and (7), we obtain:
Feenstra et al. argued that the dependencies of and
and  on tip-sample spacing and tip-sample bias tend to cancel, since they appear as ratios. This cancellation reduces the normalized conductance to the following form:
on tip-sample spacing and tip-sample bias tend to cancel, since they appear as ratios. This cancellation reduces the normalized conductance to the following form:
where normalizes T to the DOS and
normalizes T to the DOS and  describes the influence of the electric field in the tunneling gap on the decay length. Under the assumption that
describes the influence of the electric field in the tunneling gap on the decay length. Under the assumption that  and
and  vary slowly with tip-sample bias, the features in
vary slowly with tip-sample bias, the features in  reflect the sample DOS,
reflect the sample DOS,  .
.
Limitations
While STS can provide spectroscopic information with amazing spatial resolution, there are some limitations. The STM and STS lack chemical sensitivity. Since the tip-sample bias range in tunneling experiments is limited to , where
, where  is the apparent barrier height, STM and STS are only sample valence electron states. Element-specific information is generally impossible to extract from STM and STS experiments, since the chemical bond formation greatly perturbs the valence states.
is the apparent barrier height, STM and STS are only sample valence electron states. Element-specific information is generally impossible to extract from STM and STS experiments, since the chemical bond formation greatly perturbs the valence states.
At finite temperatures, the thermal broadening of the electron energy distribution due to the Fermi-distribution limits spectroscopic resolution. At ,
,  , and the sample and tip energy distribution spread are both
, and the sample and tip energy distribution spread are both  . Hence, the total energy deviation is
. Hence, the total energy deviation is  . Assuming the dispersion relation for simple metals, it follows from the uncertainty relation
. Assuming the dispersion relation for simple metals, it follows from the uncertainty relation  that
that
where is the Fermi energyFermi energyThe Fermi energy is a concept in quantum mechanics usually referring to the energy of the highest occupied quantum state in a system of fermions at absolute zero temperature....
is the Fermi energyFermi energyThe Fermi energy is a concept in quantum mechanics usually referring to the energy of the highest occupied quantum state in a system of fermions at absolute zero temperature....
, is the bottom of the valence band,
is the bottom of the valence band,  is the Fermi wave vector, and
is the Fermi wave vector, and  is the lateral resolution. Since spatial resolution depends on the tip-sample spacing, smaller tip-sample spacings and higher topographic resolution blur the features in tunneling spectra.
is the lateral resolution. Since spatial resolution depends on the tip-sample spacing, smaller tip-sample spacings and higher topographic resolution blur the features in tunneling spectra.
Despite these limitations, STS and STM provide the possibility for probing the local electronic structure of metals, semiconductors, and thin insulators on a scale unobtainable with other spectroscopic methods. Additionally, topographic and spectroscopic data can be recorded simultaneously.
Further reading
- J. Tersoff and D. R. Hamann, Phys. Rev. B 31, 805 - 813 (1985)
- M. Morgenstern et al., J. Electron Spectrosc. Relat. Phenom. 109, 127 (2000)
- G. Binnig, H. Rohrer, Ch. Gerber, and E. Weibel, Phys. Rev. Lett. 50, 120 - 123 (1983)
- G. Binnig, H. Rohrer, Ch. Gerber, and E. Weibel, Phys. Rev. Lett. 49, 57 - 61 (1982)
- G. Binnig, H. Rohrer, Ch. Gerber, and E. Weibel, Appl. Phys. Lett., Vol. 40, Issue 2, pp. 178-180 (1982)
-
-










