Space-oblique Mercator projection is a map projection

Map projection

A map projection is any method of representing the surface of a sphere or other three-dimensional body on a plane. Map projections are necessary for creating maps. All map projections distort the surface in some fashion...

.

History

The space-oblique Mercator projection (SOM) was developed by John P. Snyder

John P. Snyder

John Parr Snyder was an American cartographer most known for his work on map projections for the United States Geological Survey . Educated at Purdue and MIT as a chemical engineer, he had a lifetime interest in map projections as a hobby, but found the calculations tedious without the benefit of...

, Alden Partridge Colvocoresses

Alden Partridge Colvocoresses

Colonel Alden Partridge Colvocoresses , USA , developed in 1973 - 1979 the Space-oblique Mercator projection with John Parr Snyder and John L. Junkins...

 and John L. Junkins in 1976. Snyder had an interest in maps, originating back to his childhood and he regularly attended cartography

Cartography

Cartography is the study and practice of making maps. Combining science, aesthetics, and technique, cartography builds on the premise that reality can be modeled in ways that communicate spatial information effectively.The fundamental problems of traditional cartography are to:*Set the map's...

 conferences while on vacation. When the United States Geological Survey

United States Geological Survey

The United States Geological Survey is a scientific agency of the United States government. The scientists of the USGS study the landscape of the United States, its natural resources, and the natural hazards that threaten it. The organization has four major science disciplines, concerning biology,...

 (USGS) needed to develop a system for reducing the amount of distortion caused when satellite

Satellite

In the context of spaceflight, a satellite is an object which has been placed into orbit by human endeavour. Such objects are sometimes called artificial satellites to distinguish them from natural satellites such as the Moon....

 pictures of the ellipsoidal

Ellipse

In geometry, an ellipse is a plane curve that results from the intersection of a cone by a plane in a way that produces a closed curve. Circles are special cases of ellipses, obtained when the cutting plane is orthogonal to the cone's axis...

 Earth were printed on a flat page, they appealed for help at one such conference. Snyder worked on the problem armed with his newly purchased pocket calculator and devised the mathematical formulas needed to solve the problem. He submitted these to the USGS at no charge, starting off a new career at USGS. His formulas were used to produce maps from Landsat 4

Landsat 4

Landsat 4 is the fourth satellite of the Landsat program. It was launched on July 16th, 1982, with the primary goal of providing a global archive of satellite imagery. Although the Landsat Program is managed by NASA, data from Landsat 4 was collected and distributed by the USGS...

 images launched in the summer of 1978.

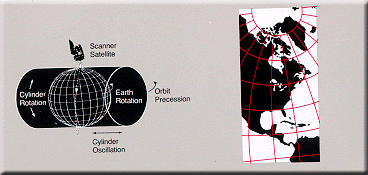
Projection description

The space-oblique Mercator projection provides continual conformal mapping

Conformal map

In mathematics, a conformal map is a function which preserves angles. In the most common case the function is between domains in the complex plane.More formally, a map,...

 of the swath sensed by a satellite. Scale

Scale (map)

The scale of a map is defined as the ratio of a distance on the map to the corresponding distance on the ground.If the region of the map is small enough for the curvature of the Earth to be neglected, then the scale may be taken as a constant ratio over the whole map....

 is true along the ground track

Ground track

A ground track or ground trace is the path on the surface of the Earth directly below an aircraft or satellite. In the case of a satellite, it is the projection of the satellite's orbit onto the surface of the Earth .A satellite ground track may be thought of as a path along the Earth's surface...

, varying 0.01 percent within the normal sensing range of the satellite. Conformality is correct within a few parts per million for the sensing range. Distortion is essentially constant along lines of constant distance parallel to the ground track. SOM is the only projection presented that takes the rotation of Earth into account.

Equations

The forward equations for the Space Oblique Mercator projection for the sphere are as follows: