
Synchronous gauge
Encyclopedia
In general relativity
, a synchronous reference system is a coordinate system
in which the metric
takes the form ,
,
where the Latin indices a and b are summed over the spatial directions and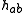 is a spatial metric. Any metric can locally be put into this form by a coordinate transformation. This coordinate condition
is a spatial metric. Any metric can locally be put into this form by a coordinate transformation. This coordinate condition
is called "synchronous" because the t coordinate defines proper time for all comoving observers. However, it is not uniquely defined, and therefore is not a gauge
, as the spacelike hypersurface
at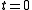 can be chosen arbitrarily. Another problem with the reference system is that caustic
can be chosen arbitrarily. Another problem with the reference system is that caustic
s can occur which cause the gauge choice to break down. These problems have caused some difficulties doing cosmological perturbation theory
in this system, however the problems are now well understood. Synchronous coordinates are generally considered the most efficient reference system for doing calculations, and are used in many modern cosmology codes, such as CMBFAST
. They are also useful for solving theoretical problems in which a spacelike hypersurface needs to be fixed, as with spacelike singularities
.
General relativity
General relativity or the general theory of relativity is the geometric theory of gravitation published by Albert Einstein in 1916. It is the current description of gravitation in modern physics...
, a synchronous reference system is a coordinate system
Coordinate system
In geometry, a coordinate system is a system which uses one or more numbers, or coordinates, to uniquely determine the position of a point or other geometric element. The order of the coordinates is significant and they are sometimes identified by their position in an ordered tuple and sometimes by...
in which the metric
Metric tensor
In the mathematical field of differential geometry, a metric tensor is a type of function defined on a manifold which takes as input a pair of tangent vectors v and w and produces a real number g in a way that generalizes many of the familiar properties of the dot product of vectors in Euclidean...
takes the form
 ,
,where the Latin indices a and b are summed over the spatial directions and
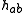 is a spatial metric. Any metric can locally be put into this form by a coordinate transformation. This coordinate condition
is a spatial metric. Any metric can locally be put into this form by a coordinate transformation. This coordinate conditionCoordinate conditions
In general relativity, the laws of physics can be expressed in a generally covariant form. In other words, the real world does not care about our coordinate systems. However, it is often useful to fix upon a particular coordinate system, in order to solve actual problems or make actual predictions...
is called "synchronous" because the t coordinate defines proper time for all comoving observers. However, it is not uniquely defined, and therefore is not a gauge
Gauge theory
In physics, gauge invariance is the property of a field theory in which different configurations of the underlying fundamental but unobservable fields result in identical observable quantities. A theory with such a property is called a gauge theory...
, as the spacelike hypersurface
Hypersurface
In geometry, a hypersurface is a generalization of the concept of hyperplane. Suppose an enveloping manifold M has n dimensions; then any submanifold of M of n − 1 dimensions is a hypersurface...
at
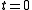 can be chosen arbitrarily. Another problem with the reference system is that caustic
can be chosen arbitrarily. Another problem with the reference system is that causticCaustic (optics)
In optics, a caustic or caustic network is the envelope of light rays reflected or refracted by a curved surface or object, or the projection of that envelope of rays on another surface. The caustic is a curve or surface to which each of the light rays is tangent, defining a boundary of an...
s can occur which cause the gauge choice to break down. These problems have caused some difficulties doing cosmological perturbation theory
Cosmological perturbation theory
In physical cosmology, cosmological perturbation theory is the theory by which the evolution of structure is understood in the big bang model. It uses general relativity to compute the gravitational forces causing small perturbations to grow and eventually seed the formation of stars, quasars,...
in this system, however the problems are now well understood. Synchronous coordinates are generally considered the most efficient reference system for doing calculations, and are used in many modern cosmology codes, such as CMBFAST
CMBFAST
In physical cosmology, CMBFAST is a computer code, written by Uros Seljak and Matias Zaldarriaga, for computing the anisotropy of the cosmic microwave background...
. They are also useful for solving theoretical problems in which a spacelike hypersurface needs to be fixed, as with spacelike singularities
Gravitational singularity
A gravitational singularity or spacetime singularity is a location where the quantities that are used to measure the gravitational field become infinite in a way that does not depend on the coordinate system...
.

