
Theorem of corresponding states
Encyclopedia
According to van der Waals, the theorem of corresponding states (or principle of corresponding states) indicates that all fluid
s, when compared at the same reduced temperature and reduced pressure, have approximately the same compressibility factor
and all deviate from ideal gas behavior to about the same degree.
Material constants that vary for each type of material are eliminated, in a recast reduced form of a constitutive equation
. The reduced variables are defined in terms of critical variables.
It originated with the work of Johannes Diderik van der Waals
in about 1873 when he used the critical temperature and critical pressure to characterize a fluid.
The most prominent example is the van der Waals equation
of state, the reduced form of which applies to all fluids.
 , where the subscript
, where the subscript  indicates the critical point
indicates the critical point
is predicted to be a constant independent of substance by many equations of state; the Van der Waals equation
e.g. predicts a value of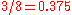 .
.
Fluid
In physics, a fluid is a substance that continually deforms under an applied shear stress. Fluids are a subset of the phases of matter and include liquids, gases, plasmas and, to some extent, plastic solids....
s, when compared at the same reduced temperature and reduced pressure, have approximately the same compressibility factor
Compressibility factor
The compressibility factor , also known as the compression factor, is a useful thermodynamic property for modifying the ideal gas law to account for the real gas behavior. In general, deviation from ideal behavior becomes more significant the closer a gas is to a phase change, the lower the...
and all deviate from ideal gas behavior to about the same degree.
Material constants that vary for each type of material are eliminated, in a recast reduced form of a constitutive equation
Constitutive equation
In physics, a constitutive equation is a relation between two physical quantities that is specific to a material or substance, and approximates the response of that material to external forces...
. The reduced variables are defined in terms of critical variables.
It originated with the work of Johannes Diderik van der Waals
Johannes Diderik van der Waals
Johannes Diderik van der Waals was a Dutch theoretical physicist and thermodynamicist famous for his work on an equation of state for gases and liquids....
in about 1873 when he used the critical temperature and critical pressure to characterize a fluid.
The most prominent example is the van der Waals equation
Van der Waals equation
The van der Waals equation is an equation of state for a fluid composed of particles that have a non-zero volume and a pairwise attractive inter-particle force It was derived by Johannes Diderik van der Waals in 1873, who received the Nobel prize in 1910 for "his work on the equation of state for...
of state, the reduced form of which applies to all fluids.
Compressibility factor at the critical point
The compressibility factor at the critical point, which is defined as , where the subscript
, where the subscript  indicates the critical point
indicates the critical pointCritical point (thermodynamics)
In physical chemistry, thermodynamics, chemistry and condensed matter physics, a critical point, also called a critical state, specifies the conditions at which a phase boundary ceases to exist...
is predicted to be a constant independent of substance by many equations of state; the Van der Waals equation
Van der Waals equation
The van der Waals equation is an equation of state for a fluid composed of particles that have a non-zero volume and a pairwise attractive inter-particle force It was derived by Johannes Diderik van der Waals in 1873, who received the Nobel prize in 1910 for "his work on the equation of state for...
e.g. predicts a value of
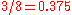 .
.| Substance | Value |
|---|---|
| H2O | 0.23 |
| 4He Helium-4 Helium-4 is a non-radioactive isotope of helium. It is by far the most abundant of the two naturally occurring isotopes of helium, making up about 99.99986% of the helium on earth. Its nucleus is the same as an alpha particle, consisting of two protons and two neutrons. Alpha decay of heavy... |
0.31 |
| He Helium Helium is the chemical element with atomic number 2 and an atomic weight of 4.002602, which is represented by the symbol He. It is a colorless, odorless, tasteless, non-toxic, inert, monatomic gas that heads the noble gas group in the periodic table... |
0.30 |
| H2 Hydrogen Hydrogen is the chemical element with atomic number 1. It is represented by the symbol H. With an average atomic weight of , hydrogen is the lightest and most abundant chemical element, constituting roughly 75% of the Universe's chemical elemental mass. Stars in the main sequence are mainly... |
0.30 |
| Ne Neon Neon is the chemical element that has the symbol Ne and an atomic number of 10. Although a very common element in the universe, it is rare on Earth. A colorless, inert noble gas under standard conditions, neon gives a distinct reddish-orange glow when used in either low-voltage neon glow lamps or... |
0.29 |
| N2 Nitrogen Nitrogen is a chemical element that has the symbol N, atomic number of 7 and atomic mass 14.00674 u. Elemental nitrogen is a colorless, odorless, tasteless, and mostly inert diatomic gas at standard conditions, constituting 78.08% by volume of Earth's atmosphere... |
0.29 |
| Ar Argon Argon is a chemical element represented by the symbol Ar. Argon has atomic number 18 and is the third element in group 18 of the periodic table . Argon is the third most common gas in the Earth's atmosphere, at 0.93%, making it more common than carbon dioxide... |
0.29 |
See also
- Van der Waals equationVan der Waals equationThe van der Waals equation is an equation of state for a fluid composed of particles that have a non-zero volume and a pairwise attractive inter-particle force It was derived by Johannes Diderik van der Waals in 1873, who received the Nobel prize in 1910 for "his work on the equation of state for...
- Equation of stateEquation of stateIn physics and thermodynamics, an equation of state is a relation between state variables. More specifically, an equation of state is a thermodynamic equation describing the state of matter under a given set of physical conditions...
s - Compressibility factorCompressibility factorThe compressibility factor , also known as the compression factor, is a useful thermodynamic property for modifying the ideal gas law to account for the real gas behavior. In general, deviation from ideal behavior becomes more significant the closer a gas is to a phase change, the lower the...
s - Johannes Diderik van der WaalsJohannes Diderik van der WaalsJohannes Diderik van der Waals was a Dutch theoretical physicist and thermodynamicist famous for his work on an equation of state for gases and liquids....
- Noro-Frenkel law of corresponding states
External links
- Properties of Natural Gases. Includes a chart of compressibility factors versus reduced pressure and reduced temperature (on last page of the PDF document)
- Theorem of corresponding states on SklogWiki.

