Main Results

• Together with James Glimm
  James Glimm
  James Gilbert Glimm is an American mathematical physicist, and Professor at the State University of New York at Stony Brook.James Glimm was born in Peoria, Illinois, USA on 24 March 1934.- Career :...
  
   and Arthur Jaffe
  Arthur Jaffe
  Arthur Jaffe is an American mathematical physicist and a professor at Harvard University. Born on December 22, 1937 he attended Princeton University as an undergraduate obtaining a degree in chemistry, and later Clare College, Cambridge, as a Marshall Scholar, obtaining a degree in mathematics...
  
   he invented the cluster expansion
  Cluster expansion
  In statistical mechanics, the cluster expansion is a power series expansion of the partition function of a statistical field theory around a model that is a union of non-interacting 0-dimensional field theories. Cluster expansions originated in the work of...
  
   approach to quantum field theory that is widely used in constructive field theory
  Constructive quantum field theory
  In mathematical physics, constructive quantum field theory is the field devoted to showing that quantum theory is mathematically compatible with special relativity. This demonstration requires new mathematics, in a sense analogous to Newton developing calculus in order to understand planetary...
  
   also nowadays.

• Together with Jürg Fröhlich
  Jürg Fröhlich
  Jürg Martin Fröhlich is a Swiss mathematician and theoretical physicist.In 1965 Fröhlich began to study mathematics and physics at Eidgenössischen Technischen Hochschule Zürich...
  
   and Barry Simon
  Barry Simon
  Barry Simon is an eminent American mathematical physicist and the IBM Professor of Mathematics and Theoretical Physics at Caltech, known for his prolific contributions in spectral theory, functional analysis, and nonrelativistic quantum mechanics , including the connections to atomic and...
  
  , he invented the approach of the infrared bound, nowadays become a classical tool to show phase transitions in various models of statistical mechanics.

• Together with Jürg Fröhlich
  Jürg Fröhlich
  Jürg Martin Fröhlich is a Swiss mathematician and theoretical physicist.In 1965 Fröhlich began to study mathematics and physics at Eidgenössischen Technischen Hochschule Zürich...
  
  , he devised a 'multi-scale analysis' to provide, for the first time, mathematical proofs of: the Kosterlitz–Thouless transition; the phase transition in the one-dimensional ferromagnetic Ising model with interactions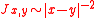 ; the Anderson localization
  Anderson localization
  In condensed matter physics, Anderson localization, also known as strong localization, is the absence of diffusion of waves in a disordered medium. This phenomenon is named after the American physicist P. W...
  
   in arbitrary dimension
  Dimension (vector space)
  In mathematics, the dimension of a vector space V is the cardinality of a basis of V. It is sometimes called Hamel dimension or algebraic dimension to distinguish it from other types of dimension...
  
  .

• Together with David Brydges, he proved that the scaling limit
  Scaling limit
  In physics or mathematics, the scaling limit is a term applied to the behaviour of a lattice model in the limit of the lattice spacing going to zero. A lattice model which approximates a continuum quantum field theory in the limit as the lattice spacing goes to zero corresponds to finding a second...
  
   of the self-avoiding walk
  Self-avoiding walk
  In mathematics, a self-avoiding walk is a sequence of moves on a lattice that does not visit the same point more than once. A self-avoiding polygon is a closed self-avoiding walk on a lattice...
  
   in dimension
  Dimension (vector space)
  In mathematics, the dimension of a vector space V is the cardinality of a basis of V. It is sometimes called Hamel dimension or algebraic dimension to distinguish it from other types of dimension...
  
   greater or equal than 5 is Gaussian, with variance
  Variance
  In probability theory and statistics, the variance is a measure of how far a set of numbers is spread out. It is one of several descriptors of a probability distribution, describing how far the numbers lie from the mean . In particular, the variance is one of the moments of a distribution...
  
   growing linearly in time. To achieve this result, they invented the technique of the lace expansion that since then has had wide application in probability on graphs.