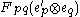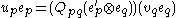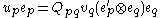Two-point tensor
Encyclopedia
Two-point tensors, or double vectors, are tensor
-like quantities which transform as vectors with respect to each of their indices and are used in continuum mechanics
to transform between reference ("material") and present ("configuration") coordinates. Examples include the first Piola-Kirchhoff stress tensor.
As with many applications of tensors, Einstein summation notation is frequently used. To clarify this notation, capital indices are often used to indicate reference coordinates and lowercase for present coordinates. Thus, a two-point tensor will have one capital and one lower-case index; for example, AjM.
actively transforms a vector u to a vector v such that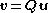
where v and u are measured in the same space and their coordinates representation is with respect to the same basis (denoted by the "e").
In contrast, a two-point tensor, G will be written as
and will transform a vector, U, in the E system to a vector, v, in the e system as
.
.
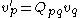For tensors suppose we then have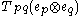
.
A tensor in the system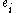
. In another system, let the same tensor be given by
We can say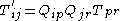
.
Then
is the routine tensor transformation. But a two-point tensor between these systems is just
which transforms as
Now, writing out in full,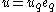
and also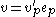
.
This then requires Q to be of the form
By definition of tensor product
,
So we can write
Thus
Incorporating (1), we have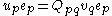
.
Tensor
Tensors are geometric objects that describe linear relations between vectors, scalars, and other tensors. Elementary examples include the dot product, the cross product, and linear maps. Vectors and scalars themselves are also tensors. A tensor can be represented as a multi-dimensional array of...
-like quantities which transform as vectors with respect to each of their indices and are used in continuum mechanics
Continuum mechanics
Continuum mechanics is a branch of mechanics that deals with the analysis of the kinematics and the mechanical behavior of materials modelled as a continuous mass rather than as discrete particles...
to transform between reference ("material") and present ("configuration") coordinates. Examples include the first Piola-Kirchhoff stress tensor.
As with many applications of tensors, Einstein summation notation is frequently used. To clarify this notation, capital indices are often used to indicate reference coordinates and lowercase for present coordinates. Thus, a two-point tensor will have one capital and one lower-case index; for example, AjM.
Continuum mechanics
A conventional tensor can be viewed as a transformation of vectors in one coordinate system to other vectors in the same coordinate system. In contrast, a two-point tensor transforms vectors from one coordinate system to another. That is, a conventional tensor,-
,
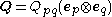actively transforms a vector u to a vector v such that
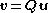
where v and u are measured in the same space and their coordinates representation is with respect to the same basis (denoted by the "e").
In contrast, a two-point tensor, G will be written as
and will transform a vector, U, in the E system to a vector, v, in the e system as
.The transformation law for two-point tensor
Suppose we have two coordinate systems one primed and another unprimed and a vectors' components transform between them as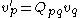.For tensors suppose we then have
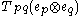.A tensor in the system
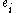. In another system, let the same tensor be given by
-
.
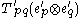We can say
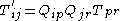.Then
is the routine tensor transformation. But a two-point tensor between these systems is just
which transforms as
-
.
The most mundane example of a two-point tensor
The most mundane example of a two-point tensor is the transformation tensor, the Q in the above discussion. Note that-
.
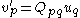Now, writing out in full,
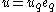
and also
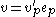.This then requires Q to be of the form
-
.
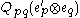By definition of tensor product
Tensor product
In mathematics, the tensor product, denoted by ⊗, may be applied in different contexts to vectors, matrices, tensors, vector spaces, algebras, topological vector spaces, and modules, among many other structures or objects. In each case the significance of the symbol is the same: the most general...
,
So we can write
Thus
Incorporating (1), we have
.
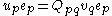