
Uniformly Cauchy sequence
Encyclopedia
In mathematics
, a sequence
of function
s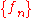 from a set S to a metric space M is said to be uniformly Cauchy if:
from a set S to a metric space M is said to be uniformly Cauchy if:
Another way of saying this is that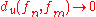 as
as  , where the uniform distance
, where the uniform distance  between two functions is defined by
between two functions is defined by
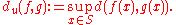
in M. This is a weaker condition than being uniformly Cauchy. Nevertheless, if the metric space M is complete, then any pointwise Cauchy sequence converges pointwise to a function from S to M. Similarly, any uniformly Cauchy sequence will tend uniformly to such a function.
The uniform Cauchy property is frequently used when the S is not just a set, but a topological space
, and M is a complete metric space. The following theorem holds:
of function
s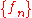 from a set S to a metric space U is said to be uniformly Cauchy if:
from a set S to a metric space U is said to be uniformly Cauchy if:
Mathematics
Mathematics is the study of quantity, space, structure, and change. Mathematicians seek out patterns and formulate new conjectures. Mathematicians resolve the truth or falsity of conjectures by mathematical proofs, which are arguments sufficient to convince other mathematicians of their validity...
, a sequence
Sequence
In mathematics, a sequence is an ordered list of objects . Like a set, it contains members , and the number of terms is called the length of the sequence. Unlike a set, order matters, and exactly the same elements can appear multiple times at different positions in the sequence...
of function
Function (mathematics)
In mathematics, a function associates one quantity, the argument of the function, also known as the input, with another quantity, the value of the function, also known as the output. A function assigns exactly one output to each input. The argument and the value may be real numbers, but they can...
s
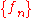 from a set S to a metric space M is said to be uniformly Cauchy if:
from a set S to a metric space M is said to be uniformly Cauchy if:- For all
 , there exists
, there exists  such that for all
such that for all 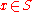 :
: 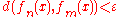 whenever
whenever 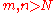 .
.
Another way of saying this is that
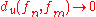 as
as  , where the uniform distance
, where the uniform distance  between two functions is defined by
between two functions is defined by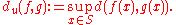
Convergence criteria
A sequence of functions {fn} from S to M is pointwise Cauchy if, for each x ∈ S, the sequence {fn(x)} is a Cauchy sequenceCauchy sequence
In mathematics, a Cauchy sequence , named after Augustin-Louis Cauchy, is a sequence whose elements become arbitrarily close to each other as the sequence progresses...
in M. This is a weaker condition than being uniformly Cauchy. Nevertheless, if the metric space M is complete, then any pointwise Cauchy sequence converges pointwise to a function from S to M. Similarly, any uniformly Cauchy sequence will tend uniformly to such a function.
The uniform Cauchy property is frequently used when the S is not just a set, but a topological space
Topological space
Topological spaces are mathematical structures that allow the formal definition of concepts such as convergence, connectedness, and continuity. They appear in virtually every branch of modern mathematics and are a central unifying notion...
, and M is a complete metric space. The following theorem holds:
- Let S be a topological space and M a complete metric space. Then any uniformly Cauchy sequence of continuous functionContinuous functionIn mathematics, a continuous function is a function for which, intuitively, "small" changes in the input result in "small" changes in the output. Otherwise, a function is said to be "discontinuous". A continuous function with a continuous inverse function is called "bicontinuous".Continuity of...
s fn : S → M tends uniformly to a unique continuous function f : S → M.
Generalization to uniform spaces
A sequenceSequence
In mathematics, a sequence is an ordered list of objects . Like a set, it contains members , and the number of terms is called the length of the sequence. Unlike a set, order matters, and exactly the same elements can appear multiple times at different positions in the sequence...
of function
Function (mathematics)
In mathematics, a function associates one quantity, the argument of the function, also known as the input, with another quantity, the value of the function, also known as the output. A function assigns exactly one output to each input. The argument and the value may be real numbers, but they can...
s
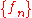 from a set S to a metric space U is said to be uniformly Cauchy if:
from a set S to a metric space U is said to be uniformly Cauchy if:- For all
 and for any entourageUniform spaceIn the mathematical field of topology, a uniform space is a set with a uniform structure. Uniform spaces are topological spaces with additional structure which is used to define uniform properties such as completeness, uniform continuity and uniform convergence.The conceptual difference between...
and for any entourageUniform spaceIn the mathematical field of topology, a uniform space is a set with a uniform structure. Uniform spaces are topological spaces with additional structure which is used to define uniform properties such as completeness, uniform continuity and uniform convergence.The conceptual difference between...
 , there exists
, there exists  such that
such that  whenever
whenever 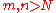 .
.

