Zimm-Bragg model
Encyclopedia
In statistical mechanics
, the Zimm
–Bragg model is a helix-coil transition model
that describes helix-coil transitions of macromolecule
s, usually polymer
chains. Most models provide a reasonable approximation of the fractional helicity of a given polypeptide; the Zimm–Bragg model differs by incorporating the ease of propagation
with respect to nucleation
.
Thus, it is possible to loosely represent a macromolecule as a string such as CCCCHCCHCHHHHHCHCCC and so forth. The number of coils and helices factors into the calculation of fractional helicity,
, defined as
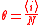
where
is the average helicity and
is the number of helix or coil units.
The Zimm–Bragg model takes the cooperativity of each segment into consideration when calculating fractional helicity. The probability of any given monomer
being a helix or coil is affected by which the previous monomer is; that is, whether the new site is a nucleation or propagation.
By convention, a coil unit ('C') is always of statistical weight 1. Addition of a helix state ('H') to a previously coiled state (nucleation) is assigned a statistical weight
, where 
is the nucleation parameter and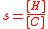
Adding a helix state to a site that is already a helix (propagation) has a statistical weight of
. For most protein
s,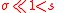
which makes the propagation of a helix more favorable than nucleation of a helix from coil state.
From these parameters, it is possible to compute the fractional helicity
. The average helicity 
is given by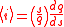
where
is the statistical weight and 
is the partition function
given by the sum of the probabilities of each site on the polypeptide. The fractional helicity is thus given by the equation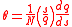
and has no long-range interactions, i.e., interactions between residues well separated along the backbone; therefore, by the famous argument of Rudolf Peierls
, it cannot undergo a phase transition
.
The statistical mechanics of the Zimm–Bragg model may be solved exactly using the transfer-matrix method. The two parameters of the Zimm–Bragg model are σ, the statistical weight
for nucleating a helix and s, the statistical weight for propagating a helix. These parameters may depend on the residue j; for example, a proline
residue may easily nucleate a helix but not propagate one; a leucine
residue may nucleate and propagate a helix easily; whereas glycine may disfavor both the nucleation and propagation of a helix. Since only nearest-neighbour interactions are considered in the Zimm–Bragg model, the full partition function
for a chain of N residues can be written as follows
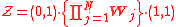
where the 2x2 transfer matrix Wj of the jth residue equals the matrix of statistical weights for the state transitions
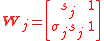
The row-column entry in the transfer matrix equals the statistical weight for making a transition from state row in residue j-1 to state column in residue j. The two states here are helix (the first) and coil (the second). Thus, the upper left entry s is the statistical weight for transitioning from helix to helix, whereas the lower left entry σs is that for transitioning from coil to helix.
Statistical mechanics
Statistical mechanics or statistical thermodynamicsThe terms statistical mechanics and statistical thermodynamics are used interchangeably...
, the Zimm
Bruno H. Zimm
Bruno Hasbrouck Zimm was an American chemist. He was a professor emeritus of chemistry and biochemistry from University of California, San Diego, and a leading polymer chemist and DNA researcher.-Early life:...
–Bragg model is a helix-coil transition model
Helix-coil transition model
Helix–coil transition models are formalized techniques in statistical mechanics developed to describe conformations of linear polymers in solution. The models are usually but not exclusively applied to polypeptides as a measure of the relative fraction of the molecule in an alpha helix conformation...
that describes helix-coil transitions of macromolecule
Macromolecule
A macromolecule is a very large molecule commonly created by some form of polymerization. In biochemistry, the term is applied to the four conventional biopolymers , as well as non-polymeric molecules with large molecular mass such as macrocycles...
s, usually polymer
Polymer
A polymer is a large molecule composed of repeating structural units. These subunits are typically connected by covalent chemical bonds...
chains. Most models provide a reasonable approximation of the fractional helicity of a given polypeptide; the Zimm–Bragg model differs by incorporating the ease of propagation
Self-replication
Self-replication is any behavior of a dynamical system that yields construction of an identical copy of that dynamical system. Biological cells, given suitable environments, reproduce by cell division. During cell division, DNA is replicated and can be transmitted to offspring during reproduction...
with respect to nucleation
Nucleation
Nucleation is the extremely localized budding of a distinct thermodynamic phase. Some examples of phases that may form by way of nucleation in liquids are gaseous bubbles, crystals or glassy regions. Creation of liquid droplets in saturated vapor is also characterized by nucleation...
.
Helix-coil transition models
Helix-coil transition models assume that polypeptides are linear chains composed of interconnected segments. Further, models group these sections into two broad categories: coils, random conglomerations of disparate unbound pieces, are represented by the letter 'C', and helices, ordered states where the chain has assumed a structure stabilized by hydrogen bonding, are represented by the letter 'H'.Thus, it is possible to loosely represent a macromolecule as a string such as CCCCHCCHCHHHHHCHCCC and so forth. The number of coils and helices factors into the calculation of fractional helicity,
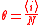
, defined aswhere
is the average helicity and is the number of helix or coil units.Zimm-Bragg
| Dimer sequence | Statistical weight |
|---|---|
 |
 |
 |
 |
 |
 |
 |
 |
The Zimm–Bragg model takes the cooperativity of each segment into consideration when calculating fractional helicity. The probability of any given monomer
Monomer
A monomer is an atom or a small molecule that may bind chemically to other monomers to form a polymer; the term "monomeric protein" may also be used to describe one of the proteins making up a multiprotein complex...
being a helix or coil is affected by which the previous monomer is; that is, whether the new site is a nucleation or propagation.
By convention, a coil unit ('C') is always of statistical weight 1. Addition of a helix state ('H') to a previously coiled state (nucleation) is assigned a statistical weight
, where is the nucleation parameter and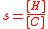Adding a helix state to a site that is already a helix (propagation) has a statistical weight of
. For most proteinProtein
Proteins are biochemical compounds consisting of one or more polypeptides typically folded into a globular or fibrous form, facilitating a biological function. A polypeptide is a single linear polymer chain of amino acids bonded together by peptide bonds between the carboxyl and amino groups of...
s,
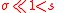
which makes the propagation of a helix more favorable than nucleation of a helix from coil state.
From these parameters, it is possible to compute the fractional helicity
. The average helicity is given by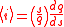where
is the statistical weight and is the partition functionPartition function (statistical mechanics)
Partition functions describe the statistical properties of a system in thermodynamic equilibrium. It is a function of temperature and other parameters, such as the volume enclosing a gas...
given by the sum of the probabilities of each site on the polypeptide. The fractional helicity is thus given by the equation
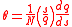
Statistical mechanics
The Zimm–Bragg model is equivalent to a one-dimensional Ising modelIsing model
The Ising model is a mathematical model of ferromagnetism in statistical mechanics. The model consists of discrete variables called spins that can be in one of two states . The spins are arranged in a graph , and each spin interacts with its nearest neighbors...
and has no long-range interactions, i.e., interactions between residues well separated along the backbone; therefore, by the famous argument of Rudolf Peierls
Rudolf Peierls
Sir Rudolf Ernst Peierls, CBE was a German-born British physicist. Rudolf Peierls had a major role in Britain's nuclear program, but he also had a role in many modern sciences...
, it cannot undergo a phase transition
Phase transition
A phase transition is the transformation of a thermodynamic system from one phase or state of matter to another.A phase of a thermodynamic system and the states of matter have uniform physical properties....
.
The statistical mechanics of the Zimm–Bragg model may be solved exactly using the transfer-matrix method. The two parameters of the Zimm–Bragg model are σ, the statistical weight
Statistical weight
In statistical mechanics, the statistical weight is the relative probability of a particular feature of a state. If the energy associated with the feature is ΔE, the statistical weight is given by the Boltzmann factor e-ΔE/kT, where k is the Boltzmann constant and T is the temperature in...
for nucleating a helix and s, the statistical weight for propagating a helix. These parameters may depend on the residue j; for example, a proline
Proline
Proline is an α-amino acid, one of the twenty DNA-encoded amino acids. Its codons are CCU, CCC, CCA, and CCG. It is not an essential amino acid, which means that the human body can synthesize it. It is unique among the 20 protein-forming amino acids in that the α-amino group is secondary...
residue may easily nucleate a helix but not propagate one; a leucine
Leucine
Leucine is a branched-chain α-amino acid with the chemical formula HO2CCHCH2CH2. Leucine is classified as a hydrophobic amino acid due to its aliphatic isobutyl side chain. It is encoded by six codons and is a major component of the subunits in ferritin, astacin and other 'buffer' proteins...
residue may nucleate and propagate a helix easily; whereas glycine may disfavor both the nucleation and propagation of a helix. Since only nearest-neighbour interactions are considered in the Zimm–Bragg model, the full partition function
Partition function (statistical mechanics)
Partition functions describe the statistical properties of a system in thermodynamic equilibrium. It is a function of temperature and other parameters, such as the volume enclosing a gas...
for a chain of N residues can be written as follows
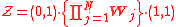
where the 2x2 transfer matrix Wj of the jth residue equals the matrix of statistical weights for the state transitions
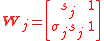
The row-column entry in the transfer matrix equals the statistical weight for making a transition from state row in residue j-1 to state column in residue j. The two states here are helix (the first) and coil (the second). Thus, the upper left entry s is the statistical weight for transitioning from helix to helix, whereas the lower left entry σs is that for transitioning from coil to helix.