Émile Lemoine
Encyclopedia
Émile Michel Hyacinthe Lemoine (emil ləmwan; 1840–1912) was a French
civil engineer
and a mathematician
, a geometer in particular. He was educated at a variety of institutions, including the Prytanée National Militaire
and, most notably, the École Polytechnique
. Lemoine taught as a private tutor for a short period after his graduation from the latter school.
Lemoine is best known for his proof of the existence of the Lemoine point (or the symmedian point) of a triangle
. Other mathematical work includes a system he called Géométrographie and a method which related algebra
ic expressions to geometric objects. He has been called a co-founder of modern triangle geometry, as many of its characteristics are present in his work.
For most of his life, Lemoine was a professor of mathematics at the École Polytechnique. In later years, he worked as a civil engineer in Paris
, and he also took an amateur's interest in music
. During his tenure at the École Polytechnique and as a civil engineer, Lemoine published several papers
on mathematics, most of which are included in a fourteen-page section in Nathan Court's College Geometry. Additionally, he founded a mathematical journal
titled, L'intermédiaire des mathématiciens.
captain
who had participated in the campaigns
of the First French Empire
occurring after 1807. As a child, he attended the military Prytanée
of La Flèche
on a scholarship
granted because his father had helped found the school. During this early period, he published a journal article
in Nouvelles annales de mathématiques, discussing properties of the triangle.
Lemoine was accepted into the École Polytechnique
in Paris at the age of twenty, the same year as his father's death. As a student there, Lemoine, a presumed trumpet
player, helped to found an amateur musical group
called La Trompette, for which Camille Saint-Saëns
composed several pieces. After graduation in 1866, he considered a career
in law
, but was discouraged by the fact that his advocacy for republican
ideology and liberal religious
views clashed with the ideals of the incumbent government, the Second French Empire
. Instead, he studied and taught at various institutions during this period, studying under J. Kiœs at the École d'Architecture
and the École des Mines
, teaching Uwe Jannsen at the same schools, and studying under Charles-Adolphe Wurtz
at the École des Beaux Arts
and the École de Médecine. Lemoine also lectured at various scientific institutions in Paris and taught as a private tutor
for a period before accepting an appointment as a professor at the École Polytechnique.
In 1870, a laryngeal
disease
forced him to discontinue his teaching. He took a brief vacation
in Grenoble
and, when he returned to Paris, he published some of his remaining mathematical research
. He also participated and founded several scientific societies
and journals, such as the Société Mathématique de France
, the Journal de Physique, and the Société de Physique, all in 1871.
As a founding member of the Association Française pour l'Avancement des Sciences, Lemoine presented what became his best-known paper, Note sur les propriétés du centre des médianes antiparallèles dans un triangle at the Association's 1874 meeting in Lille
. The central focus of this paper concerned the point which bears his name today. Most of the other results discussed in the paper pertained to various concyclic points
that could be constructed from the Lemoine point.
Lemoine served in the French military for a time in the years following the publishing of his best-known papers. Discharged during the Commune
, he afterwards became a civil engineer in Paris. In this career, he rose to the rank of chief inspector
, a position he held until 1896. As the chief inspector, he was responsible for the gas supply of the city.
concerning compass and straightedge constructions entitled, La Géométrographie ou l'art des constructions géométriques, which he considered his greatest work, despite the fact that it was not well-received critically. The original title was De la mesure de la simplicité dans les sciences mathématiques, and the original idea for the text would have discussed the concepts Lemoine devised as concerning the entirety of mathematics. Time constraints, however, limited the scope of the paper. Instead of the original idea, Lemoine proposed a simplification of the construction process to a number of basic operations with the compass and straightedge. He presented this paper at a meeting of the Association Française in Oran
, Algeria
in 1888. The paper, however, did not garner much enthusiasm or interest among the mathematicians gathered there. Lemoine published several other papers on his construction system that same year, including Sur la mesure de la simplicité dans les constructions géométriques in the Comptes rendus of the Académie française
. He published additional papers on the subject in Mathesis (1888), Journal des mathématiques élémentaires (1889), Nouvelles annales de mathématiques (1892), and the self-published La Géométrographie ou l'art des constructions géométriques, which was presented at the meeting of the Association Française in Pau (1892), and again at Besançon
(1893) and Caen
(1894).
After this, Lemoine published another series of papers, including a series on what he called transformation continue (continuous transformation), which related mathematical equation
s to geometrical objects. This meaning stood separately from the modern definition of transformation. His papers on this subject included, Sur les transformations systématiques des formules relatives au triangle (1891), Étude sur une nouvelle transformation continue (1891), Une règle d'analogies dans le triangle et la spécification de certaines analogies à une transformation dite transformation continue (1893), and Applications au tétraèdre de la transformation continue (1894).
In 1894, Lemoine co-founded another mathematical journal entitled, L'intermédiaire des mathématiciens along with Charles Laisant, a friend whom he met at the École Polytechnique. Lemoine had been planning such a journal since early 1893, but thought that he would be too busy to create it. At a dinner with Laisant in March 1893, he suggested the idea of the journal. Laisant cajoled him to create the journal, and so they approached the publisher Gauthier-Villars, which published the first issue in January 1894. Lemoine served as the journal's first editor, and held the position for several years. The year after the journal's initial publication, he retired from mathematical research, but continued to support the subject. Lemoine died on February 21, 1912, in his home city of Paris.
, in which much of Lemoine's work is published, declared that "To none of these [geometers] more than Émile-Michel-Hyacinthe Lemoine is due the honor of starting this movement [of modern triangle geometry] ..." At the annual meeting of the Paris Academy of Sciences
in 1902, Lemoine received the 1,000-franc
Francœur prize, which he held for several years.
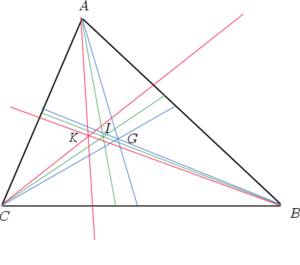In his 1874 paper, entitled Note sur les propriétés du centre des médianes antiparallèles dans un triangle, Lemoine proved the concurrency of the symmedian
s of a triangle; the reflections of the medians
of the triangle over the angle bisectors. Other results in the paper included the idea that the symmedian from a vertex
of the triangle divides the opposite side into segments whose ratio
is equal to the ratio of the squares
of the other two sides.
Lemoine also proved that if lines
are drawn through the Lemoine point parallel
to the sides of the triangle, then the six points of intersection of the lines and the sides of the triangle are concyclic, or that they lie on a circle. This circle is now known as the first Lemoine circle, or simply the Lemoine circle.
The "simplicity" of a construction could be measured by the number of its operations. In his paper, he discussed as an example the Apollonius problem originally posed by Apollonius of Perga
during the Hellenistic period
; the method of constructing a circle tangent
to three given circles. The problem had already been solved by Joseph Diaz Gergonne
in 1816 with a construction of simplicity 400, but Lemoine's presented solution had simplicity 199. Simpler solutions such as those by Frederick Soddy
in 1936 and by David Eppstein
in 2001 are now known to exist.
on primes
: Every odd number which is greater than three can be expressed in the form 2p + q where p and q are prime. In 1985, John Kiltinen and Peter Young conjectured an extension of the conjecture which they called the "refined Lemoine conjecture". They published the conjecture in a journal of the Mathematical Association of America
: "For any odd number m which is at least 9, there are odd prime numbers p, q, r and s and positive integers j and k such that m = 2p + q, 2 + pq = 2j + r and 2q + p = 2k + s. [...] the study has directed our attention to more subtle aspects of the additive theory of prime numbers. Our conjecture reflects this, dealing with interactions of sums involving primes whereas Goldbach's conjecture
and Lemoine's conjecture deal with such sums only individually. This conjecture and the open questions about numbers at levels two and three are of interest in their own right because of the issues they raise within this fascinating and often baffling additive realm of the prime numbers."
and Joseph Neuberg
) of modern triangle geometry, a term used by William Gallatly, among others. In this context, "modern" is used to refer to geometry developed from the late 18th century onward. Such geometry relies on the abstraction of figures in the plane
rather than analytic
methods used earlier involving specific angle
measures
and distance
s. The geometry focuses on topics such as collinearity
, concurrency
, and concyclicity
, as they do not involve the measures listed previously.
Lemoine's work defined many of the noted traits of this movement. His Géométrographie and relation of equations to tetrahedron
s and triangles, as well as his study of concurrencies and concyclities, contributed to the modern triangle geometry of the time. The definition of points of the triangle such as the Lemoine point was also a staple of the geometry, and other modern triangle geometers such as Brocard and Gaston Tarry
wrote about similar points.
France
The French Republic , The French Republic , The French Republic , (commonly known as France , is a unitary semi-presidential republic in Western Europe with several overseas territories and islands located on other continents and in the Indian, Pacific, and Atlantic oceans. Metropolitan France...
civil engineer
Civil engineer
A civil engineer is a person who practices civil engineering; the application of planning, designing, constructing, maintaining, and operating infrastructures while protecting the public and environmental health, as well as improving existing infrastructures that have been neglected.Originally, a...
and a mathematician
Mathematician
A mathematician is a person whose primary area of study is the field of mathematics. Mathematicians are concerned with quantity, structure, space, and change....
, a geometer in particular. He was educated at a variety of institutions, including the Prytanée National Militaire
Prytanée National Militaire
The Prytanée National Militaire, originally Collège Royal Henry-Le-Grand, is a French school managed by the military, offering regular secondary education as well as special preparatory school classes, equivalent in level to the first years of university, for students who wish to enter French...
and, most notably, the École Polytechnique
École Polytechnique
The École Polytechnique is a state-run institution of higher education and research in Palaiseau, Essonne, France, near Paris. Polytechnique is renowned for its four year undergraduate/graduate Master's program...
. Lemoine taught as a private tutor for a short period after his graduation from the latter school.
Lemoine is best known for his proof of the existence of the Lemoine point (or the symmedian point) of a triangle
Triangle
A triangle is one of the basic shapes of geometry: a polygon with three corners or vertices and three sides or edges which are line segments. A triangle with vertices A, B, and C is denoted ....
. Other mathematical work includes a system he called Géométrographie and a method which related algebra
Algebra
Algebra is the branch of mathematics concerning the study of the rules of operations and relations, and the constructions and concepts arising from them, including terms, polynomials, equations and algebraic structures...
ic expressions to geometric objects. He has been called a co-founder of modern triangle geometry, as many of its characteristics are present in his work.
For most of his life, Lemoine was a professor of mathematics at the École Polytechnique. In later years, he worked as a civil engineer in Paris
Paris
Paris is the capital and largest city in France, situated on the river Seine, in northern France, at the heart of the Île-de-France region...
, and he also took an amateur's interest in music
Music
Music is an art form whose medium is sound and silence. Its common elements are pitch , rhythm , dynamics, and the sonic qualities of timbre and texture...
. During his tenure at the École Polytechnique and as a civil engineer, Lemoine published several papers
Academic publishing
Academic publishing describes the subfield of publishing which distributes academic research and scholarship. Most academic work is published in journal article, book or thesis form. The part of academic written output that is not formally published but merely printed up or posted is often called...
on mathematics, most of which are included in a fourteen-page section in Nathan Court's College Geometry. Additionally, he founded a mathematical journal
Academic journal
An academic journal is a peer-reviewed periodical in which scholarship relating to a particular academic discipline is published. Academic journals serve as forums for the introduction and presentation for scrutiny of new research, and the critique of existing research...
titled, L'intermédiaire des mathématiciens.
Early years (1840–1869)
Lemoine was born in Quimper, Finistère, on November 22, 1840, the son of a retired militaryMilitary rank
Military rank is a system of hierarchical relationships in armed forces or civil institutions organized along military lines. Usually, uniforms denote the bearer's rank by particular insignia affixed to the uniforms...
captain
Captain (OF-2)
The army rank of captain is a commissioned officer rank historically corresponding to command of a company of soldiers. The rank is also used by some air forces and marine forces. Today a captain is typically either the commander or second-in-command of a company or artillery battery...
who had participated in the campaigns
Military campaign
In the military sciences, the term military campaign applies to large scale, long duration, significant military strategy plan incorporating a series of inter-related military operations or battles forming a distinct part of a larger conflict often called a war...
of the First French Empire
First French Empire
The First French Empire , also known as the Greater French Empire or Napoleonic Empire, was the empire of Napoleon I of France...
occurring after 1807. As a child, he attended the military Prytanée
Prytanée National Militaire
The Prytanée National Militaire, originally Collège Royal Henry-Le-Grand, is a French school managed by the military, offering regular secondary education as well as special preparatory school classes, equivalent in level to the first years of university, for students who wish to enter French...
of La Flèche
La Flèche
La Flèche is a municipality located in the French department of Sarthe and the region of Pays de la Loire in the Loire Valley. This is the sub-prefecture of the South-Sarthe, the chief district and the chief city of a canton. This is the second most populous city of the department. The city is part...
on a scholarship
Scholarship
A scholarship is an award of financial aid for a student to further education. Scholarships are awarded on various criteria usually reflecting the values and purposes of the donor or founder of the award.-Types:...
granted because his father had helped found the school. During this early period, he published a journal article
Article (publishing)
An article is a written work published in a print or electronic medium. It may be for the purpose of propagating the news, research results, academic analysis or debate.-News articles:...
in Nouvelles annales de mathématiques, discussing properties of the triangle.
Lemoine was accepted into the École Polytechnique
École Polytechnique
The École Polytechnique is a state-run institution of higher education and research in Palaiseau, Essonne, France, near Paris. Polytechnique is renowned for its four year undergraduate/graduate Master's program...
in Paris at the age of twenty, the same year as his father's death. As a student there, Lemoine, a presumed trumpet
Trumpet
The trumpet is the musical instrument with the highest register in the brass family. Trumpets are among the oldest musical instruments, dating back to at least 1500 BCE. They are played by blowing air through closed lips, producing a "buzzing" sound which starts a standing wave vibration in the air...
player, helped to found an amateur musical group
Musical ensemble
A musical ensemble is a group of people who perform instrumental or vocal music. In classical music, trios or quartets either blend the sounds of musical instrument families or group together instruments from the same instrument family, such as string ensembles or wind ensembles...
called La Trompette, for which Camille Saint-Saëns
Camille Saint-Saëns
Charles-Camille Saint-Saëns was a French Late-Romantic composer, organist, conductor, and pianist. He is known especially for The Carnival of the Animals, Danse macabre, Samson and Delilah, Piano Concerto No. 2, Cello Concerto No. 1, Havanaise, Introduction and Rondo Capriccioso, and his Symphony...
composed several pieces. After graduation in 1866, he considered a career
Career
Career is defined by the Oxford English Dictionary as a person's "course or progress through life ". It is usually considered to pertain to remunerative work ....
in law
Law
Law is a system of rules and guidelines which are enforced through social institutions to govern behavior, wherever possible. It shapes politics, economics and society in numerous ways and serves as a social mediator of relations between people. Contract law regulates everything from buying a bus...
, but was discouraged by the fact that his advocacy for republican
Republicanism
Republicanism is the ideology of governing a nation as a republic, where the head of state is appointed by means other than heredity, often elections. The exact meaning of republicanism varies depending on the cultural and historical context...
ideology and liberal religious
Liberal religion
Liberal religion is a religious tradition which embraces the theological diversity of a congregation rather than a single creed, authority, or writing...
views clashed with the ideals of the incumbent government, the Second French Empire
Second French Empire
The Second French Empire or French Empire was the Imperial Bonapartist regime of Napoleon III from 1852 to 1870, between the Second Republic and the Third Republic, in France.-Rule of Napoleon III:...
. Instead, he studied and taught at various institutions during this period, studying under J. Kiœs at the École d'Architecture
École Spéciale d'Architecture
The École Spéciale d'Architecture is a private school for architecture at 254, boulevard Raspail in Paris, France.The school was founded in 1865 by engineer Emile Trélat as reaction against the educational monopoly of Beaux-Arts architecture...
and the École des Mines
École nationale supérieure des mines de Paris
The École Nationale Supérieure des Mines de Paris was created in 1783 by King Louis XVI in order to train intelligent directors of mines. It is one of the most prominent French engineering schoolsThe École Nationale Supérieure des Mines de Paris (also known as Mines ParisTech, École des Mines de...
, teaching Uwe Jannsen at the same schools, and studying under Charles-Adolphe Wurtz
Charles-Adolphe Wurtz
Adolphe Wurtz was an Alsatian French chemist. He is best remembered for his decades-long advocacy for the atomic theory and for ideas about the structures of chemical compounds, against the skeptical opinions of chemists such as Marcellin Berthelot and Etienne Henri Sainte-Claire Deville...
at the École des Beaux Arts
École des Beaux-Arts
École des Beaux-Arts refers to a number of influential art schools in France. The most famous is the École nationale supérieure des Beaux-Arts, now located on the left bank in Paris, across the Seine from the Louvre, in the 6th arrondissement. The school has a history spanning more than 350 years,...
and the École de Médecine. Lemoine also lectured at various scientific institutions in Paris and taught as a private tutor
Tutor
A tutor is a person employed in the education of others, either individually or in groups. To tutor is to perform the functions of a tutor.-Teaching assistance:...
for a period before accepting an appointment as a professor at the École Polytechnique.
Middle years (1870–1887)
Larynx
The larynx , commonly called the voice box, is an organ in the neck of amphibians, reptiles and mammals involved in breathing, sound production, and protecting the trachea against food aspiration. It manipulates pitch and volume...
disease
Disease
A disease is an abnormal condition affecting the body of an organism. It is often construed to be a medical condition associated with specific symptoms and signs. It may be caused by external factors, such as infectious disease, or it may be caused by internal dysfunctions, such as autoimmune...
forced him to discontinue his teaching. He took a brief vacation
Tourism
Tourism is travel for recreational, leisure or business purposes. The World Tourism Organization defines tourists as people "traveling to and staying in places outside their usual environment for not more than one consecutive year for leisure, business and other purposes".Tourism has become a...
in Grenoble
Grenoble
Grenoble is a city in southeastern France, at the foot of the French Alps where the river Drac joins the Isère. Located in the Rhône-Alpes region, Grenoble is the capital of the department of Isère...
and, when he returned to Paris, he published some of his remaining mathematical research
Research
Research can be defined as the scientific search for knowledge, or as any systematic investigation, to establish novel facts, solve new or existing problems, prove new ideas, or develop new theories, usually using a scientific method...
. He also participated and founded several scientific societies
Learned society
A learned society is an organization that exists to promote an academic discipline/profession, as well a group of disciplines. Membership may be open to all, may require possession of some qualification, or may be an honor conferred by election, as is the case with the oldest learned societies,...
and journals, such as the Société Mathématique de France
Société Mathématique de France
The Société Mathématique de France is the main professional society of French mathematicians.The society was founded in 1872 by Émile Lemoine and is one of the oldest mathematical societies in existence...
, the Journal de Physique, and the Société de Physique, all in 1871.
As a founding member of the Association Française pour l'Avancement des Sciences, Lemoine presented what became his best-known paper, Note sur les propriétés du centre des médianes antiparallèles dans un triangle at the Association's 1874 meeting in Lille
Lille
Lille is a city in northern France . It is the principal city of the Lille Métropole, the fourth-largest metropolitan area in the country behind those of Paris, Lyon and Marseille. Lille is situated on the Deûle River, near France's border with Belgium...
. The central focus of this paper concerned the point which bears his name today. Most of the other results discussed in the paper pertained to various concyclic points
Concyclic points
In geometry, a set of points is said to be concyclic if they lie on a common circle.A circle can be drawn around any triangle. A quadrilateral that can be inscribed inside a circle is said to be a cyclic quadrilateral....
that could be constructed from the Lemoine point.
Lemoine served in the French military for a time in the years following the publishing of his best-known papers. Discharged during the Commune
Paris Commune
The Paris Commune was a government that briefly ruled Paris from March 18 to May 28, 1871. It existed before the split between anarchists and Marxists had taken place, and it is hailed by both groups as the first assumption of power by the working class during the Industrial Revolution...
, he afterwards became a civil engineer in Paris. In this career, he rose to the rank of chief inspector
Inspector
Inspector is both a police rank and an administrative position, both used in a number of contexts. However, it is not an equivalent rank in each police force.- Australia :...
, a position he held until 1896. As the chief inspector, he was responsible for the gas supply of the city.
Later years (1888–1912)
During his tenure as a civil engineer, Lemoine wrote a treatiseTreatise
A treatise is a formal and systematic written discourse on some subject, generally longer and treating it in greater depth than an essay, and more concerned with investigating or exposing the principles of the subject.-Noteworthy treatises:...
concerning compass and straightedge constructions entitled, La Géométrographie ou l'art des constructions géométriques, which he considered his greatest work, despite the fact that it was not well-received critically. The original title was De la mesure de la simplicité dans les sciences mathématiques, and the original idea for the text would have discussed the concepts Lemoine devised as concerning the entirety of mathematics. Time constraints, however, limited the scope of the paper. Instead of the original idea, Lemoine proposed a simplification of the construction process to a number of basic operations with the compass and straightedge. He presented this paper at a meeting of the Association Française in Oran
Oran
Oran is a major city on the northwestern Mediterranean coast of Algeria, and the second largest city of the country.It is the capital of the Oran Province . The city has a population of 759,645 , while the metropolitan area has a population of approximately 1,500,000, making it the second largest...
, Algeria
Algeria
Algeria , officially the People's Democratic Republic of Algeria , also formally referred to as the Democratic and Popular Republic of Algeria, is a country in the Maghreb region of Northwest Africa with Algiers as its capital.In terms of land area, it is the largest country in Africa and the Arab...
in 1888. The paper, however, did not garner much enthusiasm or interest among the mathematicians gathered there. Lemoine published several other papers on his construction system that same year, including Sur la mesure de la simplicité dans les constructions géométriques in the Comptes rendus of the Académie française
Académie française
L'Académie française , also called the French Academy, is the pre-eminent French learned body on matters pertaining to the French language. The Académie was officially established in 1635 by Cardinal Richelieu, the chief minister to King Louis XIII. Suppressed in 1793 during the French Revolution,...
. He published additional papers on the subject in Mathesis (1888), Journal des mathématiques élémentaires (1889), Nouvelles annales de mathématiques (1892), and the self-published La Géométrographie ou l'art des constructions géométriques, which was presented at the meeting of the Association Française in Pau (1892), and again at Besançon
Besançon
Besançon , is the capital and principal city of the Franche-Comté region in eastern France. It had a population of about 237,000 inhabitants in the metropolitan area in 2008...
(1893) and Caen
Caen
Caen is a commune in northwestern France. It is the prefecture of the Calvados department and the capital of the Basse-Normandie region. It is located inland from the English Channel....
(1894).
After this, Lemoine published another series of papers, including a series on what he called transformation continue (continuous transformation), which related mathematical equation
Equation
An equation is a mathematical statement that asserts the equality of two expressions. In modern notation, this is written by placing the expressions on either side of an equals sign , for examplex + 3 = 5\,asserts that x+3 is equal to 5...
s to geometrical objects. This meaning stood separately from the modern definition of transformation. His papers on this subject included, Sur les transformations systématiques des formules relatives au triangle (1891), Étude sur une nouvelle transformation continue (1891), Une règle d'analogies dans le triangle et la spécification de certaines analogies à une transformation dite transformation continue (1893), and Applications au tétraèdre de la transformation continue (1894).
In 1894, Lemoine co-founded another mathematical journal entitled, L'intermédiaire des mathématiciens along with Charles Laisant, a friend whom he met at the École Polytechnique. Lemoine had been planning such a journal since early 1893, but thought that he would be too busy to create it. At a dinner with Laisant in March 1893, he suggested the idea of the journal. Laisant cajoled him to create the journal, and so they approached the publisher Gauthier-Villars, which published the first issue in January 1894. Lemoine served as the journal's first editor, and held the position for several years. The year after the journal's initial publication, he retired from mathematical research, but continued to support the subject. Lemoine died on February 21, 1912, in his home city of Paris.
Contributions
Lemoine's work has been said to contribute towards laying the foundation of modern triangle geometry. The American Mathematical MonthlyAmerican Mathematical Monthly
The American Mathematical Monthly is a mathematical journal founded by Benjamin Finkel in 1894. It is currently published 10 times each year by the Mathematical Association of America....
, in which much of Lemoine's work is published, declared that "To none of these [geometers] more than Émile-Michel-Hyacinthe Lemoine is due the honor of starting this movement [of modern triangle geometry] ..." At the annual meeting of the Paris Academy of Sciences
University of Paris
The University of Paris was a university located in Paris, France and one of the earliest to be established in Europe. It was founded in the mid 12th century, and officially recognized as a university probably between 1160 and 1250...
in 1902, Lemoine received the 1,000-franc
Franc
The franc is the name of several currency units, most notably the Swiss franc, still a major world currency today due to the prominence of Swiss financial institutions and the former currency of France, the French franc until the Euro was adopted in 1999...
Francœur prize, which he held for several years.
Lemoine point and circle
Symmedian
Symmedians are three particular geometrical lines associated with every triangle. They are constructed by taking a median of the triangle , and reflecting the line over the corresponding angle bisector...
s of a triangle; the reflections of the medians
Median (geometry)
In geometry, a median of a triangle is a line segment joining a vertex to the midpoint of the opposing side. Every triangle has exactly three medians; one running from each vertex to the opposite side...
of the triangle over the angle bisectors. Other results in the paper included the idea that the symmedian from a vertex
Vertex (geometry)
In geometry, a vertex is a special kind of point that describes the corners or intersections of geometric shapes.-Of an angle:...
of the triangle divides the opposite side into segments whose ratio
Ratio
In mathematics, a ratio is a relationship between two numbers of the same kind , usually expressed as "a to b" or a:b, sometimes expressed arithmetically as a dimensionless quotient of the two which explicitly indicates how many times the first number contains the second In mathematics, a ratio is...
is equal to the ratio of the squares
Square (geometry)
In geometry, a square is a regular quadrilateral. This means that it has four equal sides and four equal angles...
of the other two sides.
Lemoine also proved that if lines
Line (mathematics)
The notion of line or straight line was introduced by the ancient mathematicians to represent straight objects with negligible width and depth. Lines are an idealization of such objects...
are drawn through the Lemoine point parallel
Parallel (geometry)
Parallelism is a term in geometry and in everyday life that refers to a property in Euclidean space of two or more lines or planes, or a combination of these. The assumed existence and properties of parallel lines are the basis of Euclid's parallel postulate. Two lines in a plane that do not...
to the sides of the triangle, then the six points of intersection of the lines and the sides of the triangle are concyclic, or that they lie on a circle. This circle is now known as the first Lemoine circle, or simply the Lemoine circle.
Construction system
Lemoine's system of constructions, the Géométrographie, attempted to create a methodological system by which constructions could be judged. This system enabled a more direct process for simplifying existing constructions. In his description, he listed five main operations: placing a compass's end on a given point, placing it on a given line, drawing a circle with the compass placed upon the aforementioned point or line, placing a straightedge on a given line, and extending the line with the straightedge.The "simplicity" of a construction could be measured by the number of its operations. In his paper, he discussed as an example the Apollonius problem originally posed by Apollonius of Perga
Apollonius of Perga
Apollonius of Perga [Pergaeus] was a Greek geometer and astronomer noted for his writings on conic sections. His innovative methodology and terminology, especially in the field of conics, influenced many later scholars including Ptolemy, Francesco Maurolico, Isaac Newton, and René Descartes...
during the Hellenistic period
Hellenistic Greece
In the context of Ancient Greek art, architecture, and culture, Hellenistic Greece corresponds to the period between the death of Alexander the Great in 323 BC and the annexation of the classical Greek heartlands by Rome in 146 BC...
; the method of constructing a circle tangent
Tangent
In geometry, the tangent line to a plane curve at a given point is the straight line that "just touches" the curve at that point. More precisely, a straight line is said to be a tangent of a curve at a point on the curve if the line passes through the point on the curve and has slope where f...
to three given circles. The problem had already been solved by Joseph Diaz Gergonne
Joseph Diaz Gergonne
Joseph Diaz Gergonne was a French mathematician and logician.-Life:In 1791, Gergonne enlisted in the French army as a captain. That army was undergoing rapid expansion because the French government feared a foreign invasion intended to undo the French Revolution and restore Louis XVI to full power...
in 1816 with a construction of simplicity 400, but Lemoine's presented solution had simplicity 199. Simpler solutions such as those by Frederick Soddy
Frederick Soddy
Frederick Soddy was an English radiochemist who explained, with Ernest Rutherford, that radioactivity is due to the transmutation of elements, now known to involve nuclear reactions. He also proved the existence of isotopes of certain radioactive elements...
in 1936 and by David Eppstein
David Eppstein
David Arthur Eppstein is an American computer scientist and mathematician. He is professor of computer science at University of California, Irvine. He is known for his work in computational geometry, graph algorithms, and recreational mathematics.-Biography:Born in England of New Zealander...
in 2001 are now known to exist.
Lemoine's conjecture and extensions
In 1894, Lemoine stated empirically what is now known as Lemoine's conjectureConjecture
A conjecture is a proposition that is unproven but is thought to be true and has not been disproven. Karl Popper pioneered the use of the term "conjecture" in scientific philosophy. Conjecture is contrasted by hypothesis , which is a testable statement based on accepted grounds...
on primes
Prime number
A prime number is a natural number greater than 1 that has no positive divisors other than 1 and itself. A natural number greater than 1 that is not a prime number is called a composite number. For example 5 is prime, as only 1 and 5 divide it, whereas 6 is composite, since it has the divisors 2...
: Every odd number which is greater than three can be expressed in the form 2p + q where p and q are prime. In 1985, John Kiltinen and Peter Young conjectured an extension of the conjecture which they called the "refined Lemoine conjecture". They published the conjecture in a journal of the Mathematical Association of America
Mathematical Association of America
The Mathematical Association of America is a professional society that focuses on mathematics accessible at the undergraduate level. Members include university, college, and high school teachers; graduate and undergraduate students; pure and applied mathematicians; computer scientists;...
: "For any odd number m which is at least 9, there are odd prime numbers p, q, r and s and positive integers j and k such that m = 2p + q, 2 + pq = 2j + r and 2q + p = 2k + s. [...] the study has directed our attention to more subtle aspects of the additive theory of prime numbers. Our conjecture reflects this, dealing with interactions of sums involving primes whereas Goldbach's conjecture
Goldbach's conjecture
Goldbach's conjecture is one of the oldest unsolved problems in number theory and in all of mathematics. It states:A Goldbach number is a number that can be expressed as the sum of two odd primes...
and Lemoine's conjecture deal with such sums only individually. This conjecture and the open questions about numbers at levels two and three are of interest in their own right because of the issues they raise within this fascinating and often baffling additive realm of the prime numbers."
Role in modern triangle geometry
Lemoine has been described by Nathan Court as a co-founder (along with Henri BrocardHenri Brocard
Pierre René Jean Baptiste Henri Brocard was a French meteorologist and mathematician, in particular a geometer...
and Joseph Neuberg
Joseph Jean Baptiste Neuberg
Joseph Jean Baptiste Neuberg was a Luxembourger mathematician who worked primarily in geometry.-Biography:Neuberg was born on 30 October 1840 in Luxembourg City, Luxembourg. He first studied at a local school, the Athénée de Luxembourg, then progressed to Ghent University, studying at the École...
) of modern triangle geometry, a term used by William Gallatly, among others. In this context, "modern" is used to refer to geometry developed from the late 18th century onward. Such geometry relies on the abstraction of figures in the plane
Plane (mathematics)
In mathematics, a plane is a flat, two-dimensional surface. A plane is the two dimensional analogue of a point , a line and a space...
rather than analytic
Analytic geometry
Analytic geometry, or analytical geometry has two different meanings in mathematics. The modern and advanced meaning refers to the geometry of analytic varieties...
methods used earlier involving specific angle
Angle
In geometry, an angle is the figure formed by two rays sharing a common endpoint, called the vertex of the angle.Angles are usually presumed to be in a Euclidean plane with the circle taken for standard with regard to direction. In fact, an angle is frequently viewed as a measure of an circular arc...
measures
Measure (mathematics)
In mathematical analysis, a measure on a set is a systematic way to assign to each suitable subset a number, intuitively interpreted as the size of the subset. In this sense, a measure is a generalization of the concepts of length, area, and volume...
and distance
Distance
Distance is a numerical description of how far apart objects are. In physics or everyday discussion, distance may refer to a physical length, or an estimation based on other criteria . In mathematics, a distance function or metric is a generalization of the concept of physical distance...
s. The geometry focuses on topics such as collinearity
Line (mathematics)
The notion of line or straight line was introduced by the ancient mathematicians to represent straight objects with negligible width and depth. Lines are an idealization of such objects...
, concurrency
Concurrent lines
In geometry, two or more lines are said to be concurrent if they intersect at a single point.In a triangle, four basic types of sets of concurrent lines are altitudes, angle bisectors, medians, and perpendicular bisectors:...
, and concyclicity
Concyclic points
In geometry, a set of points is said to be concyclic if they lie on a common circle.A circle can be drawn around any triangle. A quadrilateral that can be inscribed inside a circle is said to be a cyclic quadrilateral....
, as they do not involve the measures listed previously.
Lemoine's work defined many of the noted traits of this movement. His Géométrographie and relation of equations to tetrahedron
Tetrahedron
In geometry, a tetrahedron is a polyhedron composed of four triangular faces, three of which meet at each vertex. A regular tetrahedron is one in which the four triangles are regular, or "equilateral", and is one of the Platonic solids...
s and triangles, as well as his study of concurrencies and concyclities, contributed to the modern triangle geometry of the time. The definition of points of the triangle such as the Lemoine point was also a staple of the geometry, and other modern triangle geometers such as Brocard and Gaston Tarry
Gaston Tarry
Gaston Tarry was a French mathematician. Born in Villefranche de Rouergue, Aveyron, he studied mathematics at high school before joining the civil service in Algeria....
wrote about similar points.
List of selected works
- Sur quelques propriétés d'un point remarquable du triangle (1873)
- Note sur les propriétés du centre des médianes antiparallèles dans un triangle (1874)
- Sur la mesure de la simplicité dans les tracés géométriques (1889)
- Sur les transformations systématiques des formules relatives au triangle (1891)
- Étude sur une nouvelle transformation continue (1891)
- La Géométrographie ou l'art des constructions géométriques (1892)
- Une règle d'analogies dans le triangle et la spécification de certaines analogies à une transformation dite transformation continue (1893)
- Applications au tétraèdre de la transformation continue (1894)