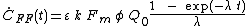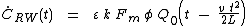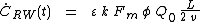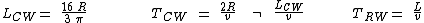
Airborne particulate radioactivity monitoring
Encyclopedia
Continuous particulate air monitors (CPAMs) have been used for years in nuclear facilities to assess airborne particulate radioactivity (APR). In more recent times they may also be used to monitor people in their homes for the presence of manmade radioactivity. These monitors can be used to trigger alarms, indicating to personnel that they should evacuate an area. This article will focus on CPAM use in nuclear power plants
, as opposed to other nuclear fuel-cycle
facilities, or laboratories, or public-safety applications.
In nuclear power plants, CPAMs are used for measuring releases of APR from the facility, monitoring levels of APR for protection of plant personnel, monitoring the air in the reactor containment structure to detect leakage from the reactor systems, and to control ventilation fans, when the APR level has exceeded a defined threshold in the ventilation system.
In general, sampling has better detection sensitivity for low levels of airborne radioactivity, due to the much larger total volume of air passing through the filter medium over the sampling interval (which may be on the order of hours), and also due to the more sophisticated forms of quantitative analysis available once the filter medium is removed from the sampler. On the other hand, monitoring with CPAMs provides nearly real-time airborne radioactivity level indication. It is common practice to refer to "sampled" air even when discussing a CPAM, i.e., as opposed to "monitored" air, which would, strictly, be more correct.
As the air passes through the collection medium (usually a form of filter paper
), particulate matter is deposited onto the filter in either a rectangular or circular pattern, depending on the instrument's design, and then the air continues on its way out of the monitor. The entire deposition area, regardless of its geometric shape, is assumed to be viewed by a radiation detector of a type appropriate for the nuclide in question.
Moving-filter monitors are often used in applications where loading of the filter medium with dust is an issue; this dust loading reduces the air flow over time. The moving-filter collection medium (“tape”) is assumed to move across the deposition area at a constant, known rate. This rate is often established in such a way that a roll of the filter tape will last about one month; a typical filter movement rate is about one inch per hour.
The rectangular-window moving filter monitor will be denoted as RW, and the circular, CW. Fixed filter is FF.
There are effluent monitoring requirements in both 10CFR20 and 10CFR50
; Appendix B to the former and Appendix I to the latter are especially important. 10CFR50 Appendix A states:
Also, Regulatory Guide 1.21, Measuring, Evaluating, and Reporting Radioactivity in Solid Wastes and Releases of Radioactive Materials in Liquid and Gaseous Effluents from Light-Water-Cooled Nuclear Power Plants is highly relevant to this CPAM application.
The following portions of 10CFR20 are relevant to the requirement for occupational exposure CPAM applications: 10CFR20.1003 (definition of Airborne Radioactivity Area), 1201, 1204, 1501, 1502, 2103.
10CFR50 Appendix A states:
This defines a requirement for monitoring the air intake for the control room, such that the exposure limits, including for inhalation exposure, shall not be exceeded. CPAMs are often used for this.
The regulatory basis for this CPAM application is found in 10CFR50:
of the nuclide being collected and measured. It is useful to define a "long-lived" (LL) nuclide to have negligible decay during the measurement interval. On the other hand, if the decay cannot be ignored, the nuclide is considered "short-lived" (SL). In general, for the monitor response models discussed below, the LL response can be obtained from the SL response by taking limits of the SL equation as the decay constant approaches zero. If there is any question about which response model to use, the SL expressions will always apply; however, the LL equations are considerably simpler and so should be used when there is no question about the half-life (e.g., 137Cs is LL).
Ratemeters of both types have the additional function of "smoothing" the output countrate estimate, i.e., reducing its variability. (This process is more correctly termed "filtering.") Ratemeters must make a tradeoff between this necessary variance reduction and their response time; a smooth output (small variance) will tend to lag behind an increase in the true pulse rate. The significance of this lag depends on the application of the monitor.
(RnTn) or natural airborne radioactivity. The mathematical treatment described in this article does not consider this situation, but it can be treated using matrix methods (see Ref [11]).
and activation product
nuclides. The modeling discussed in this article considers only one nuclide at a time. However, since the radiation emitted by each nuclide is independent of the others, so that the nuclides present on the filter medium do not interact with each other, the monitor response is the linear combination of the individual responses. Thus the overall CPAM response to a mixture is just the superposition (i.e., the sum) of the individual responses.
, for "gross beta
-gamma
" counting, or a NaI(Tl) crystal, often for simple single-channel gamma spectroscopy
. (In this context, "gross" means a measurement that does not attempt to find the specific nuclides in the sample.) Plastic scintillators are also popular. Essentially, in power reactor applications, beta and gamma are the radiations of interest for particulate monitoring. In other fuel-cycle applications, alpha
detection is of interest; in that case, the interference from RnTn is a major problem.
Radioiodine
(especially 131I) monitoring is often done using a particulate-monitor setup, but with an activated charcoal
collection medium, which can adsorb some iodine vapors as well as particulate forms. Single-channel spectroscopy is usually specified for iodine monitors. More sophisticated analysis, such as the use of HPGe
detectors and multichannel analyzers, can be used, but these systems are very expensive and not common in commercial power stations.
"Line loss" refers to the losses of particulate matter in transit from a sampling point to the monitor; thus the concentration measured would be somewhat lower than that in the original sampled air. This factor is meant to compensate for these losses. Sampling lines are specifically designed to minimize these losses, for example, by making bends gradual as opposed to right-angled. These lines (pipes) are needed since in many applications the CPAM cannot be physically located directly in the sampled air volume, such as a nuclear power plant's main stack, or the ventilation air intake for the plant control room.
"Emission abundance" refers to the fact that the disintegration of any given nucleus of the isotope of interest in the CPAM analysis may not result in the emission of the radiation being detected (e.g., a beta particle or gamma ray). Thus, overall there will be some fraction of the disintegrations that emit the radiation of interest (e.g. the 662 keV gamma ray of 137Cs is emitted in about 85% of the disintegrations of 137Cs nuclei).
which expresses the rate of change of the monitor countrate: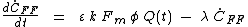
The first term accounts for the source of radioactivity from the sampled air, and the second term is the loss due to the decay of that radioactivity. A convenient way to express the solution to this equation uses the scalar convolution integral, which results in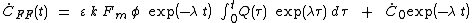
The last term accounts for any initial activity on the filter medium, and is usually set to zero (clean filter at time zero). The initial countrate of the monitor, before the concentration transient begins, is only that due to ambient background. If radon progeny are present, they are assumed to be at equilibrium and generating a constant countrate that adds to the ambient background’s countrate.
Various solutions for the time-dependent FF countrate follow directly, once a concentration time-dependence Q(t) has been specified. Note that the monitor flowrate Fm is assumed constant; if it isn't, and its time-dependence is known, then that Fm(t) would need to be placed inside the integral. Also note that the time variable in all the models is measured from the instant the concentration in the sampled air begins to increase.
 For the moving-filter CPAMs, the above expression is a starting point, but the models are considerably more complicated, due to (1) the loss of material as the filter medium moves away from the detector's field of view and (2) the differing lengths of time that parts of the filter medium have been exposed to the sampled air. The basic modeling approach is to break down the deposition regions into small differential areas and then consider how long each such area receives radioactive material from the air.
For the moving-filter CPAMs, the above expression is a starting point, but the models are considerably more complicated, due to (1) the loss of material as the filter medium moves away from the detector's field of view and (2) the differing lengths of time that parts of the filter medium have been exposed to the sampled air. The basic modeling approach is to break down the deposition regions into small differential areas and then consider how long each such area receives radioactive material from the air.
The resulting expressions are integrated across the deposition region to find the overall response. The RW solution consists of two double integrals, while the CW response solution consists of three triple integrals. A very important consideration in these models is the "transit time," which is the time required for a differential area to traverse the window along its longest dimension. As a practical matter, the transit time is the time required for all differential elements that were in the deposition window at time zero to leave the window.
This figure shows contours of constant activity on a CW deposition area, after the transit time has expired. The filter moves from left to right, and the activity increases from left to right. The differential areas on the diameter have been in the deposition window the longest, and at the far right, have been in the window, accumulating activity, for the full transit time.
Finally, to illustrate the complexity of these models, the RW response for time less than the transit time is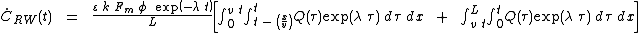
and, also, one of the CW triple integrals is superimposed on the contour plot.
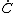 .
.
Nuclear power
Nuclear power is the use of sustained nuclear fission to generate heat and electricity. Nuclear power plants provide about 6% of the world's energy and 13–14% of the world's electricity, with the U.S., France, and Japan together accounting for about 50% of nuclear generated electricity...
, as opposed to other nuclear fuel-cycle
Nuclear fuel cycle
The nuclear fuel cycle, also called nuclear fuel chain, is the progression of nuclear fuel through a series of differing stages. It consists of steps in the front end, which are the preparation of the fuel, steps in the service period in which the fuel is used during reactor operation, and steps in...
facilities, or laboratories, or public-safety applications.
In nuclear power plants, CPAMs are used for measuring releases of APR from the facility, monitoring levels of APR for protection of plant personnel, monitoring the air in the reactor containment structure to detect leakage from the reactor systems, and to control ventilation fans, when the APR level has exceeded a defined threshold in the ventilation system.
Introduction
CPAMs use a pump to draw air through a filter medium to collect airborne particulate matter that carries very small particles of radioactive material; the air itself is not radioactive. The particulate radioactive material might be natural, e.g., radon decay products ("progeny", e.g., 212Pb), or manmade, usually fission or activation products (e.g., 137Cs), or a combination of both. There are also "gas monitors" which pass the air through a sample chamber volume which is viewed continuously by a radiation detector. Radionuclides that occur in the gaseous form (e.g., 85Kr) are not collected on the CPAM filter to any appreciable extent, so that a separate monitoring system is needed to assess these nuclide concentrations in the sampled air. These gas monitors are often placed downstream of a CPAM so that any particulate matter in the sampled air is collected by the CPAM and thus will not contaminate the gas monitor's sample chamber.Monitoring vs. sampling
In monitoring, the region of deposition of this material onto the filter medium is continuously viewed by a radiation detector, concurrent with the collection. This is as opposed to a sampling system, in which the airborne material is collected by pumping air, usually at a much higher volumetric flowrate than a CPAM, through a collection medium for some period of time, but there is no continuous radiation detection; the filter medium is removed periodically from the sampler and taken to a separate radiation detection system for analysis.In general, sampling has better detection sensitivity for low levels of airborne radioactivity, due to the much larger total volume of air passing through the filter medium over the sampling interval (which may be on the order of hours), and also due to the more sophisticated forms of quantitative analysis available once the filter medium is removed from the sampler. On the other hand, monitoring with CPAMs provides nearly real-time airborne radioactivity level indication. It is common practice to refer to "sampled" air even when discussing a CPAM, i.e., as opposed to "monitored" air, which would, strictly, be more correct.
CPAM types
There are two major types of CPAMs, fixed-filter and moving-filter. In the former, the filter medium does not move while the airborne material is collected. The latter type has two main variants, the rectangular deposition area (“window”) and the circular window. In both types of CPAM the sampled air is pulled (not pushed) by a pump through the piping of the monitor up to the structure that holds the filter medium. It is important to note that CPAM pumps are specially designed to maintain a constant volumetric flowrate.As the air passes through the collection medium (usually a form of filter paper
Filter paper
Filter paper is a semi-permeable paper barrier placed perpendicular to a liquid or air flow. It is used to separate fine solids from liquids or air.-Properties:Filter paper comes in various porosities and grades depending on the applications it is meant for...
), particulate matter is deposited onto the filter in either a rectangular or circular pattern, depending on the instrument's design, and then the air continues on its way out of the monitor. The entire deposition area, regardless of its geometric shape, is assumed to be viewed by a radiation detector of a type appropriate for the nuclide in question.
Moving-filter monitors are often used in applications where loading of the filter medium with dust is an issue; this dust loading reduces the air flow over time. The moving-filter collection medium (“tape”) is assumed to move across the deposition area at a constant, known rate. This rate is often established in such a way that a roll of the filter tape will last about one month; a typical filter movement rate is about one inch per hour.
The rectangular-window moving filter monitor will be denoted as RW, and the circular, CW. Fixed filter is FF.
CPAM applications: effluent monitoring
CPAMs are used to monitor the air effluents from nuclear facilities, notably power reactors. Here the objective is to assess the amount of certain radionuclides released from the facility. Real-time measurement of the very low concentrations released by these facilities is difficult; a more-reliable measurement of the total radioactivity released over some time interval (days, perhaps weeks) may in some cases be an acceptable approach. In effluent monitoring, a sample of the air in the plant stack is withdrawn and pumped (pulled) down to the CPAM location. This sampled air in many cases must travel a considerable distance through piping. Extracting and transporting the particulates for the CPAM to measure in such a way that the measurement is representative of what is being released from the facility is challenging.There are effluent monitoring requirements in both 10CFR20 and 10CFR50
Code of Federal Regulations
The Code of Federal Regulations is the codification of the general and permanent rules and regulations published in the Federal Register by the executive departments and agencies of the Federal Government of the United States.The CFR is published by the Office of the Federal Register, an agency...
; Appendix B to the former and Appendix I to the latter are especially important. 10CFR50 Appendix A states:
-
- Criterion 64--Monitoring radioactivity releases. Means shall be provided for monitoring the reactor containment atmosphere, spaces containing components for recirculation of loss-of-coolant accident fluids, effluent discharge paths, and the plant environs for radioactivity that may be released from normal operations, including anticipated operational occurrences, and from postulated accidents.
Also, Regulatory Guide 1.21, Measuring, Evaluating, and Reporting Radioactivity in Solid Wastes and Releases of Radioactive Materials in Liquid and Gaseous Effluents from Light-Water-Cooled Nuclear Power Plants is highly relevant to this CPAM application.
CPAM applications: occupational exposure assessment
For occupational exposure (inhalation) assessment, CPAMs may be used to monitor the air in some volume, such as a compartment in a nuclear facility where personnel are working. A difficulty with this is that, unless the air in the compartment is uniformly mixed, the measurement made at the monitor location may not be representative of the concentration of radioactive material in the air that the workers are breathing. For this application the CPAM may be physically placed directly in the occupied compartment, or it may extract sampled air from the HVAC system that serves that compartment.The following portions of 10CFR20 are relevant to the requirement for occupational exposure CPAM applications: 10CFR20.1003 (definition of Airborne Radioactivity Area), 1201, 1204, 1501, 1502, 2103.
CPAM applications: process monitoring and control
Radiation monitors in general have a number of process-control applications in nuclear power plants; a major CPAM application in this area is the monitoring of the air intake for the plant control room. In the event of an accident, high levels of airborne radioactivity could be brought into the control room by its HVAC system; the CPAM monitors this air and is intended to detect high concentrations of radioactivity and shut down the HVAC flow when necessary.10CFR50 Appendix A states:
-
- Criterion 19--Control room. A control room shall be provided from which actions can be taken to operate the nuclear power unit safely under normal conditions and to maintain it in a safe condition under accident conditions, including loss-of-coolant accidents. Adequate radiation protection shall be provided to permit access and occupancy of the control room under accident conditions without personnel receiving radiation exposures in excess of 5 rem whole body, or its equivalent to any part of the body, for the duration of the accident. Equipment at appropriate locations outside the control room shall be provided (1) with a design capability for prompt hot shutdown of the reactor, including necessary instrumentation and controls to maintain the unit in a safe condition during hot shutdown, and (2) with a potential capability for subsequent cold shutdown of the reactor through the use of suitable procedures.
This defines a requirement for monitoring the air intake for the control room, such that the exposure limits, including for inhalation exposure, shall not be exceeded. CPAMs are often used for this.
CPAM applications: reactor leak detection
Leakage from the so-called "reactor coolant pressure boundary" is required to be monitored in nuclear power plants.. Monitoring the airborne particulate radioactivity in the reactor containment structure is an acceptable method to meet this requirement, and so CPAMs are used. It is the case that when primary coolant escapes into the containment structure, certain noble gas nuclides become airborne, and subsequently decay into particulate nuclides. One of the most common of these pairs is 88Kr and 88Rb; the latter is detected by the CPAM. Relating the observed CPAM response to the 88Rb back to a leakage rate from the primary system is far from trivial.The regulatory basis for this CPAM application is found in 10CFR50:
-
- 10 CFR 50, Appendix A, "General Design Criteria for Nuclear Power Plants," Criterion 30, "Quality of reactor coolant pressure boundary," requires that means be provided for detecting and, to the extent practical, identifying the location of the source of reactor coolant leakage. The specific attributes of the reactor coolant leakage detection systems are outlined in Regulatory Positions 1 through 9 of Regulatory Guide 1.45.
-
- 10 CFR 50.36, "Technical Specifications," paragraph (c)(2)(ii)(A), specifies that a Limiting Condition for Operation be established for installed instrumentation that is used to detect and indicate in the control room a significant abnormal degradation of the reactor coolant pressure boundary. This instrumentation is required by Specification 3.4.15, "RCS Leakage Detection Instrumentation."
Importance of nuclide half-life
The response of the monitor is sensitive to the half-lifeHalf-life
Half-life, abbreviated t½, is the period of time it takes for the amount of a substance undergoing decay to decrease by half. The name was originally used to describe a characteristic of unstable atoms , but it may apply to any quantity which follows a set-rate decay.The original term, dating to...
of the nuclide being collected and measured. It is useful to define a "long-lived" (LL) nuclide to have negligible decay during the measurement interval. On the other hand, if the decay cannot be ignored, the nuclide is considered "short-lived" (SL). In general, for the monitor response models discussed below, the LL response can be obtained from the SL response by taking limits of the SL equation as the decay constant approaches zero. If there is any question about which response model to use, the SL expressions will always apply; however, the LL equations are considerably simpler and so should be used when there is no question about the half-life (e.g., 137Cs is LL).
Ratemeter
The output of the radiation detector is a random sequence of pulses, usually processed by some form of "ratemeter," which continuously estimates the rate at which the detector is responding to the radioactivity deposited on the filter medium. There are two fundamental types of ratemeters, analog and digital. The ratemeter output is called the countrate, and it varies with time.Ratemeters of both types have the additional function of "smoothing" the output countrate estimate, i.e., reducing its variability. (This process is more correctly termed "filtering.") Ratemeters must make a tradeoff between this necessary variance reduction and their response time; a smooth output (small variance) will tend to lag behind an increase in the true pulse rate. The significance of this lag depends on the application of the monitor.
Ambient background
Even when the filter medium is clean, that is, before the pump is started that pulls the air through the filter, the detector will respond to the ambient "background" radiation in the vicinity of the monitor. The countrate that results from deposited radioactivity is called the "net" countrate, and is obtained by subtracting this background countrate from the dynamically-varying countrate that is observed once the pump is started. The background is usually assumed to be constant.Integration time
The countrate of the monitor varies dynamically, so that a measurement time interval must be specified. Also, these are integrating devices, meaning that some finite time is required to accumulate radioactivity onto the filter medium. The input to the monitor is, in general, a time-dependent concentration in air of the specified nuclide. However, for the calculations given below, this concentration will be held constant over that interval.Constant-concentration time limitation
Since concentrations resulting from physical events tend to vary with time, due to dilution processes and/or a nonconstant source term (airborne radioactivity emission rate), it is not realistic to hold the concentration constant for significant lengths of time. Thus, measurement intervals on the order of several hours are not plausible for the purposes of these calculations.Parent-progeny; RnTn
There are situations in which a nuclide deposited on the CPAM filter decays into another nuclide, and that second nuclide remains on the filter. This "parent-progeny" or decay chain situation is especially relevant to so-called "radon-thoron"Radon
Radon is a chemical element with symbol Rn and atomic number 86. It is a radioactive, colorless, odorless, tasteless noble gas, occurring naturally as the decay product of uranium or thorium. Its most stable isotope, 222Rn, has a half-life of 3.8 days...
(RnTn) or natural airborne radioactivity. The mathematical treatment described in this article does not consider this situation, but it can be treated using matrix methods (see Ref [11]).
Multiple nuclides; superposition
Another issue is the fact that in a power reactor context it would be unusual for a CPAM to be collecting only a single particulate nuclide; more likely there would be a mixture of fission productFission product
Nuclear fission products are the atomic fragments left after a large atomic nucleus fissions. Typically, a large nucleus like that of uranium fissions by splitting into two smaller nuclei, along with a few neutrons and a large release of energy in the form of heat , gamma rays and neutrinos. The...
and activation product
Activation product
Activation products are materials made radioactive by neutron activation.Fission products and actinides produced by neutron absorption of nuclear fuel itself are normally referred to by those specific names, and activation product reserved for products of neutron capture by other materials, such as...
nuclides. The modeling discussed in this article considers only one nuclide at a time. However, since the radiation emitted by each nuclide is independent of the others, so that the nuclides present on the filter medium do not interact with each other, the monitor response is the linear combination of the individual responses. Thus the overall CPAM response to a mixture is just the superposition (i.e., the sum) of the individual responses.
Detector type
Most CPAMs use either a Geiger tubeGeiger counter
A Geiger counter, also called a Geiger–Müller counter, is a type of particle detector that measures ionizing radiation. They detect the emission of nuclear radiation: alpha particles, beta particles or gamma rays. A Geiger counter detects radiation by ionization produced in a low-pressure gas in a...
, for "gross beta
Beta particle
Beta particles are high-energy, high-speed electrons or positrons emitted by certain types of radioactive nuclei such as potassium-40. The beta particles emitted are a form of ionizing radiation also known as beta rays. The production of beta particles is termed beta decay...
-gamma
Gamma ray
Gamma radiation, also known as gamma rays or hyphenated as gamma-rays and denoted as γ, is electromagnetic radiation of high frequency . Gamma rays are usually naturally produced on Earth by decay of high energy states in atomic nuclei...
" counting, or a NaI(Tl) crystal, often for simple single-channel gamma spectroscopy
Gamma spectroscopy
Gamma-ray spectroscopy is the quantitative study of the energy spectra of gamma-ray sources, both nuclear laboratory, geochemical, and astrophysical. Gamma rays are the highest-energy form of electromagnetic radiation, being physically exactly like all other forms except for higher photon energy...
. (In this context, "gross" means a measurement that does not attempt to find the specific nuclides in the sample.) Plastic scintillators are also popular. Essentially, in power reactor applications, beta and gamma are the radiations of interest for particulate monitoring. In other fuel-cycle applications, alpha
Alpha particle
Alpha particles consist of two protons and two neutrons bound together into a particle identical to a helium nucleus, which is classically produced in the process of alpha decay, but may be produced also in other ways and given the same name...
detection is of interest; in that case, the interference from RnTn is a major problem.
Radioiodine
Iodine-131
Iodine-131 , also called radioiodine , is an important radioisotope of iodine. It has a radioactive decay half-life of about eight days. Its uses are mostly medical and pharmaceutical...
(especially 131I) monitoring is often done using a particulate-monitor setup, but with an activated charcoal
Activated carbon
Activated carbon, also called activated charcoal, activated coal or carbo activatus, is a form of carbon that has been processed to make it extremely porous and thus to have a very large surface area available for adsorption or chemical reactions.The word activated in the name is sometimes replaced...
collection medium, which can adsorb some iodine vapors as well as particulate forms. Single-channel spectroscopy is usually specified for iodine monitors. More sophisticated analysis, such as the use of HPGe
Semiconductor detector
This article is about particle detectors. For information about semiconductor detectors in radio, see Diode#Semiconductor_diodes, rectifier, detector and cat's-whisker detector....
detectors and multichannel analyzers, can be used, but these systems are very expensive and not common in commercial power stations.
Dynamic response of CPAMs
Detailed mathematical models that describe the dynamic, time-dependent countrate response of these monitors in a very general manner are presented in and will not be repeated here. For the purpose of this article, a few useful results from that paper will be summarized. The objective is to predict the net countrate of a CPAM for a single, specific manmade nuclide, for a given set of conditions. That predicted response can be compared to the expected background and/or interferences (nuclides other than the one sought), to assess the monitor’s detection capability. The response predictions can also be used to calculate alarm setpoints that correspond to appropriate limits (such as those in 10CFR20) on the concentration of airborne radioactivity in the sampled air.Model parameters
The parameters used in these models are summarized in this list:- Time interval (t); time; measured from start of concentration step
- Concentration (Q0); activity / volume; assumed constant over the interval
- Decay constant (λ); 1 / time; for the specified nuclide
- Media collection/retention efficiency (φ); implicitly includes line loss
- Window length or radius (L or R); length; consistent units with v
- Filter speed (v); length / time; length has same units as L or R
- Flow rate (Fm); volume / time; assumed constant over the interval
- Detection efficiency (ε); counts / disintegration; implicitly includes emission abundance
"Line loss" refers to the losses of particulate matter in transit from a sampling point to the monitor; thus the concentration measured would be somewhat lower than that in the original sampled air. This factor is meant to compensate for these losses. Sampling lines are specifically designed to minimize these losses, for example, by making bends gradual as opposed to right-angled. These lines (pipes) are needed since in many applications the CPAM cannot be physically located directly in the sampled air volume, such as a nuclear power plant's main stack, or the ventilation air intake for the plant control room.
"Emission abundance" refers to the fact that the disintegration of any given nucleus of the isotope of interest in the CPAM analysis may not result in the emission of the radiation being detected (e.g., a beta particle or gamma ray). Thus, overall there will be some fraction of the disintegrations that emit the radiation of interest (e.g. the 662 keV gamma ray of 137Cs is emitted in about 85% of the disintegrations of 137Cs nuclei).
Fixed-filter model
The response models are based on the consideration of the sources and losses of the deposited radioactivity on the filter medium. Taking the simplest case, the FF monitor, this leads to a differential equationOrdinary differential equation
In mathematics, an ordinary differential equation is a relation that contains functions of only one independent variable, and one or more of their derivatives with respect to that variable....
which expresses the rate of change of the monitor countrate:
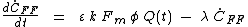
The first term accounts for the source of radioactivity from the sampled air, and the second term is the loss due to the decay of that radioactivity. A convenient way to express the solution to this equation uses the scalar convolution integral, which results in
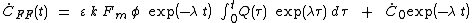
The last term accounts for any initial activity on the filter medium, and is usually set to zero (clean filter at time zero). The initial countrate of the monitor, before the concentration transient begins, is only that due to ambient background. If radon progeny are present, they are assumed to be at equilibrium and generating a constant countrate that adds to the ambient background’s countrate.
Various solutions for the time-dependent FF countrate follow directly, once a concentration time-dependence Q(t) has been specified. Note that the monitor flowrate Fm is assumed constant; if it isn't, and its time-dependence is known, then that Fm(t) would need to be placed inside the integral. Also note that the time variable in all the models is measured from the instant the concentration in the sampled air begins to increase.
Moving-filter models

The resulting expressions are integrated across the deposition region to find the overall response. The RW solution consists of two double integrals, while the CW response solution consists of three triple integrals. A very important consideration in these models is the "transit time," which is the time required for a differential area to traverse the window along its longest dimension. As a practical matter, the transit time is the time required for all differential elements that were in the deposition window at time zero to leave the window.
This figure shows contours of constant activity on a CW deposition area, after the transit time has expired. The filter moves from left to right, and the activity increases from left to right. The differential areas on the diameter have been in the deposition window the longest, and at the far right, have been in the window, accumulating activity, for the full transit time.
Finally, to illustrate the complexity of these models, the RW response for time less than the transit time is
and, also, one of the CW triple integrals is superimposed on the contour plot.
Selected CPAM response models: constant concentration
In these equations, k is a conversion constant for units reconciliation. Again, a very important parameter for moving-filter monitors is the “transit time” (T), which is the window length (or diameter) divided by the filter tape speed v. The countrate is denoted by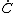 .
.Fixed-filter (FF), any half-life
Fixed-filter (FF), long-lived (LL)
Rectangular window (RW), time less than transit time T, any half-life
-

Rectangular window (RW), time less than transit time T, LL
-
- Note that as v approaches zero, these RW equations reduce to the FF solutions.
Rectangular window (RW), time greater than or equal to transit time T, any half-life
-
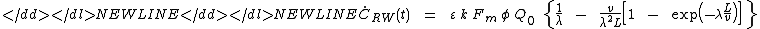
Rectangular window (RW), time greater than or equal to transit time T, LL
Circular window (CW) responses
-
- These response-model equations are quite complicated and some involve a nonelementary integralNonelementary integralIn mathematics, a nonelementary antiderivative is an antiderivative for which it can be shown that there exists no formula in terms of elementary functions . A theorem by Liouville in 1835 provided the first proof that nonelementary antiderivatives exist...
; the exact solutions can be found here. It is shown here, however, that a reasonable approximation for predicting the CW response can be obtained by using the RW equations above, with an “adjusted” window length LCW used in each occurrence of the parameter L, except that the CW transit time TCW is found from 2R / v, not from using LCW as given here in the TRW relation L / v. Thus,
- These response-model equations are quite complicated and some involve a nonelementary integral
Example CPAM response plots
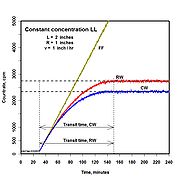 These plots show the predicted CPAM countrate responses for these parameter settings: Detection efficiency, 0.2; Flowrate, 5 cubic feet per minute (cfm); Collection efficiency, 0.7; Constant concentration, 1E-09
These plots show the predicted CPAM countrate responses for these parameter settings: Detection efficiency, 0.2; Flowrate, 5 cubic feet per minute (cfm); Collection efficiency, 0.7; Constant concentration, 1E-09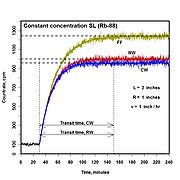
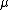 Ci/cc; Rectangular window length, 2 inches; Circular window radius, 1 inch; Media (tape) speed, 1 inch/hour. The concentration instantly steps up to its constant value when the time reaches 30 minutes, and there is a 100 count per minute (cpm) constant background. Note: A microcurieCurieThe curie is a unit of radioactivity, defined asThis is roughly the activity of 1 gram of the radium isotope 226Ra, a substance studied by the pioneers of radiology, Marie and Pierre Curie, for whom the unit was named. In addition to the curie, activity can be measured using an SI derived unit,...
Ci/cc; Rectangular window length, 2 inches; Circular window radius, 1 inch; Media (tape) speed, 1 inch/hour. The concentration instantly steps up to its constant value when the time reaches 30 minutes, and there is a 100 count per minute (cpm) constant background. Note: A microcurieCurieThe curie is a unit of radioactivity, defined asThis is roughly the activity of 1 gram of the radium isotope 226Ra, a substance studied by the pioneers of radiology, Marie and Pierre Curie, for whom the unit was named. In addition to the curie, activity can be measured using an SI derived unit,...
( Ci) is a measure of the disintegration rate, or activity, of a radioactive source; it is 2.22E06 disintegrations per minute.
Ci) is a measure of the disintegration rate, or activity, of a radioactive source; it is 2.22E06 disintegrations per minute.
In the LL plot, note that the FF countrate continues to increase. This is because there is no significant loss of radioactivity from the filter medium. The RW and CW monitors, on the other hand, approach a limiting countrate and the monitor response remains constant as long as the input concentration remains constant.
For the SL plot, all three monitor responses approach a constant level. For the FF monitor, this is due to the source and loss terms becoming equal; since 88Rb has a half-life of about 18 minutes, the loss of radioactive material from the filter medium is significant. This loss also happens on the RW and CW monitors, but there, the loss due to the filter movement also plays a role.
In both plots, PoissonPoisson distributionIn probability theory and statistics, the Poisson distribution is a discrete probability distribution that expresses the probability of a given number of events occurring in a fixed interval of time and/or space if these events occur with a known average rate and independently of the time since...
"noise" is added and a constant-gain digital filterDigital filterIn electronics, computer science and mathematics, a digital filter is a system that performs mathematical operations on a sampled, discrete-time signal to reduce or enhance certain aspects of that signal. This is in contrast to the other major type of electronic filter, the analog filter, which is...
is applied, emulating the countrate responses as they would be observed on a modern CPAM. The horizontal dotted lines are the limiting countrates calculated from the equations given in the previous section.
Also in both plots the transit times are indicated; note that these times are measured from the start of the concentration, at time 30 minutes, not from the arbitrary time zero of the plots. In these example graphs, the length of the RW and the diameter of the CW are equal; if they were not equal, then the transit times would not be equal.
The inverse problem: estimating a concentration from the observed response
Having mathematical models that can predict the CPAM response, i.e., the monitor's output, for a defined input (airborne radioactive material concentration), it is natural to ask whether the process can be "inverted." That is, given an observed CPAM output, is it possible to estimate the input to the monitor?
A misleading "quantitative method" for moving-filter CPAMs
A number of approaches to this inverse problem are addressed in detail in. Each method has its advantages and disadvantages, as one might expect, and a method that might work well for a fixed-filter monitor may be useless for a moving-filter monitor (or vice-versa).
One important conclusion from this paper is that for all practical purposes moving-filter monitors are not usable for quantitative estimation of a time-dependent concentration. The only moving-filter method that has been used historically involves a constant-concentration, LL assumption, which leads to the RW expression:
or for CW,
Thus, a concentration estimate is available only after the transit time T has expired; in most CPAM applications this time is on the order of several (e.g., 4) hours. Whether it is reasonable to assume that the concentration will stay constant for this length of time, and to further assume that only long-lived nuclides are present, is at least debatable, and it is arguable that in many practical situations these assumptions are not realistic.
For example, in power reactor leak detection applications, as mentioned in the first section of this article, CPAMs are used, and a primary nuclide of interest is 88Rb, which is far from long-lived (half-life 18 minutes). Also, in the dynamic environment of a reactor containment building the 88Rb concentration would not be expected to remain constant on a time scale of hours, as required by this measurement method.
However, realistic or not, it has for decades been the practice of CPAM vendors to provide a set of curves (graphs) based on the expressions above. Such graphs have concentration on the vertical axis, and net countrate on the horizontal axis. There often is a family of curves, parameterized on the detection efficiency (or labeled as to specific nuclides). The implication in providing these graphs is that one is to observe a net countrate, at any time, enter the graph at this value, and read off the concentration that exists at that time. To the contrary, unless the time is greater than the transit time T, the nuclide of interest is long-lived, and the concentration is constant over the entire interval, this process will lead to incorrect concentration estimates.
Quantitative methods for CPAM applications
As discussed in the referenced paper, there are at least 11 possible quantitative methods for estimating the concentration or quantities derived from it. The "concentration" may only be at a specific time, or it might be an average over some time interval; this averaging is perfectly acceptable in some applications. In a few cases, the time-dependent concentration itself can be estimated. These various methods involve the countrate, the time derivativeTime derivativeA time derivative is a derivative of a function with respect to time, usually interpreted as the rate of change of the value of the function. The variable denoting time is usually written as t\,.-Notation:...
of the countrate, the time integral of the countrate, and various combinations of these.
The countrate is, as mentioned above, developed from the raw detector pulses by either an analog or digital ratemeter. The integrated counts are easily obtained simply by accumulating the pulses in a "scaler" or, in more modern implementations, in software. Estimating the rate of change (time derivative) of the countrate is difficult to do with any reasonable precision, but modern digital signal processingDigital signal processingDigital signal processing is concerned with the representation of discrete time signals by a sequence of numbers or symbols and the processing of these signals. Digital signal processing and analog signal processing are subfields of signal processing...
methods can be used to good effect.
It turns out that it is very useful to find the time integral of the concentration, as opposed to estimating the time-dependent concentration itself. It is essential to consider this choice for any CPAM application; in many cases the integrated concentration is not only more useful in a radiological protectionHealth physicsHealth physics is a field of science concerned with radiation physics and radiation biology with the goal of providing technical information and proper techniques regarding the safe use of ionizing radiation...
sense, but is also more readily accomplished, since estimating a concentration in (more or less) real-time is difficult.
For example, the total activity released from a plant stack over a time interval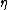 is
is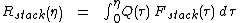
Then, for a fixed-filter monitor, assuming a constant stack and monitor flowrate, it can be shown that

so that the release is a function of both the countrate and integrated counts. This approach was implemented at the SM-1 Nuclear Power PlantArmy Nuclear Power ProgramThe Army Nuclear Power Program was a program of the United States Army to develop small pressurized water and boiling water nuclear power reactors to generate electrical and space-heating energy primarily at remote, relatively inaccessible sites. The ANPP had several notable accomplishments, but...
in the late 1960s, for estimating the releases of episodic containmentContainment buildingA containment building, in its most common usage, is a steel or reinforced concrete structure enclosing a nuclear reactor. It is designed, in any emergency, to contain the escape of radiation to a maximum pressure in the range of 60 to 200 psi...
purges, with a predominant, and strongly time-varying, nuclide of 88Rb. For a LL nuclide, the integral term vanishes, and the release depends only on the attained countrate. A similar equation applies for the occupational exposure situation, replacing the stack flowrate with a worker's breathing rate.
An interesting subtlety to these calculations is that the time in the CPAM response equations is measured from the start of a concentration transient, so that some method of detecting the resulting change in a noisy countrate must be developed. Again, this is a good application for statistical signal processing that is made possible by the use of computing power in modern CPAMs.
Which of these 11 methods to use for the applications discussed previously is not especially obvious, although there are some candidate methods that logically would be used in some applications and not in others. For example, the response time of a given CPAM quantitative method may be far too slow for some applications, and perfectly reasonable for others. The methods have varying sensitivities (detection capabilities; how small a concentration or quantity of radioactivity can reliably be detected) as well, and this must enter into the decision.
CPAM calibration
The calibration of a CPAM usually includes: (1) choosing a quantitative method; (2) estimating the parameters needed to implement that method, notably the detection efficiency for specified nuclides, as well as the sampling line loss and collection efficiency factors; (3) estimating, under specified conditions, the background response of the instrument, which is needed for calculating the detection sensitivity. This sensitivity is often called the minimum detectable concentration or MDC, assuming that a concentration is the quantity estimated by the selected quantitative method.
What is of interest for the MDC is the variability (not the level) of the CPAM background countrate. This variability is measured using the standard deviationStandard deviationStandard deviation is a widely used measure of variability or diversity used in statistics and probability theory. It shows how much variation or "dispersion" there is from the average...
; care must be taken to account for biasUnbiased estimation of standard deviationThe question of unbiased estimation of a standard deviation arises in statistics mainly as question in statistical theory. Except in some important situations, outlined later, the task has little relevance to applications of statistics since its need is avoided by standard procedures, such as the...
in this estimate due to the autocorrelationAutocorrelationAutocorrelation is the cross-correlation of a signal with itself. Informally, it is the similarity between observations as a function of the time separation between them...
of the sequential monitor readings. The autocorrelation bias can make the calculated MDC significantly smaller than is actually the case, which in turn makes the monitor appear to be capable of reliably detecting smaller concentrations than it in fact can.
An uncertainty analysisExperimental uncertainty analysisThe purpose of this introductory article is to discuss the experimental uncertainty analysis of a derived quantity, based on the uncertainties in the experimentally measured quantities that are used in some form of mathematical relationship to calculate that derived quantity...
for the estimated quantity (concentration, release, uptake) is also part of the calibration process. Other performance characteristics can be part of this process, such as estimating response time, estimating the effect of temperature changes on the monitor response, and so on.-
-