
Archimedean spiral
Encyclopedia
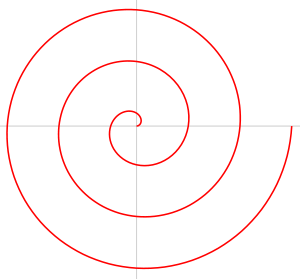
Spiral
In mathematics, a spiral is a curve which emanates from a central point, getting progressively farther away as it revolves around the point.-Spiral or helix:...
named after the 3rd century BC Greek
Ancient Greece
Ancient Greece is a civilization belonging to a period of Greek history that lasted from the Archaic period of the 8th to 6th centuries BC to the end of antiquity. Immediately following this period was the beginning of the Early Middle Ages and the Byzantine era. Included in Ancient Greece is the...
mathematician
Mathematician
A mathematician is a person whose primary area of study is the field of mathematics. Mathematicians are concerned with quantity, structure, space, and change....
Archimedes
Archimedes
Archimedes of Syracuse was a Greek mathematician, physicist, engineer, inventor, and astronomer. Although few details of his life are known, he is regarded as one of the leading scientists in classical antiquity. Among his advances in physics are the foundations of hydrostatics, statics and an...
. It is the locus
Locus (mathematics)
In geometry, a locus is a collection of points which share a property. For example a circle may be defined as the locus of points in a plane at a fixed distance from a given point....
of points corresponding to the locations over time of a point moving away from a fixed point with a constant speed along a line which rotates with constant angular velocity
Angular velocity
In physics, the angular velocity is a vector quantity which specifies the angular speed of an object and the axis about which the object is rotating. The SI unit of angular velocity is radians per second, although it may be measured in other units such as degrees per second, revolutions per...
. Equivalently, in polar coordinates
Polar coordinate system
In mathematics, the polar coordinate system is a two-dimensional coordinate system in which each point on a plane is determined by a distance from a fixed point and an angle from a fixed direction....
(r, θ) it can be described by the equation

with real numbers a and b. Changing the parameter a will turn the spiral, while b controls the distance between successive turnings.
Archimedes described such a spiral in his book On Spirals
On Spirals
On Spirals is a treatise by Archimedes in 225 BC. Although Archimedes did not discover the Archimedean spiral, he employed it in this book to square the circle and trisect an angle.-Preface:...
.
Characteristics
This Archimedean spiral is distinguished from the logarithmic spiralLogarithmic spiral
A logarithmic spiral, equiangular spiral or growth spiral is a special kind of spiral curve which often appears in nature. The logarithmic spiral was first described by Descartes and later extensively investigated by Jacob Bernoulli, who called it Spira mirabilis, "the marvelous...
by the fact that successive turnings of the spiral have a constant separation distance (equal to 2π
Pi
' is a mathematical constant that is the ratio of any circle's circumference to its diameter. is approximately equal to 3.14. Many formulae in mathematics, science, and engineering involve , which makes it one of the most important mathematical constants...
b if θ is measured in radian
Radian
Radian is the ratio between the length of an arc and its radius. The radian is the standard unit of angular measure, used in many areas of mathematics. The unit was formerly a SI supplementary unit, but this category was abolished in 1995 and the radian is now considered a SI derived unit...
s), while in a logarithmic spiral these distances form a geometric progression
Geometric progression
In mathematics, a geometric progression, also known as a geometric sequence, is a sequence of numbers where each term after the first is found by multiplying the previous one by a fixed non-zero number called the common ratio. For example, the sequence 2, 6, 18, 54, ... is a geometric progression...
.
The Archimedean spiral has two arms, one for θ > 0 and one for θ < 0. The two arms are smoothly connected at the origin. Only one arm is shown on the accompanying graph. Taking the mirror image of this arm across the y-axis will yield the other arm.
General Archimedean spiral
Sometimes the term Archimedean spiral is used for the more general group of spirals
The normal Archimedean spiral occurs when x = 1. Other spirals falling into this group include the hyperbolic spiral
Hyperbolic spiral
thumb|200px|right|Hyperbolic spiral for a=2A hyperbolic spiral is a transcendental plane curve also known as a reciprocal spiral. A hyperbolic spiral is the opposite of an Archimedean spiral and are a type of Cotes' spiral.It has the polar equation:...
, Fermat's spiral, and the lituus
Lituus (mathematics)
thumb|300px|Branch for positive rIn mathematics, a lituus is a spiral in which the angle is inversely proportional to the square of the radius .r^2\theta = k \,...
. Virtually all static spirals appearing in nature are logarithmic spiral
Logarithmic spiral
A logarithmic spiral, equiangular spiral or growth spiral is a special kind of spiral curve which often appears in nature. The logarithmic spiral was first described by Descartes and later extensively investigated by Jacob Bernoulli, who called it Spira mirabilis, "the marvelous...
s, not Archimedean ones. Many dynamic spirals (such as the Parker spiral
Parker spiral
The Parker spiral is the shape of the Sun's magnetic field as it extends through the solar system. Unlike the familiar shape of the field from a bar magnet, the Sun's extended field is twisted into an arithmetic spiral by the magnetohydrodynamic influence of the solar wind...
of the solar wind
Solar wind
The solar wind is a stream of charged particles ejected from the upper atmosphere of the Sun. It mostly consists of electrons and protons with energies usually between 1.5 and 10 keV. The stream of particles varies in temperature and speed over time...
, or the pattern made by a Catherine's wheel
Catherine wheel (firework)
The Catherine wheel is a type of firework consisting of a powder-filled spiral tube, or an angled rocket mounted with a pin through its centre...
) are Archimedean.
Constructing an Archimedean spiral
This is an extended geometrical construction, where a constant rate of expansion of the compass is applied during rotation of the compass. To generate an Archimedean spiral by hand for arts crafts, one may follow the instructions given in "Handicraft For Boys" by A. Frederick Collins, 1869.- "Make a loop in one end of a thread as before and tie the other end tightly to a large pin; wind the thread around the pin until all of it is on except the loop; push the pin through the paper on which you want to draw the spiral and into the drawing board [...]
- Next put the point of the pencil in the loop and move it around the pin just as you did in making the circle and you will find that you have drawn a very pretty geometrical spiral which is known as the spiral of Archimedes. It is so called because Archimedes was the first to explain that it was caused by a point moving with uniform angular speed and receding from the center at a constant rate."
Applications
One method of squaring the circleSquaring the circle
Squaring the circle is a problem proposed by ancient geometers. It is the challenge of constructing a square with the same area as a given circle by using only a finite number of steps with compass and straightedge...
, by relaxing the strict limitations on the use of straightedge and compass in ancient Greek geometric proofs, makes use of an Archimedean spiral.

Scroll compressor
A scroll compressor is a device for compressing air or refrigerant...
s, made from two interleaved Archimedean spirals of the same size, are used for compressing liquids and gases. The coils of watch
Watch
A watch is a small timepiece, typically worn either on the wrist or attached on a chain and carried in a pocket, with wristwatches being the most common type of watch used today. They evolved in the 17th century from spring powered clocks, which appeared in the 15th century. The first watches were...
balance spring
Balance spring
A balance spring, or hairspring, is a part used in mechanical timepieces. The balance spring, attached to the balance wheel, controls the speed at which the wheels of the timepiece turn, and thus the rate of movement of the hands...
s and the grooves of very early gramophone record
Gramophone record
A gramophone record, commonly known as a phonograph record , vinyl record , or colloquially, a record, is an analog sound storage medium consisting of a flat disc with an inscribed, modulated spiral groove...
s form Archimedean spirals, making the grooves evenly spaced and maximizing the amount of music that could be fit onto the record (although this was later changed to allow better sound quality). Asking for a patient to draw an Archimedean spiral is a way of quantifying human tremor
Tremor
A tremor is an involuntary, somewhat rhythmic, muscle contraction and relaxation involving to-and-fro movements of one or more body parts. It is the most common of all involuntary movements and can affect the hands, arms, eyes, face, head, vocal folds, trunk, and legs. Most tremors occur in the...
; this information helps in diagnosing neurological diseases. Archimedean spirals are also used in digital light processing (DLP) projection systems to minimize the "rainbow effect", making it look as if multiple colors are displayed at the same time, when in reality red, green, and blue are being cycled extremely quickly. Also, Archimedean spirals are used in food microbiology to quantify bacterial concentration through a spiral platter.
See also
- Hyperbolic spiralHyperbolic spiralthumb|200px|right|Hyperbolic spiral for a=2A hyperbolic spiral is a transcendental plane curve also known as a reciprocal spiral. A hyperbolic spiral is the opposite of an Archimedean spiral and are a type of Cotes' spiral.It has the polar equation:...
- Fermat's spiral
- Logarithmic spiralLogarithmic spiralA logarithmic spiral, equiangular spiral or growth spiral is a special kind of spiral curve which often appears in nature. The logarithmic spiral was first described by Descartes and later extensively investigated by Jacob Bernoulli, who called it Spira mirabilis, "the marvelous...
- Triple spiral symbolTriple spiralThe triple spiral or triskele is a Celtic and pre-Celtic symbol found on a number of Irish Megalithic and Neolithic sites, most notably inside the Newgrange passage tomb, on the entrance stone, and on some of the curbstones surrounding the mound....

