
Average order of an arithmetic function
Encyclopedia
In number theory
, the average order of an arithmetic function is some simpler or better-understood function which takes the same values "on average".
Let f be an arithmetic function
. We say that the average order of f is g if
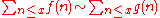
as x tends to infinity.
It is conventional to choose an approximating function g that is continuous
and monotone
.
Number theory
Number theory is a branch of pure mathematics devoted primarily to the study of the integers. Number theorists study prime numbers as well...
, the average order of an arithmetic function is some simpler or better-understood function which takes the same values "on average".
Let f be an arithmetic function
Arithmetic function
In number theory, an arithmetic function is a real or complex valued function ƒ defined on the set of natural numbers In number theory, an arithmetic (or arithmetical) function is a real or complex valued function ƒ(n) defined on the set of natural numbers In number theory, an arithmetic (or...
. We say that the average order of f is g if
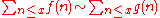
as x tends to infinity.
It is conventional to choose an approximating function g that is continuous
Continuous function
In mathematics, a continuous function is a function for which, intuitively, "small" changes in the input result in "small" changes in the output. Otherwise, a function is said to be "discontinuous". A continuous function with a continuous inverse function is called "bicontinuous".Continuity of...
and monotone
Monotonic function
In mathematics, a monotonic function is a function that preserves the given order. This concept first arose in calculus, and was later generalized to the more abstract setting of order theory....
.
Examples
- The average order of d(n), the number of divisorsDivisor functionIn mathematics, and specifically in number theory, a divisor function is an arithmetical function related to the divisors of an integer. When referred to as the divisor function, it counts the number of divisors of an integer. It appears in a number of remarkable identities, including relationships...
of n, is log(n); - The average order of σ(n), the sum of divisors of n, is nπ2 / 6;
- The average order of φ(n), Euler's totient functionEuler's totient functionIn number theory, the totient \varphi of a positive integer n is defined to be the number of positive integers less than or equal to n that are coprime to n In number theory, the totient \varphi(n) of a positive integer n is defined to be the number of positive integers less than or equal to n that...
of n, is 6n / π2; - The average order of r(n), the number of ways of expressing n as a sum of two squares, is π;
- The average order of ω(n), the number of distinct prime factorPrime factorIn number theory, the prime factors of a positive integer are the prime numbers that divide that integer exactly, without leaving a remainder. The process of finding these numbers is called integer factorization, or prime factorization. A prime factor can be visualized by understanding Euclid's...
s of n, is log log n; - The average order of Ω(n), the number of prime factors of n, is log log n;
- The prime number theoremPrime number theoremIn number theory, the prime number theorem describes the asymptotic distribution of the prime numbers. The prime number theorem gives a general description of how the primes are distributed amongst the positive integers....
is equivalent to the statement that the von Mangoldt functionVon Mangoldt functionIn mathematics, the von Mangoldt function is an arithmetic function named after German mathematician Hans von Mangoldt.-Definition:The von Mangoldt function, conventionally written as Λ, is defined as...
Λ(n) has average order 1.
See also
- Divisor summatory function
- Normal order of an arithmetic functionNormal order of an arithmetic functionIn number theory, the normal order of an arithmetic function is some simpler or better-understood function which "usually" takes the same or closely approximate values.Let ƒ be a function on the natural numbers...
- Extremal orders of an arithmetic function

