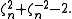CM-field
Encyclopedia
In mathematics
, a CM-field is a particular type of number field K, so named for a close connection to the theory of complex multiplication
. Another name used is J-field. Specifically, K is a quadratic extension of a totally real field which is totally imaginary, i.e. for which there is no embedding of K into .
.
In other words, there is a subfield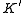 of K such that K is generated over
of K such that K is generated over  by a single square root of an element, say
by a single square root of an element, say
β =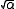 ,
,
in such a way that the minimal polynomial
of β over the rational number field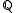 has all its roots non-real complex numbers. For this α should be chosen totally negative, so that for each embedding σ of
has all its roots non-real complex numbers. For this α should be chosen totally negative, so that for each embedding σ of 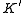 into the real number field,
into the real number field,
σ(α) < 0.
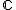 induces an automorphism on the field which is independent of the embedding into
induces an automorphism on the field which is independent of the embedding into 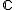 . In the notation given, it must change the sign of β.
. In the notation given, it must change the sign of β.
A number field F is a CM-field if and only if it has a "units defect", i.e. if it contains a proper subfield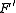 whose unit group has the same
whose unit group has the same 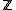 -rank as that of F. (In fact,
-rank as that of F. (In fact, 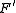 is the totally real subfield of F mentioned above.) This follows from Dirichlet's unit theorem
is the totally real subfield of F mentioned above.) This follows from Dirichlet's unit theorem
.
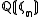 , which is generated by a primitive nth root of unity
, which is generated by a primitive nth root of unity
. It is a totally imaginary quadratic extension of the totally real field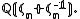
The latter is the fixed field of complex conjugation, and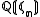 is obtained from it by adjoining a square root of
is obtained from it by adjoining a square root of 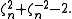
Mathematics
Mathematics is the study of quantity, space, structure, and change. Mathematicians seek out patterns and formulate new conjectures. Mathematicians resolve the truth or falsity of conjectures by mathematical proofs, which are arguments sufficient to convince other mathematicians of their validity...
, a CM-field is a particular type of number field K, so named for a close connection to the theory of complex multiplication
Complex multiplication
In mathematics, complex multiplication is the theory of elliptic curves E that have an endomorphism ring larger than the integers; and also the theory in higher dimensions of abelian varieties A having enough endomorphisms in a certain precise sense In mathematics, complex multiplication is the...
. Another name used is J-field. Specifically, K is a quadratic extension of a totally real field which is totally imaginary, i.e. for which there is no embedding of K into
 .
.In other words, there is a subfield
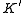 of K such that K is generated over
of K such that K is generated over  by a single square root of an element, say
by a single square root of an element, sayβ =
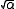 ,
,in such a way that the minimal polynomial
Minimal polynomial (field theory)
In field theory, given a field extension E / F and an element α of E that is an algebraic element over F, the minimal polynomial of α is the monic polynomial p, with coefficients in F, of least degree such that p = 0...
of β over the rational number field
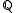 has all its roots non-real complex numbers. For this α should be chosen totally negative, so that for each embedding σ of
has all its roots non-real complex numbers. For this α should be chosen totally negative, so that for each embedding σ of 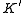 into the real number field,
into the real number field,σ(α) < 0.
Properties
One feature of a CM-field is that complex conjugation on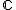 induces an automorphism on the field which is independent of the embedding into
induces an automorphism on the field which is independent of the embedding into 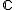 . In the notation given, it must change the sign of β.
. In the notation given, it must change the sign of β.A number field F is a CM-field if and only if it has a "units defect", i.e. if it contains a proper subfield
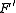 whose unit group has the same
whose unit group has the same 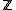 -rank as that of F. (In fact,
-rank as that of F. (In fact, 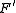 is the totally real subfield of F mentioned above.) This follows from Dirichlet's unit theorem
is the totally real subfield of F mentioned above.) This follows from Dirichlet's unit theoremDirichlet's unit theorem
In mathematics, Dirichlet's unit theorem is a basic result in algebraic number theory due to Gustav Lejeune Dirichlet. It determines the rank of the group of units in the ring OK of algebraic integers of a number field K...
.
Example
One of the most important examples of a CM-field is the cyclotomic fieldCyclotomic field
In number theory, a cyclotomic field is a number field obtained by adjoining a complex primitive root of unity to Q, the field of rational numbers...
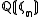 , which is generated by a primitive nth root of unity
, which is generated by a primitive nth root of unityRoot of unity
In mathematics, a root of unity, or de Moivre number, is any complex number that equals 1 when raised to some integer power n. Roots of unity are used in many branches of mathematics, and are especially important in number theory, the theory of group characters, field theory, and the discrete...
. It is a totally imaginary quadratic extension of the totally real field
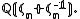
The latter is the fixed field of complex conjugation, and
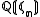 is obtained from it by adjoining a square root of
is obtained from it by adjoining a square root of