
Centered triangular number
Encyclopedia
A centered triangular number is a centered
figurate number
that represents a triangle
with a dot in the center and all other dots surrounding the center in successive triangular layers. The centered triangular number for n is given by the formula
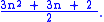
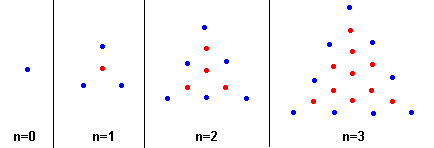
The following image shows the building of the centered triangular numbers using the associated figures: at each step the previous figure, shown in red, is surrounded by a triangle of new points, in blue.
The first few centered triangular numbers are:
Each centered triangular number from 10 onwards is the sum of three consecutive regular triangular number
s. Also each centered triangular number has a remainder of 1 when divided by three and the quotient (if positive) is the previous regular triangular number.
The sum of the first n centered triangular numbers is the magic constant
for an n by n normal magic square
for n > 2.
. The first few centered triangular primes are
(corresponding to n: 3, 4, 8, 11, 16, ...)
Centered number
The centered polygonal numbers are a class of series of figurate numbers, each formed by a central dot, surrounded by polygonal layers with a constant number of sides...
figurate number
Figurate number
The term figurate number is used by different writers for members of different sets of numbers, generalizing from triangular numbers to different shapes and different dimensions...
that represents a triangle
Triangle
A triangle is one of the basic shapes of geometry: a polygon with three corners or vertices and three sides or edges which are line segments. A triangle with vertices A, B, and C is denoted ....
with a dot in the center and all other dots surrounding the center in successive triangular layers. The centered triangular number for n is given by the formula
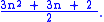
The following image shows the building of the centered triangular numbers using the associated figures: at each step the previous figure, shown in red, is surrounded by a triangle of new points, in blue.

The first few centered triangular numbers are:
- 1, 4, 1010 (number)10 is an even natural number following 9 and preceding 11.-In mathematics:Ten is a composite number, its proper divisors being , and...
, 1919 (number)19 is the natural number following 18 and preceding 20. It is a prime number.In English speech, the numbers 19 and 90 are often confused. When carefully enunciated, they differ in which syllable is stressed: 19 vs 90...
, 3131 (number)31 is the natural number following 30 and preceding 32.- In mathematics :Thirty-one is the third Mersenne prime as well as the fourth primorial prime, and together with twenty-nine, another primorial prime, it comprises a twin prime. As a Mersenne prime, 31 is related to the perfect number 496,...
, 4646 (number)46 is the natural number following 45 and preceding 47.- In mathematics :Forty-six is a Wedderburn-Etherington number, an enneagonal number and a centered triangular number. It is the sum of the totient function for the first twelve integers. 46 is the largest even integer that can't be expressed...
, 6464 (number)64 is the natural number following 63 and preceding 65.-In mathematics:Sixty-four is the square of 8, the cube of 4, and the sixth power of 2. It is the smallest number with exactly seven divisors. It is the lowest positive power of two that is adjacent to neither a Mersenne prime nor a Fermat...
, 8585 (number)85 is the natural number following 84 and preceding 86.-In mathematics:85 is an octahedral number, a centered triangular number, a centered square number, a decagonal number, and a Smith number....
, 109109 (number)109 is the natural number following 108 and preceding 110.-In mathematics:One hundred [and] nine is the 29th prime number, and also a Chen prime. The previous prime is 107, making them both twin primes...
, 136136 (number)136 is the natural number following 135 and preceding 137.-In mathematics:136 is itself a factor of the Eddington number...
, 166, 199, 235, 274, 316, 361, 409, 460, 514, 571, 631, 694, 760, 829, 901, 976, 1054, 1135, 1219, 1306, 1396, 1489, 1585, 1684, 1786, 1891, 1999, 2110, 2224, 2341, 2461, 2584, 2710, 2839, 2971, … .
Each centered triangular number from 10 onwards is the sum of three consecutive regular triangular number
Triangular number
A triangular number or triangle number numbers the objects that can form an equilateral triangle, as in the diagram on the right. The nth triangle number is the number of dots in a triangle with n dots on a side; it is the sum of the n natural numbers from 1 to n...
s. Also each centered triangular number has a remainder of 1 when divided by three and the quotient (if positive) is the previous regular triangular number.
The sum of the first n centered triangular numbers is the magic constant
Magic constant
The magic constant or magic sum of a magic square is the sum of numbers in any row, column, and diagonal of the magic square. For example, the magic square shown below has a magic constant of 15....
for an n by n normal magic square
Magic square
In recreational mathematics, a magic square of order n is an arrangement of n2 numbers, usually distinct integers, in a square, such that the n numbers in all rows, all columns, and both diagonals sum to the same constant. A normal magic square contains the integers from 1 to n2...
for n > 2.
Centered triangular prime
A centered triangular prime is a centered triangular number that is primePrime number
A prime number is a natural number greater than 1 that has no positive divisors other than 1 and itself. A natural number greater than 1 that is not a prime number is called a composite number. For example 5 is prime, as only 1 and 5 divide it, whereas 6 is composite, since it has the divisors 2...
. The first few centered triangular primes are
- 19, 31, 109, 199, 409, … .
(corresponding to n: 3, 4, 8, 11, 16, ...)

