All Topics  
Circumference

 

 
   Email Print
   Bookmark   Link
 

 

Circumference
 
 
  Topics Circumference Topic Home

The circumference is the distance around a closed curve
Curve

In mathematics, a curve consists of the points through which a continuous function moving point passes. This notion captures the intuitive idea of a geometrical dimension object, which furthermore is connectedness in the sense of having no continuous function or continuum ....
. Circumference is a kind of perimeter
Perimeter

A perimeter is a path that bounds an area. The word comes from the Greek peri and meter . The term may be used either for the path or its length....
.

circumference of a circle is the length around it. The circumference of a circle
Circle

A circle is a simple shape of Euclidean geometry consisting of those point in a plane which are the same distance from a given point called the center....
 can be calculated from its diameter
Diameter

In geometry, a diameter of a circle is any straight line segment that passes through the center of the circle and whose endpoints are on the circle....
 using the formula:

Or, substituting the diameter for the radius
RADIUS

Remote Authentication Dial In User Service is a networking protocol that provides centralized access, authorization and accounting management for people or computers to connect and use a network service....
:

where r is the radius
RADIUS

Remote Authentication Dial In User Service is a networking protocol that provides centralized access, authorization and accounting management for people or computers to connect and use a network service....
 and d is the diameter of the circle, and p (the Greek letter pi
Pi (letter)

Pi is the sixteenth letter of the Greek alphabet. In the system of Greek numerals it has a value of 80. Letters that arose from pi include Cyrillic Pe ....
) is defined
Pi

Pi or p is a mathematical constant whose value is the ratio of any circle's circumference to its diameter in Euclidean geometry; this is the same value as the ratio of a circle's area to the square of its radius....
 as the ratio of the circumference of the circle to its diameter (the numerical value of pi is 3.141 592 653 589 793...).

If desired, the above circumference formula can be derived without reference to the definition of p by using some integral calculus, as follows:

The upper half of a circle centered at the origin is the graph of the function where x runs from -r to +r.






Discussion
Ask a question about 'Circumference'
Start a new discussion about 'Circumference'
Answer questions from other users
Full Discussion Forum



Encyclopedia


The circumference is the distance around a closed curve
Curve

In mathematics, a curve consists of the points through which a continuous function moving point passes. This notion captures the intuitive idea of a geometrical dimension object, which furthermore is connectedness in the sense of having no continuous function or continuum ....
. Circumference is a kind of perimeter
Perimeter

A perimeter is a path that bounds an area. The word comes from the Greek peri and meter . The term may be used either for the path or its length....
.

Circumference of a circle

The circumference of a circle is the length around it. The circumference of a circle
Circle

A circle is a simple shape of Euclidean geometry consisting of those point in a plane which are the same distance from a given point called the center....
 can be calculated from its diameter
Diameter

In geometry, a diameter of a circle is any straight line segment that passes through the center of the circle and whose endpoints are on the circle....
 using the formula:

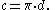
Or, substituting the diameter for the radius
RADIUS

Remote Authentication Dial In User Service is a networking protocol that provides centralized access, authorization and accounting management for people or computers to connect and use a network service....
:

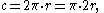
where r is the radius
RADIUS

Remote Authentication Dial In User Service is a networking protocol that provides centralized access, authorization and accounting management for people or computers to connect and use a network service....
 and d is the diameter of the circle, and p (the Greek letter pi
Pi (letter)

Pi is the sixteenth letter of the Greek alphabet. In the system of Greek numerals it has a value of 80. Letters that arose from pi include Cyrillic Pe ....
) is defined
Pi

Pi or p is a mathematical constant whose value is the ratio of any circle's circumference to its diameter in Euclidean geometry; this is the same value as the ratio of a circle's area to the square of its radius....
 as the ratio of the circumference of the circle to its diameter (the numerical value of pi is 3.141 592 653 589 793...).

If desired, the above circumference formula can be derived without reference to the definition of p by using some integral calculus, as follows:

The upper half of a circle centered at the origin is the graph of the function where x runs from -r to +r. The circumference (c) of the entire circle can be represented as twice the sum of the lengths of the infinitesimal arcs that make up this half circle. The length of a single infinitesimal part of the arc can be calculated using the Pythagorean formula
Pythagorean theorem

In mathematics, the Pythagorean theorem or Pythagoras' theorem is a relation in Euclidean geometry among the three sides of a triangle#Types of triangles....
 for the length of the hypotenuse of a rectangular triangle with side lengths dx and f'(x)dx, which gives us

Thus the circle circumference can be calculated as follows:

= =

The antiderivative
Antiderivative

In calculus, an antiderivative, primitive or indefinite integralof a function f is a function F whose derivative is equal to f, i.e., F ′ = f....
 needed to solve this definite integral is the arcsine function:

Pi (p) is the ratio of the circumference of a circle to its diameter.

Circumference of an ellipse

The circumference of an ellipse
Ellipse

In mathematics, an ellipse is the apparent shape of a circle viewed obliquely from outside it, as distinct from a hyperbola which is the shape seen from inside....
 is more problematic, as the exact solution requires finding the complete elliptic integral of the second kind. This can be achieved either via numerical integration
Numerical integration

In numerical analysis, numerical integration constitutes a broad family of algorithms for calculating the numerical value of a definite integral, and by extension, the term is also sometimes used to describe the numerical ordinary differential equations....
 (the best type being Gaussian quadrature
Gaussian quadrature

In numerical analysis, a quadrature rule is an approximation of the integral of a function , usually stated as a weighted sum of function values at specified points within the domain of integration....
) or by one of many binomial series
Binomial series

In mathematics, the binomial series generalizes the purely algebraic formula of the binomial theoremto complex values of a. It is also a special case of a Newton_series#Newton_series....
 expansions.

Where are the ellipse's semi-major
Semi-major axis

In geometry, the semi-major axis is used to describe the dimensions of ellipses and hyperbolae....
 and semi-minor
Semi-minor axis

In geometry, the semi-minor axis is a line segment associated with most conic sections . One end of the segment is the center of the conic section, and it is at right angles with the semi-major axis....
 axes, respectively, and is the ellipse's angular eccentricity
Angular eccentricity

In the study of ellipses and related geometry, various parameters in the distortion of a circle into an ellipse are identified and employed: Aspect ratio, flattening and Eccentricity ....
,

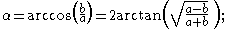
There are many different approximation
Approximation

An approximation is an Accuracy and precision representation of something that is still close enough to be useful. Although approximation is most often applied to numbers, it is also frequently applied to such things as Function , shapes, and physical laws....
s for the divided difference
Difference quotient

The primary vehicle of calculus and other higher mathematics is the Function . Its "input value" is its argument, usually a point expressible on a graph....
, with varying degrees of sophistication and corresponding accuracy.

In comparing the different approximations, the based series expansion is used to find the actual value:

Muir-1883

Probably the most accurate to its given simplicity is Thomas Muir's
Thomas Muir (mathematician)

Sir Thomas Muir was a Scotland mathematician, remembered as an authority on determinants. He was born in Stonebyres in South Lanarkshire, and brought up in the small town of Biggar, Scotland....
:


Ramanujan-1914 (#1,#2)

Srinivasa Ramanujan
Srinivasa Ramanujan

Srinivasa Ramanujan Ivengar Fellow of the Royal Society, better known as Srinivasa Ramanujan was an Indian mathematician, who, with almost no formal training in pure mathematics, made substantial contributions to mathematical analysis, number theory, infinite series and continued fractions....
 introduced two different approximations, both from 1914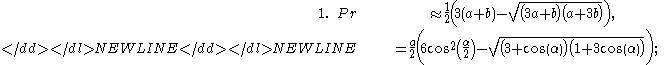




The second equation is demonstratively by far the better of the two, and may be the most accurate approximation known.


Letting a = 10000 and b = aŚcos, results with different ellipticities can be found and compared:

b Pr Ramanujan-#2 Ramanujan-#1 Muir
9975  9987.50391 11393   9987.50391 11393   9987.50391 11393   9987.50391 11389
9966  9983.00723 73047  9983.00723 73047  9983.00723 73047  9983.00723 73034
9950  9975.01566 41666  9975.01566 41666  9975.01566 41666  9975.01566 41604
9900  9950.06281 41695  9950.06281 41695  9950.06281 41695  9950.06281 40704
9000  9506.58008 71725  9506.58008 71725  9506.58008 67774  9506.57894 84209
8000  9027.79927 77219  9027.79927 77219  9027.79924 43886  9027.77786 62561
7500  8794.70009 24247  8794.70009 24240  8794.69994 52888  8794.64324 65132
6667  8417.02535 37669  8417.02535 37460  8417.02428 62059  8416.81780 56370
5000  7709.82212 59502  7709.82212 24348  7709.80054 22510  7708.38853 77837
3333  7090.18347 61693  7090.18324 21686  7089.94281 35586  7083.80287 96714
2500  6826.49114 72168  6826.48944 11189  6825.75998 22882  6814.20222 31205
1000  6468.01579 36089  6467.94103 84016  6462.57005 00576  6431.72229 28418
 100  6367.94576 97209  6366.42397 74408  6346.16560 81001  6303.80428 66621
  10  6366.22253 29150  6363.81341 42880  6340.31989 06242  6299.73805 61141
   1  6366.19804 50617  6363.65301 06191  6339.80266 34498  6299.60944 92105
iota  6366.19772 36758  6363.63636 36364  6339.74596 21556  6299.60524 94744


Circumference of a graph

In graph theory
Graph theory

In mathematics and computer science, graph theory is the study of graph : mathematical structures used to model pairwise relations between objects from a certain collection....
 the circumference of a graph
Graph (mathematics)

In mathematics a graph is an abstract representation of a set of objects where some pairs of the objects are connected by links. The interconnected objects are represented by mathematical abstractions called vertices, and the links that connect some pairs of vertices are called edges....
 refers to the longest cycle
Cycle

Cycle or Cyclic may refer to:* Motorcycle* Bicycle* Cycling, the act of riding a bicycle or tricycle* Tricycle...
 contained in that graph.

External links

  • With interactive applet and animation