Classical unified field theories
Encyclopedia
Since the 19th century, some physicists have attempted to develop a single theoretical framework that can account for the fundamental forces of nature – a unified field theory
. Classical unified field theories are attempts to create a unified field theory based on classical physics
. In particular, unification of gravitation
and electromagnetism
was actively pursued by several physicists and mathematicians in the years between World War I
and World War II
. This work spurred the purely mathematical development of differential geometry. Albert Einstein
is the best known of the many physicists who attempted to develop a classical unified field theory.
This article describes various attempts at a classical, relativistic
unified field theory. For a survey of classical relativistic field theories of gravitation that have been motivated by theoretical concerns other than unification, see Classical theories of gravitation
. For a survey of current work toward creating a quantum theory of gravitation, see quantum gravity
.
of general relativity
, and attempted to incorporate electromagnetic fields into a more general geometry, since ordinary Riemannian geometry seemed incapable of expressing the properties of the electromagnetic field. Einstein was not alone in his attempts to unify electromagnetism and gravity; a large number of mathematicians and physicists, including Hermann Weyl
, Arthur Eddington, Theodor Kaluza
, Lancelot Law Whyte
, and R. Bach also attempted to develop approaches that could unify these interactions. These scientists pursued several avenues of generalization, including extending the foundations of geometry and adding an extra spatial dimension.
, involved making the metric tensor
(which had previously been assumed to be symmetric and real-valued) into an asymmetric and/or complex-valued
tensor, and they also attempted to create a field theory for matter as well.
of Weyl, Kaluza's five-dimensional theory, Lancelot Law Whyte's theory based on the Unitary Principle and Eddington's development of affine geometry
. Einstein corresponded with these researchers, and collaborated with Kaluza, but was not yet fully involved in the unification effort.
upon which general relativity is based. His idea was to create a more general infinitesimal geometry. He noted that in addition to a metric
field there could be additional degrees of freedom along a path between two points in a manifold, and he tried to exploit this by introducing a basic method for comparison of local size measures along such a path, in terms of a gauge field. This geometry generalized Riemannian geometry in that there was a vector field
Q, in addition to the metric g, which together gave rise to both the electromagnetic and gravitational fields. This theory was mathematically sound, albeit complicated, resulting in difficult and high-order field equations. The critical mathematical ingredients in this theory, the Lagrangian
s and curvature tensor
, were worked out by Weyl and colleagues. Then Weyl carried out an extensive correspondence with Einstein and others as to its physical validity, and the theory was ultimately found to be physically unreasonable. However, Weyl's principle of gauge invariance was later applied in a modified form to quantum field theory
.
the "Unitary Principle". The history of this theoretical approach is: Michael Faraday
and James Clerk Maxwell
worked from Rudjer Boscovich's theory, which dealt with non-Euclidean and higher-dimensional geometry. This prompted mathematicians such as Gauss
and Riemann to investigate that area of mathematics. The mathematics that Riemann developed was used by Einstein in his theory of general relativity
, but that was not as extensive a description as Boscovich's theory, for which the mathematics had been only incompletely developed. Lancelot Law Whyte's ideas were adopted for experimental work by Leo Baranski, who planned a series of books based upon this theory. Only Baranski's first book was published before his death, upon which this line of investigation based upon classical physics was abandoned by academia.
was a noted astronomer who became an enthusiastic and influential promoter of Einstein's general theory of relativity. He was among the first to propose an extension of the gravitational theory based on the affine connection
as the fundamental structure field rather than the metric tensor
which was the original focus of general relativity. Affine connection is the basis for parallel transport of vectors from one space-time point to another; Eddington assumed the affine connection to be symmetric in its covariant indices, because it seemed plausible that the result of parallel-transporting one infinitesimal vector along another should produce the same result as transporting the second along the first. (Later workers revisited this assumption.)
Eddington emphasized what he considered to be epistemological considerations; for example, he thought that the cosmological constant
version of the general-relativistic field equation expressed the property that the universe was "self-gauging". Since the simplest cosmological model (the De Sitter universe
) that solves that equation is a spherically symmetric, stationary, closed universe (exhibiting a cosmological red shift
, which is more conventionally interpreted as due to expansion), it seemed to explain the overall form of the universe.
Like many other classical unified field theorists, Eddington considered that in the Einstein field equations
for general relativity the stress-energy tensor
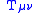, which represents matter/energy, was merely provisional, and that in a truly unified theory the source term would automatically arise as some aspect of the free-space field equations. He also shared the hope that an improved fundamental theory would explain why the two elementary particles then known (proton and electron) have quite different masses.
The Dirac equation
for the relativistic quantum electron caused Eddington to rethink his previous conviction that fundamental physical theory had to be based on tensors. He subsequently devoted his efforts into development of a "Fundamental Theory" based largely on algebraic notions (which he called "E-frames"). Unfortunately his descriptions of this theory were sketchy and difficult to understand, so very few physicists followed up on his work.
for electromagnetism is formulated within the framework of Einstein's theory of general relativity
, the electromagnetic field energy (being equivalent to mass as one would expect from Einstein's famous equation E=mc2) contributes to the stress tensor and thus to the curvature of space-time, which is the general-relativistic representation of the gravitational field; or putting it another way, certain configurations of curved space-time incorporate effects of an electromagnetic field. This suggests that a purely geometric theory ought to treat these two fields as different aspects of the same basic phenomenon. However, ordinary Riemannian geometry
is unable to describe the properties of the electromagnetic field as a purely geometric phenomenon.
Einstein tried to form a generalized theory of gravitation that would unify the gravitational and electromagnetic forces (and perhaps others), guided by a belief in a single origin for the entire set of physical laws. These attempts initially concentrated on additional geometric notions such as vierbeins and "distant parallelism", but eventually centered around treating both the metric tensor
and the affine connection
as fundamental fields. (Because they are not independent, the metric-affine theory was somewhat complicated.) In general relativity, these fields are symmetric (in the matrix sense), but since antisymmetry seemed essential for electromagnetism, the symmetry requirement was relaxed for one or both fields. Einstein's proposed unified-field equations (fundamental laws of physics) were generally derived from a variational principle
expressed in terms of the Riemann curvature tensor
for the presumed space-time manifold
.
In field theories of this kind, particles appear as limited regions in space-time in which the field strength or the energy density are particularly high. Einstein and coworker Leopold Infeld
managed to demonstrate that, in Einstein's final theory of the unified field, true singularities
of the field did have trajectories resembling point particles. However, singularities are places where the equations break down, and Einstein believed that in an ultimate theory the laws should apply everywhere, with particles being soliton
-like solutions to the (highly nonlinear) field equations. Further, the large-scale topology of the universe should impose restrictions on the solutions, such as quantization or discrete symmetries.
The degree of abstraction, combined with a relative lack of good mathematical tools for analyzing nonlinear equation systems, make it hard to connect such theories with the physical phenomena that they might describe. For example, it has been suggested that the torsion (antisymmetric part of the affine connection) might be related to isospin
rather than electromagnetism; this is related to a discrete (or "internal") symmetry known to Einstein as "displacement field duality".
Einstein became increasingly isolated in his research on a generalized theory of gravitation, and most physicists consider his attempts ultimately unsuccessful. In particular, his pursuit of a unification of the fundamental forces ignored developments in quantum physics (and vice versa), most notably the discovery of the strong nuclear force and weak nuclear force.
as the sole basis for differential geometric
structure for space-time, Erwin Schrödinger
from 1940 to 1951 thoroughly investigated pure-affine formulations of generalized gravitational theory. Although he initially assumed a symmetric affine connection, like Einstein he later considered the nonsymmetric field.
Schrödinger's most striking discovery during this work was that the metric tensor
was induced upon the manifold
via a simple construction from the Riemann curvature tensor
, which was in turn formed entirely from the affine connection. Further, taking this approach with the simplest feasible basis for the variational principle
resulted in a field equation having the form of Einstein's general-relativistic field equation with a cosmological term
arising automatically.
Skepticism from Einstein and published criticisms from other physicists discouraged Schrödinger, and his work in this area has been largely ignored.
Most scientists, though not Einstein, eventually abandoned classical theories. Current mainstream research on unified field theories
focuses on the problem of creating quantum gravity
and unifying such a theory with the other fundamental theories in physics, which are quantum theories. (Some programs, most notably string theory
, attempt to solve both of these problems at once.) With four fundamental forces now identified, gravity remains the one force whose unification proves problematic.
Although new "classical" unified field theories continue to be proposed from time to time, often involving non-traditional elements such as spinor
s, none has been generally accepted by physicists.
Unified field theory
In physics, a unified field theory, occasionally referred to as a uniform field theory, is a type of field theory that allows all that is usually thought of as fundamental forces and elementary particles to be written in terms of a single field. There is no accepted unified field theory, and thus...
. Classical unified field theories are attempts to create a unified field theory based on classical physics
Classical physics
What "classical physics" refers to depends on the context. When discussing special relativity, it refers to the Newtonian physics which preceded relativity, i.e. the branches of physics based on principles developed before the rise of relativity and quantum mechanics...
. In particular, unification of gravitation
Gravitation
Gravitation, or gravity, is a natural phenomenon by which physical bodies attract with a force proportional to their mass. Gravitation is most familiar as the agent that gives weight to objects with mass and causes them to fall to the ground when dropped...
and electromagnetism
Electromagnetism
Electromagnetism is one of the four fundamental interactions in nature. The other three are the strong interaction, the weak interaction and gravitation...
was actively pursued by several physicists and mathematicians in the years between World War I
World War I
World War I , which was predominantly called the World War or the Great War from its occurrence until 1939, and the First World War or World War I thereafter, was a major war centred in Europe that began on 28 July 1914 and lasted until 11 November 1918...
and World War II
World War II
World War II, or the Second World War , was a global conflict lasting from 1939 to 1945, involving most of the world's nations—including all of the great powers—eventually forming two opposing military alliances: the Allies and the Axis...
. This work spurred the purely mathematical development of differential geometry. Albert Einstein
Albert Einstein
Albert Einstein was a German-born theoretical physicist who developed the theory of general relativity, effecting a revolution in physics. For this achievement, Einstein is often regarded as the father of modern physics and one of the most prolific intellects in human history...
is the best known of the many physicists who attempted to develop a classical unified field theory.
This article describes various attempts at a classical, relativistic
Theory of relativity
The theory of relativity, or simply relativity, encompasses two theories of Albert Einstein: special relativity and general relativity. However, the word relativity is sometimes used in reference to Galilean invariance....
unified field theory. For a survey of classical relativistic field theories of gravitation that have been motivated by theoretical concerns other than unification, see Classical theories of gravitation
Classical theories of gravitation
The current Gold Standard Theory of Gravitation is the general theory of relativity. This is a classical, relativistic field theory of gravitation...
. For a survey of current work toward creating a quantum theory of gravitation, see quantum gravity
Quantum gravity
Quantum gravity is the field of theoretical physics which attempts to develop scientific models that unify quantum mechanics with general relativity...
.
Overview
The early attempts at creating a unified field theory began with the Riemannian geometryRiemannian geometry
Riemannian geometry is the branch of differential geometry that studies Riemannian manifolds, smooth manifolds with a Riemannian metric, i.e. with an inner product on the tangent space at each point which varies smoothly from point to point. This gives, in particular, local notions of angle, length...
of general relativity
General relativity
General relativity or the general theory of relativity is the geometric theory of gravitation published by Albert Einstein in 1916. It is the current description of gravitation in modern physics...
, and attempted to incorporate electromagnetic fields into a more general geometry, since ordinary Riemannian geometry seemed incapable of expressing the properties of the electromagnetic field. Einstein was not alone in his attempts to unify electromagnetism and gravity; a large number of mathematicians and physicists, including Hermann Weyl
Hermann Weyl
Hermann Klaus Hugo Weyl was a German mathematician and theoretical physicist. Although much of his working life was spent in Zürich, Switzerland and then Princeton, he is associated with the University of Göttingen tradition of mathematics, represented by David Hilbert and Hermann Minkowski.His...
, Arthur Eddington, Theodor Kaluza
Theodor Kaluza
Theodor Franz Eduard Kaluza was a German mathematician and physicist known for the Kaluza-Klein theory involving field equations in five-dimensional space...
, Lancelot Law Whyte
Lancelot Law Whyte
Lancelot Law Whyte was a Scottish financier and industrial engineer.He claimed to have worked with Albert Einstein on the unified field theory. He further claimed that this work was based on the theory of the 18th century natural philosopher Roger Boscovich.Whyte proposed something he called "the...
, and R. Bach also attempted to develop approaches that could unify these interactions. These scientists pursued several avenues of generalization, including extending the foundations of geometry and adding an extra spatial dimension.
Early work
The first attempts to provide a unified theory were by G. Mie in 1912 and Ernst Reichenbacher in 1916. However, these theories were unsatisfactory, as they did not incorporate general relativity – in the former case, because general relativity had yet to be formulated. These efforts, along with those of ForsterForster
Forster may refer to:* Forster, New South Wales, a town in Australia* Forster , several people with the surname* Forster Music Publisher, Inc., a sheet music publisher founded in 1916 based in Chicago...
, involved making the metric tensor
Metric tensor
In the mathematical field of differential geometry, a metric tensor is a type of function defined on a manifold which takes as input a pair of tangent vectors v and w and produces a real number g in a way that generalizes many of the familiar properties of the dot product of vectors in Euclidean...
(which had previously been assumed to be symmetric and real-valued) into an asymmetric and/or complex-valued
Complex number
A complex number is a number consisting of a real part and an imaginary part. Complex numbers extend the idea of the one-dimensional number line to the two-dimensional complex plane by using the number line for the real part and adding a vertical axis to plot the imaginary part...
tensor, and they also attempted to create a field theory for matter as well.
Differential geometry and field theory
From 1918 until 1923, there were four distinct approaches to field theory: the gauge theoryGauge theory
In physics, gauge invariance is the property of a field theory in which different configurations of the underlying fundamental but unobservable fields result in identical observable quantities. A theory with such a property is called a gauge theory...
of Weyl, Kaluza's five-dimensional theory, Lancelot Law Whyte's theory based on the Unitary Principle and Eddington's development of affine geometry
Affine geometry
In mathematics affine geometry is the study of geometric properties which remain unchanged by affine transformations, i.e. non-singular linear transformations and translations...
. Einstein corresponded with these researchers, and collaborated with Kaluza, but was not yet fully involved in the unification effort.
Weyl's infinitesimal geometry
In order to include electromagnetism into the geometry of general relativity, Hermann Weyl worked to generalize the Riemannian geometryRiemannian geometry
Riemannian geometry is the branch of differential geometry that studies Riemannian manifolds, smooth manifolds with a Riemannian metric, i.e. with an inner product on the tangent space at each point which varies smoothly from point to point. This gives, in particular, local notions of angle, length...
upon which general relativity is based. His idea was to create a more general infinitesimal geometry. He noted that in addition to a metric
Metric tensor
In the mathematical field of differential geometry, a metric tensor is a type of function defined on a manifold which takes as input a pair of tangent vectors v and w and produces a real number g in a way that generalizes many of the familiar properties of the dot product of vectors in Euclidean...
field there could be additional degrees of freedom along a path between two points in a manifold, and he tried to exploit this by introducing a basic method for comparison of local size measures along such a path, in terms of a gauge field. This geometry generalized Riemannian geometry in that there was a vector field
Vector field
In vector calculus, a vector field is an assignmentof a vector to each point in a subset of Euclidean space. A vector field in the plane for instance can be visualized as an arrow, with a given magnitude and direction, attached to each point in the plane...
Q, in addition to the metric g, which together gave rise to both the electromagnetic and gravitational fields. This theory was mathematically sound, albeit complicated, resulting in difficult and high-order field equations. The critical mathematical ingredients in this theory, the Lagrangian
Lagrangian
The Lagrangian, L, of a dynamical system is a function that summarizes the dynamics of the system. It is named after Joseph Louis Lagrange. The concept of a Lagrangian was originally introduced in a reformulation of classical mechanics by Irish mathematician William Rowan Hamilton known as...
s and curvature tensor
Curvature tensor
In differential geometry, the term curvature tensor may refer to:* the Riemann curvature tensor of a Riemannian manifold — see also Curvature of Riemannian manifolds;* the curvature of an affine connection or covariant derivative ;...
, were worked out by Weyl and colleagues. Then Weyl carried out an extensive correspondence with Einstein and others as to its physical validity, and the theory was ultimately found to be physically unreasonable. However, Weyl's principle of gauge invariance was later applied in a modified form to quantum field theory
Quantum field theory
Quantum field theory provides a theoretical framework for constructing quantum mechanical models of systems classically parametrized by an infinite number of dynamical degrees of freedom, that is, fields and many-body systems. It is the natural and quantitative language of particle physics and...
.
Kaluza's fifth dimension
Kaluza's approach to unification was to embed space-time into a five-dimensional cylindrical world; one of four space dimensions and one of time. Unlike Weyl's approach, Riemannian geometry was maintained, and the extra dimension allowed for the incorporation of the electromagnetic field vector into the geometry. Despite the relative mathematical elegance of this approach, in collaboration with Einstein and Einstein's aide Grommer it was determined that this theory did not admit a non-singular, static, spherically symmetric solution. This theory did have some influence on Einstein's later work and was further developed later by Klein in an attempt to incorporate relativity into quantum theory, in what is now known as Kaluza-Klein theory.Lancelot Law Whyte's unitary field theory
This theory was based on an organizing process called by Lancelot Law WhyteLancelot Law Whyte
Lancelot Law Whyte was a Scottish financier and industrial engineer.He claimed to have worked with Albert Einstein on the unified field theory. He further claimed that this work was based on the theory of the 18th century natural philosopher Roger Boscovich.Whyte proposed something he called "the...
the "Unitary Principle". The history of this theoretical approach is: Michael Faraday
Michael Faraday
Michael Faraday, FRS was an English chemist and physicist who contributed to the fields of electromagnetism and electrochemistry....
and James Clerk Maxwell
James Clerk Maxwell
James Clerk Maxwell of Glenlair was a Scottish physicist and mathematician. His most prominent achievement was formulating classical electromagnetic theory. This united all previously unrelated observations, experiments and equations of electricity, magnetism and optics into a consistent theory...
worked from Rudjer Boscovich's theory, which dealt with non-Euclidean and higher-dimensional geometry. This prompted mathematicians such as Gauss
Carl Friedrich Gauss
Johann Carl Friedrich Gauss was a German mathematician and scientist who contributed significantly to many fields, including number theory, statistics, analysis, differential geometry, geodesy, geophysics, electrostatics, astronomy and optics.Sometimes referred to as the Princeps mathematicorum...
and Riemann to investigate that area of mathematics. The mathematics that Riemann developed was used by Einstein in his theory of general relativity
General relativity
General relativity or the general theory of relativity is the geometric theory of gravitation published by Albert Einstein in 1916. It is the current description of gravitation in modern physics...
, but that was not as extensive a description as Boscovich's theory, for which the mathematics had been only incompletely developed. Lancelot Law Whyte's ideas were adopted for experimental work by Leo Baranski, who planned a series of books based upon this theory. Only Baranski's first book was published before his death, upon which this line of investigation based upon classical physics was abandoned by academia.
Eddington's affine geometry
Sir Arthur Stanley EddingtonArthur Stanley Eddington
Sir Arthur Stanley Eddington, OM, FRS was a British astrophysicist of the early 20th century. He was also a philosopher of science and a popularizer of science...
was a noted astronomer who became an enthusiastic and influential promoter of Einstein's general theory of relativity. He was among the first to propose an extension of the gravitational theory based on the affine connection
Affine connection
In the branch of mathematics called differential geometry, an affine connection is a geometrical object on a smooth manifold which connects nearby tangent spaces, and so permits tangent vector fields to be differentiated as if they were functions on the manifold with values in a fixed vector space...
as the fundamental structure field rather than the metric tensor
Metric tensor
In the mathematical field of differential geometry, a metric tensor is a type of function defined on a manifold which takes as input a pair of tangent vectors v and w and produces a real number g in a way that generalizes many of the familiar properties of the dot product of vectors in Euclidean...
which was the original focus of general relativity. Affine connection is the basis for parallel transport of vectors from one space-time point to another; Eddington assumed the affine connection to be symmetric in its covariant indices, because it seemed plausible that the result of parallel-transporting one infinitesimal vector along another should produce the same result as transporting the second along the first. (Later workers revisited this assumption.)
Eddington emphasized what he considered to be epistemological considerations; for example, he thought that the cosmological constant
Cosmological constant
In physical cosmology, the cosmological constant was proposed by Albert Einstein as a modification of his original theory of general relativity to achieve a stationary universe...
version of the general-relativistic field equation expressed the property that the universe was "self-gauging". Since the simplest cosmological model (the De Sitter universe
De Sitter universe
A de Sitter universe is a cosmological solution to Einstein's field equations of General Relativity which is named after Willem de Sitter. It models the universe as spatially flat and neglects ordinary matter, so the dynamics of the universe are dominated by the cosmological constant, thought to...
) that solves that equation is a spherically symmetric, stationary, closed universe (exhibiting a cosmological red shift
Red shift
-Science:* Redshift, the increase of wavelength of detected electromagnetic radiation with respect to the original wavelength of the emission* Red shift, an informal term for a bathochromic shift...
, which is more conventionally interpreted as due to expansion), it seemed to explain the overall form of the universe.
Like many other classical unified field theorists, Eddington considered that in the Einstein field equations
Einstein field equations
The Einstein field equations or Einstein's equations are a set of ten equations in Albert Einstein's general theory of relativity which describe the fundamental interaction of gravitation as a result of spacetime being curved by matter and energy...
for general relativity the stress-energy tensor
Stress-energy tensor
The stress–energy tensor is a tensor quantity in physics that describes the density and flux of energy and momentum in spacetime, generalizing the stress tensor of Newtonian physics. It is an attribute of matter, radiation, and non-gravitational force fields...
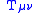, which represents matter/energy, was merely provisional, and that in a truly unified theory the source term would automatically arise as some aspect of the free-space field equations. He also shared the hope that an improved fundamental theory would explain why the two elementary particles then known (proton and electron) have quite different masses.The Dirac equation
Dirac equation
The Dirac equation is a relativistic quantum mechanical wave equation formulated by British physicist Paul Dirac in 1928. It provided a description of elementary spin-½ particles, such as electrons, consistent with both the principles of quantum mechanics and the theory of special relativity, and...
for the relativistic quantum electron caused Eddington to rethink his previous conviction that fundamental physical theory had to be based on tensors. He subsequently devoted his efforts into development of a "Fundamental Theory" based largely on algebraic notions (which he called "E-frames"). Unfortunately his descriptions of this theory were sketchy and difficult to understand, so very few physicists followed up on his work.
Einstein's geometric approaches
When the equivalent of Maxwell's equationsMaxwell's equations
Maxwell's equations are a set of partial differential equations that, together with the Lorentz force law, form the foundation of classical electrodynamics, classical optics, and electric circuits. These fields in turn underlie modern electrical and communications technologies.Maxwell's equations...
for electromagnetism is formulated within the framework of Einstein's theory of general relativity
General relativity
General relativity or the general theory of relativity is the geometric theory of gravitation published by Albert Einstein in 1916. It is the current description of gravitation in modern physics...
, the electromagnetic field energy (being equivalent to mass as one would expect from Einstein's famous equation E=mc2) contributes to the stress tensor and thus to the curvature of space-time, which is the general-relativistic representation of the gravitational field; or putting it another way, certain configurations of curved space-time incorporate effects of an electromagnetic field. This suggests that a purely geometric theory ought to treat these two fields as different aspects of the same basic phenomenon. However, ordinary Riemannian geometry
Riemannian geometry
Riemannian geometry is the branch of differential geometry that studies Riemannian manifolds, smooth manifolds with a Riemannian metric, i.e. with an inner product on the tangent space at each point which varies smoothly from point to point. This gives, in particular, local notions of angle, length...
is unable to describe the properties of the electromagnetic field as a purely geometric phenomenon.
Einstein tried to form a generalized theory of gravitation that would unify the gravitational and electromagnetic forces (and perhaps others), guided by a belief in a single origin for the entire set of physical laws. These attempts initially concentrated on additional geometric notions such as vierbeins and "distant parallelism", but eventually centered around treating both the metric tensor
Metric tensor
In the mathematical field of differential geometry, a metric tensor is a type of function defined on a manifold which takes as input a pair of tangent vectors v and w and produces a real number g in a way that generalizes many of the familiar properties of the dot product of vectors in Euclidean...
and the affine connection
Affine connection
In the branch of mathematics called differential geometry, an affine connection is a geometrical object on a smooth manifold which connects nearby tangent spaces, and so permits tangent vector fields to be differentiated as if they were functions on the manifold with values in a fixed vector space...
as fundamental fields. (Because they are not independent, the metric-affine theory was somewhat complicated.) In general relativity, these fields are symmetric (in the matrix sense), but since antisymmetry seemed essential for electromagnetism, the symmetry requirement was relaxed for one or both fields. Einstein's proposed unified-field equations (fundamental laws of physics) were generally derived from a variational principle
Variational principle
A variational principle is a scientific principle used within the calculus of variations, which develops general methods for finding functions which minimize or maximize the value of quantities that depend upon those functions...
expressed in terms of the Riemann curvature tensor
Riemann curvature tensor
In the mathematical field of differential geometry, the Riemann curvature tensor, or Riemann–Christoffel tensor after Bernhard Riemann and Elwin Bruno Christoffel, is the most standard way to express curvature of Riemannian manifolds...
for the presumed space-time manifold
Manifold
In mathematics , a manifold is a topological space that on a small enough scale resembles the Euclidean space of a specific dimension, called the dimension of the manifold....
.
In field theories of this kind, particles appear as limited regions in space-time in which the field strength or the energy density are particularly high. Einstein and coworker Leopold Infeld
Leopold Infeld
Leopold Infeld was a Polish physicist who worked mainly in Poland and Canada . He was a Rockefeller fellow at Cambridge University and a member of the Polish Academy of Sciences....
managed to demonstrate that, in Einstein's final theory of the unified field, true singularities
Mathematical singularity
In mathematics, a singularity is in general a point at which a given mathematical object is not defined, or a point of an exceptional set where it fails to be well-behaved in some particular way, such as differentiability...
of the field did have trajectories resembling point particles. However, singularities are places where the equations break down, and Einstein believed that in an ultimate theory the laws should apply everywhere, with particles being soliton
Soliton
In mathematics and physics, a soliton is a self-reinforcing solitary wave that maintains its shape while it travels at constant speed. Solitons are caused by a cancellation of nonlinear and dispersive effects in the medium...
-like solutions to the (highly nonlinear) field equations. Further, the large-scale topology of the universe should impose restrictions on the solutions, such as quantization or discrete symmetries.
The degree of abstraction, combined with a relative lack of good mathematical tools for analyzing nonlinear equation systems, make it hard to connect such theories with the physical phenomena that they might describe. For example, it has been suggested that the torsion (antisymmetric part of the affine connection) might be related to isospin
Isospin
In physics, and specifically, particle physics, isospin is a quantum number related to the strong interaction. This term was derived from isotopic spin, but the term is confusing as two isotopes of a nucleus have different numbers of nucleons; in contrast, rotations of isospin maintain the number...
rather than electromagnetism; this is related to a discrete (or "internal") symmetry known to Einstein as "displacement field duality".
Einstein became increasingly isolated in his research on a generalized theory of gravitation, and most physicists consider his attempts ultimately unsuccessful. In particular, his pursuit of a unification of the fundamental forces ignored developments in quantum physics (and vice versa), most notably the discovery of the strong nuclear force and weak nuclear force.
Schrödinger's pure-affine theory
Inspired by Einstein's approach to a unified field theory and Eddington's idea of the affine connectionAffine connection
In the branch of mathematics called differential geometry, an affine connection is a geometrical object on a smooth manifold which connects nearby tangent spaces, and so permits tangent vector fields to be differentiated as if they were functions on the manifold with values in a fixed vector space...
as the sole basis for differential geometric
Differential geometry and topology
Differential geometry is a mathematical discipline that uses the techniques of differential and integral calculus, as well as linear and multilinear algebra, to study problems in geometry. The theory of plane and space curves and of surfaces in the three-dimensional Euclidean space formed the basis...
structure for space-time, Erwin Schrödinger
Erwin Schrödinger
Erwin Rudolf Josef Alexander Schrödinger was an Austrian physicist and theoretical biologist who was one of the fathers of quantum mechanics, and is famed for a number of important contributions to physics, especially the Schrödinger equation, for which he received the Nobel Prize in Physics in 1933...
from 1940 to 1951 thoroughly investigated pure-affine formulations of generalized gravitational theory. Although he initially assumed a symmetric affine connection, like Einstein he later considered the nonsymmetric field.
Schrödinger's most striking discovery during this work was that the metric tensor
Metric tensor
In the mathematical field of differential geometry, a metric tensor is a type of function defined on a manifold which takes as input a pair of tangent vectors v and w and produces a real number g in a way that generalizes many of the familiar properties of the dot product of vectors in Euclidean...
was induced upon the manifold
Manifold
In mathematics , a manifold is a topological space that on a small enough scale resembles the Euclidean space of a specific dimension, called the dimension of the manifold....
via a simple construction from the Riemann curvature tensor
Riemann curvature tensor
In the mathematical field of differential geometry, the Riemann curvature tensor, or Riemann–Christoffel tensor after Bernhard Riemann and Elwin Bruno Christoffel, is the most standard way to express curvature of Riemannian manifolds...
, which was in turn formed entirely from the affine connection. Further, taking this approach with the simplest feasible basis for the variational principle
Variational principle
A variational principle is a scientific principle used within the calculus of variations, which develops general methods for finding functions which minimize or maximize the value of quantities that depend upon those functions...
resulted in a field equation having the form of Einstein's general-relativistic field equation with a cosmological term
Cosmological constant
In physical cosmology, the cosmological constant was proposed by Albert Einstein as a modification of his original theory of general relativity to achieve a stationary universe...
arising automatically.
Skepticism from Einstein and published criticisms from other physicists discouraged Schrödinger, and his work in this area has been largely ignored.
Later work
After the 1930s, progressively fewer scientists worked on classical unification, due to the continual development of quantum theory and the difficulties encountered in developing a quantum theory of gravity. Einstein continued to work on unified field theories of gravity and electromagnetism, but he became increasingly isolated in this research, which he pursued until his death. Despite the publicity of this work due to Einstein's celebrity status, it never resulted in a resounding success.Most scientists, though not Einstein, eventually abandoned classical theories. Current mainstream research on unified field theories
Unified field theory
In physics, a unified field theory, occasionally referred to as a uniform field theory, is a type of field theory that allows all that is usually thought of as fundamental forces and elementary particles to be written in terms of a single field. There is no accepted unified field theory, and thus...
focuses on the problem of creating quantum gravity
Quantum gravity
Quantum gravity is the field of theoretical physics which attempts to develop scientific models that unify quantum mechanics with general relativity...
and unifying such a theory with the other fundamental theories in physics, which are quantum theories. (Some programs, most notably string theory
String theory
String theory is an active research framework in particle physics that attempts to reconcile quantum mechanics and general relativity. It is a contender for a theory of everything , a manner of describing the known fundamental forces and matter in a mathematically complete system...
, attempt to solve both of these problems at once.) With four fundamental forces now identified, gravity remains the one force whose unification proves problematic.
Although new "classical" unified field theories continue to be proposed from time to time, often involving non-traditional elements such as spinor
Spinor
In mathematics and physics, in particular in the theory of the orthogonal groups , spinors are elements of a complex vector space introduced to expand the notion of spatial vector. Unlike tensors, the space of spinors cannot be built up in a unique and natural way from spatial vectors...
s, none has been generally accepted by physicists.