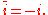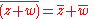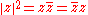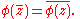Complex conjugate - AbsoluteAstronomy.com

Mathematics

Mathematics is the study of quantity, space, structure, and change. Mathematicians seek out patterns and formulate new conjectures. Mathematicians resolve the truth or falsity of conjectures by mathematical proofs, which are arguments sufficient to convince other mathematicians of their validity...

, complex conjugates are a pair of complex number

Complex number

A complex number is a number consisting of a real part and an imaginary part. Complex numbers extend the idea of the one-dimensional number line to the two-dimensional complex plane by using the number line for the real part and adding a vertical axis to plot the imaginary part...

s, both having the same real

Real number

In mathematics, a real number is a value that represents a quantity along a continuum, such as -5 , 4/3 , 8.6 , √2 and π...

part, but with imaginary

Imaginary number

An imaginary number is any number whose square is a real number less than zero. When any real number is squared, the result is never negative, but the square of an imaginary number is always negative...

parts of equal magnitude and opposite sign

Sign (mathematics)

In mathematics, the word sign refers to the property of being positive or negative. Every nonzero real number is either positive or negative, and therefore has a sign. Zero itself is signless, although in some contexts it makes sense to consider a signed zero...

s. For example, 3 + 4i and 3 − 4i are complex conjugates.

The conjugate of the complex number

where

and

are real number

Real number

In mathematics, a real number is a value that represents a quantity along a continuum, such as -5 , 4/3 , 8.6 , √2 and π...

s, is

For example,

An alternative notation for the complex conjugate is

. However, the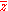

notation avoids confusion with the notation for the conjugate transpose

Conjugate transpose

In mathematics, the conjugate transpose, Hermitian transpose, Hermitian conjugate, or adjoint matrix of an m-by-n matrix A with complex entries is the n-by-m matrix A* obtained from A by taking the transpose and then taking the complex conjugate of each entry...

of a matrix

Matrix (mathematics)

In mathematics, a matrix is a rectangular array of numbers, symbols, or expressions. The individual items in a matrix are called its elements or entries. An example of a matrix with six elements isMatrices of the same size can be added or subtracted element by element...

, which can be thought of as a generalization of complex conjugation. The star-notation is preferred in physics

Physics

Physics is a natural science that involves the study of matter and its motion through spacetime, along with related concepts such as energy and force. More broadly, it is the general analysis of nature, conducted in order to understand how the universe behaves.Physics is one of the oldest academic...

while the bar-notation is more common in pure mathematics

Mathematics

. If a complex number is represented as a 2×2 matrix, the notations are identical.

Complex numbers are considered points in the complex plane

Complex plane

In mathematics, the complex plane or z-plane is a geometric representation of the complex numbers established by the real axis and the orthogonal imaginary axis...

, a variation of the Cartesian coordinate system

Cartesian coordinate system

A Cartesian coordinate system specifies each point uniquely in a plane by a pair of numerical coordinates, which are the signed distances from the point to two fixed perpendicular directed lines, measured in the same unit of length...

where both axes are real number lines that cross at the origin, however, the

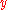-axis is a product of real numbers multiplied by +/-

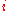. On the illustration, the

-axis is called the real axis, labeled Re, while the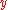

-axis is called the imaginary axis, labeled Im. The plane defined by the Re and Im axes represents the space of all possible complex numbers. In this view, complex conjugation corresponds to reflection of a complex number at the x-axis, equivalent to a

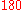degree rotation of the complex plane about the Re axis.

In polar form, the conjugate of

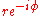. This can be shown using Euler's formula

Euler's formula

Euler's formula, named after Leonhard Euler, is a mathematical formula in complex analysis that establishes the deep relationship between the trigonometric functions and the complex exponential function...

Pairs of complex conjugates are significant because the imaginary unit

Imaginary unit

In mathematics, the imaginary unit allows the real number system ℝ to be extended to the complex number system ℂ, which in turn provides at least one root for every polynomial . The imaginary unit is denoted by , , or the Greek...

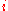is qualitatively indistinct from its additive and multiplicative inverse

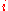, as they both satisfy the definition for the imaginary unit:

. Thus in most "natural" settings, if a complex number provides a solution to a problem, so does its conjugate, such as is the case for complex solutions of the quadratic formula with real coefficients.

Properties

These properties apply for all complex numbers z and w, unless stated otherwise, and can be easily proven by writing z and w in the form a + ib.

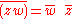(note the reversed arguments if z and w don't commute)

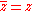if and only if z is real

for any integer n

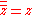, involution (i.e., the conjugate of the conjugate of a complex number z is again that number)

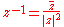if z is non-zero

The latter formula is the method of choice to compute the inverse of a complex number if it is given in rectangular coordinates.

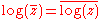if z is non-zero

In general, if

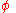is a holomorphic function

Holomorphic function

In mathematics, holomorphic functions are the central objects of study in complex analysis. A holomorphic function is a complex-valued function of one or more complex variables that is complex differentiable in a neighborhood of every point in its domain...

whose restriction to the real numbers is real-valued, and

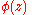is defined, then

Consequently, if

is a polynomial

Polynomial

In mathematics, a polynomial is an expression of finite length constructed from variables and constants, using only the operations of addition, subtraction, multiplication, and non-negative integer exponents...

with real

Real number

In mathematics, a real number is a value that represents a quantity along a continuum, such as -5 , 4/3 , 8.6 , √2 and π...

coefficients, and

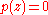, then

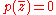as well. Thus, non-real roots of real polynomials occur in complex conjugate pairs (see Complex conjugate root theorem

Complex conjugate root theorem

In mathematics, the complex conjugate root theorem states that if P is a polynomial in one variable with real coefficients, and a + bi is a root of P with a and b real numbers, then its complex conjugate a − bi is also a root of P.It follows from this , that if the degree...

).

The map

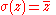from

is a homeomorphism

Homeomorphism

In the mathematical field of topology, a homeomorphism or topological isomorphism or bicontinuous function is a continuous function between topological spaces that has a continuous inverse function. Homeomorphisms are the isomorphisms in the category of topological spaces—that is, they are...

(where the topology on

is taken to be the standard topology) and antilinear, if one considers

as a complex vector space

Vector space

A vector space is a mathematical structure formed by a collection of vectors: objects that may be added together and multiplied by numbers, called scalars in this context. Scalars are often taken to be real numbers, but one may also consider vector spaces with scalar multiplication by complex...

over itself. Even though it appears to be a well-behaved

Well-behaved

Mathematicians very frequently speak of whether a mathematical object — a function, a set, a space of one sort or another — is "well-behaved" or not. The term has no fixed formal definition, and is dependent on mathematical interests, fashion, and taste...

function, it is not holomorphic; it reverses orientation whereas holomorphic functions locally preserve orientation. It is bijective and compatible with the arithmetical operations, and hence is a field

Field (mathematics)

In abstract algebra, a field is a commutative ring whose nonzero elements form a group under multiplication. As such it is an algebraic structure with notions of addition, subtraction, multiplication, and division, satisfying certain axioms...

automorphism

Automorphism

In mathematics, an automorphism is an isomorphism from a mathematical object to itself. It is, in some sense, a symmetry of the object, and a way of mapping the object to itself while preserving all of its structure. The set of all automorphisms of an object forms a group, called the automorphism...

. As it keeps the real numbers fixed, it is an element of the Galois group

Galois group

In mathematics, more specifically in the area of modern algebra known as Galois theory, the Galois group of a certain type of field extension is a specific group associated with the field extension...

of the field extension

Field extension

In abstract algebra, field extensions are the main object of study in field theory. The general idea is to start with a base field and construct in some manner a larger field which contains the base field and satisfies additional properties...

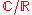. This Galois group has only two elements:

and the identity on

. Thus the only two field automorphisms of

that leave the real numbers fixed are the identity map and complex conjugation.

Use as a variable

Once a complex number

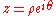is given, its conjugate is sufficient to reproduce the parts of the z-variable:

Thus the pair of variables

and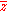

also serve up the plane as do x,y 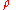and

and

. Furthermore, the

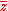variable is useful in specifying lines in the plane:

is a line through the origin and perpendicular to

since the real part of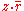

is zero only when the cosine of the angle between

and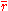

is zero. Similarly, for a fixed complex unit u = exp(b i), the equation:

determines the line through

in the direction of u.

These uses of the conjugate of z as a variable are illustrated in Frank Morley

Frank Morley

Frank Morley was a leading mathematician, known mostly for his teaching and research in the fields of algebra and geometry...

's book Inversive Geometry (1933), written with his son Frank Vigor Morley.

Generalizations

The other planar real algebras, dual numbers, and split-complex number

Split-complex number

In abstract algebra, the split-complex numbers are a two-dimensional commutative algebra over the real numbers different from the complex numbers. Every split-complex number has the formwhere x and y are real numbers...

s are also explicated by use of complex conjugation.

For matrices of complex numbers

.

Taking the conjugate transpose

Conjugate transpose

(or adjoint) of complex matrices

Matrix (mathematics)

generalizes complex conjugation. Even more general is the concept of adjoint operator for operators on (possibly infinite-dimensional) complex Hilbert space

Hilbert space

The mathematical concept of a Hilbert space, named after David Hilbert, generalizes the notion of Euclidean space. It extends the methods of vector algebra and calculus from the two-dimensional Euclidean plane and three-dimensional space to spaces with any finite or infinite number of dimensions...

s. All this is subsumed by the *-operations of C*-algebras.

One may also define a conjugation for quaternion

Quaternion

In mathematics, the quaternions are a number system that extends the complex numbers. They were first described by Irish mathematician Sir William Rowan Hamilton in 1843 and applied to mechanics in three-dimensional space...

s and coquaternion

Coquaternion

In abstract algebra, the split-quaternions or coquaternions are elements of a 4-dimensional associative algebra introduced by James Cockle in 1849 under the latter name. Like the quaternions introduced by Hamilton in 1843, they form a four dimensional real vector space equipped with a...

s: the conjugate of

.

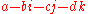Note that all these generalizations are multiplicative only if the factors are reversed:

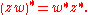
Since the multiplication of planar real algebras is commutative, this reversal is not needed there.

There is also an abstract notion of conjugation for vector spaces

over the complex number

Complex number

s. In this context,
any antilinear map

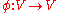that satisfies

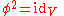, where and is the identity map
Identity map
An identity map is a database access design pattern used to improve performance by providing a context-specific in-memory cache to prevent duplicate retrieval of the same object data from the database....

on ,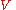
for all , , 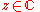and
for all , ,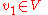

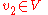is called a complex conjugation, or a real structure

Real structure

In mathematics, a real structure on a complex vector space is a way to decompose the complex vector space in the direct sum of two real vector spaces...

. As the involution

is antilinear, it cannot be the identity map on

.
Of course,

is a

-linear transformation of

, if one notes that every complex space V has a real form obtained by taking the same vector

Vector

Vector, a Latin word meaning "carrier", may refer in English to:-In computer science:*A one-dimensional array**Vector , a data type in the C++ Standard Template Library...

s as in the original set

Set

A set is a collection of well defined and distinct objects, considered as an object in its own right. Sets are one of the most fundamental concepts in mathematics. Developed at the end of the 19th century, set theory is now a ubiquitous part of mathematics, and can be used as a foundation from...

and restricting the scalars to be real. The above properties actually define a real structure

Real structure

In mathematics, a real structure on a complex vector space is a way to decompose the complex vector space in the direct sum of two real vector spaces...

on the complex vector space

.
One example of this notion is the conjugate transpose operation of complex matrices defined above. It should be remarked that on generic complex vector spaces there is no canonical
Canonical
Canonical is an adjective derived from canon. Canon comes from the greek word κανών kanon, "rule" or "measuring stick" , and is used in various meanings....

notion of complex conjugation.

The source of this article is wikipedia, the free encyclopedia. The text of this article is licensed under the GFDL.