Equioscillation theorem
Encyclopedia
The equioscillation theorem concerns the approximation
of continuous function
s using polynomial
s when the merit function is the maximum difference (uniform norm). Its discovery is attributed to Chebyshev
.
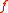be a continuous function from 
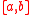to 
. Among all the polynomials of degree 
, the polynomial 
minimizes the uniform norm of the difference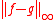 
if and only if there are 
points 
such that 
where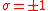 
.
s are available, the most common being the Remez algorithm
.
Approximation theory
In mathematics, approximation theory is concerned with how functions can best be approximated with simpler functions, and with quantitatively characterizing the errors introduced thereby...
of continuous function
Continuous function
In mathematics, a continuous function is a function for which, intuitively, "small" changes in the input result in "small" changes in the output. Otherwise, a function is said to be "discontinuous". A continuous function with a continuous inverse function is called "bicontinuous".Continuity of...
s using polynomial
Polynomial
In mathematics, a polynomial is an expression of finite length constructed from variables and constants, using only the operations of addition, subtraction, multiplication, and non-negative integer exponents...
s when the merit function is the maximum difference (uniform norm). Its discovery is attributed to Chebyshev
Pafnuty Chebyshev
Pafnuty Lvovich Chebyshev was a Russian mathematician. His name can be alternatively transliterated as Chebychev, Chebysheff, Chebyshov, Tschebyshev, Tchebycheff, or Tschebyscheff .-Early years:One of nine children, Chebyshev was born in the village of Okatovo in the district of Borovsk,...
.
Statement
Let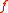 be a continuous function from 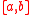to . Among all the polynomials of degree , the polynomial minimizes the uniform norm of the difference 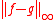if and only if there are points such that where 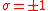.Algorithms
Several minimax approximation algorithmMinimax approximation algorithm
Polynomial expansions such as the Taylor series expansion are often convenient for theoretical work but less useful for practical applications. For practical work it is often desirable to minimize the maximum absolute or relative error of a polynomial fit for any given number of terms in an effort...
s are available, the most common being the Remez algorithm
Remez algorithm
The Remez algorithm , published by Evgeny Yakovlevich Remez in 1934 is an iterative algorithm used to find simple approximations to functions, specifically, approximations by functions in a Chebyshev space that are the best in the uniform norm L∞ sense.A typical...
.