Fluxon
Encyclopedia
In physics
, a fluxon is a quantum
of electromagnetic flux
. The term may have any of several related meanings.
, a fluxon (aka Abrikosov vortex
) is a small whisker of normal phase surrounded by superconducting phase. Supercurrent
s circulate around its center. The magnetic field through such a whisker and its neighborhood, which has size of the order of London penetration depth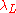
(~100 nm), is quantized because of the phase properties of the magnetic
vector potential
in quantum electrodynamics
.
In the context of long Josephson junction
s, a fluxon (aka Josephson vortex
) is made of circulating supercurrent
s and has no normal core. Supercurrents circulate just around the mathematical center of a fluxon. Again, the magnetic flux created by circulating supercurrent
s is equal to a magnetic flux quantum
.
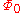modeling, a fluxon is a discretized magnetic field line, representing a finite amount of magnetic flux in a localized bundle in the model. Fluxon models are explicitly designed to preserve the topology
of the magnetic field, overcoming numerical resistivity
effects in Eulerian models
.
Physics
Physics is a natural science that involves the study of matter and its motion through spacetime, along with related concepts such as energy and force. More broadly, it is the general analysis of nature, conducted in order to understand how the universe behaves.Physics is one of the oldest academic...
, a fluxon is a quantum
Quantum
In physics, a quantum is the minimum amount of any physical entity involved in an interaction. Behind this, one finds the fundamental notion that a physical property may be "quantized," referred to as "the hypothesis of quantization". This means that the magnitude can take on only certain discrete...
of electromagnetic flux
Electromagnetic flux
Electromagnetic flux may refer to one of the following:*Flux or flux density of electromagnetic radiation*Electric flux and magnetic flux....
. The term may have any of several related meanings.
Superconductivity
In the context of superconductivitySuperconductivity
Superconductivity is a phenomenon of exactly zero electrical resistance occurring in certain materials below a characteristic temperature. It was discovered by Heike Kamerlingh Onnes on April 8, 1911 in Leiden. Like ferromagnetism and atomic spectral lines, superconductivity is a quantum...
, a fluxon (aka Abrikosov vortex
Abrikosov vortex
In superconductivity, an Abrikosov vortex is a vortex of supercurrent in a type-II superconductor. The supercurrent circulates around the normal core of the vortex. The core has a size \sim\xi — the superconducting coherence length...
) is a small whisker of normal phase surrounded by superconducting phase. Supercurrent
Supercurrent
A supercurrent is a superconducting current, that is, electric current which flows without dissipation....
s circulate around its center. The magnetic field through such a whisker and its neighborhood, which has size of the order of London penetration depth
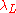(~100 nm), is quantized because of the phase properties of the magneticMagnetic potential
The term magnetic potential can be used for either of two quantities in classical electromagnetism: the magnetic vector potential, A, and the magnetic scalar potential, ψ...
vector potential
Vector potential
In vector calculus, a vector potential is a vector field whose curl is a given vector field. This is analogous to a scalar potential, which is a scalar field whose negative gradient is a given vector field....
in quantum electrodynamics
Quantum electrodynamics
Quantum electrodynamics is the relativistic quantum field theory of electrodynamics. In essence, it describes how light and matter interact and is the first theory where full agreement between quantum mechanics and special relativity is achieved...
.
In the context of long Josephson junction
Long Josephson junction
In superconductivity, a long Josephson junction is a Josephson junction which has one or more dimensions longer than the Josephson penetration depth \lambda_J. This definition is not strict....
s, a fluxon (aka Josephson vortex
Josephson vortex
In superconductivity, a Josephson vortex is a vortex of supercurrent in a long Josephson junction . The supercurrents circulate around the vortex center which is situated inside the Josephson barrier...
) is made of circulating supercurrent
Supercurrent
A supercurrent is a superconducting current, that is, electric current which flows without dissipation....
s and has no normal core. Supercurrents circulate just around the mathematical center of a fluxon. Again, the magnetic flux created by circulating supercurrent
Supercurrent
A supercurrent is a superconducting current, that is, electric current which flows without dissipation....
s is equal to a magnetic flux quantum
Magnetic flux quantum
The magnetic flux quantum Φ0 is the quantum of magnetic flux passing through a superconductor. The phenomenon of flux quantization was discovered B. S. Deaver and W. M. Fairbank and, independently, by R. Doll and M. Nabauer, in 1961...
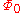.Magnetohydrodynamics modeling
In the context of numerical MHDMagnetohydrodynamics
Magnetohydrodynamics is an academic discipline which studies the dynamics of electrically conducting fluids. Examples of such fluids include plasmas, liquid metals, and salt water or electrolytes...
modeling, a fluxon is a discretized magnetic field line, representing a finite amount of magnetic flux in a localized bundle in the model. Fluxon models are explicitly designed to preserve the topology
Topology
Topology is a major area of mathematics concerned with properties that are preserved under continuous deformations of objects, such as deformations that involve stretching, but no tearing or gluing...
of the magnetic field, overcoming numerical resistivity
Numerical resistivity
Numerical resistivity is a problem in computer simulations of ideal magnetohydrodynamics . It is a form of numerical diffusion. In near-ideal MHD systems, the magnetic field can diffuse only very slowly through the plasma or fluid of the system; it is rate-limited by the resistivity of the fluid...
effects in Eulerian models
Lagrangian and Eulerian coordinates
In fluid dynamics and finite-deformation plasticity the Lagrangian specification of the flow field is a way of looking at fluid motion where the observer follows an individual fluid parcel as it moves through space and time. Plotting the position of an individual parcel through time gives the...
.