
Hirzebruch surface
Encyclopedia
In mathematics, a Hirzebruch surface is a ruled surface
over the projective line
. They were studied by .
Σn is the P1 bundle over P1
associated to the sheaf
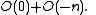
The notation here means: O(n) is the n-th tensor power of the Serre twist sheaf O(1), the invertible sheaf
or line bundle
with associated Cartier divisor a single point. The surface Σ0 is isomorphic to P1×P1, and Σ1 is isomorphic to P2 blown up at a point so is not minimal.
the projective bundle of O(-n) and the curve C is the zero section. This curve has self-intersection number −n, and is the only irreducible curve with negative self intersection number. The only irreducible curves with zero self intersection number are the fibers of the Hirzebruch surface (considered as a fiber bundle over P1). The Picard group is generated by the curve C and one of the fibers, and these generators have intersection matrix
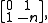
so the bilinear form is two dimensional unimodular, and is even or odd depending on whether n is even or odd.
The Hirzebruch surface Σn (n > 1) blown up at a point on the special curve C is isomorphic to Σn-1 blown up at a point not on the special curve.
Ruled surface
In geometry, a surface S is ruled if through every point of S there is a straight line that lies on S. The most familiar examples are the plane and the curved surface of a cylinder or cone...
over the projective line
Projective line
In mathematics, a projective line is a one-dimensional projective space. The projective line over a field K, denoted P1, may be defined as the set of one-dimensional subspaces of the two-dimensional vector space K2 .For the generalisation to the projective line over an associative ring, see...
. They were studied by .
Definition
The Hirzebruch surfaceΣn is the P1 bundle over P1
associated to the sheaf
Sheaf (mathematics)
In mathematics, a sheaf is a tool for systematically tracking locally defined data attached to the open sets of a topological space. The data can be restricted to smaller open sets, and the data assigned to an open set is equivalent to all collections of compatible data assigned to collections of...
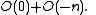
The notation here means: O(n) is the n-th tensor power of the Serre twist sheaf O(1), the invertible sheaf
Invertible sheaf
In mathematics, an invertible sheaf is a coherent sheaf S on a ringed space X, for which there is an inverse T with respect to tensor product of OX-modules. It is the equivalent in algebraic geometry of the topological notion of a line bundle...
or line bundle
Line bundle
In mathematics, a line bundle expresses the concept of a line that varies from point to point of a space. For example a curve in the plane having a tangent line at each point determines a varying line: the tangent bundle is a way of organising these...
with associated Cartier divisor a single point. The surface Σ0 is isomorphic to P1×P1, and Σ1 is isomorphic to P2 blown up at a point so is not minimal.
Properties
Hirzebruch surfaces for n>0 have a special rational curve C on them: The surface isthe projective bundle of O(-n) and the curve C is the zero section. This curve has self-intersection number −n, and is the only irreducible curve with negative self intersection number. The only irreducible curves with zero self intersection number are the fibers of the Hirzebruch surface (considered as a fiber bundle over P1). The Picard group is generated by the curve C and one of the fibers, and these generators have intersection matrix
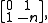
so the bilinear form is two dimensional unimodular, and is even or odd depending on whether n is even or odd.
The Hirzebruch surface Σn (n > 1) blown up at a point on the special curve C is isomorphic to Σn-1 blown up at a point not on the special curve.

